We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We continue the study of the boundedness of the operator
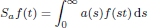
on the set of decreasing functions in Lp(w). This operator was first introduced by Braverman and Lai and also studied by Andersen, and although the weighted weak-type estimate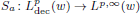 
was completely solved, the characterization of the weights w such that 
is bounded is still open for the case in which p > 1. The solution of this problem will have applications in the study of the boundedness on weighted Lorentz spaces of important operators in harmonic analysis.
