A carousel is an automated warehousing system consisting of a large number of drawers rotating in a closed loop. In this paper, we study the travel time needed to pick a list of items when the carousel operates under the nearest item heuristic. We find a closed form expression for the distribution and all moments of the travel time. We also analyse the asymptotic behaviour of the travel time when the number of items tends to infinity. All results follow from probabilistic arguments based on properties of uniform order statistics.

Explicit evaluations of the symmetric Euler integral $\int _{0}^{1}\,{{u}^{\alpha }}{{(1-u)}^{\alpha }}f(u)\,du$ are obtained for some particular functions $f$. These evaluations are related to duplication formulae for Appell’s hypergeometric function 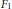${{F}_{1}}$ which give reductions of 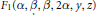${{F}_{1}}(\alpha ,\beta ,\beta ,2\alpha ,y,z)$ in terms of more elementary functions for arbitrary 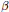$\beta $ with 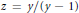$z=y/(y-1)$ and for 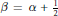$\beta =\alpha +\frac{1}{2}$ with arbitrary $y,z$. These duplication formulae generalize the evaluations of some symmetric Euler integrals implied by the following result: if a standard Brownian bridge is sampled at time 0, time 1, and at $n$ independent randomtimes with uniformdistribution on 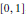$[0,1]$, then the broken line approximation to the bridge obtained from these 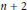$n+2$ values has a total variation whose mean square is 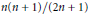$n(n+1)/(2n+1)$.