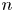Let $p$ be a prime number. For a positive integer $n$ and a real number $\xi$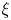, let $\lambda _n (\xi )$ 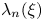denote the supremum of the real numbers $\lambda$ 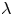for which there are infinitely many integer tuples $(x_0, x_1, \ldots , x_n)$ 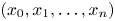such that $| x_0 \xi - x_1|_p, \ldots , | x_0 \xi ^{n} - x_n|_p$ 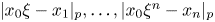are all less than $X^{-\lambda - 1}$, where $X$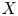 is the maximum of $|x_0|, |x_1|, \ldots , |x_n|$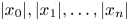. We establish new results on the Hausdorff dimension of the set of real numbers $\xi$ 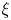for which $\lambda _n (\xi )$ 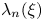is equal to (or greater than or equal to) a given value.

For the approximation in Lp-norm, we determine the weakly asymptotic orders for the simultaneous approximation errors of Sobolev classes by piecewise cubic Hermite interpolation with equidistant knots. For p = 1, ∞, we obtain its values. By these results we know that for the Sobolev classes, the approximation errors by piecewise cubic Hermite interpolation are weakly equivalent to the corresponding infinite-dimensional Kolmogorov widths. At the same time, the approximation errors of derivatives are weakly equivalent to the corresponding infinite-dimensional Kolmogorov widths.

In 1969, H. Davenport and W. M. Schmidt studied the problem of approximation to a real number $\xi$ by algebraic integers of degree at most 3. They did so, using geometry of numbers, by resorting to the dual problem of finding simultaneous approximations to $\xi$ and $\xi^2$ by rational numbers with the same denominator. In this paper, we show that their measure of approximation for the dual problem is optimal and that it is realized for a countable set of real numbers $\xi$. We give several properties of these numbers including measures of approximation by rational numbers, by quadratic real numbers and by algebraic integers of degree at most 3.