We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let 
$A$ be a separable amenable purely infinite simple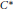 
${{C}^{*}}$-algebra which satisfies the Universal Coefficient Theorem. We prove that $A$ is weakly semiprojective if and only if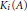 
${{K}_{i}}(A\text{)}$ is a countable direct sum of finitely generated groups 
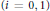$\left( i\,=\,0,\,1 \right)$. Therefore, if $A$ is such a 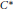${{C}^{*}}$-algebra, for any 
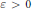$\varepsilon \,>\,0$ and any finite subset 
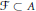$\mathcal{F}\,\subset \,A$ there exist 
$\delta \,>\,0$ and a finite subset 
$G\,\subset \,A$ satisfying the following: for any contractive positive linear map 
$L\,:\,A\,\to \,B$ (for any 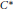${{C}^{*}}$-algebra 
$B$) with 
$||L\left( ab \right)\,-\,L\left( a \right)L\left( b \right)||\,<\,\delta$ for 
$a,\,b\,\in \,\mathcal{G}$ there exists a homomorphism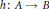 
$h:\,A\,\to \,B$ such that 
$||\,h\left( a \right)\,-\,L\left( a \right)||\,<\,\varepsilon$ for 
$a\,\in \,\mathcal{F}$.
