Let $R$ be a prime ring of characteristic diòerent from $2$, let 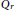${{Q}_{r}}$ be its right Martindale quotient ring, and let $C$ be its extended centroid. Suppose that $F$ is a generalized skew derivation of $R,\,L$ a non-central Lie ideal of 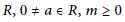$R,\,0\,\ne \,a\,\in \,R,\,m\,\ge \,0$ and 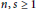$n,\,s\,\ge \,1$ fixed integers. If

$$a{{\left( {{u}^{m}}F\left( u \right){{u}^{n}} \right)}^{s}}\,=\,0$$

for all $u\,\in \,L$, then either $R\,\subseteq \,{{M}_{2}}\left( C \right)$, the ring of $2\,\times \,2$ matrices over $C$, or $m\,=\,0$ and there exists $b\,\in \,{{Q}_{r}}$ such that $F\left( x \right)\,=\,bx$, for any $x\,\in \,R$, with $ab\,=\,0$.

In this paper we prove the following result: let $m,n\geq 1$ be distinct integers, let $R$ be an $mn(m+n)|m-n|$-torsion free semiprime ring and let $D:R\rightarrow R$ be an $(m,n)$-Jordan derivation, that is an additive mapping satisfying the relation $(m+n)D(x^{2})=2mD(x)x+2nxD(x)$ for $x\in R$. Then $D$ is a derivation which maps $R$ into its centre.

Let $R$ be a ring and let $g$ be an endomorphism of $R$. The additive mapping $d:\,R\,\to \,R$ is called a Jordan semiderivation of $R$, associated with $g$, if

$$d\left( {{x}^{2}} \right)=d\left( x \right)x+g\left( x \right)d\left( x \right)=d\left( x \right)g\left( x \right)+xd\left( x \right)\,\text{and}\,d\left( g\left( x \right) \right)=g\left( d\left( x \right) \right)$$

for all $x\,\in \,R$. The additive mapping $F:\,R\,\to \,R$ is called a generalized Jordan semiderivation of $R$, related to the Jordan semiderivation $d$ and endomorphism $g$, if

$$F\left( {{x}^{2}} \right)=F\left( x \right)x+g\left( x \right)d\left( x \right)=F\left( x \right)g\left( x \right)+xd\left( x \right)\,\,and\,F\left( g\left( x \right) \right)=g\left( F\left( x \right) \right)$$

for all $x\,\in \,R$. In this paper we prove that if $R$ is a prime ring of characteristic different from 2, $g$ an endomorphism of $R,\,d$ a Jordan semiderivation associated with $g,\,F$ a generalized Jordan semiderivation associated with $d$ and $g$, then $F$ is a generalized semiderivation of $R$ and $d$ is a semiderivation of $R$. Moreover, if $R$ is commutative, then $F\,=\,d$.

Let $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}R$ be a semiprime ring with extended centroid $C$ and with maximal right ring of quotients $Q_{mr}(R)$. Let $d{:}\ R\to Q_{mr}(R)$ be an additive map and $b\in Q_{mr}(R)$. An additive map $\delta {:}\ R\to Q_{mr}(R)$ is called a (left) $b$-generalized derivation with associated map $d$ if $\delta (xy)=\delta (x)y+bxd(y)$ for all $x, y\in R$. This gives a unified viewpoint of derivations, generalized derivations and generalized $\sigma $-derivations with an X-inner automorphism $\sigma $. We give a complete characterization of $b$-generalized derivations of $R$ having nilpotent values of bounded index. This extends several known results in the literature.

Let R be a prime ring, let I be a nonzero ideal of R and let n be a fixed positive integer. We prove that if the characteristic of R is either 0 or a prime p that is greater than 2n, then an additive map d that satisfies d(xn+1)=∑ nj=0xn−jd(x)xj for all x∈I must be a derivation.

Let K be a commutative ring with unity, R a prime K-algebra of characteristic different from 2, d and δ non-zero derivations of R, f (x1,…, xn) a multilinear polynomial over K.If

then f(x1,…,xnis central-valued on R.

Let R be a prime ring with extended centroid C, $\rho$ a non-zero right ideal of R and let $f(X_1,\dots,X_t)$ be a polynomial, having no constant term, over C. Suppose that $f(X_1,\dots,X_t)$ is not central-valued on RC. We denote by $f(\rho)$ the additive subgroup of RC> generated by all elements $f(x_1,\dots,x_t)$ for $x_i\in\rho$. The main goals of this note are to prove two results concerning the extension properties of finiteness conditions as follows.

(I) If $f(\rho)$ spans a non-zero finite-dimensional $C$-subspace of $RC$, then $\dim_CRC$ is finite.

(II) If $f(\rho)\ne0$ and is a finite set, then $R$ itself is a finite ring.

AMS 2000 Mathematics subject classification: Primary 16N60; 16R50

We present an operator algebraic approach to Wigner's unitary-antiunitary theorem using some classical results from ring theory. To show how effective this approach is, we prove a generalization of this celebrated theorem for Hilbert modules over matrix algebras. We also present a Wigner-type result for maps on prime C*-algebras.

Let R be a prime ring of characteristic not 2 and d a derivation of R. It is shown that if d2n is a derivation of R, where n is a positive integer, then d2n-1 = 0.

In this paper we prove algebraic generalizations of some results of C. J. K. Batty and A. B. Thaheem, concerned with the identity α + α−1 = β + β−1 where α and β are automorphisms of a C*-algebra. The main result asserts that if automorphisms α, β of a semiprime ring R satisfy α + α-1 = β + β−1 then there exist invariant ideals U1, U2 and U3 of R such that Ui ∩ Uj = 0, i ≠ j, U1 ⊕ U2 ⊕ U3 is an essential ideal, α = β on U1, α = β−1 on U2, and α2 = β2 = α−2 on U3. Furthermore, if the annihilator of any ideal in R is a direct summand (in particular, if R is a von Neumann algebra), then U1 ⊕ U2 ⊕ U3 = R.

Let D be a nonzero derivation of a noncommutative prime ring R, and let U be the subring of R generated by all [D(x), x], x ∞ R. A classical theorem of Posner asserts that U is not contained in the center of R. Under the additional assumption that the characteristic of R is not 2, we prove a more general result stating that U contains a nonzero left ideal of R as well as a nonzero right ideal of R.