We investigate mean convergence of Lagrange interpolation at the zeros of orthogonal polynomials pn(W2, x) for Erdös weights W2 = e-2Q. The archetypal example is Wk,α = exp(—Qk,α), where

α > 1, k ≥ 1, and is the k-th iterated exponential. Following is our main result: Let 1 < p < ∞, Δ ∊ ℝ, k > 0. Let Ln[f] denote the Lagrange interpolation polynomial to ƒ at the zeros of pn(W2, x) = pn(e-2Q, x). Then for

to hold for every continuous function ƒ: ℝ —> ℝ satisfying

it is necessary and sufficient that

We complete our investigations of mean convergence of Lagrange interpolation at the zeros of orthogonal polynomials pn(W2, x) for Erdős weights W2 = e-2Q. The archetypal example is Wk,α = exp(—Qk,α), where

α > 1, k ≥ 1, and 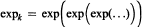is the k-th iterated exponential. Following is our main result: Let 1 < p < 4 and α ∊ ℝ Let Ln[f] denote the Lagrange interpolation polynomial to ƒ at the zeros of pn(W2, x) = pn(e-2Q, x). Then for

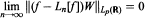
to hold for every continuous function ƒ:ℝ. —> ℝ satisfying

it is necessary and sufficient that α > 1/p. This is, essentially, an extension of the Erdös-Turan theorem on L2 convergence. In an earlier paper, we analyzed convergence for all p > 1, showing the necessity and sufficiency of using the weighting factor 1 + Q for all p > 4. Our proofs of convergence are based on converse quadrature sum estimates, that are established using methods of H. König.

Weighted LP mean convergence of Hermite-Fejér interpolation based on the zeros of orthogonal polynomials with respect to the weight |x|2α+1(l — x2)β(α, β > — 1) is investigated. A necessary and sufficient condition for such convergence for all continuous functions is given. Meanwhile divergence of Hermite-Fejér interpolation in LP with p > 2 is obtained. This gives a possible answer to Problem 17 of P. Turân [J. Approx. Theory, 29(1980), p. 40].