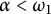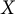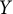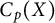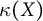Let $\alpha < \omega _1$ be a prime component, and let $X$ and $Y$ be metric spaces. In [8], it was shown that if $C_p(X)$ and $C_p(Y)$ are linearly homeomorphic, then the scattered heights $\kappa (X)$ and 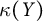$\kappa (Y)$ of 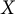$X$ and 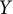$Y$ satisfy 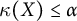$\kappa (X) \leq \alpha $ if and only if 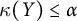$\kappa (Y) \leq \alpha $. We will prove that this also holds if 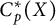$C_p^*(X)$ and 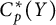$C_p^*(Y)$ are linearly homeomorphic and that these results do not hold for arbitrary Tychonov spaces. We will also prove that if $C_p^*(X)$ and 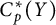$C_p^*(Y)$ are linearly homeomorphic, then $\kappa (X) < \alpha $ if and only if 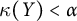$\kappa (Y) < \alpha $, which was shown in [9] for $\alpha = \omega $. This last statement is not always true for linearly homeomorphic 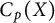$C_p(X)$ and 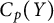$C_p(Y)$. We will show that if 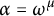$\alpha = \omega ^{\mu }$ where $\mu < \omega _1$ is a successor ordinal, it is true, but for all other prime components, this is not the case. Finally, we will prove that if $C_p^*(X)$ and 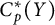$C_p^*(Y)$ are linearly homeomorphic, then $X$ is scattered if and only if 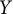$Y$ is scattered. This result does not directly follow from the above results. We will clarify why the results for linearly homeomorphic spaces 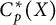$C_p^*(X)$ and 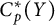$C_p^*(Y)$ do require a different and more complex approach than the one that was used for linearly homeomorphic spaces $C_p(X)$ and $C_p(Y)$.