We prove the analog of the Morel–Voevodsky localization theorem for framed motivic spaces. We deduce that framed motivic spectra are equivalent to motivic spectra over arbitrary schemes, and we give a new construction of the motivic cohomology of arbitrary schemes.

In the joint work with Elmanto, Hoyois, Khan and Sosnilo, we computed infinite $\mathbb{P}^{1}$-loop spaces of motivic Thom spectra using the technique of framed correspondences. This result allows us to express non-negative $\mathbb{G}_{m}$-homotopy groups of motivic Thom spectra in terms of geometric generators and relations. Using this explicit description, we show that the unit map of the algebraic special linear cobordism spectrum induces an isomorphism on $\mathbb{G}_{m}$-homotopy sheaves.

Given a field $k$ of characteristic zero and 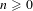$n\geqslant 0$, we prove that 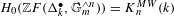$H_{0}(\mathbb{Z}F(\unicode[STIX]{x1D6E5}_{k}^{\bullet },\mathbb{G}_{m}^{\wedge n}))=K_{n}^{MW}(k)$, where 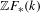$\mathbb{Z}F_{\ast }(k)$ is the category of linear framed correspondences of algebraic 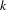$k$-varieties, introduced by Garkusha and Panin [The triangulated category of linear framed motives $DM_{fr}^{eff}(k)$, in preparation] (see [Garkusha and Panin, Framed motives of algebraic varieties (after V. Voevodsky), Preprint, 2014, arXiv:1409.4372], § 7 as well), and 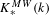$K_{\ast }^{MW}(k)$ is the Milnor–Witt 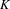$K$-theory of the base field 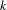$k$.