We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper, we investigate the two-dimensional shrinking target problem in beta-dynamical systems. Let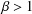 
$\unicode[STIX]{x1D6FD}>1$ be a real number and define the 
$\unicode[STIX]{x1D6FD}$-transformation on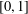 
$[0,1]$ by 
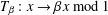$T_{\unicode[STIX]{x1D6FD}}:x\rightarrow \unicode[STIX]{x1D6FD}x\;\text{mod}\;1$. Let 
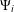$\unicode[STIX]{x1D6F9}_{i}$ (
$i=1,2$) be two positive functions on 
$\mathbb{N}$ such that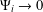 
$\unicode[STIX]{x1D6F9}_{i}\rightarrow 0$ when 
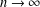$n\rightarrow \infty$. We determine the Lebesgue measure and Hausdorff dimension for the 
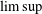$\limsup$ set for any fixed 
$$\begin{eqnarray}W(T_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6F9}_{1},\unicode[STIX]{x1D6F9}_{2})=\{(x,y)\in [0,1]^{2}:|T_{\unicode[STIX]{x1D6FD}}^{n}x-x_{0}|<\unicode[STIX]{x1D6F9}_{1}(n),|T_{\unicode[STIX]{x1D6FD}}^{n}y-y_{0}|<\unicode[STIX]{x1D6F9}_{2}(n)\text{ for infinitely many }n\in \mathbb{N}\}\end{eqnarray}$$
$x_{0},y_{0}\in [0,1]$.
