A new oscillation criterion is given for the delay differential equation 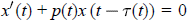 ${x}'\,(t)\,+\,p(t)x\,(t\,-\,\text{ }\!\!\tau\!\!\text{ (t)})\,=\,0$, where
${x}'\,(t)\,+\,p(t)x\,(t\,-\,\text{ }\!\!\tau\!\!\text{ (t)})\,=\,0$, where 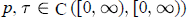 $p,\,\text{ }\!\!\tau\!\!\text{ }\,\in \,\text{C}\,\text{( }\!\ $T$ defined by
$T$ defined by  $T(t)\,=\,t-\,\text{ }\!\!\tau\!\!\text{ }\,\text{(t),}\,\text{t}\ge \,\text{0}$ is increasing and such that
$T(t)\,=\,t-\,\text{ }\!\!\tau\!\!\text{ }\,\text{(t),}\,\text{t}\ge \,\text{0}$ is increasing and such that 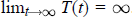 ${{\lim }_{t\to \infty }}\,T(t)\,=\,\infty $. This criterion concerns the case where
${{\lim }_{t\to \infty }}\,T(t)\,=\,\infty $. This criterion concerns the case where  $\lim \,{{\inf }_{t\to \infty }}\int _{T(t)}^{t}p(s)ds\le \frac{1}{e}$.
$\lim \,{{\inf }_{t\to \infty }}\int _{T(t)}^{t}p(s)ds\le \frac{1}{e}$.