We study the semilinear Schrödinger equation
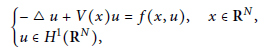 $$\left\{ _{u\,\,\in \,\,{{H}^{1}}({{\mathbf{R}}^{N}}),}^{-\Delta \,u+V(x)u=f(x,u),\,\,\,\,\,x\in \,\,{{\mathbf{R}}^{N}},} \right.$$
$$\left\{ _{u\,\,\in \,\,{{H}^{1}}({{\mathbf{R}}^{N}}),}^{-\Delta \,u+V(x)u=f(x,u),\,\,\,\,\,x\in \,\,{{\mathbf{R}}^{N}},} \right.$$
where 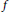 $f$ is a superlinear, subcritical nonlinearity. It focuses on the case where
$f$ is a superlinear, subcritical nonlinearity. It focuses on the case where 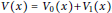 $V(x)={{V}_{0}}(x)+{{V}_{1}}(x)$,
$V(x)={{V}_{0}}(x)+{{V}_{1}}(x)$, 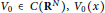 ${{V}_{0}}\in C({{\mathbf{R}}^{N}}),\,{{V}_{0}}(x)$ is 1-periodic in each of
${{V}_{0}}\in C({{\mathbf{R}}^{N}}),\,{{V}_{0}}(x)$ is 1-periodic in each of 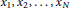 ${{x}_{1}},{{x}_{2}},...,{{x}_{N}}$,
${{x}_{1}},{{x}_{2}},...,{{x}_{N}}$, 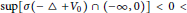 $\sup [\sigma (-\Delta +{{V}_{0}})\,\cap \,(-\infty ,0)]<0<$
$\sup [\sigma (-\Delta +{{V}_{0}})\,\cap \,(-\infty ,0)]<0<$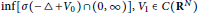 $\inf [\sigma (-\Delta +{{V}_{0}})\cap (0,\infty )],\,{{V}_{1}}\in C({{\mathbf{R}}^{N}})$, and
$\inf [\sigma (-\Delta +{{V}_{0}})\cap (0,\infty )],\,{{V}_{1}}\in C({{\mathbf{R}}^{N}})$, and 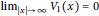 ${{\lim }_{|x|\to \infty }}\,{{V}_{1}}(x)=0$. A new super-quadratic condition is obtained that is weaker than some well-known results.
${{\lim }_{|x|\to \infty }}\,{{V}_{1}}(x)=0$. A new super-quadratic condition is obtained that is weaker than some well-known results.