Following ideas used by Drewnowski and Wilansky we prove that if  $I$ is an infinite dimensional and infinite codimensional closed ideal in a complete metrizable locally solid Riesz space and
$I$ is an infinite dimensional and infinite codimensional closed ideal in a complete metrizable locally solid Riesz space and  $I$ does not contain any order copy of
$I$ does not contain any order copy of 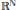 ${{\mathbb{R}}^{\mathbb{N}}}$ then there exists a closed, separable, discrete Riesz subspace
${{\mathbb{R}}^{\mathbb{N}}}$ then there exists a closed, separable, discrete Riesz subspace  $G$ such that the topology induced on
$G$ such that the topology induced on  $G$ is Lebesgue,
$G$ is Lebesgue, 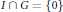 $I\,\bigcap \,G\,=\,\left\{ 0 \right\}$, and
$I\,\bigcap \,G\,=\,\left\{ 0 \right\}$, and  $I\,+\,G$ is not closed.
$I\,+\,G$ is not closed.