Let 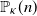 $\mathbb{P}_\kappa(n)$ be the probability that n points
$\mathbb{P}_\kappa(n)$ be the probability that n points 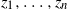 $z_1,\ldots,z_n$ picked uniformly and independently in
$z_1,\ldots,z_n$ picked uniformly and independently in 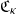 $\mathfrak{C}_\kappa$, a regular
$\mathfrak{C}_\kappa$, a regular  $\kappa$-gon with area 1, are in convex position, that is, form the vertex set of a convex polygon. In this paper, we compute
$\kappa$-gon with area 1, are in convex position, that is, form the vertex set of a convex polygon. In this paper, we compute 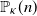 $\mathbb{P}_\kappa(n)$ up to asymptotic equivalence, as
$\mathbb{P}_\kappa(n)$ up to asymptotic equivalence, as 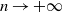 $n\to+\infty$, for all
$n\to+\infty$, for all 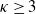 $\kappa\geq 3$, which improves on a famous result of Bárány (Ann. Prob. 27, 1999). The second purpose of this paper is to establish a limit theorem which describes the fluctuations around the limit shape of an n-tuple of points in convex position when
$\kappa\geq 3$, which improves on a famous result of Bárány (Ann. Prob. 27, 1999). The second purpose of this paper is to establish a limit theorem which describes the fluctuations around the limit shape of an n-tuple of points in convex position when  $n\to+\infty$. Finally, we give an asymptotically exact algorithm for the random generation of
$n\to+\infty$. Finally, we give an asymptotically exact algorithm for the random generation of 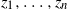 $z_1,\ldots,z_n$, conditioned to be in convex position in
$z_1,\ldots,z_n$, conditioned to be in convex position in  $\mathfrak{C}_\kappa$.
$\mathfrak{C}_\kappa$.