We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Jean-Philippe Anker made an interesting conjecture in [2] about the growth of the heat kernel on symmetric spaces of noncompact type. For any symmetric space of noncompact type, we can write
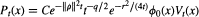
where ϕ0 is the Legendre function and q, "the dimension at infinity", is chosen such that limt—>∞Vt(x) = 1 for all x. Anker's conjecture can be stated as follows: there exists a constant C > 0 such that
where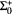 
is the set of positive indivisible roots. The behaviour of the function ϕ0 is well known (see [1]).
The main goal of this paper is to establish the conjecture for the spaces SU*(2n)/ Sp(n).
