We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Given a domain 
${\rm\Omega}$ of a complete Riemannian manifold 
${\mathcal{M}}$, define 
${\mathcal{A}}$ to be the Laplacian with Neumann boundary condition on 
${\rm\Omega}$. We prove that, under appropriate conditions, the corresponding heat kernel satisfies the Gaussian upper bound Here 
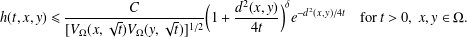$$\begin{eqnarray}h(t,x,y)\leq \frac{C}{[V_{{\rm\Omega}}(x,\sqrt{t})V_{{\rm\Omega}}(y,\sqrt{t})]^{1/2}}\biggl(1+\frac{d^{2}(x,y)}{4t}\biggr)^{{\it\delta}}e^{-d^{2}(x,y)/4t}\quad \text{for}~t>0,~x,y\in {\rm\Omega}.\end{eqnarray}$$
$d$ is the geodesic distance on 
${\mathcal{M}}$, 
$V_{{\rm\Omega}}(x,r)$ is the Riemannian volume of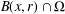 
$B(x,r)\cap {\rm\Omega}$, where 
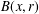$B(x,r)$ is the geodesic ball of centre 
$x$ and radius 
$r$, and 
${\it\delta}$ is a constant related to the doubling property of 
${\rm\Omega}$. As a consequence we obtain analyticity of the semigroup 
$e^{-t{\mathcal{A}}}$ on 
$L^{p}({\rm\Omega})$ for all 
$p\in [1,\infty )$ as well as a spectral multiplier result.
The growth properties at infinity for eigenfunctions corresponding to embedded eigenvalues of the Neumann Laplacian on horn-like domains are studied. For domains that pinch at polynomial rate, it is shown that the eigenfunctions vanish at infinity faster than the reciprocal of any polynomial. For a class of domains that pinch at an exponential rate, weaker, 
${{L}^{2}}$ bounds are proven. A corollary is that eigenvalues can accumulate only at zero or infinity.
