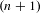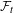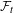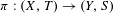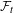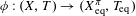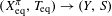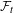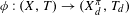This paper examines the distribution of V2 in Yiddish and its effects on extraction. Specifically, we show that both Spec-to-Spec antilocality (Erlewine 2020) and minimality (Rizzi 2006) constrain wh-extractions from embedded clauses in Yiddish. This explains the pattern of that-trace effects in Yiddish, as well as the apparent absence of an escape hatch in certain constructions.

Let $\unicode[STIX]{x1D70B}:(X,T)\rightarrow (Y,S)$ be an extension between minimal systems; we consider its relative sensitivity. We obtain that $\unicode[STIX]{x1D70B}$ is relatively $n$-sensitive if and only if the relative $n$-regionally proximal relation contains a point whose coordinates are distinct; and the structure of $\unicode[STIX]{x1D70B}$ which is relatively $n$-sensitive but not relatively 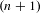$(n+1)$-sensitive is determined. Let ${\mathcal{F}}_{t}$ be the families consisting of thick sets. We introduce notions of relative block 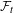${\mathcal{F}}_{t}$-sensitivity and relatively strong 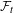${\mathcal{F}}_{t}$-sensitivity. Let 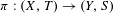$\unicode[STIX]{x1D70B}:(X,T)\rightarrow (Y,S)$ be an extension between minimal systems. Then the following Auslander–Yorke type dichotomy theorems are obtained: (1) $\unicode[STIX]{x1D70B}$ is either relatively block 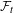${\mathcal{F}}_{t}$-sensitive or 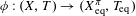$\unicode[STIX]{x1D719}:(X,T)\rightarrow (X_{\text{eq}}^{\unicode[STIX]{x1D70B}},T_{\text{eq}})$ is a proximal extension where 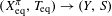$(X_{\text{eq}}^{\unicode[STIX]{x1D70B}},T_{\text{eq}})\rightarrow (Y,S)$ is the maximal equicontinuous factor of $\unicode[STIX]{x1D70B}$. (2) $\unicode[STIX]{x1D70B}$ is either relatively strongly 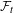${\mathcal{F}}_{t}$-sensitive or 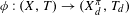$\unicode[STIX]{x1D719}:(X,T)\rightarrow (X_{d}^{\unicode[STIX]{x1D70B}},T_{d})$ is a proximal extension where $(X_{d}^{\unicode[STIX]{x1D70B}},T_{d})\rightarrow (Y,S)$ is the maximal distal factor of $\unicode[STIX]{x1D70B}$.

We extend the classical notion of an outer action $\alpha $ of a group $G$ on a unital ring $A$ to the case when $\alpha $ is a partial action on ideals, all of which have local units. We show that if $\alpha $ is an outer partial action of an abelian group $G$, then its associated partial skew group ring $A\,{{\star }_{\alpha }}\,G$ is simple if and only if $A$ is $G$-simple. This result is applied to partial skew group rings associated with two different types of partial dynamical systems.

A stationary stochastic process must satisfy various requirements to make it a realistic model for a phenomenon in the real world. Some of these requirements are quantitative, such as agreement of distribution or moments. Other, more qualitative requirements deal with the general behavior of the process. Two such requirements are non-singularity and asymptotic independence. Each will be discussed from a variety of points of view, and given precise definition in a succession of progressively stronger forms.