We consider a finite-dimensional control system $(\Sigma)\ \ \dot
x(t)=f(x(t),u(t))$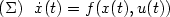 , such that there exists a feedback stabilizer k
that renders $\dot x=f(x,k(x))$
, such that there exists a feedback stabilizer k
that renders $\dot x=f(x,k(x))$ globally asymptotically
stable. Moreover, for (H,p,q) with H an output map and $1\leq
p\leq q\leq \infty$
globally asymptotically
stable. Moreover, for (H,p,q) with H an output map and $1\leq
p\leq q\leq \infty$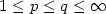 , we assume that there exists a ${\cal {K}}_{\infty}$
, we assume that there exists a ${\cal {K}}_{\infty}$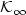 -function
α such that $\|H(x_u)\|_q\leq \alpha(\|u\|_p)$
-function
α such that $\|H(x_u)\|_q\leq \alpha(\|u\|_p)$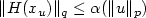 , where xu is the
maximal solution of $(\Sigma)_k \ \ \dot x(t)=f(x(t),k(x(t))+u(t))$
, where xu is the
maximal solution of $(\Sigma)_k \ \ \dot x(t)=f(x(t),k(x(t))+u(t))$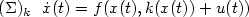 ,
corresponding to u and to the initial condition x(0)=0. Then, the
gain function $G_{(H,p,q)}$
,
corresponding to u and to the initial condition x(0)=0. Then, the
gain function $G_{(H,p,q)}$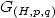 of (H,p,q) given by 14.5cm
$$
G_{(H,p,q)}(X)\stackrel{\rm def}{=}\sup_{\|u\|_p=X}\|H(x_u)\|_q,
$$
of (H,p,q) given by 14.5cm
$$
G_{(H,p,q)}(X)\stackrel{\rm def}{=}\sup_{\|u\|_p=X}\|H(x_u)\|_q,
$$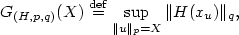 is well-defined. We call profile of k for (H,p,q) any
${\cal {K}}_{\infty}$
is well-defined. We call profile of k for (H,p,q) any
${\cal {K}}_{\infty}$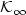 -function which is of the same order of magnitude
as $G_{(H,p,q)}$
-function which is of the same order of magnitude
as $G_{(H,p,q)}$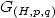 . For the double integrator subject to input saturation
and stabilized by
$k_L(x)=-(1\ 1)^Tx$
. For the double integrator subject to input saturation
and stabilized by
$k_L(x)=-(1\ 1)^Tx$ , we determine the profiles corresponding to the main
output maps. In particular, if $\sigma_0$
, we determine the profiles corresponding to the main
output maps. In particular, if $\sigma_0$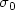 is used to denote the standard
saturation function, we show that the L2-gain from the output of the
saturation nonlinearity to u of the system $\ddot x=\sigma_0(-x-\dot x+u)$
is used to denote the standard
saturation function, we show that the L2-gain from the output of the
saturation nonlinearity to u of the system $\ddot x=\sigma_0(-x-\dot x+u)$ with $x(0)= \dot x(0)=0$
with $x(0)= \dot x(0)=0$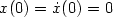 , is finite. We also provide a class of feedback
stabilizers kF that have a linear profile for (x,p,p), $1\leq p\leq \infty$
, is finite. We also provide a class of feedback
stabilizers kF that have a linear profile for (x,p,p), $1\leq p\leq \infty$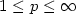 .
For instance,
we show that the L2-gains from x and $\dot x$
.
For instance,
we show that the L2-gains from x and $\dot x$ to u of the
system $\ddot x=\sigma_0(-x-\dot x-(\dot x)^3+u)$
to u of the
system $\ddot x=\sigma_0(-x-\dot x-(\dot x)^3+u)$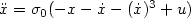 with $x(0)= \dot x(0)=0$
with $x(0)= \dot x(0)=0$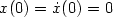 ,
are finite.
,
are finite.