We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let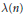 
$\text{ }\!\!\lambda\!\!\text{ }\left( n \right)$ denote the Liouville function. Complementary to the prime number theorem, Chowla
conjectured that
*
$$\sum\limits_{n\le x}{\lambda \,\left( f\left( n \right) \right)}=o\left( x \right)$$
for any polynomial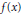 
$f\left( x \right)$ with integer coefficients which is not of form 
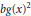$bg{{\left( x \right)}^{2}}$.
We investigate the behaviour of the function 
$L_{\alpha }(x) = \sum _{n\leq x}\lambda (n)/n^{\alpha }$, where 
$\lambda (n)$ is the Liouville function and 
$\alpha $ is a real parameter. The case where 
$\alpha =0$ was investigated by Pólya; the case 
$\alpha =1$, by Turán. The question of the existence of sign changes in both of these cases is related to the Riemann hypothesis. Using both analytic and computational methods, we investigate similar problems for the more general family 
$L_{\alpha }(x)$, where 
$0\leq \alpha \leq 1$, and their relationship to the Riemann hypothesis and other properties of the zeros of the Riemann zeta function. The case where 
$\alpha =1/2$is of particular interest.
