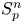The existence of isometric embedding of $S_q^m$ into $S_p^n$, where $1\leq p\neq q\leq \infty$ and $m,n\geq 2$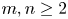, has been recently studied in [6]. In this article, we extend the study of isometric embeddability beyond the above-mentioned range of $p$ 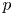and $q$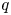. More precisely, we show that there is no isometric embedding of the commutative quasi-Banach space $\ell _q^m(\mathbb {R})$ 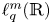into $\ell _p^n(\mathbb {R})$, where $(q,p)\in (0,\infty )\times (0,1)$ and $p\neq q$. As non-commutative quasi-Banach spaces, we show that there is no isometric embedding of $S_q^m$ into $S_p^n$, where $(q,p)\in (0,2)\setminus \{1\}\times (0,1)$ $\cup \, \{1\}\times (0,1)\setminus \left \{\!\frac {1}{n}:n\in \mathbb {N}\right \}$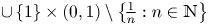 $\cup \, \{\infty \}\times (0,1)\setminus \left \{\!\frac {1}{n}:n\in \mathbb {N}\right \}$ 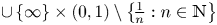and $p\neq q$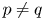. Moreover, in some restrictive cases, we also show that there is no isometric embedding of $S_q^m$ into $S_p^n$, where $(q,p)\in [2, \infty )\times (0,1)$. A new tool in our paper is the non-commutative Clarkson's inequality for Schatten class operators. Other tools involved are the Kato–Rellich theorem and multiple operator integrals in perturbation theory, followed by intricate computations involving power-series analysis.