Let G be a compact group, let $\mathcal {B}$ be a unital C$^*$-algebra, and let $(\mathcal {A},G,\alpha )$ be a free C$^*$-dynamical system, in the sense of Ellwood, with fixed point algebra $\mathcal {B}$. We prove that $(\mathcal {A},G,\alpha )$ can be realized as the G-continuous part of the invariants of an equivariant coaction of G on a corner of 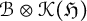$\mathcal {B} \otimes {\mathcal {K}}({\mathfrak {H}})$ for a certain Hilbert space ${\mathfrak {H}}$ that arises from the freeness of the action. This extends a result by Wassermann for free and ergodic C$^*$-dynamical systems. As an application, we show that any faithful $^*$-representation of $\mathcal {B}$ on a Hilbert space 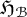${\mathfrak {H}}_{\mathcal {B}}$ gives rise to a faithful covariant representation of 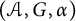$(\mathcal {A},G,\alpha )$ on some truncation of 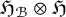${\mathfrak {H}}_{\mathcal {B}} \otimes {\mathfrak {H}}$.

The chance objection to incompatibilist accounts of free action maintains that undetermined actions are not under the agent's control. Some attempts to circumvent this objection locate chance in events posterior to the action. Indeterministic-causation theories locate chance in events prior to the action. However, neither type of response gives an account of free action which avoids the chance objection. Chance must be located at the act of will if actions are to be both undetermined and under the agent's control. This dissolves the apparent paradox of Frankfurt-type cases as well as the chance objection to incompatibilist free will.

It has been conjectured that if $G= \mathop{({ \mathbb{Z} }_{p} )}\nolimits ^{r} $ acts freely on a finite $CW$-complex $X$ which is homotopy equivalent to a product of spheres ${S}^{{n}_{1} } \times {S}^{{n}_{2} } \times \cdots \times {S}^{{n}_{k} } $, then $r\leq k$. We address this question with the relaxation that $X$ is finite-dimensional, and show that, to answer the question, it suffices to consider the case where the dimensions of the spheres are greater than or equal to $2$.