We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We consider the nonlinear Dirac equation
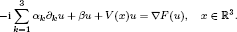
The potential function V satisfies the conditions that the essential spectrum of the Dirac operator 
is 
and this Dirac operator has infinitely many eigenvalues in (−1, 1) accumulating at 1. This potential function V may change sign in ℝ3 and contains the classical Coulomb potential V (x) = −γ/|x| with γ > 0 as a special case. The nonlinearity F satisfies the resonance-type condition lim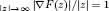
. Under some additional conditions on V and F, we prove that this equation has infinitely many solutions.
By making use of Merle's general shooting method we investigate Dirac equations of the form
Here it is possible that F(0) = −∞ and that F(s) defined on (0,+∞) is not monotonously nondecreasing. Our results cover some known ones as a special case.
