An efficient numerical method is proposed for the solution of Love's integral equation
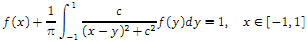
where c > 0 is a small parameter, by using a sinc Nyström method based on a double exponential transformation. The method is derived using the property that the solution ƒ(x) of Love's integral equation satisfies ƒ (x) → 0.5 for x ∈ (−1, 1) when the parameter c → 0. Numerical results show that the proposed method is very efficient.