Given an open, bounded set $\Omega $ in $\mathbb {R}^N$, we consider the minimization of the anisotropic Cheeger constant 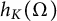$h_K(\Omega )$ with respect to the anisotropy K, under a volume constraint on the associated unit ball. In the planar case, under the assumption that K is a convex, centrally symmetric body, we prove the existence of a minimizer. Moreover, if $\Omega $ is a ball, we show that the optimal anisotropy K is not a ball and that, among all regular polygons, the square provides the minimal value.

We prove a reverse Faber–Krahn inequality for the Cheeger constant, stating that every convex body in ℝ2 has an affine image such that the product between its Cheeger constant and the square root of its area is not larger than the same quantity for the regular triangle. An analogous result holds for centrally symmetric convex bodies with the regular triangle replaced by the square. We also prove a Mahler-type inequality for the Cheeger constant, stating that every axisymmetric convex body in ℝ2 has a linear image such that the product between its Cheeger constant and the Cheeger constant of its polar body is not larger than the same quantity for the square.

We construct Cheeger-type bounds for the second eigenvalue of a substochastic transition probability matrix in terms of the Markov chain's conductance and metastability (and vice versa) with respect to its quasistationary distribution, extending classical results for stochastic transition matrices.

This article deals with the numerical computation of the Cheeger constant and the approximation of the maximal Cheeger set of a given subset of ${\mathbb R}^d$ . This problem is motivated by landslide modelling as well as by the continuous maximal flow problem. Using the fact that the maximal Cheeger set can be approximated by solving a rather simple projection problem, we propose a numerical strategy to compute maximal Cheeger sets and Cheeger constants.

We compare dependence in stochastically monotone Markov processes with partially ordered Polish state spaces using the concordance and supermodular orders. We show necessary and sufficient conditions for the concordance order to hold both in terms of the one-step transition probabilities for discrete-time processes and in terms of the corresponding infinitesimal generators for continuous-time processes. We give examples showing that a stochastic monotonicity assumption is not necessary for such orderings. We indicate relations between dependence orderings and, variously, the asymptotic variance-reduction effect in Monte Carlo Markov chains, Cheeger constants, and positive dependence for Markov processes.