We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
The structure of groups in which every element has prime power order (CP-groups) is extensively studied. We first investigate the properties of group $G$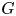
such that each element of $G\setminus N$
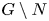has prime power order. It is proved that $N$
is solvable or every non-solvable chief factor $H/K$
of $G$
satisfying $H\leq N$
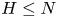is isomorphic to $PSL_2(3^f)$
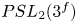with $f$
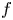a 2-power. This partially answers the question proposed by Lewis in 2023, asking whether $G\cong M_{10}$
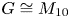? Furthermore, we prove that if each element $x\in G\backslash N$
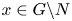has prime power order and ${\bf C}_G(x)$
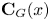is maximal in $G$
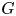, then $N$
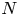is solvable. Relying on this, we give the structure of group $G$
with normal subgroup $N$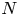
such that ${\bf C}_G(x)$
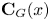is maximal in $G$
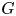for any element $x\in G\setminus N$
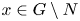. Finally, we investigate the structure of a normal subgroup $N$
when the centralizer ${\bf C}_G(x)$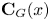
is maximal in $G$
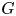for any element $x\in N\setminus {\bf Z}(N)$
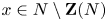, which is a generalization of results of Zhao, Chen, and Guo in 2020, investigating a special case that $N=G$
for our main result. We also provide a new proof for Zhao, Chen, and Guo's results above.
