We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
It is known that in an infinite very weakly cancellative semigroup with size 
$\kappa $, any central set can be partitioned into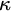 
$\kappa $ central sets. Furthermore, if 
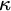$\kappa $ contains 
$\lambda $ almost disjoint sets, then any central set contains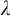 
$\lambda $ almost disjoint central sets. Similar results hold for thick sets, very thick sets and piecewise syndetic sets. In this article, we investigate three other notions of largeness: quasi-central sets, C-sets, and J-sets. We obtain that the statement applies for quasi-central sets. If the semigroup is commutative, then the statement holds for C-sets. Moreover, if 
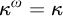$\kappa ^\omega = \kappa $, then the statement holds for J-sets.
