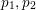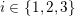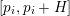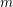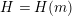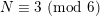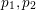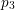In this paper we prove two results concerning Vinogradov’s three primes theorem with primes that can be called almost twin primes. First, for any  $m$, every sufficiently large odd integer
$m$, every sufficiently large odd integer  $N$ can be written as a sum of three primes
$N$ can be written as a sum of three primes 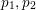 $p_{1},p_{2}$ and
$p_{1},p_{2}$ and  $p_{3}$ such that, for each
$p_{3}$ such that, for each 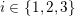 $i\in \{1,2,3\}$, the interval
$i\in \{1,2,3\}$, the interval 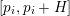 $[p_{i},p_{i}+H]$ contains at least
$[p_{i},p_{i}+H]$ contains at least 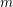 $m$ primes, for some
$m$ primes, for some 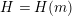 $H=H(m)$. Second, every sufficiently large integer
$H=H(m)$. Second, every sufficiently large integer 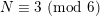 $N\equiv 3~(\text{mod}~6)$ can be written as a sum of three primes
$N\equiv 3~(\text{mod}~6)$ can be written as a sum of three primes 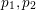 $p_{1},p_{2}$ and
$p_{1},p_{2}$ and 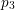 $p_{3}$ such that, for each
$p_{3}$ such that, for each 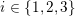 $i\in \{1,2,3\}$,
$i\in \{1,2,3\}$,  $p_{i}+2$ has at most two prime factors.
$p_{i}+2$ has at most two prime factors.