We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper, we derive new differential Harnack estimates of Li–Yau type for positive smooth solutions to a class of nonlinear parabolic equations in the form\[ {\mathscr L}_\phi^{\mathsf a} [w]:= \left[ \frac{\partial}{\partial t} - \mathsf{a}(x,t) - \Delta_\phi \right] w (x,t) = \mathscr G(t, x, w(x,t)), \quad t>0, \]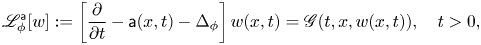on smooth metric measure spaces where the metric and potential are time dependent and evolve under a $({\mathsf k},\, m)$
-super Perelman–Ricci flow. A number of consequences, most notably, a parabolic Harnack inequality, a class of Hamilton type global curvature-free estimates and a general Liouville type theorem together with some consequences are established. Some special cases are presented to illustrate the strength of the results.
