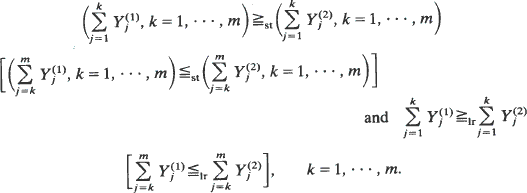We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
The equilibrium rate rY of a random variable Y with support on non-negative integers is defined by rY(0) = 0 and rY(n) = P[Y = n – 1]/P[Y – n],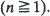 
Let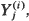 
(j = 1, …, m; i = 1,2) be 2m independent random variables that have proportional equilibrium rates with 
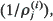(j = 1, …, m; i = 1, 2) as the constant of proportionality. When the equilibrium rate is increasing and concave [convex] it is shown that 
, …, 
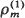) majorizes 
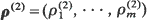implies 
, …, 
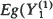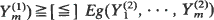for all increasing Schur-convex [concave] functions 
whenever the expectations exist. In addition if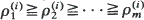 
, (i = 1, 2), then
