We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper, the dimension of the nonuniform bivariate spline space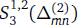 
is discussed based on the theory of multivariate spline space. Moreover, by means of the Conformality of Smoothing Cofactor Method, the basis of 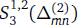composed of two sets of splines are worked out in the form of the values at ten domain points in each triangular cell, both of which possess distinct local supports. Furthermore, the explicit coefficients in terms of B-net are obtained for the two sets of splines respectively.
