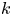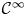Let $X$, $Y$ be nonsingular real algebraic sets. A map $\varphi \colon X \to Y$ is said to be $k$-regulous, where $k$ is a nonnegative integer, if it is of class $\mathcal {C}^k$ and the restriction of $\varphi$ to some Zariski open dense subset of $X$ is a regular map. Assuming that $Y$ is uniformly rational, and $k \geq 1$, we prove that a $\mathcal {C}^{\infty }$ map $f \colon X \to Y$ can be approximated by $k$-regulous maps in the $\mathcal {C}^k$ topology if and only if $f$ is homotopic to a $k$-regulous map. The class of uniformly rational real algebraic varieties includes spheres, Grassmannians and rational nonsingular surfaces, and is stable under blowing up nonsingular centers. Furthermore, taking $Y=\mathbb {S}^p$ (the unit $p$-dimensional sphere), we obtain several new results on approximation of $\mathcal {C}^{\infty }$ maps from $X$ into $\mathbb {S}^p$ by 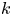$k$-regulous maps in the $\mathcal {C}^k$ topology, for $k \geq 0$.

Given a $\text{TQFT}$ in dimension $d\,+\,1$, and an infinite cyclic covering of a closed ($d\,+\,1$)-dimensional manifold $M$, we define an invariant taking values in a strong shift equivalence class of matrices. The notion of strong shift equivalence originated in R. Williams’ work in symbolic dynamics. The Turaev-Viro module associated to a $\text{TQFT}$ and an infinite cyclic covering is then given by the Jordan form of this matrix away from zero. This invariant is also defined if the boundary of $M$ has an ${{S}^{1}}$ factor and the infinite cyclic cover of the boundary is standard. We define a variant of a $\text{TQFT}$ associated to a finite group $G$ which has been studied by Quinn. In this way, we recover a link invariant due to D. Silver and S. Williams. We also obtain a variation on the Silver-Williams invariant, by using the $\text{TQFT}$ associated to $G$ in its unmodified form.

We give necessary and sufficient conditions for a norm-compact subset of a Hilbert space to admit a ${{C}^{1}}$ embedding into a finite dimensional Euclidean space. Using quasibundles, we prove a structure theorem saying that the stratum of $n$-dimensional points is contained in an $n$-dimensional ${{C}^{1}}$ submanifold of the ambient Hilbert space. This work sharpens and extends earlier results of $\text{G}$. Glaeser on paratingents. As byproducts we obtain smoothing theorems for compact subsets of Hilbert space and disjunction theorems for locally compact subsets of Euclidean space.