In this note, we first give a characterization of super weakly compact convex sets of a Banach space $X$: a closed bounded convex set $K\,\subset \,X$ is super weakly compact if and only if there exists a 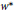${{w}^{*}}$ lower semicontinuous seminorm $P$ with $P\,\ge \,{{\sigma }_{K}}\,\equiv \,{{\sup }_{x\in K}}\left\langle \,\cdot \,,\,x \right\rangle $ such that 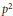${{P}^{2}}$ is uniformly Fréchet differentiable on each bounded set of ${{X}^{*}}$. Then we present a representation theoremfor the dual of the semigroup swcc$\left( X \right)$ consisting of all the nonempty super weakly compact convex sets of the space $X$.

We give precise conditions under which the composition of a norm with a convex function yields a uniformly convex function on a Banach space. Various applications are given to functions of power type. The results are dualized to study uniform smoothness and several examples are provided.

We give a multidirectional mean value inequality with second order information. This result extends the classical Clarke-Ledyaev's inequality to the second order. As application, we give the uniqueness of viscosity solution of second order Hamilton-Jacobi equations in finite dimensions.

Formulas for the Clarke subdifferential are always expressed in the form of inclusion. The equality form in these formulas generally requires the functions to be directionally regular. This paper studies the directional regularity of the general class of extended-real-valued functions that are directionally Lipschitzian. Connections with the concept of subdifferential regularity are also established.

Given a free ideal J of subsets of a set X, we consider games where player ONE plays an increasing sequence of elements of the σ-completion of J, and player TWO tries to cover the union of this sequence by playing one set at a time from J. We describe various conditions under which player TWO has a winning strategy that uses only information about the most recent k moves of ONE, and apply some of these results to the Banach-Mazurgame.

We study the relationships between Gateaux, Fréchet and weak Hadamard differentiability of convex functions and of equivalent norms. As a consequence we provide related characterizations of infinite dimensional Banach spaces and of Banach spaces containing ł1. Explicit examples are given. Some renormings of WCG Asplund spaces are made in this vein.