 $\sqrt {n}$
$\sqrt {n}$
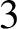 $3$
-manifolds
$3$
-manifolds
 $3$
-manifolds containing infinitely many totally geodesic surfaces
$3$
-manifolds containing infinitely many totally geodesic surfaces