We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove existence and multiplicity of solutions for the problem
where 
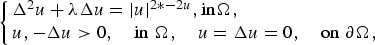$$\left\{ {\matrix{ {\Delta ^2u + \lambda \Delta u = \vert u \vert ^{2*-2u},{\rm in }\Omega ,} \hfill \hfill \hfill \hfill \cr {u,-\Delta u > 0,\quad {\rm in}\;\Omega ,\quad u = \Delta u = 0,\quad {\rm on}\;\partial \Omega ,} \cr } } \right.$$
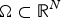$\Omega \subset {\open R}^N$, 
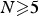$N \ges 5$, is a bounded regular domain, 
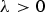$\lambda >0$ and 
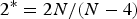$2^*=2N/(N-4)$ is the critical Sobolev exponent for the embedding of 
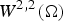$W^{2,2}(\Omega )$ into the Lebesgue spaces.
