The endomorphism monoid of a model-theoretic structure carries two interesting topologies: on the one hand, the topology of pointwise convergence induced externally by the action of the endomorphisms on the domain via evaluation; on the other hand, the Zariski topology induced within the monoid by (non-)solutions to equations. For all concrete endomorphism monoids of $\omega $-categorical structures on which the Zariski topology has been analysed thus far, the two topologies were shown to coincide, in turn yielding that the pointwise topology is the coarsest Hausdorff semigroup topology on those endomorphism monoids.

We establish two systematic reasons for the two topologies to agree, formulated in terms of the model-complete core of the structure. Further, we give an example of an $\omega $-categorical structure on whose endomorphism monoid the topology of pointwise convergence and the Zariski topology differ, answering a question of Elliott, Jonušas, Mitchell, Péresse, and Pinsker.

A union ultrafilter is an ultrafilter over the finite subsets of ω that has a base of sets of the form ${\text{FU}}\left( X \right)$, where X is an infinite pairwise disjoint family and ${\text{FU}}(X) = \left\{ {\bigcup {F|F} \in [X]^{ < \omega } \setminus \{ \emptyset \} } \right\}$. The existence of these ultrafilters is not provable from the $ZFC$ axioms, but is known to follow from the assumption that ${\text{cov}}\left( \mathcal{M} \right) = \mathfrak{c}$. In this article we obtain various models of $ZFC$ that satisfy the existence of union ultrafilters while at the same time ${\text{cov}}\left( \mathcal{M} \right) = \mathfrak{c}$.

A paper of Almeida and Trotter [‘The pseudoidentity problem and reducibility for completely regular semigroups’, Bull. Aust. Math. Soc.63 (2001), 407–433] makes essential use of free profinite semigroupoids over profinite graphs with infinitely many vertices. It has since been shown that such structures must be handled with great care. In this note, it is verified that the required properties hold for the profinite graphs considered by Almeida and Trotter, thereby filling the gaps in the proof.

Let 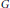$G$ be an infinite discrete group and let 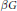$\beta G$ be the Stone-Čech compactification of 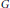$G$. We take the points of 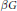$\beta G$ to be the ultrafilters on 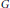$G$, identifying the principal ultrafilters with the points of 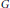$G$. The set 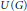$U\left( G \right)$ of uniform ultrafilters on 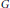$G$ is a closed two-sided ideal of 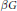$\beta G$. For every 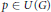$p\,\in \,U\left( G \right)$, define 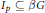${{I}_{p}}\,\subseteq \,\beta G$ by 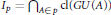${{I}_{p}}\,=\,{{\bigcap }_{A\in p}}\text{cl}\left( GU\left( A \right) \right)$, where 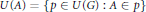$U\left( A \right)\,=\,\left\{ p\,\in \,U\left( G \right)\,:\,A\in \,p \right\}$. We show that if 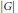$\left| G \right|$ is a regular cardinal, then 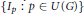$\left\{ {{I}_{p}}\,:\,p\,\in \,U\left( G \right) \right\}$ is the finest decomposition of 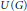$U\left( G \right)$ into closed left ideals of 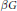$\beta G$ such that the corresponding quotient space of 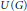$U\left( G \right)$ is Hausdorff.

A locally compact semilattice with open principal filters is a zero-dimensional scattered space. Cardinal invariants of locally compact and compact semilattices with open principal filters are investigated. Structure of topological semilattices on the one-point Alexandroff compactification of an uncountable discrete space and linearly ordered compact semilattices with open principal filters are researched.

A classical result of I. Glicksberg and K. de Leeuw asserts that the almost periodic compactification of a direct product S × T of abelian semigroups with identity is (canonically isomorphic to) the direct product of the almost periodic compactiflcations of S and T. Some efforts have been made to generalize this result and recently H. D. Junghenn and B. T. Lerner have proved a theorem giving necessary and sufficient conditions for an F-compactification of a semidirect product S⊗σT to be a semidirect product of compactiflcations of S and T. A different such theorem is presented here along with a number of corollaries and examples which illustrate its scope and limitations. Some behaviour that can occur for semidirect products, but not for direct products, is exposed