Let R be a discrete valuation ring of field of fractions K and of residue field k of characteristic $p> 0$. In an earlier work, we studied the question of extending torsors over K-curves into torsors over R-regular models of the curves in the case when the structural K-group scheme of the torsor admits a finite flat model over R. In this paper, we first give a simpler description of the problem in the case where the curve is semistable using recent work in Holmes, Molcho, Orecchia, and Poiret (2023, Journal für die Reine und Angewandte Mathematik [Crelle’s Journal] 230, 115–159) and Molcho and Wise (2022, Compositio Mathematica 158, 1477–1562). Second, if R is assumed to be Henselian and Japanese, we solve the problem of extending torsors by combining our previous work together with results in Antei and Emsalem (2018, Nagoya Mathematical Journal 230, 18–34) and Phung and Dos Santos (2023, Algebraic Geometry 230, 1–40), including the case where the structural group does not admit a finite flat R-model.

Let G be a group and let V be an algebraic variety over an algebraically closed field K. Let A denote the set of K-points of V. We introduce algebraic sofic subshifts ${\Sigma \subset A^G}$ and study endomorphisms $\tau \colon \Sigma \to \Sigma $. We generalize several results for dynamical invariant sets and nilpotency of $\tau $ that are well known for finite alphabet cellular automata. Under mild assumptions, we prove that $\tau $ is nilpotent if and only if its limit set, that is, the intersection of the images of its iterates, is a singleton. If moreover G is infinite, finitely generated and $\Sigma $ is topologically mixing, we show that $\tau $ is nilpotent if and only if its limit set consists of periodic configurations and has a finite set of alphabet values.

We study the symplectic geometry of derived intersections of Lagrangian morphisms. In particular, we show that for a functional $f : X \rightarrow \mathbb {A}_{k}^{1}$, the derived critical locus has a natural Lagrangian fibration $\textbf {Crit}(f) \rightarrow X$. In the case where f is nondegenerate and the strict critical locus is smooth, we show that the Lagrangian fibration on the derived critical locus is determined by the Hessian quadratic form.

The purpose of this note is to correct a mistake in the article “A curve selection lemma in spaces of arcs and the image of the Nash map” Compositio Math. 142 (2006), 119–130. It is due to an overlooked hypothesis in the definition of generically stable subset of the space of arcs X∞ of a variety X defined over a perfect field k.

In this paper we extend the generalized algebraic fundamental group constructed in Esnault and Hogadi, (Trans. Amer. Math. Soc. 364(5) (2012), 2429–2442) to general fibered categories using the language of gerbes. As an application we obtain a Tannakian interpretation for the Nori fundamental gerbe defined in Borne and Vistoli (J. Algebraic Geom. (2014), S1056–3911, 00638-X) for nonsmooth non-pseudo-proper algebraic stacks.

We study two notions of purity in categories of sheaves: the categorical and the geometric. It is shown that pure injective envelopes exist in both cases under very general assumptions on the scheme. Finally, we introduce the class of locally absolutely pure (quasi-coherent) sheaves with respect to the geometrical purity, and characterize locally Noetherian closed subschemes of a projective scheme in terms of the new class.

We give a bound on embedding dimensions of geometric generic fibers in terms of the dimension of the base, for fibrations in positive characteristic. This generalizes the well-known fact that for fibrations over curves, the geometric generic fiber is reduced. We illustrate our results with Fermat hypersurfaces and genus 1 curves.

Let $\mathbb K$ be an algebraically closed field, let $X$ be a $\mathbb K$-variety, and let $X(\mathbb K)$ be the set of closed points in $X$. A constructible set$C$ in $X(\mathbb K)$ is a finite union of subsets $Y(\mathbb K)$ for subvarieties $Y$ in $X$. A constructible function$f:X(\mathbb K)\rightarrow\mathbb Q$ has $f(X(\mathbb K))$ finite and $f^{-1}(c)$ constructible for all $c\ne 0$. Write CF$(X)$ for the vector space of such $f$. Let $\phi:X\rightarrow Y$ and $\psi: Y\rightarrow Z$ be morphisms of ${\mathbb C}$-varieties. MacPherson defined a linear pushforward CF$(\phi):{\rm CF}(X)\rightarrow{\rm CF}(Y)$ by ‘integration’ with respect to the topological Euler characteristic. It is functorial, that is, CF$(\psi\circ\phi)={\rm CF}(\psi)\circ{\rm CF}(\phi)$. This was extended to $\mathbb K$ of characteristic zero by Kennedy.

This paper generalizes these results to $\mathbb K$-schemes and Artin$\mathbb K$-stacks with affine stabilizer groups. We define the notions of Euler characteristic for constructible sets in $\mathbb K$-schemes and $\mathbb K$-stacks, and pushforwards and pullbacks of constructible functions, with functorial behaviour. Pushforwards and pullbacks commute in Cartesian squares. We also define pseudomorphisms, a generalization of morphisms well suited to constructible functions problems.

We define a motivic analogue of the Haar measure for groups of the form $G(k((t)))$, where $k$ is an algebraically closed field of characteristic zero, and $G$ is a reductive algebraic group defined over $k$. A classical Haar measure on such groups does not exist since they are not locally compact. We use the theory of motivic integration introduced by M. Kontsevich to define an additive function on a certain natural Boolean algebra of subsets of $G(k((t)))$. This function takes values in the so-called dimensional completion of the Grothendieck ring of the category of varieties over the base field. It is invariant under translations by all elements of $G(k((t)))$, and therefore we call it a motivic analogue of Haar measure. We give an explicit construction of the motivic Haar measure, and then prove that the result is independent of all the choices that are made in the process.

We prove a finiteness result in the space of arcs $X_\infty$ of a singular variety X, which is an extension of the stability result of Denef and Loeser (Germs of arcs on singular algebraic varieties and motivic integration, Invent. Math. 135 (1999), 201–232). From this follows a curve selection lemma for generically stable subsets of $X_\infty$. As a consequence, we extend to all dimensions the problem of wedges, proposed by Lejeune-Jalabert (Arcs analytiques et résolution minimale des singularités des surfaces quasi-homogénes, Lecture Notes in Mathematics, vol. 777 (Springer, Berlin, 1980), 303–336), and we obtain that an affirmative answer to this problem is equivalent to the surjectivity of the Nash map. This implies, for instance, that the Nash map is bijective for sandwiched surface singularities.

We study the Hilbert functions of fat points in 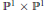${{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$. If $Z\,\subseteq \,{{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$ is an arbitrary fat point scheme, then it can be shown that for every 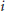$i$ and 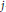$j$ the values of the Hilbert function 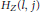${{H}_{Z}}(l,\,j)$ and 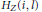${{H}_{Z}}(i,\,l)$ eventually become constant for 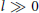$l\,\gg \,0$. We show how to determine these eventual values by using only the multiplicities of the points, and the relative positions of the points in 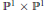${{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$. This enables us to compute all but a finite number values of 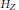${{H}_{Z}}$ without using the coordinates of points. We also characterize the 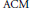$\text{ACM}$ fat point schemes using our description of the eventual behaviour. In fact, in the case that $Z\,\subseteq \,{{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$ is 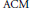$\text{ACM}$, then the entire Hilbert function and its minimal free resolution depend solely on knowing the eventual values of the Hilbert function.

Let $f:X\to Y$ be a Cohen–Macaulay map of finite type between Noetherian schemes, and $g:Y'\to Y$ a map, with Y′ Noetherian. Let $f':X'\to Y'$ be the base change of f under g and $g':X'\to X$ the base change of g under f. We show that there is a canonical isomorphism $\theta_g^f: {g'}^*\omega_f \simeq \omega_{f'}$, where $\omega_f$ and $\omega_{f'}$ are the relative dualizing sheaves. The map $\theta_g^f$ is easily described when f is proper, and has a more subtle description when f is not. If f is smooth we show that $\theta_g^f$ corresponds to the canonical identification $g'^*\Omega_f^r= \Omega_{f'}^r$ of differential forms, where r is the relative dimension of f. This work is closely related to B. Conrad's work on base change. However, our approach to the problems and our viewpoint are very different from Conrad's: dualizing complexes and their Cousin versions, residual complexes, do not appear in this paper.

On a smooth $n$-dimensional complete variety $X$ over $\mathbb{C}$ we show that the trace map ${{\bar{\theta }}_{X}}\,:\,{{H}^{n}}(X,\,\Omega _{X}^{n})\,\to \,\mathbb{C}$ arising from Lipman's version of Grothendieck duality in $\left[ \text{L} \right]$ agrees with

$${{(2\pi i)}^{-n}}{{(-1)}^{n(n-1)/2}}\,\int_{X}{:\,H_{DR}^{2n}\,(X,\,\mathbb{C})\,\to \,\mathbb{C}}$$

under the Dolbeault isomorphism.