2.1. Experimental database

All schlieren and some of the PIV experiments were conducted in the Laboratory for Turbulence Research in Aerospace and Combustion (LTRAC) Supersonic Jet Facility, with a subset of PIV experiments carried out in the newer LTRAC Gas Jet Facility. The two facilities are very similar in plenum construction, but the Gas Jet Facility has Perspex walls at a distance of $60D$ (where D is nozzle exit diameter) from the nozzle centreline on all sides, changing the far-field boundary conditions. Both facilities are supplied with dry compressed air at a stagnation temperature of approximately 298 K, regulated to within $1\,\%$ of the target pressure by a Fairchild 100 high-flow pressure regulator. Pressure in the plenum chambers was monitored by an RS-461 pressure transducer with an uncertainty of ${\pm }0.25\,\%$. The same nozzle was used for all measurements: a 15 mm diameter purely converging nozzle, with a radius of curvature of 67.15 mm, ending with a 5 mm parallel section at the nozzle exit, and an external lip thickness of 5 mm. The plenum-to-nozzle contraction ratio is in excess of $100\,{:}\,1$. Given the short parallel nozzle section and high contraction ratio, the boundary layer at the nozzle exit is expected to be extremely thin and likely laminar. Both optical facilities contain numerous hard reflective surfaces, and thus can in no way be considered anechoic. Exemplar measurements of screech directivity were obtained in the LTRAC Supersonic Anechoic Jet Facility (SJAF), using a G.R.A.S. Type 46BE 1/4” pre-amplified microphone, recorded on a National Instruments DAQ at a sample rate of 250 kHz. The nozzle in this facility is smaller ($D=8\ \textrm {mm}$), with a lower contraction ratio; the boundary layer may be slightly thicker in this facility. The acoustic spectra presented in figure 2 both display the well-known directivity pattern of jet screech, which has been most carefully characterized in the work of Norum (Reference Norum1983). Norum identified that the fundamental screech tone radiated most strongly at $\phi =160^{\circ }$ (here $\phi$ is the polar angle measured from the downstream axis) across a wide range of pressure ratios and modes of jet screech. For the screech modes associated with azimuthal mode $m=1$, there was a minimum in screech tone amplitude at $\phi =90^{\circ }$, but a maximum for the first harmonic. In the present data, for the jet at $NPR=3.4$, the same trend is observed; increasing directivity of the fundamental as the observer is moved upstream, and a minimum in the sideline direction for the fundamental, but a maximum for the first harmonic. For the $NPR = 2.25$ jet, an increase in tone amplitude is observed as the observer is moved upstream, however, the effect is far less pronounced than for the higher-pressure ratio jet. The minimum for the fundamental at $\phi =90^{\circ }$ is also not observed for this condition. The present set-up of the SAJF cannot produce far-field data at the extreme upstream angles available in the data of Norum (Reference Norum1983), but the key trend of upstream directivity is evident even in the smaller range of angles available.

Figure 2. Representative far-field ($50D$ from nozzle exit) acoustic spectra for various polar angles $\phi$, measured from the downstream axis. Symbols indicate peaks of the fundamental screech tone.

Several flow conditions are considered here in detail, covering several ‘stages’ of jet screech, as summarized in table 1. Flow visualizations are provided for some other cases beyond those listed in this table. Here, nozzle pressure ratio ($NPR$) is defined as the ratio between the plenum and the ambient pressure: $NPR = p_0/p_{\infty }$, and ideally expanded Mach number $M_j$ is calculated assuming isentropic expansion to ambient pressure. Reynolds and Strouhal number are likewise calculated based on ideally expanded conditions: $Re=U_j \rho _j D_j / \mu$, $St=f_sU_j/D_j$. Here, U, $\rho$ and D refer to axial velocity, density and diameter respectively, with the subscript j indicating the ideally expanded condition. Note the slight correction of Strouhal numbers from those presented in Edgington-Mitchell et al. (Reference Edgington-Mitchell, Jaunet, Jordan, Towne, Soria and Honnery2018a).

Table 1. Jet conditions.

The schlieren images were obtained using various configurations of a classical Toepler Z-type schlieren apparatus with mirrors of focal length 2032 mm (Mitchell, Honnery & Soria Reference Mitchell, Honnery and Soria2012). High-speed schlieren images were acquired using a Shimadzu HPV-1 with a resolution of $312 \times 260\ \textrm {px}$, using an exposure time of 250 ns. Illumination for the high-speed images was provided by a Metz Mecablitz flash. The schlieren imaging apparatus records an image whose intensity is proportional to the path-integrated density gradient, with a directionality determined by the orientation of the spatial cutoff. For the monochrome schlieren images, a standard razor blade is used as the spatial cutoff. The cutoff is oriented to capture the axial density gradient, such that the intensity of the images ($I$) in this paper represent $I(x,y) \propto \int \partial \rho /\partial x \, \textrm {d}z$; here $x$ is positive in the downstream axis, $y$ is the transverse direction and $z$ represents the path of integration orthogonal to the other two axes. High-resolution colour images were obtained by replacing the flash unit with a pulsed white LED (Willert, Mitchell & Soria Reference Willert, Mitchell and Soria2012) focused onto a four-colour mask. The knife edge was replaced with an iris cutoff, such that the direction of the density gradient is encoded in the colour of the resultant image.

The PIV datasets for the $NPR = 2.10$ and $NPR = 2.25$ cases, which correspond to the toroidal ‘A’ mode of jet screech, were acquired in the LTRAC Gas Jet Facility and have previously been presented in Edgington-Mitchell et al. (Reference Edgington-Mitchell, Jaunet, Jordan, Towne, Soria and Honnery2018a). The dataset for the $NPR = 3.4$ case, which corresponds to a helical ‘C’ mode of screech, was obtained in the Supersonic Jet Facility, and has previously been presented in Edgington-Mitchell et al. (Reference Edgington-Mitchell, Oberleithner, Honnery and Soria2014c) and Tan et al. (Reference Tan, Soria, Honnery and Edgington-Mitchell2017). Pertinent details such as final interrogation window size $IW_1$, vector spacing $\Delta X$, through-plane integration distance based on light sheet or depth of field $Z$ and field of view $FOV$ are presented in table 2. For all cases the same seeding was used, in the form of 600 nm diameter smoke particles (Mitchell, Honnery & Soria Reference Mitchell, Honnery and Soria2013), and the multi-grid algorithm of Soria (Reference Soria1996) was used to analyse the image pairs. Contours of mean axial velocity for the three PIV datasets are presented in figure 3.

Figure 3. Mean axial velocity, normalized by jet exit velocity $U_E$ for: (a) $NPR = 2.10$; (b) $NPR = 2.25$; (c) $NPR = 3.4$.

Table 2. PIV parameters.

2.2. Decomposition of velocity data

The PIV data presented here are not time resolved with respect to the screech frequency. Consequently the shock-leakage behaviour (which cannot be modelled as a steady process as per Shariff & Manning (Reference Shariff and Manning2013)) cannot be directly interrogated by analysing the raw data. However, reconstruction of a phase cycle of the screech phenomenon can facilitate assessment of shock leakage even if the original snapshots are statistically independent. Here, this phase reconstruction is done via a proper orthogonal decomposition (POD).

Although the flows considered in this paper are highly turbulent, the resonance process that produces the discrete-frequency screech tone is a result only of a series of coherent downstream-propagating wavepackets at one particular wavelength. Following Hussain & Reynolds (Reference Hussain and Reynolds1970), the fluctuations in the jet may thus be decomposed into a mean ($\boldsymbol {U}$), coherent fluctuations at the screech frequency $\boldsymbol {u}^c$ and a stochastic component ($\boldsymbol {u}''$)

(2.1)\begin{equation} \boldsymbol{u}(\boldsymbol{x},t) = \boldsymbol{U}(\boldsymbol{x})+\boldsymbol{u}^{c}(\boldsymbol{x},t)+\boldsymbol{u}''(\boldsymbol{x},t). \end{equation}

It has been demonstrated consistently in prior work that snapshot proper orthogonal decomposition (Sirovich Reference Sirovich1987) can extract the coherent component associated with the screech process. For the POD, the autocovariance matrix is constructed from the velocity snapshots $\boldsymbol {V}$ such that

(2.2)\begin{equation} \boldsymbol{R}=\boldsymbol{V}^T\boldsymbol{V}. \end{equation}

The solution of the eigenvalue problem

(2.3)\begin{equation} R\boldsymbol{v}=\lambda \boldsymbol{v} \end{equation}

yields the eigenpairs ($\lambda ,\boldsymbol {v}$) from which the spatial POD modes are constructed as

(2.4)\begin{equation} \boldsymbol{\phi}_n(x,y)=\frac{\boldsymbol{V}\boldsymbol{v}_n(t)}{\|\boldsymbol{Vv}_n(t)\|}. \end{equation}

The coefficients at each time $t$ for each mode $n$ can be expressed as

(2.5)\begin{equation} \boldsymbol{a}_n(t)=\boldsymbol{v}_n(t)\|\boldsymbol{Vv}_n(t)\|. \end{equation}

Coherent fluctuations are reconstructed according to the sum of weighted modes

(2.6)\begin{equation} \boldsymbol{u}_c(\boldsymbol{x},t)=\sum^2_{n=1}\boldsymbol{\phi}_n(x)\boldsymbol{a}_n^T(t). \end{equation}

If a pair of POD modes that represent a travelling wave can be identified, and if the coherent fluctuations can be represented only by a single pair of these modes, then the phase cycle associated with screech can be reconstructed directly from this POD modes pair (Oberleithner et al. Reference Oberleithner, Sieber, Nayeri, Paschereit, Petz, Hege, Noack and Wygnanski2011; Jaunet, Collin & Delville Reference Jaunet, Collin and Delville2016). By defining $a_1 - \textrm {i}a_2=\hat {a}\textrm {e}^{-\textrm {i} \omega _s t}$, a phase angle can be associated with each snapshot.

The axial velocity fluctuations associated with the leading pair of POD modes for each case are provided in figure 4, along with a joint probability density function (JPDF) of the mode coefficients plotted against each other. The Lissajous curve overlaid on the JPDF in black represents the mean radius of the mode pair at each phase angle; the closed circle indicates that these pairs of modes represent a travelling wave structure (Taira et al. Reference Taira, Brunton, Dawson, Rowley, Colonius, McKeon, Schmidt, Gordeyev, Theofilis and Ukeiley2017). The radius here is analogous to the amplitude of the coherent fluctuations, and the distribution of the JPDF about the mean radius hence indicates a degree of variation in the strength of the coherent fluctuation. The effect of this fluctuation amplitude on the shock-leakage process is considered in § 5.

Figure 4. (a,d,g) Coherent axial velocity fluctuation association associated with first POD mode. (b,e,h) Coherent axial velocity fluctuation association associated with second POD mode. (c,f,i) Probability density of temporal coefficients for leading two POD modes. All contours are normalized by maximum value.

2.2.1. Decomposition of schlieren data

While the ultra-high-speed image sequences contain a wealth of information about the flow dynamics, the limitations of the experiment can make this information difficult to extract. Observation of shock leakage requires operating the camera at the limit of its capacity at one million frames per second. In this regime the signal to noise ratio is quite poor. The limitations of the schlieren technique are even more severe; in the jet near field the hydrodynamic fluctuations associated with downstream-travelling structures are significantly stronger than the fluctuations associated with the upstream-propagating acoustic waves produced by shock leakage. High sensitivity in the schlieren system is required to visualize the acoustic waves, but with a classical schlieren system there is an inverse relationship between measuring range and sensitivity; resolving the acoustic waves means the downstream-propagating structures saturate the schlieren image. In an attempt to educe only the components of the schlieren image associated with the shock-leakage phenomenon, a Fourier decomposition is performed on the data. Since shock leakage is the consequence of an upstream motion of the shock tip, and the conversion of a section of that shock tip into an upstream-propagating wave, the phenomenon is most clearly visualized only in components with negative (upstream) phase velocity. The intensity of the images is a function of both space and time: $I(x,y,t)$, and can thus be decomposed as

(2.7)\begin{equation} I(x,y,t) = \sum_{k}\sum_{\omega}\hat{I}_{k,\omega}\textrm{e}^{\textrm{i}kx}\textrm{e}^{-\textrm{i}\omega t}. \end{equation}

The data can then be filtered to show only components propagating upstream (i.e. axial wavenumber $k < 0$) at the screech frequency $\omega _s$. The reconstructed intensity field can be written in terms of the temporal Fourier coefficient $\hat {a}$

(2.8)\begin{equation} I(x,y,t) = \hat{a}\textrm{e}^{-\textrm{i}\omega_s t}\sum_{k<0}\hat{I}_{k,\omega_s}\textrm{e}^{\textrm{i}kx}. \end{equation}

2.3. Coherent structure identification via fields of finite-time Lyapunov exponents

Shock leakage is expected to occur at the saddle point between vortices, where the local vorticity is a minimum (Suzuki & Lele Reference Suzuki and Lele2003). These saddle points and the shear layer can be identified in the velocity data through the use of Lagrangian coherent structures (LCS) (Haller Reference Haller2015). In addition to facilitating an objective definition of the saddle points, LCS also enable a more intuitive comparison between the structures observed in the flow visualizations of § 3 and the velocity data of § 4. In this work the identification of LCS is performed by tracking contours of maxima in the field of finite-time Lyapunov exponents (FTLEs), following the methodology of Premchand et al. (Reference Premchand, George, Raghunathan, Unni, Sujith and Nair2019).

The Lyapunov exponent measures the degree of attraction or repulsion between neighbouring packets of fluid. As the FTLE is tracked backwards in time, local maxima in the FTLE represent unstable manifolds in the flow; these unstable manifolds, defined as regions of where the fluid particles converge along material surfaces, are analogous to LCS in the flow field, which educes the shear layer. The Lyapunov exponent is defined as per

(2.9)\begin{equation} \sigma^T_{t_0}(\boldsymbol{x_0},t_0)=\frac{1}{|T|}\ln\sqrt{\lambda_{max}(C^t_{t_{0}}(\boldsymbol{x_0},t_0))}. \end{equation}

Here, $C^t_{t_0}$ is the right Cauchy–Green tensor, defined from the Jacobian of a mapping of the flow field $F^t_{t_0} := x(t: t_0, \boldsymbol {x_0})$, as per (2.10). The gradient of the flow map $F$ is a $3\times 3$ matrix for a three-dimensional velocity field, termed the deformation gradient tensor. Its elements represent the gradient of the new location of a fluid parcel $\boldsymbol {x}$ with respect to its starting location $\boldsymbol {x_0}$, i.e. ${\partial \boldsymbol {x}}/{\partial \boldsymbol {x}_{\boldsymbol {0}}}$. The right Cauchy–Green tensor can be constructed by premultiplying the transpose of the deformation gradient tensor with itself and represents the square of the local changes in fluid displacement. This eliminates the effects of a pure rotation of the fluid parcel and provides a rotation-independent deformation metric.

(2.10)\begin{equation} C^t_{t_{0}}=\left[\boldsymbol{\nabla} F^t_{t_0}(\boldsymbol{x_0})\right]^\intercal \boldsymbol{\nabla} F^t_{t_0}(\boldsymbol{x_0}). \end{equation}

As defined here, $\sigma ^T_{t_0}(\boldsymbol {x_0},t_0)$ measures the separation rate of initially adjacent fluid particles over the time period $T=t_0-t={1.5}/{f_s}$.

Again, following the approach of Premchand et al. (Reference Premchand, George, Raghunathan, Unni, Sujith and Nair2019), in this work the particle advection is performed backwards in time, such that the maxima of the FTLE contours represent attracting rather than repelling LCS, as indicated in figure 5.

Figure 5. Schematic showing fluid parcel advection in forward time; the red line represents the forward time FTLE, and the blue line the backward time FTLE.

2.4. Geometrical acoustics model

Here, a summary of the fundamental precepts of the ray-tracing model of Shariff & Manning (Reference Shariff and Manning2013) is presented; for a full discussion the reader is referred to the original work. Following from the earlier work of Manning & Lele (Reference Manning and Lele2000), the shocks within the jet are treated as standing acoustic waves, whose behaviour is modelled using geometrical acoustics. In the implementation of Shariff and Manning, the waves are visualized as ‘streaks’ produced by continuously injecting particles that obey the ray-tracing equations. From the original ray-tracing equations in an unsteady base flow $V_i$, where $x_i(t)$ is a position along a ray, $k_i(t)$ is the associated wave vector and $i$ is an index over the spatial dimensions

(2.11a,b)\begin{equation} \frac{\textrm{d}x_i}{\textrm{d}t}=\frac{\partial \omega}{\partial k_i}, \quad \frac{\textrm{d}k_i}{\textrm{d}t}=-\frac{\partial \omega}{\partial x_i}. \end{equation}

Shariff and Manning demonstrate a reduction to the following form:

(2.12)\begin{gather} \frac{\textrm{d}x_i}{\textrm{d}t}= V_i + \frac{k_i c(\boldsymbol{x},t)}{|\boldsymbol{k}|}, \end{gather}

(2.13)\begin{gather} \frac{\textrm{d}k_i}{\textrm{d}t}= -k_j \frac{\partial V_j}{\partial x_i} - |\boldsymbol{k}| \frac{\partial c(\boldsymbol{x},t)}{\partial x_i}. \end{gather}

Equation (2.12) dictates the that the change in particle position is determined by both advection by the base flow $V_i$, and the wave's own propagation, with direction given by $k_i$ and velocity by $c$. Equation (2.13) links the change in wave propagation direction $k_i$ (refraction) to the mean flow velocity gradient ${\partial V_j}/{\partial x_i}$ and gradients in the local speed of sound ${\partial c(\boldsymbol {x},t)}/{\partial x_i}$. In the original theoretical conception of Shariff and Manning, the synthetic velocity field was time periodic. As has been shown in the preceding subsection, by construction the decomposed experimental data are also time periodic.

2.5. Shock-leakage model

The implementation of the geometric acoustics framework of Shariff & Manning (Reference Shariff and Manning2013) with the experimental data is accomplished via the following:

1. (i) The datasets described in § 2.1 are decomposed via the methodology presented in § 2.2. On the basis of this decomposition a screech cycle is reconstructed across 2000 representations of the reduced-order velocity field. Each ‘time step’ in this reconstruction represents a $0.18^{\circ }$ increment in one single phase cycle of the screech process.

2. (ii) The reconstructed coherent velocity fields are smoothed using a $3\times 3$ Gaussian kernel.

3. (iii) At each time step in the screech cycle, particles obeying the equations stated in § 2.4 are injected at the centreline of the first shock cell, with the wave angle $\theta _k=\tan ^{-1}(k_2/k_1)$ set to the local Mach angle.

4. (iv) All particles already injected into the flow evolve as per (2.12) and (2.13) at each time step.

5. (v) The process is repeated over 20 screech cycles.

In total, 40 000 particles are injected for each case. A few caveats must be placed on the interpretation of the results of this model. As no temperature data are directly available from the PIV measurements, the second term in (2.13) is neglected; the speed of sound is assumed to remain constant in the flow. The velocity fields reconstructed as per § 2.2 already contain shock structures, and these structures will influence the evolution of the particles in a way not intended in the original conception of the model. Attempts to model the temperature fluctuations from the PIV data were found to significantly exacerbate the effect of the shock structures in the base flow data; neglecting temperature fluctuation to some extent ameliorates the impact of these shocks. The model is only implemented in a two-dimensional sense, with planar base flow data. For the $NPR = 3.4$ case, where the oscillations associated with the jet correspond to the $m=1$ mode, the model will not include the azimuthal velocity, which is significant. Even for the lower pressure ratio cases, the actual jet is axisymmetric, rather than planar. A cylindrical coordinate formulation would thus be more appropriate, but in the present work a planar model was used to enable direct comparison with the work of Shariff & Manning (Reference Shariff and Manning2013). Finally, note that in the original model there is a consideration given to wave energy and amplitude, but these properties are neglected in the present analysis. The model is sensitive to small variations in the experimental data, and to aid in interpretation, symmetry has been enforced about the jet centreline in the $NPR = 2.10$ and $NPR=2.25$ datasets by mirroring the data across the centreline. None of the results discussed in this paper are altered by this enforced symmetry, but there are fewer spurious ray streaks to complicate interpretation of the images. Given the numerous assumptions and simplifications in the implementation of the model, which is itself based on significant simplifications of the original physics, the strict predictive power of this implementation is obviously limited. However, with these caveats in mind, as shown in § 4, the model nonetheless performs remarkably well when compared to the flow visualizations presented in the following section.

3.1. Shock leakage associated with asymmetric screech modes

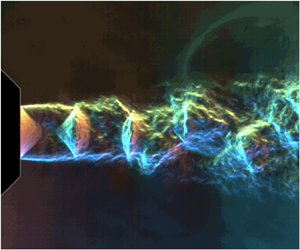
An image sequence for a twin-jet configuration with an internozzle spacing of $s/D=3.0$ and $NPR = 3.1$ is presented in figure 6. The image has been cropped to only show a section of the lower jet. In figure 6(a), the fifth shock cell is indicated with a red arrow. In the raw image on the left of the panel, a large vortex associated with the Kelvin–Helmholtz wavepacket is visible just upstream of the shock. The processed image on the right of the panel retains only those components with an upstream phase velocity as per (2.8); the outer section of the shock is already tilting upstream as it interacts with the front of the vortex. As the vortex passes the shock in figure 6(b), the shock is observed to extend radially well past its quasi-steady-state position (although in a flow with such strong vortices, it is debatable whether a steady-state position truly exists). An acoustic wave produced by the upper jet is visible in this and subsequent figures, but is not yet relevant to the discussion. By figure 6(c) a section of the shock can be seen propagating away from the jet in a small arc, but within the jet the shock is no longer moving upstream. As the vortex moves downstream and away from the shock in subsequent panels, the sharp acoustic wavefront resulting from the shock leakage can be seen moving back upstream, highlighted with the red arrow in the final figure. As the leakage is occurring on the upper side of the jet, upstream tilting of the shock on the lower half of the jet in preparation for a leakage event on that side is already evident in figure 6(d) onwards. The video sequence from which figure 6 is extracted is presented in supplementary movie 1 available at https://doi.org/10.1017/jfm.2020.945.

Figure 6. Sequence of $\textrm {d}\rho /{\textrm {d}x}$ schlieren images for a twin jet at $NPR = 3.1$, with $8\ \mathrm {\mu }\textrm {s}$ spacing between subsequent frames. On the left of each panel is the raw schlieren image. On the right of each panel is the result of the Fourier filtering to preserve only components with upstream phase velocity. The red arrows indicate the shock in (a), and the resultant acoustic wave in (f).

The same mechanism can be observed occurring at multiple points in both jets in a single image sequence, as demonstrated in figure 7. Here three discrete shock leakage events and their resultant acoustic waves are enumerated 1–3 as indicated in the figure. A series of videos demonstrating a range of shock-leakage examples for the twin-jet configuration are presented in supplementary movie 2.

Figure 7. Sequence of $\textrm {d}\rho /{\textrm {d}x}$ schlieren images for a twin jet at $NPR = 3.1$. On the left of each panel is the raw schlieren image. On the right of each panel is the result of the Fourier filtering to preserve only components with upstream phase velocity. The arrows track three discrete shock-leakage events between frames. The panels span a period of $30\ \mathrm {\mu }\textrm {s}$ but are not equispaced in time; the interframe time is $20\ \mathrm {\mu }\textrm {s}$ and $10\ \mathrm {\mu }\textrm {s}$ respectively.

In single axisymmetric jets, it has long been recognized that the flapping ‘B’ screech mode is associated with the largest vortical structures and often the highest acoustic tone amplitudes. The precession of the flapping axis also makes the ‘B’ mode one of the most difficult to study, as both planar and path-integrated measurement techniques are sensitive to the orientation of the flapping axis. Figure 8 presents an image sequence capturing a flapping motion almost orthogonal to the viewing angle. In the image, the fourth shock cell (in the centre of the frame) is observed to produce a strong upstream-propagating wave via the same mechanism as observed for the twin-jet configuration. Supplementary movie 3 provides a range of examples of shock leakage in the flapping mode for a jet at $NPR = 2.8$.

Figure 8. Sequence of $\textrm {d}\rho /{\textrm {d}x}$ schlieren images for a single jet at $NPR = 2.8$ with $8\ \mathrm {\mu }\textrm {s}$ spacing between subsequent frames. On the left of each panel is the raw schlieren image. On the right of each panel is the result of the Fourier filtering to preserve only components with upstream phase velocity.

3.2. Shock leakage associated with axisymmetric screech modes

Figure 9 presents a series of visualizations of shock leakage occurring at the fourth shock cell for a jet at $NPR = 2.25$, which is screeching in the ‘A2’ ($m=0$) screech mode. The phenomenon is significantly more difficult to observe here, so a schematic representation has been included in the figure to aid in interpretation. The process is consistent with the shock leakage model, and qualitatively similar to the mechanism observed in the flapping jets. Figure 9(a) shows a large toroidal vortex ring just upstream of the fourth shock cell. The Fourier-filtered image shows that the outer sections of the shock are already beginning to tilt upstream at this point. As the vortex passes the shock, the radial extension of the shock tip, followed by the emission of an upstream-propagating wave, are visible. Although the vortex is axisymmetric, as is the initial motion of the shock, in this particular image sequence the acoustic wave at the bottom of the jet is much more difficult to observe. This is emblematic of the difficulty in visualizing shock leakage in the $m=0$ mode. While at the point of generation the upstream-propagating wave is visible as a discrete wavefront, this wavefront will be generated around the entire azimuth of the jet. The schlieren image results from an integration of three-dimensional field generated by this distribution of waves, which means the initial sharp wavefront is rapidly smeared into one difficult to distinguish in a still image. The video sequence from which figure 9 is extracted is presented in supplementary movie 4.

Figure 9. Sequence of $\textrm {d}\rho /{\textrm {d}x}$ schlieren images for a single jet at $NPR = 2.25$ with $8\ \mathrm {\mu }\textrm {s}$ spacing between subsequent frames. On the left of each panel is the raw schlieren image. In the centre of each panel is the result of the Fourier filtering to preserve only components with upstream phase velocity, with the dashed red rectangle indicating the zoomed region on the right of each panel; on the right is a schematic illustrating the shape of the upstream wave.

Figure 9 was selected as it provided a reasonably clear visualization of shock leakage in the $m=0$ mode, but only at one shock tip. Figure 10 instead presents a sequence where shock leakage is visible at both the third and fourth shock cells, and on both the upper and lower sides of the jet. The resultant acoustic waves in this case are, however, far less clear. In figure 10(a), a vortex is visible having already passed the fourth shock cell. A weak shock-leakage event is highlighted at the fourth shock cell in the red circles. At the same time, a subsequent vortex is approaching the third shock cell, and in the Fourier-filtered image it can be observed that the upstream tilting of the outer portions of the third shock has commenced. In the subsequent images, even as shock leakage is occurring at the third shock cell (highlighted in figure 10e), the same vortex is beginning to induce the shock motion that is a precursor to shock leakage in the fourth shock cell.

Figure 10. Sequence of $\textrm {d}\rho /{\textrm {d}x}$ schlieren images for a single jet at $NPR = 2.25$ with $8\ \mathrm {\mu }\textrm {s}$ spacing between subsequent frames. On the left of each panel is the raw schlieren image. On the right of each panel is the result of the Fourier filtering to preserve only components with upstream phase velocity. Red circles highlight shock-leakage events.

From consideration of the cases presented here, and of the much larger dataset omitted for brevity, some general statements can be made. Firstly, during interaction with a toroidal vortex, the entire shock moves upstream at the beginning of the shock-leakage process, with the degree of motion increasing with radius. This is consistent with the observations of Panda (Reference Panda1998) and André, Castelain & Bailly (Reference André, Castelain and Bailly2012). During interaction with a vortex associated with a flapping mode, the first few shock cells could be said to undergo both a radial translation and rotation. At the later shock cells, it is debatable whether it is accurate to discuss the shock as some kind of steady-state object that undergoes periodic perturbation. Instead, as previously demonstrated in Panda (Reference Panda1998) and also evident here, in the presence of the very large vortices associated with a ‘B’ type flapping instability, the conical oblique shock that is typically assumed does not really exist in an instantaneous sense: shocks form, split, translate and dissipate during the passage of the shear-layer vortices. During the passage of a vortex, the shock forms, then translates radially outwards. The outer part of the shock rotates in the upstream direction, before a section of it propagates away from the jet entirely. In both cases, a small section of the shock tip separates from the rest of the wave and begins to propagate, spreading out cylindrically (at least in a path-integrated representation). This is consistent with the ‘shock leakage’ as first described in Manning & Lele (Reference Manning and Lele2000); interaction with the vortex leaves the shock tip beyond the sonic line, and once the vortex moves downstream, this section of the shock must become upstream propagating to satisfy the governing equations. As it propagates, it expands radially and consequently begins to decay.

The shock-leakage process is stochastic and intermittent. In the case of the flapping single jet, the degree of intermittency is difficult to assess, due to the precession of the flapping plane. However, in the $m=0$ single jet, leakage is sometimes visible from the third shock cell, sometimes the fourth, sometimes the fifth and sometimes all three. It is sometimes stronger on one side of the jet than the other. The extent of this variation is evident in supplementary movie 5, which presents a selection of recordings a jet at $NPR = 2.25$. In the twin-jet configuration the process is more regular, with leakage consistently observed at each of the shock cells visible in frame; however, large variations in the apparent strength of the resultant wave are evident in almost every image sequence. It must be emphasized that an absence of evidence is not necessarily evidence of absence; the limitations of the flow visualization technique mean that leakage may not always be observed even if it does occur. Nonetheless, it seems evident that there is variance in both the strength of the shock-leakage events and the size and strength of the shear-layer vortices. An explanation for this variation will be provided as part of § 5.

4.1. Shock-leakage processes

A time series demonstrating the leakage process is shown for $NPR = 2.25$ in figure 14, with particles from the shock-leakage model in white, overlaid on contours of the Lyapunov exponent. As noted in figure 12, the furthest upstream position at which leakage occurs is at approximately $x/D \approx 3$. In the top image of figure 14, two particle streaks are intercepting the shear layer at this point; these correspond to the oblique shocks of the fourth shock cell. At this time in the phase cycle, these streaks are encountering the saddle point between vortices of the Kelvin–Helmholtz wavepacket, where strong reductions in local vorticity occur. Between the first and second images in figure 14, this reduction in vorticity allows the streak to leak out past the shear layer, and form a wavefront at approximately $x/D \approx 3$. This wavefront is strongly reminiscent of that observed in the schlieren sequences of figure 9(c). One-third of a screech cycle later, the wave can be observed propagating in the upstream direction, at $x/D \approx 2.5$.

Figure 14. Three points in the screech phase cycle, separated by $120^{\circ }$, for a jet at $NPR = 2.25$. Colours represent magnitude of the Lyapunov exponent $\sigma$.

A similar series is presented for the $NPR = 2.10$ jet in figure 15. At this condition, the fluctuations in vorticity are significantly stronger than for $NPR = 2.25$, as will be discussed in § 5. As a consequence of this increase in vorticity fluctuation, shock leakage occurs closer to the nozzle, with the first image showing the initiation of a leakage process at $x/D \approx 1.9$. By the second image, the wavefront generated by this leakage is visible extending well outside the jet shear layer, and by the third image it is propagating back to the nozzle at $x/D \approx 1.4$. Leakage from cells further downstream is again visible in these images. An animation of both cases, showing both the results of the ray-tracing model and the contours of FTLE maxima, is presented in supplementary movie 6.

Figure 15. Three points in the screech phase cycle, separated by $120^{\circ }$, for a jet at $NPR = 2.10$. Colours represent magnitude of the Lyapunov exponent $\sigma$.

Figure 16 presents a magnified look at the fourth shock cell for $NPR = 2.25$ during a shock-leakage process. Here, the Lyapunov exponent is represented by the black contour lines, the colour contours represent coherent vorticity fluctuations and the white dots are the particles from the shock-leakage model. The behaviour described in Suzuki & Lele (Reference Suzuki and Lele2003) and Shariff & Manning (Reference Shariff and Manning2013) is evident here: when a ray streak interacts with a vortex, it is reflected back into the core of the jet. When a streak instead interacts with a saddle point between vortices, a portion may leak past the sonic line if the fluctuation in vorticity is sufficiently strong, whereupon the streak then propagates upstream. To illustrate this, two exemplar particles are tracked in time in figure 16. The green diamond tracks a particle that reaches the centre of the shear layer of the jet coinciding with the arrival of a vortex, and the red circle tracks one that reaches the centre of the shear layer at a saddle point between vortices. The strong vorticity associated with the Kelvin–Helmholtz wavepacket acts to rotate the propagation direction of the green particle, reflecting it from the shear layer and redirecting it back towards the core of the jet. By contrast, the local minima in vorticity encountered by the red particle is insufficient to achieve a total internal reflection, and the particle is instead able to leak past the sonic line and propagate upstream.

Figure 16. Demonstration that ray streaks are reflected from vortices, but leak through saddle points, at the fourth shock cell for an $NPR = 2.25$ jet. Black contours represent Lyapunov exponent $\sigma$, colour contours represent coherent out-of-plane vorticity. Green diamond and red circle each track a given particle across the three snapshots.

From the motion of the green particle in figure 16, it is evident that the interaction of the shock with the vortex drives the upstream translation of the shock, which acts as a precursor to the emission of the acoustic wave. It is, however, not until the shock encounters the saddle point between vortices that the leakage event occurs. This description appears consistent with the visualizations in figures 8 and 9; the upstream motion of the shock, as demonstrated in the Fourier-filtered images, begins with the arrival of the vortex. The emission of the upstream-propagating wave, however, does not occur until the vortex is well past the shock location; the results of the ray-tracing model provide an explanation for the observations in the high-speed schlieren.