II. Current Situation

Since 2005, compliance with IAS-IFRS standards has been compulsory for all investment vehicles quoted on European stock exchanges, including wine funds. Furthermore, the recent European directive 2011/61/EU on Alternative Investment Fund Managers (AIFM) highlights a growing interest by supranational bodies in improving transparency in the market of alternative strategies, including funds that used to be less regulated. The regulation “IFRS 13 Fair Value Measurement” took effect in January 2013. In this framework, fair value is defined as “the price that would be received to sell an asset or paid to transfer a liability in an orderly transaction between market participants at the measurement date.” For nonfinancial assets, the selected valuation method must be appropriate for the measurement, consistent with their “highest and best use.” While this notion makes sense for physical assets, such as real estate or machinery (that can be rented or exploited), the “highest and best use” of a stock held by a wine fund is limited to storing it in a well-tempered cellar or wine refrigerator. As a consequence, the fair value of a wine stock must necessarily rely on IFRS 13 (24): it should correspond to a transaction taking place in the principal market for the asset or liability or, in the absence of a principal market, in the most advantageous market for the asset or liability. Since there is no centralized, or principal, market for wine, the most advantageous market is defined as the one that maximizes the amount that would be received to sell the asset after taking into account transaction costs and transport costs (IFRS 13: A1).

Wine funds currently implement various methods to value their stocks. Table 1 presents some funds of wine as an investment and which valuation they use, if published. Most of these funds trade heavily in French wines, typically representing about 80 percent of the portfolio. Some funds, such as the Wine Investment Fund, invest almost entirely in Bordeaux.

Table 1 List of Wine Investment Funds

None of the funds appears to use a historical cost approach, in which inventories are valued at the acquisition price. On the contrary, several funds already rely on a market approach to value their stocks, even though IFRS 13 compliance is not obvious in that case. However, until 2017, auditors may sign off on a market valuation approach of a fund even where full IFRS 13 compliance has not been established. Interestingly, some funds seem to use the valuation methodology promoted by the London International Vintners Exchange (Liv-ex), an Internet and telephone transaction platform for wine professionals. The company brands itself as the “industry standard” and “the official valuer for a number of leading wine funds.”

The Liv-ex platform is organized in a similar fashion as a stock exchange: bids or offers are put on the platform by professionals. When a trade takes place, both counterparties are notified of the transaction. Within 14 days, the seller then delivers the wine to the Liv-ex warehouse, which is verified by Liv-ex. Simultaneously, the buyer sends the funds to Liv-ex, which transfers the money to the seller within three weeks, whereas the buyer can either collect the wine at the warehouse or have it delivered.

The Liv-ex exploits available information on its platform to produce valuations of wines. The valuation method is the following: After the submission of a list of wines to be valued, the exchange verifies the current best offer for each wine in its own system and at other dealers. The valuer then observes the best bid on the platform and looks at the most recent transaction (within the previous 30 days). If it lies within the bid-offer spread, then this transaction is used for valuation; otherwise, the mid-price is computed as the average between the bid and the offer. The scenario becomes more complex when no offer is available. In this case, Liv-ex relies on an undisclosed list of offer prices by merchants “identified as the major stockholders of wine.” If no offer was available in the previous 30 days neither at a dealer nor on the Liv-ex, then the valuation is performed by a “valuation committee” that uses “off-market bids and offers, historical list prices and transaction data.” If no bid is available, the bid is estimated from the average spreads to “orphan offers,” defined as “an offer price where [Liv-ex has] no corresponding bid. Orphans can be both live exchange offers or merchant list prices.” The Liv-ex does not include auction prices in its calculation “due to a lack of standardization of auction lots,” which can make weekly prices “very volatile with large swings” and because “auction commissions can vary.”

Despite being an interesting approach, the method seems to fail to satisfy requirements for fair-value computation of wine as a financial asset, especially in terms of the IFRS 13. First, although it is a very successful venture with 400 members and more than 1,000 transactions per month, there is little evidence that Liv-ex is the most advantageous market for selling any type of wine that could be held by a fund. According to Liv-ex, in 2010, Bordeaux wines accounted for 95% of its exchanges, with five Premiers Crus comprising 61% of Liv-ex trades by value: Château Lafite-Rothschild (Pauillac), Château Latour (Pauillac), Château Margaux (Margaux), Château Haut-Brion (Pessac, Graves), Château Mouton-Rothschild (Pauillac).

In 2011, more than US$150 million worth of wine was traded on the Liv-ex platform, which is a considerable amount in absolute value but undeniably smaller than the yearly US$400 million worth of transactions the same year at the major auction houses Acker Merrall and Condit, Christie's International, Sotheby's, Zachys, and Hart Davis Hart Wine Co. In some cases, depending on ask prices of dealers to estimate a bid price instead of relying on auction house transactions that are publicly available seems an unreasonable choice, given the opacity of dealer prices and the relative importance of large auction houses in the secondary market for wine (according to Liv-ex, auctions account for roughly 10% of the market), especially as far as old vintages and collectible wines are concerned.

First, as stated by Jones and Storchmann (Reference Jones and Storchmann2001), wines are “traded all over the world in established wine auctions. The system guarantees, similar to a stock market, a comparatively high price transparency. Therefore, it can be assumed that auction prices indicate the relative (economic) scarcity and therefore the international esteem for those wines.” Second, unlike auctions, the Liv-ex is based on standard contracts that assume a similar quality among wines with similar features. This approach, well suited to recent vintages, prevents investors from gaining complementary information about the condition of older wines. In the case of auctions, by contrast, Ashenfelter (Reference Ashenfelter1989) highlights that, at wine auctions, “revealing information tends to remove uncertainty and make low bidders more aggressive; this puts upward pressure on the bidding of others, which is in the interest of the auctioneer.” Similarly, Muth et al. (Reference Muth, Liu, Koontz and Lawrence2008) showed that in the market for fed cattle, auction barn prices are higher than equivalent forward prices. Pagano and Röell (Reference Pagano and Röell1996) proved that “the implicit bid-ask spread in a transparent auction is tighter than in a less transparent dealer market.” For the art market, Bocart and Oosterlinck (Reference Bocart and Oosterlinck2011) showed that large auction houses act as agents, mitigating authenticity issues.

Finally, one can reasonably question the independence of an exchange that excludes its competitors (auction houses) but includes data from its clients or prospects (dealers). The inclusion of a valuation committee in the event of the absence of data makes stakeholders clueless about the methodology and data eventually used to perform a valuation. In any case, a conflict of interest is possible between an exchange that acts simultaneously as intermediary and expert and a fund whose fee, like the exchange, depends on the price level.

III. New Approach to Valuation of Wine as an Investment

IFRS 13 provides three degrees of hierarchy in inputs that can be used for fair value measurement. The idea behind the hierarchy is that lower levels are preferred: Level 1 inputs are “quoted prices in active markets for identical assets or liabilities that the entity can access at the measurement date” (IFRS 13: 76). Level 2 inputs “are derived mainly from or corroborated by observable market data by correlation or other means (‘market-corroborated inputs’)” (IFRS 13: 81). Level 3 inputs are unobservable inputs used “with the best information available in the circumstances, which might include the entity's own data, taking into account all information about market participant assumptions that is reasonably available” (IFRS 13: 87–89).

In the case of wine, Level 1 inputs are not readily available, especially considering the fact that available exchanges (Hong Kong Wine Exchange, BWinex in the Bordeaux region, Vinetrade in Japan, and BBX and Liv-ex in the U.K., to name but a few) are highly specialized and do not represent the market with the greatest volume and level of activity for the asset or liability. Level 2 inputs, however, are accessible to wine funds since, first, they observe their own transactions, and, second, they observe prices reached at auction and on electronic platforms. Level 3 inputs are also significant for wine funds because they concern intrinsic qualities that generate profit. Indeed, their strategies often involve acquisition and selling tactics that best exploit their position in the market because they can benefit from significant economies of scale. Furthermore, they can act as liquidity providers and add a liquidity premium. They can best adjust their movements in a market prone to dysfunction, as mentioned by Ashenfelter (Reference Ashenfelter1989): “at the first wine auction I ever attended, I saw the repeal of the law of one price,” referring to the declining price anomaly in wine auctions provoked by non-optimal absentee bidders (Ginsburgh, Reference Ginsburgh1998). Naturally, funds' strategies differ. Some specifically focus on heavily traded Bordeaux wines and try to track the overall price levels, whereas others play in niche markets of collectibles. They trade intensively in the over-the-counter market for restaurants, dealers, and collectors. Our approach to fair valuation of a wine fund combines inputs from Levels 2 and 3.

Level 2 inputs consist of observed transactions, both those made by the fund and observable prices reached at auction, buyer's premium included, for identical wines. The auction market can be considered the most advantageous market because it is open to all and applies an English auction system, known to be the one that maximizes seller revenues among auction mechanisms (Lopomo, Reference Lopomo1998). Unfortunately, because of heterogeneity at auction, different prices for seemingly identical wines (e.g., with respect to domain, vintage, and format) are observed at different auction houses in the same month. A straightforward approach consists of averaging prices observed simultaneously, so as to obtain, over time, an evolution in the average price of a given wine.

(1) $${w_{it}} = \displaystyle{1 \over {N_{it}}} \sum \nolimits_j^{N_{it}} {\,p_{itj}},$$

where w _it is the value of wine i at time t, p _itj is the jth transaction of wine i sold at time t and N _it is the amount of identical wines i sold at time t.

This naive methodology suffers from various drawbacks, including sensitivity to outliers. Also, as Equation (1) can be seen as a particular case of hedonic regression whose explanatory variables consist of only a single constant term and time dummies, Bocart and Hafner (Reference Bocart and Hafner2012) show that the traditional fixed effects estimator of volatility 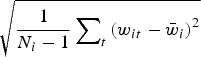$\sqrt {\displaystyle{1 \over {{N_i} - 1}} \sum \nolimits_t {\rm }{{({w_{it}} - {{\bar w}_i})}^2}} $ overestimates the volatility of the underlying value dynamics. We suggest instead the construction of a price index based on a random effect estimator of each type of wine over time. Such an estimator is naturally more robust to outliers thanks to a smoothing effect. The model is:

(2) $${\rm log}\left({\,p_{itj}} \right) = C_i + \beta _{it} + \nu _{ijt}. $$

(3) $$\beta_{it} = \beta_{it - 1} + \xi_{it}.$$

The term C _i is a constant, β _it is the margil impact of time on prices of wine i, and $\nu _{ijt} \sim N\left({0,\sigma _{\nu_i} ^2} \right)$ . In Equation (3), we suppose that β _it is a random walk, with $\xi _{it} \sim N\left( {0,\sigma _{\xi _i} ^2} \right)$ and which, for identification, is restricted to have a mean of zero.

The model can be estimated as in Bocart and Hafner (Reference Bocart and Hafner2015). At a first stage, estimate

(4) $${\rm log}\left({\,p_{itj}}\right) = C_i + \eta_{itj} $$

using ordinary least squares (OLS), where η _itj  = β _it  + ν _itj . At a second stage, estimate β _it using a Kalman filter. A wine i's fair value w _iT at time T is then estimated as

(5) $${{\tilde w}_{iT}} = \sum \nolimits_{\tau = 1}^T \sum \nolimits_{\,j = 1}^{{N_{i\tau}}} {\exp}\left({\widehat{{{\beta _{\rm \iota T}}}} - \widehat{{{\beta _{\rm \iota \tau }}}}} \right){\,p_{i\tau j}}{{\rm \lambda} _{i\tau }},$$

where N _iτ is the total number of transactions observed at auction of wine i at time τ, λ _iτ is the weight allocated to the τ-th period, allowing, for instance, more recent observations to be given more weight. The particular case ${\rm \lambda} _{i\tau} = \displaystyle{1 \over {N_{i\tau}}} \delta _{\tau _T} $ (where $\delta _{\tau _T} $ is the Kronr delta) yields the classical approach of Equation (1). Alternatively, ${\rm \lambda} _{i\tau} = \displaystyle{1 \over {TN_{i\tau}}} $ gives all past time periods equal weight in the current valuation. We use this equal weighting in our empirical example in the following section. Another direct extension of the model is aggregation of wines per domain or per vintage by including additional variables in Equation (4). Also, a predictor of future prices can be derived from the Kalman filter approach as

(6) $$\widehat{{{\log}\left( {\,p_{\iota j\left( {T + 1} \right)}} \right)}} = \widehat{{C_\iota}} + \hat \beta _{i\left( {T + 1} \right)}.$$

If predictions of the price are required, corrections such as the one proposed by Jones and Zanola (Reference Jones and Zanola2010) are straightforward to implement in order to obtain an unbiased predictor.

IV. Empirical Results

To test the suggested method, we collect data on all sales of the most popularFootnote ¹ wines sold at auction at Christie's and Sotheby's between February 2007 and December 2013.Footnote ² The database consists of 26,640 observed transactions on 232 different wines whose list is available in the online appendix. Selling prices are converted to USD/bottle. In order to mimic a wine fund's portfolio, we simulate a portfolio made up of one bottle of each of the 232 different wines available in our sample. This is purely for illustrative purposes, and clearly any other type of portfolio, for example, value weighted, could have been chosen alternatively.

Each wine is reevaluated monthly. If no observation is available for a given month, the estimated fair value of the previous month is forwarded. Annualized volatility of each wine is also computed and is 25% on average.

In the online appendix, the individual monthly valuation of each of the 232 wines is provided. As expected, the price average method resulting from Equation (1) yields an unstable valuation. At individual wine levels, unreasonable spikes can be observed. Figure 1 compares the filtered valuation of the wine with the largest number of transactions (Mouton-Rothschild 1982) with the price average over time. A clear spike is visible, due to an abnormal transaction recorded at auction, and higher volatility when the price average method is used. Such effects are smoothed in the filtered version of the valuation. If the database is cleaned for outliers by discarding, for example, the top and bottom 0.1% of observations, the spike effect diminishes, but the effect from a more volatile price average method remains.

Figure 1 Filtered Versus Classical Average Price Valuations of Mouton-Rothschild 1982

At the portfolio level, Figure 2 plots the two types of valuation for our virtual portfolio made of the cumulative value of the 232 wines over time. A dotted line indicates the classical price average methodology, whereas the plain line shows the filtered version of the valuation. The filtered version exhibits a smoother progression.

Figure 2 Filtered Versus Classical Average Price Valuations for Portfolio of All 232 Wines

Finally, a more precise estimation of returns can also show relationships and dynamics among different wines. For instance, Figure 3 illustrates a tight relationship between 1996 Bordeaux wines whose domain is located between St-Estephe and Margaux in the Médoc region. These similar patterns are in sharp contrast with those for other Bordeaux wines (see Figure 4), such as those located farther south in the Pessac-Leognan area (Haut-Brion and Mission Haut-Brion) or even farther east in the Pomerol area. These nuances are not captured by the classical estimator in Equation (1). In the context of valuing a portfolio, such an analysis can be useful, for instance, in creating peer groups with corresponding price indices.

Figure 3 Monthly Price Indexes of Selected Wines from the Area Between St-Estephe and Margaux, 1996 Vintage

Figure 4 Monthly Price Indexes of Selected Wines from Outside the Area Between St-Estephe and Margaux, 1996 Vintage

Source: Map created by Ralf Powierski (www.info-graphic.de).

Figure 5 Map of the Bordeaux Region