Introduction
Freezing tolerance of lichens manifests either in their resistance to low temperature or to the length of the low temperature period. Some lichen species may survive the liquid nitrogen temperature (-196°C) regardless of the rate of cooling, e.g. two species of the genus Umbilicaria (U. decussata (Vill.) Zahlbr. and U. vellea (L.) Ach.) survive slow cooling down to -196°C and fast cooling down to -78°C, or to -50°C (Kappen Reference Kappen1993). The prolonged frozen storage (3.5 years at -60°C) of Alectoria ochroleuca (Hoffm.) Massal. does not change its basic photosynthetic and respiratory responses after 12 hours incubation at an elevated temperature (Larson Reference Larson1978), and Cladonia foliacea (Huds.) Willd. (= Cladonia alcicornis) recovers its photosynthetic activity after prolonged freezing (96 and 110 weeks at -15°C) (Lange Reference Lange1966).
Although in C. foliacea the photosynthetic activity was detected at -24°C in laboratory conditions (Lange Reference Lange1965), the lowest temperature (-17°C) for CO2 exchange rates in the natural habitat (Cape Geology, Antarctica) was recorded for Umbilicaria aprina Nyl. thalli. The dark respiration of U. aprina significantly decreased at -7°C and finally ceased at -11°C (Schroeter et al. Reference Schroeter, Green, Kappen and Seppelt1994). Light maximum value of net photosynthesis declined with temperature in two steps: i) rapidly, between -1°C and -9°C to about 10% of the rate at +1°C, and then ii) more slowly, to very low rates at -17°C. They suggested that a two-step mechanism of net photosynthesis decline with decreasing temperature might be the result of increase of CO2 diffusion resistance, possibly by the ice nucleation activity (INA), which occurs in water saturated thalli of Umbilicaria aprina at -5.4°C (Schroeter & Scheidegger Reference Schroeter and Scheidegger1995).
Many lichen species reveal biological INA in thallus liquid extracts at temperatures much higher than the low temperature limit of their photosynthetic activity (Nash et al. Reference Nash III, Kappen, Loesch, Larson and Matthes-Sears1987, Kieft Reference Kieft1988, Kieft & Ahmadjian Reference Kieft and Ahmadjian1989, Kieft & Ruscetti Reference Kieft and Ruscetti1990, Schroeter & Scheidegger Reference Schroeter and Scheidegger1995). The stimulation of ice crystallite growths in extracellular spaces and/or guts is also one of the effective strategies used by some arthropods to deal with extremely low temperatures (Zachariassen Reference Zachariassen1989, Block et al. Reference Block, Grubor-Lajsic and Worland1993, Worland et al. Reference Worland, Block and Rothery1993, Block Reference Block1995).
The effectiveness of photosynthetic activity of U. aprina far below 0°C focused our attention on the bound water behaviour at temperatures as low as -65°C, and the thermal immobilization of various water fractions differentiated by the proximity to the thallus surfaces. As the mild dehydration of intracellular space (Harańczyk et al. Reference Harańczyk, Gaździński and Olech2000a, Reference Harańczyk, Gaździński and Olech2000b) (often accompanied by the stimulated ice nucleation in extracellular spaces (Schroeter & Scheidegger Reference Schroeter and Scheidegger1995, Harańczyk et al. Reference Harańczyk, Grandjean and Olech2003a, Reference Harańczyk, Grandjean, Olech and Michalik2003b)) is one of the ways to deal with freezing, the observation of thermal behaviour of water bound in thalli, dehydrated to very low water level, is expected to provide additional insight into this very interesting survival mechanism.
We applied techniques involving hydration kinetics, sorption isotherm, proton nuclear magnetic resonance (NMR) high power relaxometry, proton NMR spectra, and differential scanning calorimetry (DSC) to study bound water freezing in the lichen Umbilicaria aprina.
Materials and methods
Sampling was carried out in Schirmacher Oasis, Dronning Maud Land, in the summer 2003–04, during the 23rd Indian Antarctic Expedition. Umbilicaria aprina thalli were collected from temporary streams flowing from melting glaciers. In such an untypical habitat lichens grow on boulders and pebbles immersed in water during the short summer period, and are covered by ice in the winter.
Air-dry thalli were stored at p/p0 ≈ 40% and at room temperature with the hydration level Δm/m0 = 0.096 ± 0.010, where m0 is the dry mass of the sample, and Δm is mass of water taken up.
Before hydration, air-dry thalli were incubated for 164 hours over silica gel (p/p0 = 0%), dehydrating to the hydration level of Δm/m0 = 0.048 ± 0.004, then pieces were placed in NMR tubes. The samples to be used for DSC scans were hydrated prior to sealing in DSC capsules.
The samples were hydrated from the gaseous phase over the water surface (p/p0 = 100%) for t = 4.5 h (Δm/m0 = 0.125 ± 0.006), for 22.5 h (Δm/m0 = 0.173 ± 0.010), for 37 h (Δm/m0 = 0.213 ± 0.008), and for 55.5 h (Δm/m0 = 0.332 ± 0.008).
The vitality tests performed using methylene blue staining showed that no less than 68 ± 5% photobiont cells were alive either in thalli samples which had been used in NMR or in those after 21 days in the capsules used for DSC courses. After completing the temperature courses, the dry mass of the thallus was determined after heating at 70°C for 72 h. Higher temperatures were not used as they may cause the decomposition of some organic constituents of the thallus (Gaff Reference Gaff1977).
Proton free induction decays (FIDs) were recorded on WNS HB-65 high power relaxometer (Waterloo NMR Spectrometers, St Agatha, Ontario, Canada). The resonance frequency was 30 MHz (at B0 = 0.7 T), the transmitter power was 400 W, the pulse length 
/2 = 1.4 − 1.5 μs. The high power of the pulse allowed us to observe the total proton signal. FIDs were acquired using a Compuscope 2000 card in an IBM clone, controlling the spectrometer, and averaged over 2000 accumulations. Repetition time was 2.003 s. The temperature was varied between 25°C and -60°C and was stabilized in a gaseous nitrogen stream.
Proton spectra were acquired with a Bruker Avance III spectrometer (Bruker Biospin), designed for solids, operating at the resonance frequency 300 MHz (at B0 = 7 T), with the transmitter power used equal to 400 W. The pulse length was 
/2 = 1.5 μs, dead time was 7.5 μs, and repetition time was 2 s.
The data obtained were analysed using the FID analysing procedure of a two-dimensional (in time domain) NMR signal-analysing program CracSpin written at the Jagiellonian University, Cracow (Węglarz & Harańczyk Reference Węglarz and Harańczyk2000).
Differential scanning calorimetry scans were performed in Perkin Elmer DSC 8000 calorimeter using 30 μl aluminum pans. The calorimeter was calibrated by means of the melting points of indium and water. The mass of the sample used was c. 8 mg. Differential scanning calorimetry curves were recorded while cooling from room temperature down to -70°C, followed by heating of the samples, returning to the initial temperature. The heating/cooling rates were varied between 0.1 and 20°C min-1. Since the peak onset temperature was almost independent of the rates tested, a rate of 20°C min-1 was used. Onset and peak temperatures as well as transition enthalpies have been calculated by Perkin Elmer software.
Results
Proton free induction decays
Figure 1 shows the proton FID for Umbilicaria aprina hydrated to Δm/m0 = 0.097. The FID is composed of a solid component and two exponential components from the protons of thallus liquids. The solid signal, with the amplitude S, deriving from protons of the solid matrix of the thallus may be fitted either by the Gaussian (Eq. (1a)) or Abragam (Eq. (1b)) function (Abragam Reference Abragam1961). The exponential components are interpreted as coming from protons of water tightly or loosely bound in the thallus with the amplitudes equal to L1 and L2, respectively (Harańczyk et al. Reference Harańczyk, Bacior, Jastrzębska and Olech2009a).
![\[--><$$>\eqalign {FID(t)\, = &\,S\, \cdot \,\exp \left( {{\rm{ {\hbox {-}} }}{{{\left( {\frac{t}{{T_{{\it 2S}}^{\ast} }}} \right)}}^2} } \right)\,+ \,{{L}_1}\, \cdot \,{\rm{exp}}\left( {{\rm{ \hbox{-} }}\frac{t}{{T_{{ \it 2{{L}_1}}}^{\ast} }}} \right)\, \cr &+ \,{{L}_{2}}\, \cdot \,{\rm{exp}}\left( {{\rm{ \hbox{-} }}\frac{t}{{T_{{\it 2{{L}_2}}}^{\ast} }}} \right).\qquad\qquad\qquad\qquad\quad\ {\rm(1a)}\eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU1.gif?pub-status=live)
![\[--><$$>\eqalign {FID(t)\, = &\,S \,\cdot \,\exp \left( {{\rm{ \hbox {-} }}{{{\left( {\frac{t}{{T_{{\it 2S}}^{\ast} }}} \right)}}^2} } \right)\frac{{\sin (at)}}{{at}}\, + \,{{L}_1} \cdot \exp \left( {{\rm{ \hbox {-} }}\frac{t}{{T_{{\it 2{{L}_1}}}^{\ast} }}} \right)\, \cr &+ \,{{L}_{2}}\, \cdot \,\exp \left( {{\rm{ \hbox {-} }}\frac{t}{{T_{{\it 2{{L}_2}}}^{\ast} }}} \right). \qquad\qquad\qquad\qquad\quad{\rm(1b)}\eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU2.gif?pub-status=live)
Fig. 1 a. Proton free induction decay recorded for Umbilicaria aprina Nyl. thalli at 30 MHz, with the pulse length 
/2 = 1.4 μs at the temperature t = 24°C. The relative mass increase was Δm/m0 = 0.097. b. The residual function calculated as the difference between the fitted and recorded values of the FID signal, which for any recorded point does not exceed 4.3%.
is the transverse proton relaxation time of the solid component taken as the 1/e-value of Gaussian solid signal, and 
and 
are the transverse proton relaxation times of the liquid-like magnetization components L1 and L2, respectively.
The relaxation time 
for the Gaussian component does not vary much with thallus hydration level (Harańczyk et al. Reference Harańczyk, Bacior and Olech2008), suggesting that the structure and molecular dynamics of the thallus solid matrix is hardly modified by the hydration process. Thus, the solid signal amplitude, S, may be used as a reference amplitude to scale the amplitudes of liquid components.
The L2 signal comes from water loosely bound on thallus surface and for higher hydration level from free water fraction. The 
≈ 510 μs is shortened by B0 inhomogeneities, according to Timur (Reference Timur1969).
![\[--><$$> {\rm{1/}}{{T}_{{\it 2}}^{\ast}} \,{\rm{ = }}\,{\rm{1/}}{{T}_{\it 2}}\,{\rm{ + }}\,\rgamma \rDelta {{B}_{\it0}}{\rm{/2,}} \eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU6.gif?pub-status=live){kind=small}
where T2 is spin-spin relaxation time, γ is gyromagnetic ratio, and ΔB0 is a change of magnetic field B0 within the sample. The solid components and the short exponential components are negligibly affected by the B0 inhomogeneities, but T2 for the L2 component, associated with loosely bound water pools (e.g. extra- and intramolecular loosely bound water fractions) is much more shortened by ΔB0 (Eq. (2)).
Proton NMR spectra
Figure 2 shows proton NMR spectra recorded at 24°C for U. aprina thallus at various hydration levels. The line is composed of the broad solid component successfully fitted by the Gaussian function and the narrow component coming from water bound in U. aprina thallus. Gaussian approximation resembles the ‘hat-like’ function which is the Fourier transformation of Abragam function detected in time domain for FIDs (Derbyshire et al. Reference Derbyshire, van Den Bosch, van Dusschoten, MacNaughtan, Farhat, Hemminga and Mitchell2004).
Fig. 2 Proton spectra detected for Umbilicaria aprina Nyl. thalli at 300 MHz, with the pulse length 
/2 = 1.5 μs at the temperature t = 24°C. The relative mass increase was a. Δm/m0 = 0.119, b. Δm/m0 = 0.178, c. Δm/m0 = 0.235, and d. Δm/m0 = 0.358.
Proton magnetic resonance spectra recorded for the pulse with the power sufficient to detect solid and liquid signal components for U. aprina thalli are the superpositions of the solid component, well fitted by a Gaussian function, and the liquid component fitted by Lorentzian function:
![\[--><$$>\eqalignno {A{\rm{(}}\upsilon {\rm{)}}\,{\rm{ = }}\,&{{A}_S}{\rm{/\rDelta }}{{\upsilon }_{G \cdot }}\surd \pi {\rm{/2}}\,{\rm{ exp( {\hbox -} 2(}}\upsilon \,{\rm{ \hbox {-} }}\,{{\upsilon }_G}{\rm{/\rDelta }}{{\upsilon }_G}{{{\rm{)}}}^{\rm{2}}} {\rm{)}}\cr &\,{\rm{ + }}\,{\rm{2}}{{A}_L}{\rm{/}}\pi {\rm{(\rDelta }}{{\upsilon }_L}{\rm{/4(}}\upsilon \,{\rm{ \hbox {-} }}\,{{\upsilon }_L}{{{\rm{)}}}^{\rm{2}}} \,{\rm{ + }}\,{\rm{\rDelta }}{{\upsilon }_L}^{2} {\rm{),}} <$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU7.gif?pub-status=live){kind=small}
where ΔυG and ΔυL are the half-widths for the Gaussian and Lorentzian components of the NMR line, respectively, υG and υL are Gaussian and Lorenztian peak positions, respectively, and finally AS and AL are the amplitudes of the Gaussian and Lorentzian peaks, respectively.
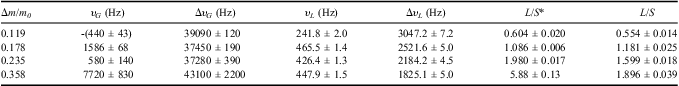
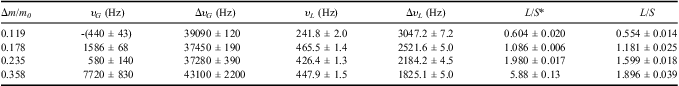
Table I gives the parameters obtained by fitting Eq. (3) to the spectra. The half-width of the solid Gaussian line component increased from ΔυG = 37 kHz up to 43 kHz, with hydration level increase from Δm/m0 = 0.119 up to 0.358, whereas for the liquid component ΔυL increased from 1.8 kHz to 3.0 kHz for the same change in hydration level. The liquid to solid ratios, L/S, obtained in the time domain are similar to those obtained in the frequency domain. An exception is noted for the spectrum recorded for the thallus hydrated to Δm/m0 = 0.358, for which the area under the solid peak is decreased. This may be an effect of a ripple form of baseline caused by phase drift.
Table I The parameters of 1H-NMR spectra recorded for Umbilicaria aprina Nyl. thalli at temperature t = 24°C. The samples were hydrated to Δm/m0; peak position are υG and υL for Gaussian and for Lorentzian line components respectively; line half-widths are ΔυG and ΔυL for Gaussian and Lorentzian line components, respectively; L/S* is the ratio of the area under the liquid line to the area under the solid line; L/S is the ratio of the liquid signal amplitude to solid amplitude, recorded in time domain (FID). Similar hydration levels were used in these two sets of experiments. The peak positions were scaled to the resonance frequency, υ0 = 300131000.0 Hz.
The relaxation time for the solid (Gaussian) component, 
, may be calculated from
![\[--><$$> {{T}_{{\it 2G}}^{\ast}} \,{\rm{ = }}\,\surd 2 {\rm{/}}\pi {\rm{.\rDelta }}{{\upsilon }_G}{\rm{,}} \eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU8.gif?pub-status=live){kind=small}
whereas the relaxation time for the liquid component, 
, from
![\[--><$$> {{T}_{{\it 2L}}^{\ast}} \,{\rm{ = }}\,1{\rm{/}}\pi {\rm{.\rDelta }}{{\upsilon }_L}{\rm{.}} \eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU9.gif?pub-status=live){kind=small}
The values of relaxation times calculated from the parameters fitted for proton spectra (Eqs (4) & (5)), in the frequency-domain experiment, agree with those fitted from proton FIDs, in the time-domain measurement. If the relaxation times are defined for the half-intensity value of the line, for the solid component they vary from 
= 10 μs up to 12 μs, whereas for liquid component 
= 104 − 174 μs. If the relaxation times are recalculated for 1/e value, for the solid component they vary from 14.5 μs up to 17 μs, which is close to the values obtained in time-domain experiment. The difference between the relaxation times for the liquid component, 
, is greater than that for the solid component and is caused by higher value of ΔB0 (Eq. (2)) in the frequency-domain experimental setup.
Temperature dependency of proton FID parameters
Figure 3 shows the temperature dependencies of the parameter a for the U. aprina thalli at the hydration level varied from Δm/m0 = 0.125 up to 0.332. For given temperature the value of a parameter depends on hydration level of thallus only weakly, suggesting that for hydration levels between Δm/m0 = 0.125 and 0.332 the hydration process does not much modify the solid matrix of thallus. In the temperature range from 25°C to -35°C the solid component line widths (proportional to the value of a) remains unchanged, which indicates the absence of phase transitions. For the temperatures below -35°C the solid component NMR line becomes broader, which means the immobilization of some protons of the thallus solid matrix. The line widths increase up to ΔυG = 35 kHz.
Fig. 3 The temperature dependence of the parameter a for Abragam function fitted to solid component signal (thallus solid matrix line widths) calculated for Umbilicaria aprina Nyl. thalli with various hydration levels: Δm/m0 = 0.125 (squares), Δm/m0 = 0.173 (upward triangles), 0.213 (downward triangles), and 0.332 (circles). Open symbols = cooling down, dotted symbols = heating up.
Figure 4a–d presents the Arrhenius plots of the proton spin-spin relaxation times for all FID signal components detected in U. aprina thalli hydrated to Δm/m0 = 0.125, 0.173, 0.213, and Δm/m0 = 0.332, respectively. For the temperatures higher than -40°C the liquid part of FID signal is fitted by two exponentials L1 and L2 coming from water tightly bound and loosely bound to the surfaces of the thallus, respectively. For the sample hydrated to the highest hydration level (Δm/m0 = 0.332) and for the temperatures above -11°C only one exponential component L2, coming from loosely bound water, was detected. As the signal of L2 decreased with the decreasing temperature, below -11°C only the smaller amplitude, the tightly bound water component, L1, was fitted. Below -40°C, for all hydration levels investigated, the loosely bound water component, L2, was not detected, leaving only the exponential FID component of tightly bound water.
Fig. 4 a–d. The temperature dependence of proton FID relaxation times for Umbilicaria aprina Nyl. thalli hydrated to Δm/m0 = 0.125, 0.173, 0.213, and to Δm/m0 = 0.332, respectively. Solid Gaussian component (S) = closed circles, tightly bound water component (L1) = open triangles, and loosely bound water fraction (L2) = open squares. For temperatures below -40°C the loosely bound water signal component was not detected. Dotted symbols for heating up of the samples.
For the solid signal component the relaxation time, 
, does not vary much with the temperature (Fig. 3), suggesting that the structure of the solid matrix of the thallus is not appreciably modified by the hydration process (Harańczyk et al. Reference Harańczyk, Bacior and Olech2008). Thus we used solid signal amplitude, S, to scale the amplitudes of liquid components for every given temperature.
The temperature dependence of total liquid signal, expressed in the units of solid signal, (L1 + L2)/S, is shown in Fig. 5 (open circles). The amplitude of liquid signal decreases continuously with temperature decrease. The co-operative bound water freezing should manifest as the discrete decrease in the proton liquid signal, which was not observed. With decreasing temperature the contribution of the tightly bound water signal, L1, increases at the expense of the loosely bound water signal L2 and below -40°C the L2 component is no longer detected. The change occurs smoothly over broad temperature range.
Fig. 5 The total liquid signal, (L1+L2)/S (open circles), L1/S (open triangles), and L2/S (open squares), temperature dependency for Umbilicaria aprina Nyl. thalli hydrated to a. Δm/m0 = 0.125, b. Δm/m0 = 0.173, c. Δm/m0 = 0.213, and d. Δm/m0 = 0.332, respectively. Dotted symbols for heating courses.
DSC temperature scans
The differential scanning calorimetry reveals bound water freezing in U. aprina thalli, at a temperature which did not change with the cooling speed being varied from 0.1°C min-1 up to 20°C min-1. The onset temperatures were -15.68°C and -15.52°C, for thalli hydrated to Δm/m0 = 0.70, and to 0.76, respectively (see Fig. 6a & b). Thus, all other temperature trials were performed with the cooling/heating rate equal to 20°C min-1.
Fig. 6 Calorimetric scans of freezing (peak down) and melting (peak up) of water bound in Umbilicaria aprina Nyl. thalli recorded at two different rates, a. 0.1°C min-1, and b. 20°C min-1. The hydration level of the thallus was Δm/m0 = 0.76, and 0.70, respectively.
For U. aprina thalli the onset temperatures for melting and for freezing (Fig. 7) depend linearly on the thallus hydration level, according to the formulae
![\[--><$$>{{t}_m}\left[ {^\circ C} \right]\, = \,\left( {30.7\, \pm \,5.4} \right) \cdot {\rm{\rDelta }}m/{{m}_0}\,{\rm{ \hbox {-} }}\,\left( {{\rm{28}}{\rm{.5}}\, \pm \,{\rm{20}}{\rm{.6}}} \right),\eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU10.gif?pub-status=live){kind=small}
![\[--><$$> {{t}_f}\left[ {^\circ C} \right]\, = \,\left( {24.1\, \pm \,4.2} \right) \cdot {\rm{\rDelta }}m/{{m}_0}\,{\rm{ \hbox {-} }}\,\left( {{\rm{34}}{\rm{.5}}\, \pm \,{\rm{2}}{\rm{.4}}} \right). \eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU11.gif?pub-status=live){kind=small}
Fig. 7 The bound water freezing (open squares) and melting (open triangles) onset temperatures recorded for Umbilicaria aprina Nyl. thalli as a function of the relative mass increase Δm/m0.
The linear dependence of water melting temperature on hydration level correlates the freezing onset with the sizes of water compartments in porous material (Harańczyk Reference Harańczyk2003, and references therein). This means that heterogeneous ice nucleation is responsible for bound water freezing in U. aprina thallus.
The estimated bound water melting temperature for zero-hydration of the thallus equals tm = -(28.5 ± 2.6)°C, whereas the freezing temperature equals tf = -(24.1 ± 4.2)°C. For melting onset and for freezing onset the estimated temperatures are substantially higher than the singularity temperature of water (Angell Reference Angell1982).
The transition enthalpy, ΔHf and ΔHm, of freezing and of melting (see Fig. 8), respectively, linearly depends on hydration level of U. aprina thallus (Eq. (7a) & (7b)):
![\[--><$$>{\rm{\rDelta }}{{H}_f}{\rm{(}}J{\rm{/}}g{\rm{)}}\,{\rm{ = }}\,{\rm{(148}}{\rm{.5}}\, \pm \,1{\rm{3}}{\rm{.4)}}{\rm{.\rDelta }}m{\rm{/}}{{m}_{\it0}}\,{\rm{ {\hbox-} }}\,{\rm{(30}}{\rm{.0}}\, \pm \,{\rm{7}}{\rm{.2),}}\eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU12.gif?pub-status=live){kind=small}
![\[--><$$>{\rm{\rDelta }}{{H}_m}{\rm{(}}J{\rm{/}}g{\rm{)}}\,{\rm{ = }}\,{\rm{(139}}{\rm{.7}}\, \pm \,{\rm{3}}{\rm{.8)}}{\rm{.\rDelta }}m{\rm{/}}{{m}_{\it0}}\,{\rm{ {\hbox-} }}\,{\rm{(17}}{\rm{.8}}\, \pm \,3.{\rm{8)}}{\rm{.}}\eqno\rm<$$><!--\]](https://static.cambridge.org/binary/version/id/urn:cambridge.org:id:binary:20160316070717030-0422:S0954102012000041_eqnU13.gif?pub-status=live){kind=small}
Fig. 8 The transition enthalpy for bound water freezing (open squares) and melting (open triangles) for Umbilicaria aprina Nyl. thalli as a function of the relative mass increase Δm/m0.
In the linear approximation, the hydration level for which the transition enthalpy, ΔH, reaches zero with decreasing hydration level equals Δm/m0 = 0.202 ± 0.050 for cooling (for freezing), and Δm/m0 = 0.127 ± 0.027 for heating of the sample (for melting). This value approximates the minimal hydration level at which the co-operative freezing of bound water occurs.
Freeze-thaw cycles
Calorimetric freeze-thaw scans were performed between room temperature and -70°C. The number of cycles was n = 22. The melting temperature, tm, slightly decreases with the number of the cycle, whereas a change in freezing temperature, tf, is not seen (Fig. 9). The temperature difference between bound water melting and bound water freezing onsets, tm - tf, decreases for n ≤ 5 cycles, and remain constant (tm - tf = (8.0 ± 0.2)) for n > 5 cycles (Fig. 10). This suggests that the structural changes in the thallus appear only if the temperature is periodically changed not too many times. This may reflect the adaptation of thallus to subsequent freeze-thaw cycles.
Fig. 9 The freezing (open squares) and melting (open triangles) peak temperatures recorded as a function of freeze-thawing cycles for Umbilicaria aprina Nyl. thalli hydrated to Δm/m0 = 0.744.
Fig. 10 The difference between bound water melting and water freezing onset temperatures, tm - tf, detected as a function of freeze-thawing cycles for Umbilicaria aprina Nyl. thalli hydrated to Δm/m0 = 0.744.
Discussion
In many biological microheterogeneous systems, e.g. in dentine, dental enamel, shells of molluscs, bark and bast (e.g. Harańczyk et al. Reference Harańczyk, Gaździński and Olech1998, and references therein), the solid signal is usually nearly Gaussian in form, with the relaxation time, 
, close to the value obtained by us for U. aprina thallus. This suggests similar distribution of local magnetic fields in organic matter (Pintar Reference Pintar1991). The ‘beat pattern’ observed in the solid part of FID signal suggests that the solid component contribution may be successfully fitted by an Abragam function (Derbyshire et al. Reference Derbyshire, van Den Bosch, van Dusschoten, MacNaughtan, Farhat, Hemminga and Mitchell2004). The parameter a in Abragam function yields the half-widths of the NMR line solid component as: Δω = 2.a (ΔυG = a/
). The ‘beat pattern’ in FID function manifests itself in frequency domain as a ‘hat-like’ form of the solid line component (Derbyshire et al. Reference Derbyshire, van Den Bosch, van Dusschoten, MacNaughtan, Farhat, Hemminga and Mitchell2004). The observed water fractions are differentiated by their mobility and, thus, by their binding and/or proximity to the thallus surfaces, which means that intracellular water as well as extracellular water fraction usually contributes to both these water fractions. In proton FID the 
≈ 60 μs of the L1 component recorded for U. aprina is close to that recorded for tightly bound water of lichen thalli (Harańczyk et al. Reference Harańczyk, Gaździński and Olech2000a, Reference Harańczyk, Leja and Strzałka2006a, Reference Harańczyk, Bacior, Jastrzębska and Olech2009a), as well as of biological macromolecules (Harańczyk et al. Reference Harańczyk, Czak, Nowak and Nizioł2010) or photosynthetic membranes (Harańczyk et al. Reference Harańczyk, Leja, Jemioła-Rzemińska and Strzałka2006b, Reference Harańczyk, Pietrzyk, Leja and Olech2009b, Reference Harańczyk, Bacior, Jamróz, Jemioła-Rzemińska and Strzałka2009c).
The upper limits of hydration levels in situ for lichens of the genus Umbilicaria significantly exceed the hydration level obtained by us for U. aprina hydrated from gaseous phase, e.g. for Umbilicaria spodochroa (Hoffm.) DC. in Lam. & DC. Δm/m0 varies between 1.0 and 5.0 (Kappen et al. Reference Kappen, Schroeter, Hestmark and Winkler1996a), for U. cinereorufescens (Schaer.) Frey. Δm/m0 = 1.55, and for U. polyrrhiza (L.) Fr. Δm/m0 = 3.10 (Valladares et al. Reference Valladares, Sancho and Ascaso1998). For U. decussata the optimal net photosynthesis is recorded at a lower hydration level of Δm/m0 = 1.0, for U. aprina at Δm/m0 = 1.2, for Usnea antarctica Du Rietz and for Usnea sphacelata R. Br. at Δm/m0 = 0.85 (Kappen & Breuer Reference Kappen and Breuer1991), which are values closer to those used in our experiments.
Polyhydric alcohols and simple sugars may act as cryoprotectants for Antarctic arthropods and for a variety of plants (Kaurin et al. Reference Kaurin, Juttila and Hansen1981, Block & Sømme Reference Block and Sømme1983, Duman Reference Duman1984). Polyol content in Antarctic lichen thalli, as identified by 13C-NMR, varied between 17 mg g-1 for Candelariella flava (Dodge & Baker) Castello & Nimis (= Candelariella hallettenensis (Murray) Ørsted) and 65 mg g-1 for Usnea antarctica, with dominating contributions from arabitol, mannitol and ribitol, whereas sorbitol was not detected. Sugar content was approximately one order of magnitude smaller than the content of polyols (Chapman et al. Reference Chapman, Roser and Seppelt1994). The polyols (ribitol, mannitol, arabitol), as essential metabolites, were present in thalli of Evernia esorediosa (Müll. Arg.) Du Rietz, in Ramalina subbreviuscula Asah., and in Ramalina sublitoralis Ash., at constant level (up to 3.4% w/w of arabitol in Ramalina subbreviuscula) under field conditions. Glucose and fructose were not found, and the significant content of monosugars was only forced by external osmotic conditions (Hamada et al. Reference Hamada, Okazaki and Shinozaki1994). For Umbilicaria decussata the carbohydrate level changes only slightly with the thallus hydration level (Melick & Seppelt Reference Melick and Seppelt1994).
The cryoprotective action of polyols is based on blocking the formation of ice crystallites by steric mismatch of hydrogen bonds which may be formed between them and water. Although the overall concentration of polyols detected in the thallus is not sufficient to promote a significant decrease of freezing point of cellular aqueous medium, if polyols are localized mainly in intracellular spaces, they may contribute to the freezing protection mechanism in lichens.
The observed transformation of bulk water to less mobile fraction during the cooling of Umbilicaria aprina thalli suggests that the steric mismatch is not the only effect responsible for the freezing resistance of lichens. If the freezing protection mechanism was based on steric effects alone, drastic changes in motional properties of bound water should not be observed. The immobilization of water suggests the presence of an active mechanism, based on formation of a long range molecular network, increases the area of liquid-solid interface, thus increasing the tightly bound water amount, e.g. a gel-like structure from liquid substances formation.
The mechanism of water transfer from loosely bound, freezing fraction to tightly bound, non-freezing fraction occurring with decreased temperature was observed in fruticose lichens, in Cladonia mitis Sandst., Himantormia lugubris (Hue) I. M. Lamb, and Usnea aurantiaco-atra (Jacq.) Bory (Harańczyk et al. Reference Harańczyk, Gaździński and Olech2000a, Reference Harańczyk, Grandjean, Olech and Michalik2003b). We present here the first observation of loosely bound to tightly bound water transfer in a lichen with a foliose thallus.
The solid component immobilization detected as increase in parameter a of Abragam function describing the solid line component in time domain (FID), may be connected with the discontinuous increase in activation energy at -30°C, seen on the Arrhenius plot for proton spin-lattice relaxation time T1 in dry Cladonia mitis thalli (Harańczyk et al. Reference Harańczyk, Gaździński and Olech2000b). The phase transition in Cladonia mitis was not accompanied by the co-operative bound water immobilization (freezing), which was also observed by us in U. aprina. Although there is no evidence on phase transitions in the lichen thallus, there are some reports on phase transitions from liquid crystalline to gel phase occurring for some fraction of lipids, e.g. lipid liposomes, formed from the extracted spinach lipids at -30°C and at -40°C (Jensen et al. Reference Jensen, Heber and Oettmeier1981), in tomato chloroplast lipids at -29°C (Graham & Patterson Reference Graham and Patterson1982), and in soya lecithin between -20°C and -30°C (Quinn & Williams Reference Quinn and Williams1983).
The temperature for freezing of bound water detected in the present study (Fig. 7) for U. aprina thallus was far below the value -6°C reported by Schroeter et al. (Reference Schroeter, Green, Kappen and Seppelt1994). Although they used differential thermal analysis (DTA) which is a more sensitive method, it does not provide quantitative parameters for thermal peaks, which DSC does. We suggest that the difference cannot be caused by the method of the measurement. The dependence of the bound water freezing temperature on hydration suggests a different molecular mechanism for the freezing process. The linear dependency of the freezing temperature of supercooled water on hydration is characteristic for heterogeneous nucleation of ice crystallites (Angell Reference Angell1982, and references therein). In U. aprina thallus the freezing temperature dependence on hydration level is approximately linear, reflecting the real size distribution of water micro-compartments filled with increased water content. However, this observation indicates that the mechanism of stimulated ice nucleation did not act in U. aprina harvested from glacial streams in Schirmacher Oasis. This observation suggests that U. aprina may change its survival strategy from freezing tolerance to freezing avoidance.
Insects are able to fundamentally change their survival strategy (Block Reference Block1995). Larvae of two beetle species, Cucujus clavipes Fabr., and Dendroides canadensis Latr., populating the same sites in northern Indiana, switched from freeze tolerance to freeze avoidance in a relatively short period of time. The change in strategy was induced by a sequence of mild winters and probably occurred between the winters 1979–80 and 1980–81 when ice nucleating agents were lost (Duman Reference Duman1984, Horwath & Duman Reference Horwath and Duman1984). However, the molecular mechanism triggering the change of the survival strategy from freezing tolerance to freezing avoidance is not yet known either for insects or for lichens.
Acknowledgements
The research was carried out with the equipment purchased thanks to the financial support of the European Regional Development Fund in the framework of the Polish Innovation Economy Operational Program (contract no. POIG.02.01.00-12-023/08). We are thankful to colleagues from Maitri Station and participants of the 23rd Indian Antarctic Expedition for their help during the field studies. We would like to thank the reviewers for their comments and suggested improvements.
