No CrossRef data available.
Article contents
Expansion properties of double standard maps
Published online by Cambridge University Press: 05 July 2022
Abstract
For the family of double standard maps 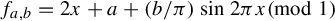 $f_{a,b}=2x+a+({b}/{\pi }) \sin 2\pi x \pmod {1}$ we investigate the structure of the space of parameters a when
$f_{a,b}=2x+a+({b}/{\pi }) \sin 2\pi x \pmod {1}$ we investigate the structure of the space of parameters a when 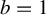 $b=1$ and when
$b=1$ and when 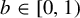 $b\in [0,1)$. In the first case the maps have a critical point, but for a set of parameters
$b\in [0,1)$. In the first case the maps have a critical point, but for a set of parameters 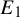 $E_1$ of positive Lebesgue measure there is an invariant absolutely continuous measure for
$E_1$ of positive Lebesgue measure there is an invariant absolutely continuous measure for  $f_{a,1}$. In the second case there is an open non-empty set
$f_{a,1}$. In the second case there is an open non-empty set 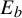 $E_b$ of parameters for which the map
$E_b$ of parameters for which the map 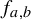 $f_{a,b}$ is expanding. We show that as
$f_{a,b}$ is expanding. We show that as  $b\nearrow 1$, the set
$b\nearrow 1$, the set 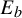 $E_b$ accumulates on many points of
$E_b$ accumulates on many points of  $E_1$ in a regular way from the measure point of view.
$E_1$ in a regular way from the measure point of view.
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press



