I. Introduction
Growers' vineyard practices are one of the main determinants of grape quality.Footnote 1 But wineries face a classic agency relationship with its growers—that is, it is in the cooperative's (winery) interest for growers to deliver quality grapes. However, two problems arise: first, wineries are unable to observe the grower's effort level (moral hazard). Their remuneration is based only on proxies—that is, observable indicators—of the grape quality after they are delivered to the winery. Second, the growers have different levels of ability and productivity (adverse selection).
In this paper, we study a wine cooperative in southwestern France that implements an incentive compensation system to induce growers to make the maximum effort toward the achievement of quality. To do so, it has created a differentiated payment system and a system of promotions. Compensation is not based on the absolute quality of their grape but, rather, on a relative performance scheme, in which the output of each individual is compared to that of his peers. This compensation scheme is similar to the one used in tournaments (Green and Stokey, Reference Green and Stokey1983; Knoeber, Reference Knoeber1989; Lazear and Rosen, Reference Lazear and Rosen1981). In our case, the cooperative promotes competition between growers, first, by promising the possibility for a grower to be “promoted” to a higher-quality contract and, second, by providing a price differential within each quality contract, an additional incentive for growers to seek higher prices. At the same time, the cooperative faces the adverse selection problem by organizing the competition between different homogeneous groups. For this, the cooperative proposes a menu of contracts and monitors the production process.
Our empirical results from a database of 1,219 contracts confirm that the tournament mechanism aims to (1) provide some incentives in the event of a common shock that may affect all the growers; and (2) reduce the heterogeneity among the growers participating in the same contest depending on the contract chosen.
This paper is organized as follows. Section II provides a short overview of the theoretical approaches when agency problems are at stake in the production of quality grapes and tournament theory. Section III describes the wine cooperative's contract stages and the organization of our database. Section IV introduces the empirical model. Results are presented in Section V. Section VI offers some concluding remarks.
II. Quality Incentives and Contracts
Although explicit contracts in wine grape production have been used largely between independent growers and wineries (Fares, Reference Fares2009; Fraser, Reference Fraser2005; Gaucher et al., Reference Gaucher, Soler and Tanguy2002; Goodhue et al., Reference Goodhue, Helen, Lee and Sumner2003), in this paper we focus on cooperative organizations, in which the growers are both suppliers of the grapes and members of the cooperative. The cooperative faces a classic agency relationship with its growers (Alchian and Demsetz, Reference Alchian and Demsetz1972; Jensen and Meckling, Reference Jensen and Meckling1976; Pennerstorfer and Weiss, Reference Pennerstorfer and Weiss2013). In the case of cooperative-grower, or principal-agent, one party (grower) has an informational advantage over another cooperative that can be exploited to the benefit of the advantaged party (Jensen and Meckling, Reference Jensen and Meckling1976). The first kind of asymmetric information is the adverse selection problem. This means that a grower is supposed to know his characteristics, skills, and competencies (his type), but the cooperative cannot observe these characteristics without a cost. To overcome the problem of adverse selection, the cooperative offers a “menu of contracts.” If the menu is well designed, the grower can reveal his type by choosing the contract that maximizes his utility. The second kind of problem is that of moral hazard , in which the cooperative cannot monitor the efforts toward achieving grape quality undertaken by the grower after the contract is signed. Therefore, the contract offered by the cooperative also has to give incentives toward effort. An optimal compensation scheme based on the observable output is defined. When the grower is risk averse, this optimal compensation scheme faces the “incentive versus insurance” trade-off (Sappington, Reference Sappington1991). In this case, a piece-rate mechanism (with a fixed rate of base pay) can be optimal.
In practice, the vineyard contract relationship departs from this simple model because the output (quality) is costly to monitor (Gaucher et al., Reference Gaucher, Soler and Tanguy2002). First, there is no easy way to control (thus, enforce) the grower's vineyard practices. Even if the cooperative's technicians visit the vineyard during the year and provide extension services, the actual work in the vineyard is not observed. Second, there is no easy ex post evaluation of the output (grape quality), although there are tests based on pre-established parameters, such as sugar content (Alston et al., Reference Alston, Fuller, Lapsley and Soleas2011) or the sanitary and physiochemical proprieties of grapes that could provide some indication (Montaigne et al., Reference Montaigne, Rousset, Traversac, Coudrec, Hannin, d'Hauteville and Montaigne2007). However, they do not directly reflect the grower's level of effort. Finally, there is no “mechanical” relationship between the application of specific vineyard practices and the qualitative end result for grapes. Elements related to the pedoclimatic environment of parcels of land or yearly meteorological conditions could influence output (Ashenfelter, Reference Ashenfelter2008; Ashenfelter and Storchmann, Reference Ashenfelter and Storchmann2010).
In cases where monitoring is either unreliable or costly, tournaments can offer a desirable incentive scheme (Lazear and Rosen, Reference Lazear and Rosen1981). This compensation system remunerates individual performance not according to the absolute value of their output but on the position their output rank compared to that of peers. In what follows, we characterize the efficient solution of a compensation scheme piece rate (Section II.A), before analyzing the specificities of a differentiated compensation scheme, in which agents can be heterogeneous (Section II.B).
A. Agency Problems and Piece-Rate Compensation Scheme
The timing of the relationship between the cooperative and the grower is defined as follows. In the first period, the cooperative offers a menu of contracts and the grower chooses the contract that maximizes his expected utility. In the second period, given the contract chosen, grower i undertakes an investment or effort level e i that increases his performance—that is, the quality q i of grapes produced (output). Making an investment or exerting effort is costly to the grower: C(e i) = θ iei 2/2 denotes the cost of investment or effort, with θ i the grower's ability or productive parameter. Quality may also be affected by a common shock (η) and idiosyncratic shock (ε i). The shocks follow an independent and identically distributed normal distribution with a mean of zero, and the common shock has a variance σ η2 while the idiosyncratic shock has a variance σ ε2. The quality production function can be written as follows
$$q_i = e_i + \eta + \varepsilon _i $$The cooperative cannot observe the grower's effort and thus cannot verify the extent to which the quality produced (or some of its proxies) is due to effort or to shocks, either common or idiosyncratic.
If a piece-rate contract can be used to remunerate growers (Gibbons, Reference Gibbons1987; Lazear and Rosen, Reference Lazear and Rosen1981), the growers are paid based on output (quality). Because output q i is dependent on effort e i, a piece-rate contract should encourage greater effort and penalties could be applied if the observed output is insufficient. Following Lazear and Rosen (Reference Lazear and Rosen1981), we suppose for ease of exposition that growers are risk neutral, and free entry in a competitive output market sets the value of the product at V per unit. Let r i be the piece rate, then grower net income is r iqi – C(e i). A risk-neutral grower chooses e i to maximize his utility
$$E[r_i q_i - c(e_i )] = [r_i (e_i ) - c(e_i )]$$The first-order condition of this program is r i = e i/θ i, that is, the piece rate is equal to the marginal cost of effort. Because the cooperative is also supposed to be risk neutral, its expected profit is E[Vq i − riqi] = (V − r i)e i. Because the free–entry assumption implies r i = V, substituting in Equation (2) and obtaining the derivative yields
$$C^{{\prime}} (e_i ) = V$$That is, the marginal cost of effort equals its social return. The optimal effort level is then e i* = θ iV. This result suggests that a piece-rate program is an efficient compensation scheme. However, since it is costly and difficult for the cooperative to directly measure the absolute level of each grower's output,Footnote 2 it can be more efficient to observe the relative position of each grower by ranking the different output levels (Prendergast, Reference Prendergast1999).
B. Tournaments and Relative Compensation Schemes
The wine cooperative's differentiated payment system studied in this paper is like a tournament. Tournaments remunerate individual performance, not on their absolute value but on the position of their output ranking compared to that of their peers. The literature on tournaments (Green and Stokey, Reference Green and Stokey1983; Knoeber, Reference Knoeber1989; Lazear and Rosen, Reference Lazear and Rosen1981) shows that the cases in which the principal promotes competition between agents because monitoring of output itself is either unreliable or costly. In our case, the cooperative creates incentives to work harder, first, by promising a grower the possibility to be “promoted” to a higher-quality contract and, second, by providing a price differential within each quality contract that provides growers with an additional incentive to work harder for a better quality.
Promotions are used to sort workers based on their talents and reward them accordingly (Rosen, Reference Rosen1982). In a tournament system, the winning prize is a promotion, which should be an incentive for the agents to improve their effort level (Prendergast, Reference Prendergast1999). Lazear and Rosen (Reference Lazear and Rosen1981) were the first to study this type of payment system. In the presence of high monitoring costs, with regard to effort and final output, this type of payment system is preferred because it would be less expensive to observe the relative position of agents than to measure their individual production levels. Green and Stokey (Reference Green and Stokey1983) and Nalebuff and Stiglitz (Reference Nalebuff and Stiglitz1983) deepened the analysis by observing that tournaments are particularly preferred when the agents are confronted with a common shock (random variable that might represent economic conditions that affect all the agents). As stated in Tsoulouhas (Reference Tsoulouhas2010) and Tsoulouhas and Marinakis (Reference Tsoulouhas, Knoeber and Agrawal2007), when agent production activities are subject to a common shock, individual performance is not a sufficient metric for individual effort. The performance levels obtained by the rest of the agents convey an informative signal about the common shock and, thus, the effort choice of any given agent. Therefore, tournaments constitute a position closer to the first-best solution because the principal uses the available information more efficiently. By removing common uncertainty from the responsibility of agents and by charging a premium for this insurance, the principal increases his profit without hurting the agents.
To give an idea of the main result of the tournament mechanism, we follow the simple model of Lazear and Rosen (Reference Lazear and Rosen1981) in which the two agents (growers) are rewarded for their performance with one of two prizes, t 1 or t 2, where t 1 > t 2. The higher prize t 1 goes to the grower with the better performance, while the lower prize goes to the grower with the poorer performance. The probability of that grower i will win the higher prize depends positively upon his own efforts (e i). If P is the probability of winning the tournament,Footnote 3 the expected payoff to grower i is
$$P(e_i )[t_i - C(e_{_i})] + (1 - P(e_i ))[t_2 - C(e_i )] = P(e_i )(t_1 - t_2 ) + t_2 - C(e_i )$$Assuming risk neutrality and holding the opponent's action constant, grower i will choose e i to maximize Equation (4). By deriving the grower payoff with respect to e i, the first-order condition is P′(e i)(t 1 − t 2) = C′(e i). In a symmetric Nash equilibrium, where grower j reasons similarly to grower i, the effort level implemented is
$$e = (t_1 \!\!-\! t_2 )\left[ {\displaystyle{{\,p{\rm ^{\prime}}} \over \theta}}\right]$$This implies that effort depends positively upon: (1) the marginal effect of effort on the probability of winning (P′); and (2) the prize differential (t 1 – t 2). This latter implies that effort is unaffected by changes in the absolute level of prizes that leave this differential constant.Footnote 4 This price differential mechanism of the tournament provides protection to growers against common shocks. Indeed, if these shocks are systematically applied, universally boosting or decreasing production signals does not change the rankings between growers and thus their expected payoff. This tournament mechanism can achieve an efficient solution (e = e*) by choosing a prize differential such that
$$(t_1 - t_2 ) = \left[ {\displaystyle{v \over {\,p{\rm ^{\prime}}}}} \right]$$This result shows that the tournament mechanism can also achieve an efficient outcome when the growers are risk neutral. When they are risk averse, this efficiency result cannot be obtained with piece rates and tournaments due to the trade-off between incentives and insurance. When there are no common shocks and only idiosyncratic shocks, the piece rates do provide an improvement over tournaments. In contrast, relative pay in tournaments can yield greater effort than pieces rates when a common shock is sufficiently large (Tsoulouhas and Marinakis, Reference Tsoulouhas and Marinakis2007).
The empirical literature on tournaments that studied the North American broiler production industry confirms the theoretical predictions (Knoeber, Reference Knoeber1989; Knoeber and Thurman, Reference Knoeber and Thurman1994). It finds that: (1) the extent of this payment depends on the grower's relative performance relative to others (measured by the grower's settlement cost compared to an average); (2) changes in the level of prizes that do not change the prize differential motivate no change in chicken production (and, thus, farmer effort). Ehrenberg and Bognanno (Reference Ehrenberg and Bognanno1990) also provide interesting empirical confirmation of tournament's effect on the effort of competitors through their analysis of professional golfers. Main et al. (Reference Main, O'Reilly and Wade1993) show that a promotion from corporate vice-president to president generates a pay increase, which is positively correlated with the number of vice-presidents competing for the position. That is, executive pay is the prize of a tournament rather than compensation for value added. Eriksson (Reference Eriksson1999) presents similar findings by regressing firm performance (log (profit/sales)) by pay differentials (the gap between CEO and VP pay), controlling for industry and firm size. His pay differential yields a statistically significant coefficient, which offers some evidence of the main prediction of the theory.
C. Organizing the Contest Between Heterogeneous Growers
In the previous simple models, the growers were assumed to be homogeneous. In practice, growers can be heterogeneous, and thus each one will respond differently in the tournament to the prize differential or promotion. That is, growers with a high cost of effort may choose zero effort because of their competitive disadvantage, or take excessive risks in the hope of leaping ahead of the frontrunner. Therefore, when agents are heterogeneous relative performance evaluation via tournaments exposes agents to uncertainty about the average agent ability. The rationale is that, unlike piece rates, tournaments filter common uncertainty from the responsibility of agents, who pay a premium for this insurance. Tournaments expose agents to the idiosyncratic shocks of other agents, so tournaments become less desirable when the variance of the distribution of ability types (heterogeneity) is large (Tsoulouhas and Marinakis, Reference Tsoulouhas and Marinakis2007; Tsoulouhas and Vukina, Reference Tsoulouhas and Vukina2001).
This problem of adverse selection in tournaments has been documented by Lazear and Rosen (Reference Lazear and Rosen1981), who show that, without a specific mechanism, agents would not self-select since it is more interesting for all agents to be in the high-ability group. Consider the simple case of two different types of growers, who know their type (ability) although the cooperative does not. There are two leagues: league θ H, which yields the most efficient outcome given the high-ability people, and league θ L, which yields the highest outcome given low-ability growers. The problem is that league θ H is preferred since it generates a higher payoff. This leads the lower type θ L, to contaminate league θ H, which results in a mixed “league” bag of growers of different abilities. That is, growers have no incentive to sort themselves in the two leagues according to their abilities. As shown above, this “league composition risk” (Tsoulouhas and Vukina, Reference Tsoulouhas and Vukina2001) can lead to inefficiency since growers will provide less effort and or take more risks. Bull et al. (Reference Bull, Schotter and Weigelt1987) found that there is a large variation in tournament settings with respect to effort levels, and despite introducing more information, such as the opponent's effort levels, there is still a great deal of variation. Starting from this early experimental tournament paper, several lab experimental studies examine the relationship between exertion of effort and heterogeneity. For example, Gurtler and Harbring (Reference Gurtler and Harbring2010), Tsoulouhas et al. (Reference Tsoulouhas, Knoeber and Agrawal2007), and Vandegrift and Yavas (Reference Vandegrift and Yavas2010) find support for the prediction that equilibrium effort is lower in asymmetric tournaments because reduced effort does not change a player's relative position. However, Eriksson et al. (Reference Eriksson, Teyssier and Villeval2009) show that previous experimental evidence regarding the variability of effort in tournaments is misleading because the experiments have not accounted for sorting—that is, agents typically choose to participate in a tournament. So, they used a setup similar to that of Bull et al. (Reference Bull, Schotter and Weigelt1987), except that the participants had a choice when they participate between piece rates and relative pay. They find that those who choose the tournament exert, on average, more effort. After conducting the experiments, they also distributed a questionnaire with several lottery decisions to elicit the risk aversion of each participant. They found that risk-adverse agents tend to choose the piece-rate scheme. This sorting leads to two homogeneous groups, which reduces the between-subject variance dramatically in the tournaments.
Another solution to sort between heterogeneous agents is to offer a menu of contracts. Following Bhattacharya and Guasch (Reference Bhattacharya and Guasch1988), Riis (Reference Riis2010) shows that when agents are heterogeneous ex ante and the optimal discriminatory prize premium is nonmonotonic in ability, efficiency can be restored if agents choose from a menu. Wang and Jaenicke (Reference Wang and Jaenicke2005) show that a menu of two contracts can also simultaneously shift surplus from low- to high-ability growers. Further, a “cream-skimming” contract that pools only growers above a certain ability level can, under certain circumstances, increase the expected utility of participating growers and reduce the expected profit of the principal (processor). In contrast, Tsoulouhas (Reference Tsoulouhas2013) shows that no menu of contract is needed ex ante since the ex post tournament mechanism can sort between growers if they are known not to be very heterogeneous.
In our case, the wine-making cooperative tries to screen the growers by their ability and organize contest in subgroups in which growers can be the least heterogeneous possible. This is done in two steps. First, ex ante growers are screened and reveal their type by choosing the contract that maximizes their expected utility in the menu of contracts offered by the cooperative. Second, before the harvest and the contest, the cooperative can downgrade growers and their parcels of land that are unable to achieve a minimum level of quality within their group. This helps to reduce the variance of the outcome within the group after the harvest . After this problem of heterogeneity is solved, the tournament mechanism is probably the best alternative when a common shock is sufficiently large. Indeed, it eliminates the variation in revenue caused by factors common to all growers, either variations of measurement or variations related to the environment. This is particularly true in the case of cooperatives, which are delimited to a geographic area, making it more likely that growers will be affected by climatic hazards. Tournaments should also reduce the cost related to measuring performance and maintain incentives for maximum effort, while removing common shocks for all participants.Footnote 5
III. Data and Methods: The Case of the Saint Mont Vineyard
The Saint Mont vineyard,Footnote 6 as well as the cooperative studied here,Footnote 7 has experienced a transformation similar to that of other cooperatives in southwestern France, from mass wine production to more quality-focused production. This shift has translated into a payment system that guides producers toward medium- and long-term planning and strongly encourages them to exert efforts toward improving the quality of grapes (Chiffoleau et al., Reference Chiffoleau and Karantinis2007). In this section, we present the different steps in the contractual process from the moment a grower chooses a contract to the arrival of grapes at the cooperative after harvest. This information is registered in the cooperative's traceability database, which allows for better management and monitoring of the grape production. We use this database to verify (1) the efficiency of the cooperative's tournament compensation system—that is, whether a promotion has a positive effect on effort and thus on final qualitative output; (2) the effect of the monitoring mechanism and menu of contracts on the organization of the efficiency contest.
A. The Timing of Contracts
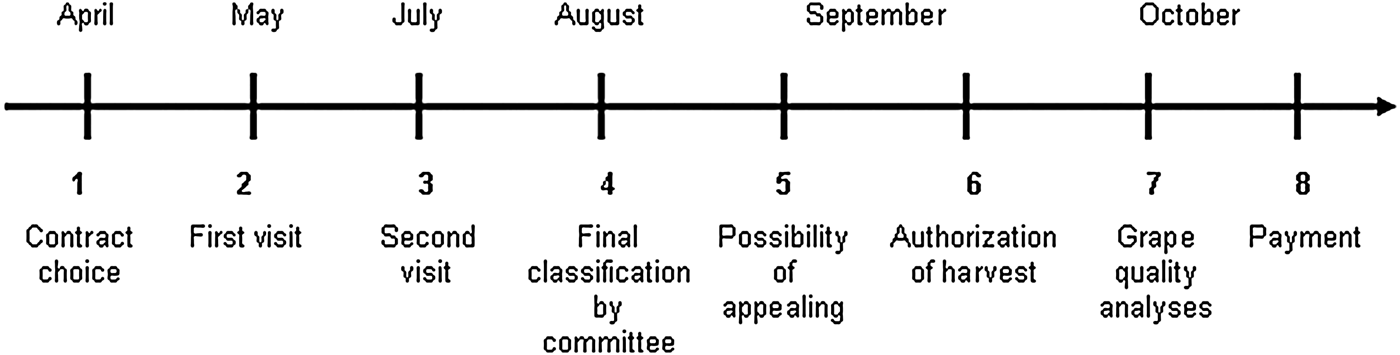
The contractual process begins in April of each year (see Figure 1), when growers commit one or more parcels of land to a quality contract until September, when the grapes are harvested.Footnote 8
Figure 1 Steps in the Wine-Grape Production Contract
In step 1, the cooperative offers a menu of contracts A, B, C. The quality requirements and the cost of each contract are such that the grower's profit is represented by 
$\prod^A \geq \prod^B \geq \prod^C $. These contracts have certain requirements that must be followed. At this step, the cooperative takes some preventive measures to screen heterogeneous growers and parcels of land. For example, to commit a plot of land to contract A, the parcels of land must belong to a domaine or château (property), or they must have been committed to a contract A for the previous three years.
Steps 2 and 3 consist of visits from technicians to evaluate, validate, and guide the work of the grower. These visits are part of the “monitoring” process, which aims to avoid moral hazards or hidden actions, such as noncompliance with the contract.Footnote 9 In doing so, the cooperative can screen growers again. Indeed, through monitoring the cooperative aims to ensure that there are homogeneous groups before the harvest and the contest.
If everything happens according to plan, the grower who exerted all the necessary effort and complied with the contract should produce a better quality grape than the one who did not. However, land characteristics (e.g., location, pedoclimatic conditions) may not be consistent with the desired quality. Consequently, if a plot committed to a certain contract did not achieve its potential—in our example, land that is not suitable for contract A—all efforts made by the grower would end up being pointless because he could be penalized after the qualitative analyses. On the contrary, if the land has greater potential, with only a little effort from the grower the results could indicate a high-quality wine.Footnote 10
Nonetheless, the risk, especially regarding the land's potential, would raise one problem regarding tournaments. If agents know—or believe they know—about the high potential of their land, they could make a little effort and, with high-quality production, place highly in their tournaments. On the contrary, those who believe that they own “low-potential land” will make little effort to remedy the situation because they will conclude that their land is going to be downgraded anyway.
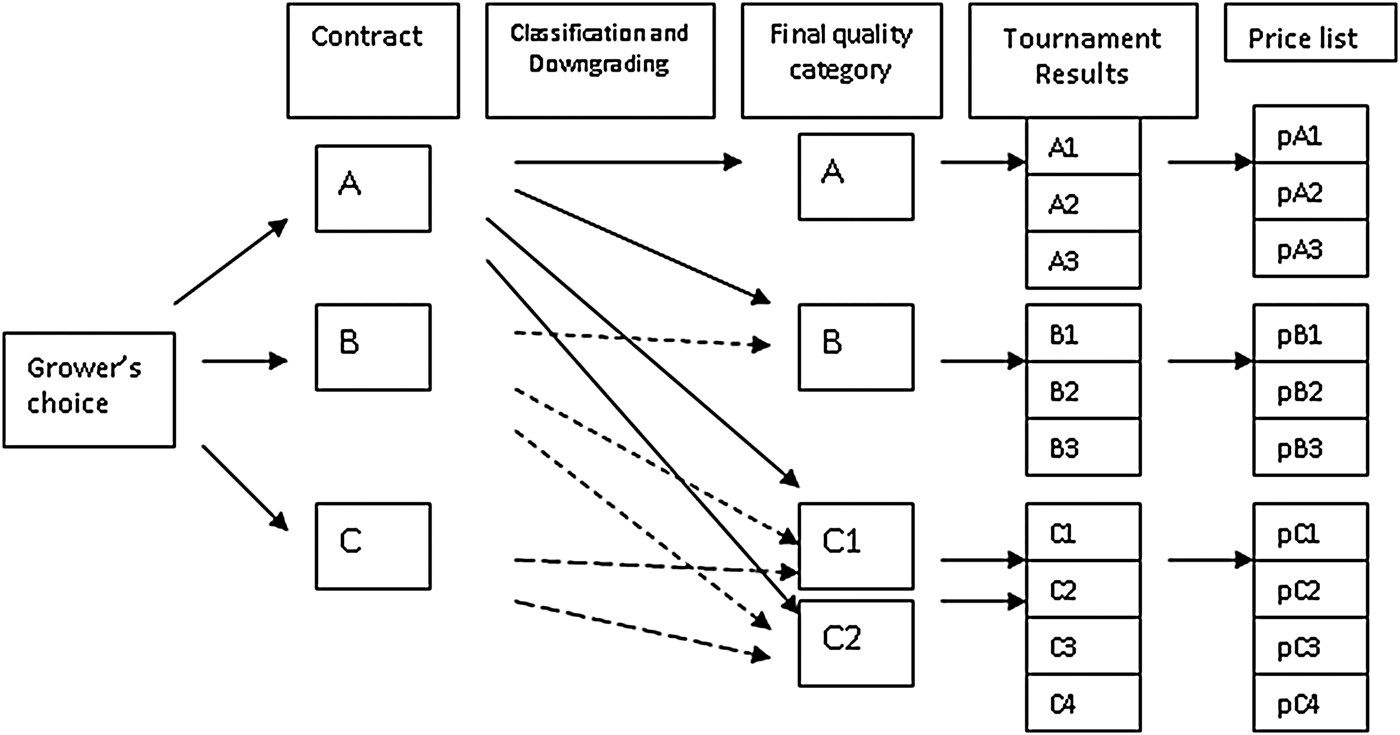
In step 4, a committee composed of growers and technicians proceeds to classify—and eventually downgrade—the various parcels of land (see Figure 2). This classification helps the cooperative to determine the quality of grapes that will arrive at their facilities after harvest and foresee the type of remuneration that the grower will receive. For example, a parcel of land under contract A (at this step) is downgraded to B or C because the requirements of the contract were not met.Footnote 11
Figure 2 Classifying and Downgrading Wine-Grape Parcels of Land
This is a crucial moment because the fulfillment of the contract is verified. For instance, if the grower commits a plot of land to contract A, he/she expects to be paid according to the A price scale (pA1 to pA3; see Figure 2), depending on the results of the contribution analysis. However, if they agreed to contract A but find themselves with several parcels of land downgraded to C1 or C2, their compensation will be heavily penalized because they will have the right to expect only prices pC1 to pC3.
There is an additional feature in the payment system (a contractual provision). The compensation for each parcel of land is a function of the price and the yield of all plots of land within the contract, but there are ceilings in terms of yield (as a measure of quality).Footnote 12 In the case of contract A (tournament A), a grower's compensation (prices pA1 to pA3) will be paid only up to a yield of 45 hectoliters/ha. After breaching that ceiling, yield will be paid at a price p c (complement price), which is close to 1/5 of pA1.Footnote 13
The penalty for surpassing the fixed yield ceiling, and incurring a loss, is another incentive for additional effort. The contract, especially high-quality contracts (A and B), stipulates a mode of cultivation that requires strong management of the expected yield. For instance, the process of disbudding and pruning the vine is very costly in terms of working hours and differs from one contract to another.Footnote 14 This means that growers committed to contract A will not only play in tournament A, but they must also be mindful of their yield and make the maximum effort to manage the yield below the respective ceilings. In any case, growers can appeal the step 4 decision (step 5).
In step 6, samples are taken, and three ripeness tests are carried out, in order for the cooperative's technicians to allow the grapes to be harvested. This shows the authority mechanism used by the cooperative, which may have different interests from the grower, who, for instance, might prefer to harvest at another time. In step 7, samples of each contribution are analyzed, and a corresponding score is calculated in accordance with its group average quality. In step 8, the remuneration is made according to the final classification (result of the tournament) and the ceiling criteria in terms of the yield set in the price scale.
B. The Database
The main database, referred to as the “Geowine StMont” database, contains two types of information from different sources. The first type comes from the wine cooperative's traceability database.Footnote 15 It contains information on each grower's individual contribution of grapes since 2000. It provides detailed information on each grape contribution (e.g., yield, grape variety), characteristics of each parcel of land , the type of contract used, the parcel of land's classification (from step 4), and the final compensation category. These data are further complemented by information provided by the cooperative, such as the price paid for each compensation category and individual information about growers, such as age, gender, and total arable land surface. The second type comes from the work of researchers at the École d'Ingénieurs de Purpan (Gay, Reference Gay2010) and includes geographic variables such as soil, altitude, slope, and orientation exposition.Footnote 16
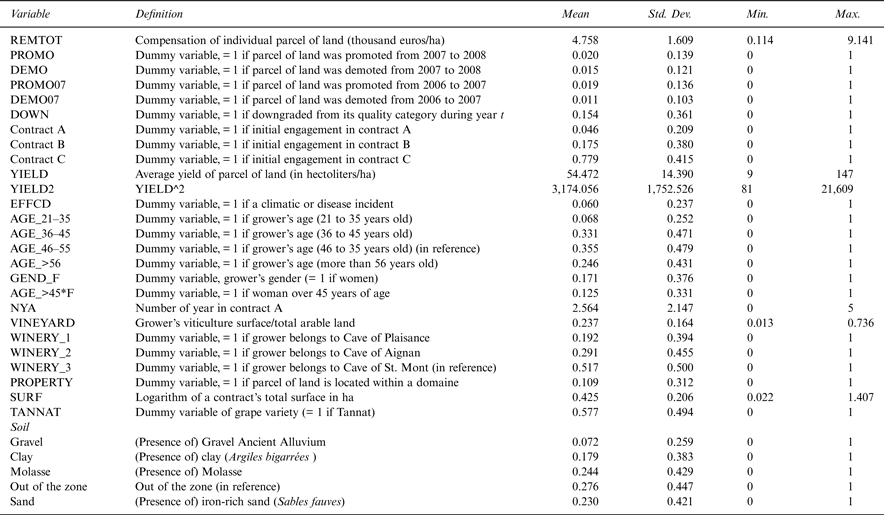
The test is carried out on a sample of 1,219 parcels of land belonging to 120 growers. Summary statistics and definition of variables are presented in Table 1.
Table 1 Summary Statistics and Description of Variables (N = 1,219)
Source: Geowine St. Mont database (Gay, Reference Gay2010), 2008 harvest.
(1) Variables
The analysis was performed using data from the 2008 harvest. The main reason for this choice is that, after 2007, contracts became more differentiated in terms of quality requirements, but in 2007, many vines were damaged by environmental factors (hailstorms). In addition, we take into account the grape production only for red wines because they have three contract types (instead of two for whites) and they represent almost 80% of all grape production in the Saint Mont AOP.
Our dependent variable, REMTOT, is payment for each parcel of land's provision of grapes (in thousands of euros for each hectoliter/hectare). This payment does not include the costs subtracted by the cooperative for actual wine production but is an indication of the quality of grapes because the price paid for each parcel of land depends upon the quality category to which it was assigned after the downgrading process (step 4) and upon the price category after analysis (step 7). The type of contract initially chosen by the grower can be A (contract A), B (contract B), or C (contract C). The quality requirements are higher in contract A than contract B, and higher than contract C.
The first group of independent variables concerns prediction of the tournament model and the contractual provisions. The potential of promotion to a higher-quality category should have a positive effect on effort exerted toward quality. The probability of promotion increases with the number of promotions offered and decreases with the number of contestants (Heutel, Reference Heutel2009). We consider the dummy variable PROMO, which equals 1 if the parcel of land has been promoted from the previous year (t − 1 to t). It could indicate that the grower wants to prove that he/she deserved the promotion. Inversely, we consider a dummy variable DEMO that tests whether the parcel of land has been demoted in category from the previous year.
Furthermore, we consider a variable to capture the monitoring activity of the cooperative. Tournament theory indicates that the higher the monitoring efficiency of the principal, the greater the effort from the agent should be (Prendergast, Reference Prendergast1999). The variable DOWN indicates the parcel of land has been downgraded at step 4 (Figure 1) during the season (in year t). As previously mentioned, at this stage, a committee of growers and technicians (from the cooperative) evaluates the grower's work and determine whether the contract has been followed. If it has, the parcel of land remains in the same quality category; if not, it is downgraded to a lower-quality category. In addition, this variable takes into account the downgrading of a parcel of land only where the grower's effort did not meet the contract specifications (moral hazard). It does not take into consideration those that are downgraded due to a climatic event or a vine disease.Footnote 17 The variable EFFCD indicates whether a parcel of land suffered from a climatic or a disease event.
The variables YIELD and YIELD2 consider the average yield of each individual parcel of land . The yield is measured in hectoliters/hectare and is relevant because, in viticulture, higher quality is associated with lower yields. The square of the yield, YIELD2, will allow us to observe whether, above a certain ceiling, an additional unit of yield would have a negative effect on quality. The control of yields is probably the most important provision of quality contracts in viticulture. Concerning the grower, we take into consideration the grower's age (AGE), gender (GEND), and number of years of experience in contract A (NYA). We also consider the percentage of vines over total arable land (VINEYARD), which signifies the grower's degree of specialization in viticulture.
Finally, we consider variables related to the fixed characteristics of the land plot. The variable PROPERTY indicates that the land belongs to a château or a domaine. SURF refers to the parcel of land's total surface (in hectares). TANNAT indicates whether or not the variety of grape is Tannat.Footnote 18 The variable SOIL, which has five classes—that is, four classes of soil representing 74.3% of all parcels of land and one class, considered “outside the zone,” representing the remaining land. The four types of soil are mainly those with high levels of gravel, clay, molasse, and iron-rich sand.
IV. The Empirical Model
In this section, we outline the empirical analysis. The two main objectives are, first, to test the efficiency of the cooperative's tournament compensation scheme system, in other words, to verify that a promotion has a positive effect on effort (and thus on final qualitative output); and, second, to test the efficiency of the screening/selection mechanism to reduce heterogeneity among growers and land.
We hypothesize that the qualitative performance of land, thus the grower's effort, is dependent of the initial choice of contract. The choice of a specific contract (step 1 of the process) by growers depends on the characteristics of the land , the grower's experience, and performance in previous years. Thus, we test for the existence of a selection bias since the effort level of growers is likely to depend on the type of contract chosen. The choice made by the grower seems to be sequential, because he/she first chooses a contract for its parcel of land and then chooses the level of effort, which to a certain extent defines the total compensation associated with the land.
The first part is a binary outcome equation that models the probability of accepting a contract i, i = A, B, C. The probability of accepting a contract i can be described as follows
$$S_i ^{\ast} = x_1 ^{\rm ^{\prime}} \beta _1 + u_1 $$where
$${S_i} = \left\{ {\matrix{{1\quad if} & {{S_i}^{\ast} \gt 0}\hfill\cr{0\quad if} & {otherwise} } } \right.$$The binary decision S i to choose a contract i is modeled as the outcome of an unobserved latent variable S i*, and we observe that the contract is adopted (S i = 1) when S t* > 0. It is assumed that S i* is a linear function of the exogenous covariates x 1 and a random component u 1.
The second part uses a linear regression to model the total compensation obtained by the land, given the decision on contract adoption. This can be expressed as
$$\pi = x_2 ^{\rm ^{\prime}} \beta _2 + u_2 $$where π is the total compensation for the parcel of land, x 2 is a vector of exogenous explanatory variables, and u 2 is a random component. Because the error terms u 1 and u 2 are supposed to be uncorrelated, the two parts are assumed to be independent and are usually estimated separately. However, this can be viewed as a limitation of the model. Indeed, if the land generating a positive compensation is not randomly selected from the population, after controlling for regressors, then the results of the second-stage regression suffer from selection bias.
In what follows, we use the Heckman (Reference Heckman1979) selection model, in which the possibility of such selection bias is considered by allowing for possible dependence in the two parts of the model. That is, let the latent variable π *denote the total compensation obtained by the land, in which the compensation is observed when π * > 0. In contrast to the hurdle model, π * is observed only when S t*>0. In such a model, the errors u 1 and u 2 might be correlated and have a bivariate normal distribution with zero means and covariance matrix:
$$\left[ { {\matrix{ {\rm \sigma}& \rho \cr \rho & 1 }} } \right]$$Because the error u 1 in Equation (7) is believed to be correlated with the outcome variable, we need to correct for this bias by finding variables that are associated with holding a contract i, but are not associated with the outcomes of contract j.
The two-step method of estimation is based on the conditional expectation
$$\eqalign{ E(\pi |x,S_i ^{\ast} \gt 0) &= x_2 ^{\prime} \beta _2 + E(u_2 |S_i ^{\ast} \gt 0) \cr & = x_2 ^{\prime} \beta _2 + \rho \lambda (x_1 ^{\prime} \beta _1 )} $$ The method is as follows: (1) first estimate the probit equation by maximum likelihood to obtain estimates of β 1. For each observation in the selected sample, compute the inverse of the Mill's ratio or the nonselection hazards 
$\hat \lambda = \phi (x_1^{\prime} \hat \beta_1)/ \Phi(x_1^{\prime} \beta_1)$, where φ and Φ are the standard normal density and distribution functions respectively; (2) estimate β 2 and β λ = ρσ by least squares regression of π on x and 
$\hat \lambda$. For more robust identification, we also impose exclusion restrictions, which require that the selection equation have at least one exogenous variable excluded from the outcome equation. In our estimation, we use the variable PROPERTY because it has some effect on the selection and does not directly affect the compensation. With this exclusion restriction, we have x 1 ≠ x 2, which may reduce the collinearity problem between the variable 
$\lambda (x_1 ^{\rm ^{\prime}} \hat \beta _1 )$ with other regressors (x 2) in the compensation equation. But because this problem in the two-step method of estimation generates higher standard errors than those of the maximum likelihood estimation procedure, we use also use this procedure.
V. Results and Interpretation
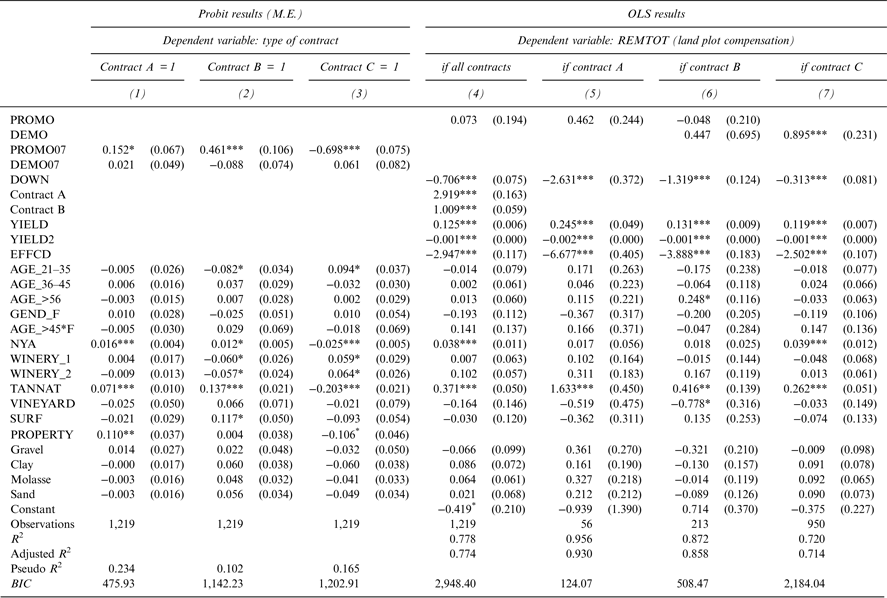
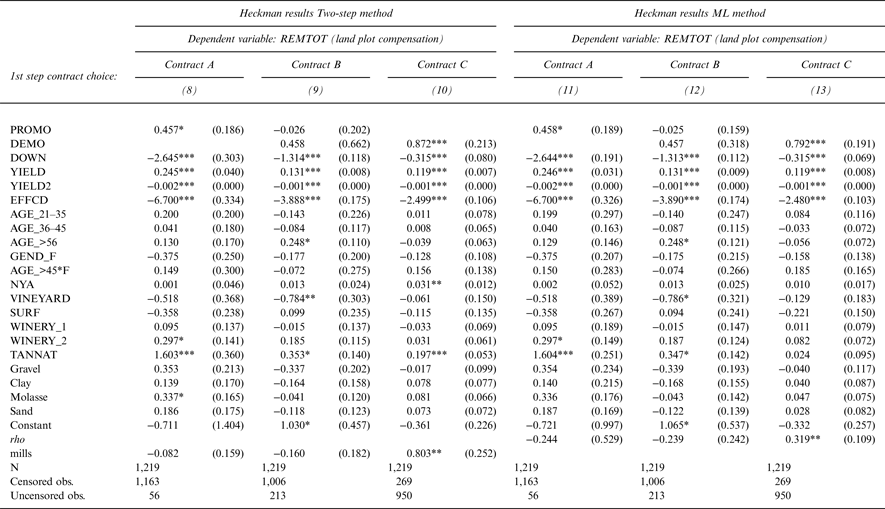
We present marginal effects of probit and OLS regressions for each contract in Table 2 and results from the second step of the Heckman model in Table 3.
Table 2 Determinants of Contract Choice and Compensation
Robust standard errors in parentheses. M.E. = Marginal effects.
* p < 0.05, ** p < 0.01, ***p < 0.001
Table 3 Efficiency of Compensation System with Sample Selection
Robust standard errors in parentheses. * p < 0.05, ** p < 0.01, *** p < 0.001.
In the probit results, we obtain the factors explaining the choice of a contract (Equation (7)). In the second step of the Heckman model, the factors explaining the qualitative performance of parcels of land (Equation (8)). The first group of variables in our regressions corresponds to tournament mechanisms and contract provisions, then those related to the harvest, growers' characteristics, and finally to land characteristics.
A. The Determinants of Contract Choice
The results from the probit model (Table 2) show the factors associated with the choice of each of the quality contracts. For contract A, model 1, our results show that four variables can explain this choice. First, the fact that the land has been promoted from year t − 2 and the number of years that the grower has had a contract A (NYA), but also the fact that the land belongs to a property and that the grape variety is Tannat. The variable NYA reflects a reputation effect, or experience, but related to only such high-quality contracts. Generic experience, usually captured with an age variable, is not significant, at least for high-quality contracts.
The fact that high-quality contracts are associated with a property is not surprising. First, it is a requirement for obtaining a high-quality contract. Second, it means that grapes from only one property will be mixed together in making the wine and bottled at the property. Finally, the use of Tannat is also expected because it is the main variety of the Saint Mont AOP and the one preferred in the production for high-quality wines.
For contract B, we found a negative and significant effect of age (between ages 21 and 35) and the number of years with a contract A. It seems that in this case young growers are less likely to have contract B; rather, they start with the less demanding contract C. It also seems that the more years of experience a grower has with contract A, the more likely it is that the grower will continue to choose A or B contracts. In addition, the wineries to which the growers belong play a significant role in the type of contract they choose. Belonging to the Cave de Saint Mont winery determines in part the choice of contract B, while those who belong to the Aignan and Plaissance winery are more likely to choose contracts C. With regard to the characteristics of land, we found several factors that explain the contract choice: the size of the parcel of land (SURF) and the grape variety (TANNAT).
We found the opposite results for the selection of contract C, a negative effect for promotion, age (young growers), NYA (number of years in contract A), grape variety (TANNAT), and PROPERTY. It seems that the cooperative takes some measures, as defined in the contracts’ specifications (step 1, Figure 1), to screen growers in their contract choices.
B. The Efficiency of the Wine-Grape Tournament
In this section, we discuss the factors that explain the qualitative output of grapes, measured by the dependent variable REMTOT—that is, the compensation paid to a grower for an individual parcel of land. In Table 2 we show the results of ordinary least squares (OLS) regressions for all types of contracts. However, we assume that the qualitative performance of parcels of land, thus grower's effort, is dependent of their initial choice of contract. Therefore, we discuss here the results of the second step of the Heckman model, in which we account for this selection bias (Table 3).
Using STATA's two-step method for the Heckman model, we automatically obtain the inverse Mills ratio. Using the maximum likelihood method, we obtain a χ2 test that verifies that ρ is significantly different from zero, rejecting the null hypothesis that the equations are not independent from each other. The results of the maximum likelihood, as well as the two-step, Heckman model shows, first, that the correlation between the two equations is significant only for the choice of contract C. It is not the case, however, for contracts A and B.
The compensation system is organized such that a grower's performance can be taken into account by the cooperative in order to “promote” the parcel of land to a higher-quality category or to “demote” it to a lower-quality category. In models 8 and 11, we observe that after a parcel of land has been promoted (PROMO) to this category (from the previous year), the effect on its compensation (on its quality result) is positive and significant. For contract B, being promoted to this category does not have a significant influence on final output. However, the fact that the parcel of land was demoted (DEMO), meaning that the previous year it had a higher-quality contract, has a positive and significant effect on contract C. This could be interpreted as the fact that growers will exercise greater effort in order to be promoted again, or “return,” to the higher-quality contract, which they had earlier.
The monitoring variable, DOWN, has a negative and significant effect on all three contracts. This means that the cooperative was able to identify and downgrade parcels of land that are not complying with the contract specifications (step 4, Figure 1). Choosing a contract A and then being downgraded at this stage will translate into a loss of about 2,644 euros for the grower. This variable does not include the downgrading of land affected by a climatic or a disease event; such effects are captured by the variable EFFCD, which has a logical negative effect on the final output. A similar effect is found in models 9–11 and 12–13—that is, in the choice of contracts B and C.
As seen in section III.A, the ceiling, in terms of yield, imposed on the cooperative's specifications (an additional contractual provision) is both an incentive for maximum effort and a penalty. The quality of grapes is related to a controlled (and fixed) yield ceiling. But, because the price paid being a function of yield, we have a positive and significant effect for the variable YIELD. However, this relationship becomes negative (variable YIELD2) after a certain ceiling is exceeded.
The results of models 8 through 13 indicate that the yield rule has the same result, regardless of the type of contract chosen. We show this relationship between qualitative output and yield in Figure 3. We notice a slight difference between high-quality contract A and the rest. The high-quality contract A is associated with low yields and high compensation. However, for contracts B and C, the penalties for exceeding the yield ceilings are less dramatic than they are for contract A. The behavior, at least in terms of respecting yield, is similar whether the initial contract is B or C.
Figure 3 Parcel of Land's Compensation by Yield
With regard to the grower characteristics, age seems to have a role in quality output. Growers over the age of 45 produce higher-quality output for contract B than other growers. However, the experience of the grower in high-quality contract A (NYA) plays a positive role on the qualitative output for contract C (model 13). This result could mean that the more experience a grower has in high-quality contracts, the more likely he is to do better in terms of quality effort. Overall, this result could mean that after a grower is used to the exigencies of high-quality specifications, it is more likely that he/she will apply those “good practices” to all the contracts. It seems that being specialized in viticulture (VINEYARD) has a negative impact on the qualitative output of grapes for growers with contract B. There is also a slightly positive effect for growers with contract A who belong to the Cave d'Aignan winery.
As for the land characteristics, the main grape variety (TANNAT) plays positive role in compensation for contracts A through C. Quality is thus highly associated with grape variety.
Finally, with regard to the land's pedoclimatic environment, we tested the influence on final qualitative output of the type of soil in which the parcel of land is located. We found that high-quality contracts (model 11) held on molasse soil are likely to increase by 390 euros the compensation of such contracts, all things being equal. Because we lack expertise in agronomy and viticulture, we are not able to explain the relationship between this type of soil and the final qualitative output of grapes. However, if a positive relationship between this type of soil and the qualitative output of contract A would have some management implications for the cooperative. First, it would mean that some types of soils are better suited than others for certain modes of cultivation (quality contracts). This can lead the cooperative to replant vines or to reallocate the contracts proposed to growers to improve the overall quality of grapes. Second, it could also raise doubts about the incentive tournament system. In fact, growers who own land with “rich” soil might have an advantage, regardless of their effort, compared to their peers and thus exert limited effort.
VI. Conclusion
In this paper, we have focused on the relationship between a wine cooperative and its member-growers and the incentive mechanisms used to produce quality grapes. The cooperative's traceability database provides information to decision makers to better manage the production of quality grapes, which we used to test our hypotheses on data from the 2008 harvest. More precisely, we show how a wine cooperative in southwestern France uses a differentiated payment system and contractual provisions, in which promotion (or demotion) to (from) higher-quality contracts works as an incentive toward efforts to improve quality. This incentive system is similar to that of a tournament. In addition, the wine cooperative guides the growers by offering a menu of contracts and by monitoring their activities. The first mechanism is used when the grower chooses the contract. The access to high-quality contracts is explained by the accumulated experience with higher-quality contracts and previous promotions but also by the fact that land is part of a property or grows a specific grape variety: Tannat. The age of growers appears to be a detriment for accessing high-quality contracts, as younger people start with lower-quality contracts.
Testing the tournament system itself, we found that promotion and demotion from quality engagement in previous years significantly influence quality performance. After someone has been demoted from the previous year, he/she will exert greater effort in order to be promoted, or “return,” to a higher-quality contract. Monitoring plays a big role in the tournament system, as the decision whether to downgrade a parcel of land to a lower quality if the minimum quality is not achieved can reduce the tournament mechanism's efficiency. Finally, for all three types of contracts yield control acts as both an incentive for maximum effort and a penalty.
Our results confirm that the tournament mechanism built by the cooperative has two objectives: (1) providing incentives in the presence of a common shock that might affect all the growers; (2) reducing the heterogeneity among growers engaged in the same contest in order to increase the incentives provided by the tournament compensation scheme. This paper contributes to the tournament and contract literature in viticulture by showing that relative performance compensation systems, which offer the possibility of promotion to higher-quality contracts, act as an incentive for growers to exert higher levels of effort.
