4.1. Acoustic characteristics of the baseline nozzle at different NPR values

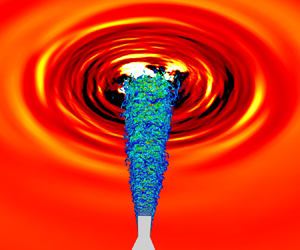
Figure 10 shows the contours of instantaneous pressure fluctuations in the minor-axis plane, coupling with three-dimensional vortical structures in the shear layers, visualized by isosurfaces of the $Q$ criterion (Lesieur, Métais & Comte Reference Lesieur, Métais and Comte2005) and coloured by streamwise velocities. Small-scale stripe vortices induced by the Kelvin–Helmholtz instability (Yule Reference Yule1978; Liepmann & Gharib Reference Liepmann and Gharib1992) are seen near the nozzle lips. As they convect downstream, these vortical structures roll up and develop into large-scale hairpin-like vortices. In the further downstream regions, the fragmentation of vortical structures into smaller-scale vortices occurs due to the turbulent mixing. From the contours of pressure fluctuations, upstream-propagating acoustic waves (referred to as screech tones) and downstream-propagating turbulent mixing noise are clearly resolved for the jets at all $NPR$ values. At $NPR = 3.0$, the screech tone is more intense than those in the other two cases, which is highly linked to the strong flapping motion of jet plume in the minor-axis plane (Gojon et al. Reference Gojon, Gutmark and Mihaescu2019; Chen et al. Reference Chen, Gojon and Mihaescu2021, Reference Chen, Qiang, Wu, Yang and Li2024). The strongest turbulent mixing noise is observed at $NPR = 5.0$, which is mainly attributed to the highest convective velocities in the shear layer.

Figure 10. Contours of instantaneous pressure fluctuations ($-0.005 \le p{'}/p_{\infty } \le 0.005$) in the minor-axis plane, with isosurfaces of the second invariant of velocity gradient tensors ($Q_{2nd} = 10$) coloured by streamwise velocities ($0 \le u/u_{j} \le 1$) for the jets at $NPR = (a)\ 2.3$, (b) 3.0 and (c) 5.0.

The far-field noise at observers with a distance of $55D_{eq}$ from the centre of the nozzle exit is calculated with the FW-H method. The spectra of the far-field noise in the two planes are plotted in figure 11 as a function of the emission angle ($\theta$). Three typical acoustic components (Tam Reference Tam1995), screech tone, BBSAN and turbulent mixing noise (TMN), are observed in the supersonic jets, regardless of the $NPR$. The frequencies of the screech tone are observed at $St = 0.66$, 0.38 and 0.22 for the flow conditions at $NPR = 2.3$, 3.0 and 5.0, respectively, which are in good accordance with the predictions of Reference TamTam's (Reference Tam1988) model. At $NPR = 2.3$ and 3.0, the first harmonics of the screech tones are presented in the direction perpendicular to the jet axis ($\theta \approx 90^{\circ }$), similar to the observations in Tam, Parrish & Viswanathan (Reference Tam, Parrish and Viswanathan2014), but not seen at $NPR = 5.0$. The BBSAN is prominent in the sideline directions ($30^{\circ } \lesssim \theta \lesssim 120^{\circ }$) and spreads in a wide frequency band. It is gradually shifted towards higher $St$, as the emission angle increases. The TMN is mainly evident in the downstream directions ($\theta \gtrsim 140^{\circ }$) at low frequencies, and the amplitudes of TMN at $NPR = 5.0$ are much larger than those at smaller $NPR$ values. Compared with the noise emission in the minor-axis plane, no essential difference is observed in the major-axis plane. Nevertheless, the amplitudes of the screech tones and their harmonics are relatively weak in the major-axis plane. The BBSAN in the major-axis plane seems to be amplified at $NPR = 5.0$, which is probably associated with more intense shock cells formed in the major-axis plane than those in the minor-axis plane, as seen in figures 4(c) and 4(d).

Figure 11. Far-field noise spectra in (a–c) the minor-axis plane and (d–f) the major-axis plane for the jets at $NPR = (a{,}d) 2.3$, (b,e) 3.0 and (c,f) 5.0.

To clarify the near-field oscillating mode of the jet plume, Fourier transformation is applied to the time-series pressure data saved at the grid points in the two principal planes, and 8192 consecutive snapshots with the non-dimensional time interval of 0.04 are used. The phase fields at the frequency of the dominant screech tone are depicted in figure 12. The rectangular jets obey different oscillating modes at different $NPR$ values. At $NPR = 2.3$, as displayed in figures 12(a) and 12(d), the patterns of the phase fields are seen to be symmetric with respect to the jet centreline both in the minor- and major-axis planes. It indicates that the jet at $NPR = 2.3$ oscillates in a symmetric mode (i.e. A mode). At $NPR = 3.0$, an anti-symmetric pattern is exhibited in the minor-axis plane, and a symmetric pattern in the major-axis plane, which suggests that the jet plume is governed by a flapping mode (i.e. B mode) along the minor axis. At $NPR = 5.0$, the phase fields are shown to be anti-symmetric in both planes, forming a distinguishing helical mode (i.e. C mode). The mode switching from A to C mode closely resembles the experimental study of Powell, Umeda & Ishii (Reference Powell, Umeda and Ishii1992), who reported that, as the $NPR$ increases, the jet plume exhausted from a convergent circular nozzle switched from a symmetrical pattern to a flapping pattern, then to a helical pattern. Meanwhile, they also observed that the frequency of the screech tone was reduced with increasing $NPR$.

Figure 12. Fourier phase fields at the dominant screech frequencies in (a–c) the minor-axis plane and (d–f) the major-axis plane for the jets at $NPR = (a{,}d)\ 2.3$, (b,e) 3.0 and (c,f) 5.0.

The phase values along $y/h = 2.35$ in the minor-axis plane and $z/h = 2.35$ in the major-axis plane are extracted and plotted in figure 13, aiming to determine the source location of the dominant screech tone. The propagation direction of acoustic waves can be determined by the sign of the phase slope (Mercier, Castelain & Bailly Reference Mercier, Castelain and Bailly2017; Li et al. Reference Li, He, Zhang, Hao and Yao2019). Positive phase slope represents upstream-propagating acoustic waves, and a negative one characterizes downstream-travelling waves. Identified from figures 13(a) and 13(b), the screech tones at $NPR = 2.3$ and 3.0 are generated at $x/h \approx 2.5$ and 6.5, respectively, where the sign of the phase slope changes. The locations correspond to the end of the third and fourth shock cells in the jet plumes oscillating in the symmetric mode and flapping mode, respectively. At $NPR = 5.0$, the source location of the screech tone appears around $x/h = 12 \sim 14.5$, as seen in figure 13(c), corresponding to the region between the fifth and sixth shock cells. As the $NPR$ value increases, the source location of the screech tone moves further downstream, and a non-compact source location appears when the jet oscillates in the helical mode. These features are in line with the experimental study of rectangular jets by Karnam et al. (Reference Karnam, Baier and Gutmark2019).

Figure 13. Fourier phase values along $y/h = 2.35$ (black solid line) in the minor-axis plane and $z/h = 2.35$ (red dashed line) in the major-axis plane: (a) corresponds to the jet at $NPR = 2.3$, (b) corresponds to the jet at $NPR = 3.0$, (c) corresponds to the jet at $NPR = 5.0$.

4.2. Effects of bevelled nozzles on time-averaged flow fields and turbulent statistics

The contours of time-averaged streamwise velocities (normalized by $u_j$) in the two planes of the nozzles at $NPR = 2.3$ are shown in figure 14. At the exit of the baseline nozzle, the Mach disk is clearly captured due to the mutual interception of oblique shocks originating from the nozzle lips (Paramanantham, Janakiram & Gopalapillai Reference Paramanantham, Janakiram and Gopalapillai2022). For the single-bevelled nozzle, the jet plume in the minor-axis plane is deflected towards the direction of the long lip side, and the shock-cell structures are significantly altered in the deflected jet plume with an oblique shock wave at the exit of the nozzle. For the double-bevelled nozzle, the shock cells remain symmetric in the two planes. However, in comparison with the baseline nozzle, the length of the potential core is reduced due to the premature pressure recovery (Wu & New Reference Wu and New2017), and its width is significantly expanded in the major-axis plane.

Figure 14. Contours of the time-averaged streamwise velocities in (upper half) the minor-axis plane and (lower half) the major-axis plane for (a) the baseline nozzle, (b) the single-bevelled nozzle and (c) the double-bevelled nozzle at $NPR = 2.3$. The streamwise velocities are normalized by the velocities of ideally expanded jets $u_j$.

Figure 15 shows the contours of time-averaged streamwise velocities at $NPR = 3.0$. No Mach disk is formed at the exit of the baseline nozzle, and interactions of oblique shock waves with the shear layer appear in the jet plume. Similar to the case at $NPR = 2.3$, the jet plume in the minor-axis plane of the single-bevelled nozzle is deflected towards the direction of the long lip side, and two oblique shocks intersect at a position close to the long lip. Differently, triangular shock cells are alternately developed in the potential core. For the double-bevelled nozzle, the suppression of the potential core and the enhancement of the shear-layer mixing can be also seen in figure 15(c).

Figure 15. Contours of the time-averaged streamwise velocities in (upper half) the minor-axis plane and (lower half) the major-axis plane for (a) the baseline nozzle, (b) the single-bevelled nozzle and (c) the double-bevelled nozzle at $NPR = 3.0$. The streamwise velocities are normalized by the velocities of ideally expanded jets $u_j$.

Figure 16 displays the contours of time-averaged streamwise velocities at $NPR = 5.0$. As the jet at $NPR = 5.0$ is under-expanded, the shock-cell structures are characterized by barrel shocks (Franquet et al. Reference Franquet, Perrier, Gibout and Bruel2015; Zapryagaev, Kavun & Kiselev Reference Zapryagaev, Kavun and Kiselev2022). The jet plume exhausted from the single-bevelled nozzle is deflected towards the direction of the short lip side, which is different from the over-expanded jets at $NPR = 2.3$ and 3.0. For the double-bevelled nozzle, the width of the potential core is seen to be expanded in the minor-axis plane and compressed in the major-axis plane, which are in contrary to the situations in the over-expanded jets. The phenomenon is related to the premature expansion, which results in the early flow divergence at the nozzle exit along the minor-axis direction.

Figure 16. Contours of the time-averaged streamwise velocities in (upper half) the minor-axis plane and (lower half) the major-axis plane for (a) the baseline nozzle, (b) the single-bevelled nozzle and (c) the double-bevelled nozzle at $NPR = 5.0$. The streamwise velocities are normalized by the velocities of ideally expanded jets $u_j$.

To explain the mechanism responsible for the plume deflection, figure 17(a–c) displays the contours of ${\boldsymbol {V}} \boldsymbol {\cdot } \boldsymbol {\nabla } {\boldsymbol {P}}$ in the minor-axis plane, which are used to identify the shocks (dark) and the expansions (light) in the regions where the pressure gradient ($\boldsymbol {\nabla } {\boldsymbol {P}}$) and velocity (${\boldsymbol {V}}$) are aligned with each other or not. The influences of the throat shocks inside the nozzle can be neglected, as the shock structures are identical inside the three nozzles. The main distinctions arise from the shocks generated at the nozzle exit. For the over-expanded jets (see (a,b)), asymmetric shocks are observed at the nozzle exits, whereas asymmetric expansion fans appear at the nozzle lips of the under-expanded jet (see (c)). The appearance of shocks or expansions is well known to be associated with the nozzle pressure returning to ambient pressure. Therefore, the pressure recovery near the nozzle lips is the key factor for the generation of asymmetric shocks or expansions. The corresponding time-averaged pressure fields are shown in figure 17(d–f). The local pressure difference at the nozzle exit is an indicator to judge the plume deflection. At $NPR = 2.3$ and 3.0, the short lip side undergoes an earlier pressure relief than the long lip side, causing a significantly higher pressure near the short lip side compared with the long lip side at the same streamwise location close to the nozzle exit. The pressure distribution is unbalanced between the short and long lips, which is consistent with the asynchronism of pressure recovery (Wlezien & Kibens Reference Wlezien and Kibens1988). The high-pressure region (marked with a black ‘*’) near the short lip drives the plume to deflect towards the low-pressure region (marked with a red ‘$\times$’) near the long lip. For the case at $NPR = 5.0$, the positions of high- and low-pressure regions are opposite, showing an inverted scenario.

Figure 17. Time-averaged contours of (a–c) ${\boldsymbol {V}} \boldsymbol {\cdot } \boldsymbol {\nabla } {\boldsymbol {P}}$ and (d–f) pressure ($\bar p/{p_\infty }$) in the minor-axis plane for the single-bevelled nozzle at $NPR = (a{,}d)\ 2.3$, (b,e) 3.0 and (c,f) 5.0.

The deflection angles ($\beta$) of the jet plumes exhausted from the single-bevelled nozzle are plotted in figure 18. Negative deflection angles represent the deflections towards the direction of the long lip side, otherwise the short lip side. The numerical results of the single-bevelled circular jets in the study of Wu & New (Reference Wu and New2017) are also added for a qualitative comparison. As the $NPR$ value increases, the deflection angle is increased in a roughly proportional manner (Wu & New Reference Wu and New2017), and changed from negative to positive value once the jet is shifted from over-expanded to under-expanded condition. The variations in deflection angles with $NPR$ values are ascribed to the different levels of static pressure imbalance along the minor-axis direction at different $NPR$ values.

Figure 18. Deflection angles of the jet plumes exhausted from the single-bevelled nozzle.

The Mach numbers along the centrelines of jet plumes are extracted and plotted in figure 19. Note that the jet centrelines in the single-bevelled nozzles are defined along the deflected jet plumes, rather than the $x$ axis of the nozzle. At $NPR = 2.3$, since Mach disks exist at the exits of the baseline and double-bevelled nozzles, as displayed in figures 14(a) and 14(c), the flows past the Mach disk are all subsonic; whereas the flow along the jet centreline of the single-bevelled nozzle is alternatively supersonic and subsonic, allowing for the generation of oblique shock waves in the jet potential core. At $NPR = 3.0$ and 5.0, shock cells exist in jet potential cores, and the strengths of shock cells are weakened in the single-bevelled nozzles, particularly at $NPR = 3.0$. The length of the potential core in the double-bevelled nozzle is the shortest among three nozzles, regardless of the $NPR$ value. At the same time, the Mach number at a given position in the far wake region is the smallest in the double-bevelled nozzle, reflecting the strongest shear-layer mixing effect in this nozzle configuration.

Figure 19. Mach number distributions along the centrelines of jet plumes at $NPR = (a) 2.3$, (b) 3.0 and (c) 5.0. Here, the $s$-axis denotes the transformed axis based on the $x$-axis of the nozzle in the direction of the potential core.

To clarify of the features of shear-layer mixing in the transverse direction, the velocity half-width ($\delta _{0.5}$) at a given streamwise location is calculated from the jet centreline to the corresponding transverse coordinate where the mean streamwise velocity is $50\,\%$ of its value at the centreline. Note that, for the cases of single-bevelled nozzle, the half-widths on the upper and lower sides of the nozzle are different due to the mixing characteristics of the deflected jets. Figure 20 compares the variations of velocity half-widths in the streamwise direction. For the baseline nozzles, the half-widths are seen to grow rapidly in the minor-axis plane, but remain nearly constant or grow slowly in the major-axis plane. The larger shear-layer growth rates and spreading rates along the minor-axis direction are consistent with the previous studies (Zaman Reference Zaman1996; Gojon et al. Reference Gojon, Gutmark and Mihaescu2019; Chakrabarti et al. Reference Chakrabarti, Gaitonde, Nair Unnikrishnan, Stack, Baier, Karnam and Gutmark2022). For the flow exhausted from the bevelled nozzles, the spreading rates are drastically changed. (i) At $NPR = 2.3$, the half-widths in both planes are decreased considerably by using the single-bevelled nozzle, although the jet experiences more spreading at the downstream ($x/h > 11$) of the lower side. The increased spreading indicates there exists a jet-mixing preference on the deflected side. The double-bevelled nozzle accelerates the spreading significantly in the major-axis plane (see (d)), but the suppressions are observed in the minor-axis plane (see (a)). (ii) At $NPR = 3.0$, the impact of the single-bevelled nozzle resembles that at $NPR = 2.3$, with the exception that the jet-mixing preference indistinctively shifts to the upper side of the nozzle (see (b)). The jet with the double-bevelled nozzle spreads at a relatively faster rate than the baseline jet in both planes, particularly in the major-axis plane. (iii) At $NPR = 5.0$, the single-bevelled nozzle is seen to expand and compress the half-widths on the lower and upper sides, respectively, without a discernible influence in the major-axis plane. The spreading rate of the jet with the double-bevelled nozzle shows an significant increase in the minor-axis plane, while exhibiting the opposite behaviour in the major-axis plane, which differs from the observations under the over-expanded conditions. In summary, the plots in figure 20 reveal that the single-bevelled nozzles can suppress the shear-layer mixing in the major-axis plane to varied extents, and the enhanced mixing effects show directional preferences in the minor-axis plane under different conditions. The double-bevelled nozzle has actions to significantly enhance the entrainment and mixing effect in one plane and suppress the spreading in the other plane. The enhanced mixing effect dominates the overall spreading rate, resulting in shorter potential cores (Ioannou & Laizet Reference Ioannou and Laizet2018), as displayed in figure 19.

Figure 20. Streamwise velocity half-width of the jets in (a–c) the minor-axis plane and (d–f) the major-axis plane at $NPR = (a{,}d)\ 2.3$, (b,e) 2.3 and (c,f) 5.0.

The cross-sectional contours of normalized turbulent kinetic energy (TKE$/{u_j^2}$) at four streamwise locations ($x/h = 2$, 6, 10 and 14) of the jets at three $NPR$ values are displayed in figure 21. For the baseline nozzles (see (a–c)), the TKE distributions imitate the shape of the nozzle exit at the section close to the nozzle. Further downstream, the axis-switching phenomena in rectangular jets (Chen & Yu Reference Chen and Yu2014; Kim & Park Reference Kim and Park2020) can be observed, especially at the largest $NPR$. The sharp corners are able to induce four streamwise vortex pairs in the downstream sections (Grinstein Reference Grinstein1995; Bhide et al. Reference Bhide, Siddappaji and Abdallah2021), which are responsible for the inward penetrations of the TKE at the four edges and outward spreads at the four sharp corners. The TKE magnitudes at the edges are relatively stronger than those at the corners. For the single-bevelled nozzles (see (d–f)), the TKE distributions are asymmetric with respect to the major-axis plane, which is attributed to the asymmetric intensities of the corner vortex pairs. The stronger vortices on the short lip sides result in the relatively wider mixing layers than those on the long lip sides. The influences of the single bevel on the TKE magnitudes and axis switching are not obvious, if compared with the baseline nozzle. Different situations are observed in the cases of double-bevelled nozzles (g–i). The vortical effects of sharp corners appear to be absent, and the distribution and evolution of the TKE levels are preferred in one direction. At $x/h = 6$ and 10, the TKE magnitudes are significantly enhanced at all $NPR$ values, and the TKE distributions are elongated in the major-axis direction of the over-expanded jets ($NPR = 2.3$ and 3.0), but elongated in the minor-axis direction of the under-expanded jet ($NPR = 5.0$).

Figure 21. Cross-sectional contours of turbulent kinetic energy at different streamwise locations of the jets issued from (a–c) the baseline nozzle, (d–f) the single-bevelled nozzle and (g–i) the double-bevelled nozzle at $NPR = (a{,}d{,}g)\ 2.3$, (b,e,h) 3.0 and (c,f,i) 5.0.

It is known that the modifications of mean flow structures inadvertently influence the aerodynamic performance. We calculate the nozzle thrust by applying the following integrated equation at the nozzle exit plane:

(4.1) \begin{align} Thrust = {\underbrace{\unicode{x222F} {\dot m{u_e}\, {\rm d} A}}_{\textit{Momentum thrust}}} + {\underbrace{\unicode{x222F} {( {{p_e} - {p_\infty }})\, {\rm d} A}}_{\textit{Pressure thrust}}}, \end{align}

where $\dot m$ is the mass flow rate, ${u_e}$ is the axial velocity at the nozzle exit, ${p_e}$ is the static pressure at the nozzle exit, ${p_\infty }$ is the ambient pressure, ${\rm d} A$ is the element area projected along the axial direction and $\unicode{x222F}$ denotes surface integration. Figure 22 shows the non-dimensional thrusts and corresponding thrust loss coefficients for the nozzles. At the over-expanded condition (${p_e} < {p_\infty }$), the pressure thrust contributes negatively to the gross thrust; conversely, it contributes positively at the under-expanded condition (${p_e} > {p_\infty }$). The momentum thrust dominates the gross thrust, regardless of the inflow conditions. As seen in figure 22(d–f), the gross thrusts are increased for all bevelled nozzles, and the increased values are specified in table 3. By using the single-bevelled nozzle, the momentum thrust is reduced and the pressure thrust is increased, regardless of the jet operating conditions. The increase of gross thrust results from the increase of pressure thrust. The degradation in momentum thrust is attributed to the reduction of the mass flow rate, as the effective flow area of the single-bevelled nozzle is reduced due to the non-uniform pressure distribution at the exit plane (Viswanathan Reference Viswanathan2005; Viswanathan & Czech Reference Viswanathan and Czech2011). The reduced shock strength at the single-bevelled nozzle exit has a beneficial effect for pressure thrust improvement. In the cases of double-bevelled nozzle, the increase of gross thrust is determined by the increased pressure thrust at $NPR=2.3$ and 3.0, and momentum thrust at $NPR=5.0$. It can be seen that the momentum thrust is lower than that of the baseline nozzle at $NPR = 2.3$, which is related to the drop in axial flow rate resulting from the suppression of the jet width along the minor-axis direction (shown in figure 20a). However, the improvement is observed at $NPR = 3.0$ and 5.0, and it benefits from the growth of the jet spreading along the minor-axis direction, as displayed in figures 20(b) and 20(c). Increased pressure thrust is seen as a result of weakened shock wave on the double-bevelled exit plane at the over-expanded conditions, whereas the enhanced expansion fan on the exit leads to a loss in pressure at the under-expanded condition.

Figure 22. Comparisons of (a–c) non-dimensional thrusts and (d–f) thrust loss coefficients between the baseline and bevelled nozzles at $NPR = (a{,}d)\ 2.3$, (b,e) 3.0 and (c,f) 5.0.

Table 3. The gross thrust loss coefficients.

4.3. Effects of bevelled nozzles on the far-field noise radiation

The sound pressure levels at far-field observers with a distance of $55 D_{eq}$ to the nozzle origin are calculated with the FW-H acoustic analogy method. The parameter settings are the same as those introduced in § 3.3. Note that, for the single-bevelled nozzles, the FW-H permeable surfaces are designed along the deflected jet plumes, in order to prevent intersecting with the nonlinear turbulence. The observers in the minor-axis planes of the single-bevelled nozzles are separated into upper (i.e. short lip side) and lower (i.e. long lip side) ones, considering the asymmetries of jet plumes with respect to the $x$-axis.

Figure 23 shows the variations of OASPLs in the two planes. For the symmetrically oscillating jet at $NPR = 2.3$ (see (a,d)), the single-bevelled nozzle is capable of reducing the OASPLs at the upstream angels ($\theta < 45^{\circ }$) by 1–3 dB in the minor-axis plane and approximately 1 dB in the major plane. The OASPLs at lower observers of the single-bevelled nozzle are mostly larger than those of upper ones, leading to noise increases at the downstream angles if compared with the baseline nozzle. It is associated with the stronger mixing noise generated by the fast shear-layer spreading at the downstream locations of the single-bevelled jet, as shown earlier in figure 20(a). Using the double-bevelled nozzle, efficient noise reductions are obtained at all emission angles in the minor-axis plane, with a maximum reduction of 5 dB seen at $\theta = 35^{\circ }$; whereas, in the major plane, noise reductions only appear at upstream angles. Overall, the double-bevelled nozzle performs better than the single-bevelled nozzle in reducing the OASPLs, except at $85^{\circ } \leq \theta \leq 150^{\circ }$ in the major-axis plane. This differs from the observation in the experimental study of the supersonic circular jet (Wei et al. Reference Wei, Chua, Lu, Lim, Mariani, Cui and New2022), in which the double-bevelled nozzle does not suppress the noise levels but amplifies the amplitude of the screech tone significantly. It should be noted that the oscillation mode of the circular jet (Wei et al. Reference Wei, Chua, Lu, Lim, Mariani, Cui and New2022) is helical (i.e. anti-symmetric), but the current rectangular jet at $NPR = 2.3$ oscillates in a symmetric mode.

Figure 23. Far-field OASPLs comparison between the jets issued from the baseline nozzle and the jets issued from the bevelled nozzle at various emission angles in (a–c) the minor-axis plane and (d–f) the major-axis plane at $NPR = (a{,}d)\ 2.3$, (b,e) 3.0 and (c,f) 5.0.

For the flapping jet at $NPR = 3.0$, as shown in (b,e), the OASPLs at all emission angles are reduced in both planes by using the single-bevelled nozzle, especially at upstream angles, where maximum reductions of 6 and 3 dB are obtained in the minor- and major-axis plane, respectively. For the double-bevelled nozzle, the suppressions of OASPLs are obviously seen at $\theta < 135^{\circ }$ in the major-axis plane, but dramatic increases are shown in the upstream ($\theta \leq 55^{\circ }$) and sideline ($85^{\circ } \leq \theta \leq 110^{\circ }$) directions of the minor-axis plane. Regarding the helically oscillating jet at $NPR = 5.0$, as seen in (c,f), the bevelled nozzle has fewer influences on the OASPLs, if compared with the jets at $NPR = 2.3$ and 3.0. Importantly, the double-bevelled nozzle is able to suppress the peak noise levels at $\theta \approx 150^{\circ }$, but causes considerable increases at upstream and sideline emission angles.

The variations of OASPLs are linked to the features of the noise spectra. Here, the noise spectra at three emission angles ($\theta = 35^{\circ }, 90^{\circ }$ and $140^{\circ }$) in the minor-axis plane are displayed in figure 24, attempting to explain the changes of OASPLs in figure 23(a–c). At $NPR = 2.3$, the screech tone at $\theta = 35^{\circ }$ is evidently mitigated by the single-bevelled nozzle and completely eliminated by the double-bevelled nozzle. It is directly related to the noise suppression at the upstream emission angles (see figure 23a), where the screech tone dominates the overall sound pressure levels. At $\theta = 90^{\circ }$, the harmonics of the screech tone is suppressed by the bevelled nozzles, which may cause the reduction of OASPLs. However, the OASPL at this angle is increased in the case of single-bevelled nozzle, which is ascribed to the enhanced broadband noise at $0.8 \lesssim St \lesssim 4.0$. The enhanced broadband noise is mostly related to the increase of the shock strength and its interaction with the shear layer, as seen in figure 19(a). At $NPR = 3.0$, the elimination or amplification of the screech tone (or its harmonics) at $\theta = 35^{\circ }$ and $90^{\circ }$ is the key reason for the changes of OASPLs shown in figure 23(b). In the case of the double-bevelled nozzle, the frequencies of the screech tone and its harmonics are shifted to higher values, which are attributed to the shortened shock-cell spacings (see figure 19b), supported by the theory of Powell (Reference Powell1953). The noise spectra at $NPR = 5.0$ have no apparent difference among the three nozzles, except that the screech tone at $\theta = 35^{\circ }$ is enhanced and shifted to a higher frequency by the double-bevelled nozzle. These features also support the variations of OASPLs in figure 23(c).

Figure 24. Far-field spectra comparison between the jets issued from the baseline nozzle and the jets issued from the bevelled nozzle at three emission angles in the minor-axis plane at $NPR = (a)\ 2.3$, (b) 3.0 and (c) 5.0.

4.4. Two-point space–time cross-correlation analysis

In order to investigate the propagation of flow and acoustic waves in the jet shear layers, two-point space–time cross-correlation analysis is performed using the fluctuating pressure data obtained along the lower shear layers in the minor-axis plane. The reference point is positioned at $x/h=5.0$, and 150 targeted points are equally distributed from the nozzle lip to the streamwise location of $x/h = 15$. The correlations are presented in figure 25. The maximum correlation is visible at $(x/h, \tau ) = (5.0, 0)$, since the cross-correlation is transformed into the auto-correlation at the reference point.

Figure 25. The space–time cross-correlations of pressure fluctuations along the shear layers in the minor-axis plane for (a,d,g) the baseline nozzles, (b,e,h) the single-bevelled nozzles and (c,f,i) the double-bevelled nozzles, at $NPR = (a\unicode{x2013}c)\ 2.3$, (d–f) 3.0 and (g–i) 5.0.

The results of the baseline nozzle are shown in (a,d,g). Well-organized stripes with two kinds of slopes are periodically arranged along the time-lag axis. The positive slopes imply that the acoustic waves are propagating in the upstream direction, and the negative slopes are related to the downstream-travelling hydrodynamic waves. The time lag exhibits the same periodic interval for the acoustic and hydrodynamic waves, which also corresponds to the dominant frequency of the screech. It reveals that the downstream-propagating acoustic waves at the dominant frequency of screech are linked to the convection of coherent flow structures in the shear layer. The correlation coefficients are highest at $NPR = 3.0$ and relatively faint at $NPR = 5.0$. This phenomenon is in line with the amplitudes of the screech shown in figure 11. For the bevelled nozzles, the situations are similar. Stripe patterns are observed in (b,f,i), at the conditions with the generation of screech. For the cases without screech, the organized stripes disappear. For instance, at $NPR = 3.0$, the stripe patterns are analogous between the baseline and double-bevelled nozzle, and the correlation coefficients are amplified in the double-bevelled nozzle, suggesting that the screech is more evident than that in the baseline nozzle. The invisible stripe patterns in the single-bevelled nozzle indicate the elimination of the screech.

4.5. Dynamic mode decomposition

Dynamic mode decomposition (DMD) is a data-driven modal decomposition method to identify relevant dynamical structures in turbulent flows (Schmid Reference Schmid2010; Schmid et al. Reference Schmid, Li, Juniper and Pust2010; Taira et al. Reference Taira, Brunton, Dawson, Rowley, Colonius, McKeon, Schmidt, Gordeyev, Theofilis and Ukeiley2017). Given a sequence of $m$ snapshots of the flow field, the sequence is condensed into a matrix notation

(4.2)\begin{equation} {\boldsymbol{\mathsf{Q}}}_1^{m} = [ {{{{\mathsf{q}}}_1},{{{\mathsf{q}}}_2}, \ldots ,{{{\mathsf{q}}}_{m}}} ].\end{equation}

Assuming there exists a linear operator ${\boldsymbol{\mathsf{B}}}$, the snapshot ${{\boldsymbol {q}}_{i + 1}}$ is able to be obtained from the previous snapshot ${{\boldsymbol {q}}_i}$, that is

(4.3)\begin{equation} {{\boldsymbol{q}}_{i + 1}} = {\boldsymbol{\mathsf{B}}}{{\boldsymbol{q}}_i}, \end{equation}

where the matrix ${\boldsymbol{\mathsf{B}}}$ represents the system matrix of the high-dimensional flow field, which describes the dominantly dynamical behaviour of the flow. Then we have

(4.4)\begin{equation} {\boldsymbol{\mathsf{Q}}}_2^{m} = [{{\boldsymbol{q}}_2},{{\boldsymbol{q}}_3}, \ldots ,{{\boldsymbol{q}}_{m}}] = [{\boldsymbol{\mathsf{B}}}{{\boldsymbol{q}}_1},{\boldsymbol{\mathsf{B}}}{{\boldsymbol{q}}_2}, \ldots ,{\boldsymbol{\mathsf{B}}}{{\boldsymbol{q}}_{m-1}}] = {\boldsymbol{\mathsf{B}}}{\boldsymbol{\mathsf{Q}}}_1^{m-1}.\end{equation}

As the number of snapshots ($m$) increases, the sequence ${\boldsymbol{\mathsf{Q}}}_1^{m}$ is able to capture the dominant feature of flow field, and the last snapshot ${{\boldsymbol {q}}_{m}}$ can be expressed as a linear combination of the previous snapshots

(4.5)\begin{equation} {{\boldsymbol{q}}_{m}} = {c_1}{{\boldsymbol{q}}_1} + {c_2}{{\boldsymbol{q}}_2} + \cdots + {c_{m-1}}{{\boldsymbol{q}}_{m-1}}= {\boldsymbol{\mathsf{Q}}}_1^{m-1}{\boldsymbol{c}},\end{equation}

where the vector ${\boldsymbol {c}} = ({c_1}, {c_2}, \ldots, {c_{m-1}})$, and the coefficient ${c_i}$ can be obtained by solving the least-squares solution of (4.5) utilizing singular value decomposition. Then, (4.4) is further written as

(4.6)\begin{equation} {\boldsymbol{\mathsf{B}}}{\boldsymbol{\mathsf{Q}}}_1^{m-1}= {\boldsymbol{\mathsf{Q}}}_2^{m} = {\boldsymbol{\mathsf{Q}}}_1^{m-1}{\boldsymbol{\mathsf{C}}}, \end{equation}

with the matrix ${\boldsymbol{\mathsf{C}}}$ being

(4.7) \begin{equation} {\boldsymbol{\mathsf{C}}} = \left[ \begin{array}{@{}ccccc@{}} 0 & 0 & \cdots & 0 & {{c_1}}\\ 1 & 0 & \cdots & 0 & {{c_2}}\\ 0 & 1 & \cdots & 0 & {{c_3}}\\ \vdots & {} & \ddots & {} & \vdots \\ 0 & 0 & \cdots & 1 & {{c_{m-1}}} \end{array} \right]. \end{equation}

With the above matrix ${\boldsymbol{\mathsf{C}}}$, we definite the residual of the linear combination as

(4.8)\begin{equation} {\boldsymbol{r}} = {\boldsymbol{q}}_{m} - {\boldsymbol{\mathsf{Q}}}_1^{m-1}{\boldsymbol{c}}. \end{equation}

When the residual ${\boldsymbol {r}}$ is minimized, the eigenvalues of the matrix ${\boldsymbol{\mathsf{C}}}$ are approximations of the eigenvalues of the matrix ${\boldsymbol{\mathsf{B}}}$. These eigenvalues are mapped onto the complex plane by a logarithmic mapping, in which the real part represents the growth rate of the mode, and the imaginary part gives the frequency information. The eigenvector ($\boldsymbol {\upsilon }$) or the spatial variation of the DMD mode (${\boldsymbol {D}}$) can be obtained from the eigenvector (${\boldsymbol {T}}$) of the matrix ${\boldsymbol{\mathsf{C}}}$ and the matrix ${\boldsymbol{\mathsf{Q}}}$, that is,

(4.9)\begin{equation} {\boldsymbol{D}} = {\boldsymbol{\mathsf{Q}}}_1^{m-1}{\boldsymbol{T}}^{-1}. \end{equation}

The $L2$ norm of the eigenvector $\boldsymbol {\upsilon }$ is regarded as the energy of each mode. More mathematical theories and implementation details of the DMD algorithm are given in Schmid (Reference Schmid2010).

In this study, we use DMD to extract the dynamical structures of shear-layer oscillations and the motions of shock cells at pertinent frequencies. The DMD analyses of the nine cases (three nozzles at three $NPR$ values) are performed by using the pressure fluctuations in the minor- and major-axis planes. In each case, a total of 4096 consecutive snapshots with a non-dimensional time interval of 0.05 are employed. The time duration are approximately 46 times longer than the largest period of the screech tone at $St = 0.22$ (i.e. the non-dimensional time is approximately equal to 4.38).

The energy spectra of the DMD modes are plotted in figure 26. For the baseline nozzle at $NPR = 2.3$, the dominant mode has a peak frequency at $St = 0.66$, which is consistent with the frequency of the screech tone in the noise spectrum, as shown in figure 24(a). Meanwhile, the harmonic mode at the frequency of $St = 1.32$ also corresponds to the first harmonics of the screech tone. It indicates that the identified low-rank dynamical structures are linked to the generation of the tonal noise (Lim et al. Reference Lim, Wei, Zang, Vevek and Cui2020; Karami & Soria Reference Karami and Soria2021). With the single- and double-bevelled nozzles, the peak in the energy spectra is suppressed or eliminated, implying that the spatial structures in the baseline case are disturbed. Figures 26(b) and 26(c) show that the frequencies of the most energetic modes of the baseline cases at $NPR = 3.0$ and 5.0 are also consistent with the frequencies of the dominant screech tones. Differently, the distinct dynamic modes are absent by using the single-bevelled nozzle; whereas the double-bevelled nozzle enhances the energy of DMD modes associated with the screech tone and its harmonics. These features are similar to those in the noise spectra.

Figure 26. The DMD energy spectra in the minor-axis plane for the jets at $NPR = (a)\ 2.3$, (b) 3.0 and (c) 5.0. The red circles represent the chosen dominant modes.

Figure 27 illustrates the spatial distributions of the real part of the DMD modes at the most energetic frequencies, which are marked by red circles in figure 26. Note that, for the case without a dominant frequency, we choose the same frequency as the baseline nozzle. The wavepackets in figure 27 represent the spatially modulating standing waves (Westley & Woolley Reference Westley and Woolley1975; Panda Reference Panda1999; Gojon & Bogey Reference Gojon and Bogey2017), which characterize the interactions between the upstream-propagating acoustic waves and the downstream-travelling hydrodynamic instability waves. The symmetric/anti-symmetric patterns of the baseline nozzle are perfectly consistent with the distributions of the Fourier phase fields in figure 12, but disturbed by the bevelled nozzles. At $NPR = 2.3$, the well-organized symmetric mode remains in the single-bevelled nozzle, but tilted along the centreline of the deflected potential core. However, chaotic and fragmented patterns are seen in the shear layer of the double-bevelled nozzle, and the upstream-propagating acoustic stripes are invisible, suggesting the destruction of the fluid-acoustic feedback loop and the absence of the screech tone in the upstream direction. At $NPR = 3.0$ and 5.0, anti-symmetric modes are seen in the minor-axis planes of the baseline and double-bevelled nozzles, but disappear in the cases of single-bevelled nozzle. It supports that the regularly oscillating shear layer is a vital factor for the generation of the screech tone.

Figure 27. The real part of the DMD modes at the dominant frequencies in the minor-axis plane for (a,d,g) the baseline nozzles, (b,e,h) the single-bevelled nozzles and (c,f,i) the double-bevelled nozzles at $NPR = (a\unicode{x2013}c)\ 2.3$, (d–f) 3.0 and (g–i) 5.0.

The DMD modes in the major-axis planes are shown in figure 28. Note that the major-axis planes in the cases of single-bevelled nozzle are deflected along the centrelines of jet plumes. Well-organized wavepackets are seen in the cases with the generation of screech tones, and fragmented structures are seen in the cases without the generation of screech tones, similar to the observations in the minor-axis planes shown in figure 27. Importantly, in the cases with the generation of screech tones, the oscillating modes of shear layers are not altered by the bevelled nozzles, that is, the symmetrical mode in the single-bevelled nozzle at $NPR = 2.3$, the flapping mode in the double-bevelled nozzle at $NPR = 3.0$ and the helical mode in the double-bevelled nozzle at $NPR = 5.0$, are in line with the modes in the baseline nozzles at corresponding $NPR$ values. On the other hand, in the cases without the generation of screech tones, the regularly oscillating modes are absent.

Figure 28. The real part of the DMD modes at the dominant frequencies in the major-axis plane for (a,d,g) the baseline nozzles, (b,e,h) the single-bevelled nozzles and (c,f,i) the double-bevelled nozzles at $NPR = (a\unicode{x2013}c)\ 2.3$, (d–f) 3.0 and (g–i) 5.0.

Figure 23(b) has suggested that considerable increases of OASPLs are observed in the sideline emission angles of the double-bevelled nozzle. Here, we explain this phenomenon by showing the first harmonic DMD modes in the minor-axis planes of the baseline and double-bevelled nozzles at $NPR = 3.0$, as displayed in figure 29. It can be seen that the acoustic beams are non-compactly distributed and mainly radiated in the sideline directions ($\theta \approx 90^{\circ }$). Their scales are smaller than those of the dominant DMD modes, as expected. Tam et al. (Reference Tam, Parrish and Viswanathan2014) stated that the high-order harmonic tones are initiated from the interaction of the nonlinear harmonic instability waves and the shock cells, and their directional radiation features are different from those of the dominant screech tones. The most energetic instability wave, which drives the fluid-acoustic feedback loop, can be transferred to high-order modes, and cause intense harmonic tones radiated in different directions (Tam et al. Reference Tam, Parrish and Viswanathan2014; Semlitsch et al. Reference Semlitsch, Malla, Gutmark and Mihăescu2020). In the current jets, the harmonic instability wavepackets in the jet plumes are observed to be symmetric, together with symmetric acoustic beams radiated in the sideline directions, which are in contrast to the asymmetric features of the flapping jets at the dominant screech frequencies. The patterns of the harmonic instabilities and acoustic waves are more regular and stronger in the jet with double-bevelled nozzle, as shown in figure 29(b), providing evidence for the noise level increase in the sideline emission angles.

Figure 29. The real part of the DMD modes at the first harmonic frequencies in the minor-axis plane for (a) the baseline nozzle and (b) the double-bevelled nozzle at $NPR = 3.0$.

4.6. Frequency–wavenumber analysis

Four fundamental components, including downstream-convecting turbulent structures (Sinha et al. Reference Sinha, Rodríguez, Brès and Colonius2014), shock leakage (Suzuki & Lele Reference Suzuki and Lele2003; Edgington-Mitchell et al. Reference Edgington-Mitchell, Weightman, Lock, Kirby, Nair, Soria and Honnery2021b), upstream-travelling acoustic waves and the receptivity mechanism near the nozzle lips (Karami et al. Reference Karami, Stegeman, Ooi, Theofilis and Soria2020), exist in the fluid-acoustic feedback mechanism, which is responsible for the generation of the screech tones in supersonic jets (Edgington-Mitchell Reference Edgington-Mitchell2019; Edgington-Mitchell et al. Reference Edgington-Mitchell, Wang, Nogueira, Schmidt, Jaunet, Duke, Jordan and Towne2021a). The upstream-propagating guided-jet modes (i.e. the neutral acoustic modes) (Shen & Tam Reference Shen and Tam2002; Edgington-Mitchell et al. Reference Edgington-Mitchell, Jaunet, Jordan, Towne, Soria and Honnery2018; Mancinelli et al. Reference Mancinelli, Jaunet, Jordan and Towne2019), rather than the classical free-stream sound waves (Powell Reference Powell2002), play a vital role in closing the resonance loop. In this subsection, the spatio-temporal features of the jet shear layers are analysed by using a vortex-sheet model proposed by Tam & Norum (Reference Tam and Norum1992), to shed light on the existence of upstream-propagating guided-jet modes, and to illustrate that these waves are directly related to the generation of the screech tones, as well as to examine the impacts of the bevel cuts on these guided-jet modes and the screech feedback mechanisms.

Based on the linearized equations of a compressible inviscid flow, the vortex-sheet model (Tam & Norum Reference Tam and Norum1992) can predict the dispersion relations of the guided-jet wave modes, which are given as

(4.10)\begin{equation} \frac{[( \omega - u_jk)^2/a_j^2 - k^2 ]^{1/2} \rho _\infty \omega^2}{( k^2 - \omega^2/a_\infty^2 )^{1/2} \rho _j (\omega - u_j k )^2} - \cot \{ [( \omega - u_jk )^2/a_j^2 - k^2]^{1/2} h_j/2 \} = 0,\end{equation}

for symmetric modes, and

(4.11)\begin{equation} \frac{[(\omega - u_jk)^2/a_j^2 - k^2 ]^{1/2} \rho_\infty \omega^2}{(k^2 - \omega^2/ a_\infty^2 )^{1/2} \rho_j ( \omega - u_jk )^2} + \tan \{ [(\omega - u_j k)^2/a_j^2 - k^2 ]^{1/2} h_j/2\} = 0,\end{equation}

for anti-symmetric modes, where $k$ is the wavenumber, $\omega$ is the angular frequency, $a_{j}$ and $a_{\infty }$ are the speeds of sound in the ideally expanded jet and the ambient air, respectively, and $\rho _{j}$ and $\rho _{\infty }$ are the densities in the ideally expanded jet and the ambient air, respectively. Figure 30 shows the dispersion relations of the guided-jet modes predicted using the vortex-sheet model for the jets at three $NPR$ values. The dispersion relations (solid lines) of the symmetric modes (referred to as S1, S2 and S3) and the anti-symmetric modes (referred to as A1, A2 and A3) are displayed in (a) and (b), respectively. The relations with negative slopes represent the guided-jet modes propagating upstream with negative group velocities. The dashed lines, which connect the lower limits (marked by triangles) of the negative slopes, denote the speeds of the upstream-propagating sound waves, i.e. $a_{\infty }$. The upper limits of the negative slopes correspond to the zero slopes of the relation curves. It can be seen that upstream-propagating guided-jet modes can only exist within an allowable frequency range, that is the range between the upper and lower limits, and the propagation velocities of the waves are smaller than the ambient speeds of sound waves.

Figure 30. The vortex-sheet dispersion relations of (a) the symmetric and (b) the anti-symmetric guided-jet wave modes (solid lines) for three jets issued from the baseline nozzle. The triangles indicate the lower limits of the modes. The dispersion relations of acoustic waves are represented by the dashed lines.

The simulated pressure fluctuations in the minor-axis plane along $y/h = -0.5$ from $x/h =0$ to 15 are spatio-temporally Fourier transformed into the frequency–wavenumber spectra shown in figure 31. For the cases of the single-bevelled nozzle, we use the instantaneous data along the deflected shear layers. The theoretical predictions of selected guided-jet wave modes are also added in figure 31 for comparison. In the positive wavenumber regions ($k>0$), the high contour levels are seen to be organized along the line of $k = {\omega }/u_{c}$ for all cases, where $u_{c} = 0.68u_{j}$ is the convective velocity of the Kelvin–Helmholtz instability waves. The line represents the trace of the downstream-convecting turbulent structures, which is a hydrodynamic component in the feedback loop. In the negative wavenumber regions ($k<0$), the clusters of the contours help to indicate the frequencies of the dominant screech tones and their relations with the theoretically predicted guided-jet wave modes. For the cases with the generation of screech tones, as shown in (a,b,d,f,g,i), the contours are clustered at the same frequency of the corresponding dominant screech tone, and fall between the upper and lower limits of a theoretically predicted guided-jet wave mode, that is, the symmetric mode S2 in the baseline nozzle at $NPR = 2.3$, the symmetric mode S2 in the single-bevelled nozzle at $NPR = 2.3$, the anti-symmetric mode A1 in the baseline nozzle at $NPR = 3.0$, the anti-symmetric mode A1 in the double-bevelled nozzle at $NPR = 3.0$, the anti-symmetric mode A1 in the baseline nozzle at $NPR = 5.0$ and the anti-symmetric mode A1 in the double-bevelled nozzle at $NPR = 5.0$. The symmetric/anti-symmetric features are identical to the observations of the Fourier phase analysis, further confirming the dynamical modes (symmetric, flapping and helical modes) of jet oscillations observed in § 4.4. The above results also reveal that, at the frequency of the dominant screech tone, the upstream-propagating guided-jet modes propagate inside the jet plume with the group velocities close to the speed of sound waves, and they make contributions to the closure of the feedback mechanism. Compared with the baseline nozzle, the magnitudes of the clustering bands are suppressed in the single-bevelled nozzle at $NPR = 2.3$, while they are amplified in the double-bevelled nozzles at $NPR = 2.3$ and 5.0. The suppression/enhancement of the guided-jet modes corresponds to the reduction/increase in the noise levels of the screech tones, which have been discussed in § 4.3, further emphasizing the importance of the guided-jet modes in the feedback loop. On the other hand, for the cases without the generation of the screech tones, as displayed in (c,e,h), no evident band is clustered in the allowable frequency range between the upper and lower limits of a theoretically predicted mode, as expected. This suggests that no neutral waves exist to trigger the acoustic resonance and further supports that the upstream-propagating guided-jet mode is a critical factor in closing the feedback loop.

Figure 31. Frequency–wavenumber spectra of the pressure fluctuations in the minor-axis plane along the shear layers for the jets issued from (a,d,g) the baseline nozzle, (b,e,h) the single-bevelled nozzle and (c,f,i) the double-bevelled nozzle at $NPR = (a\unicode{x2013}c)\ 2.3$, (d–f) 3.0 and (g–i) 5.0. The solid lines provide the theoretical dispersion relations of guided-jet wave modes plotted in the same way as in figure 30. The red and blue dashed lines represent the dispersion relations of downstream-convecting structures and upstream-propagating acoustic waves, respectively.

Figure 32 shows the contours of frequency–wavenumber spectra in the major-axis plane calculated by using the pressure fluctuations along $z/h = 1$ (deflected shear layers in the single-bevelled jets) from $x/h = 0$ to 15. Similar to the observations in the minor-axis plane, the clustering bands in the negative wavenumber regions illustrate the existence of the guided-jet modes, and their symmetric/anti-symmetric features are identical to the observations of the Fourier phase and DMD analysis. Differently, the magnitudes of the guided-jet modes are relatively weaker in the major-axis plane, especially when the flapping mode governs the oscillations of the jet plume, i.e. the baseline and double-bevelled nozzles at $NPR = 3.0$, displayed in (d,f).

Figure 32. Frequency–wavenumber spectra of the pressure fluctuations in the major-axis plane along the shear layers for the jets issued from (a,d,g) the baseline nozzle, (b,e,h) the single-bevelled nozzle and (c,f,i) the double-bevelled nozzle at $NPR = (a\unicode{x2013}c)\ 2.3$, (d–f) 3.0 and (g–i) 5.0. The solid lines provide the theoretical dispersion relations of guided-jet wave modes plotted in the same way as in figure 30. The red and blue dashed lines represent the dispersion relations of downstream-convecting structures and upstream-propagating acoustic waves, respectively.