A one-sided shift of finite type $(\mathsf{X}_{A},\unicode[STIX]{x1D70E}_{A})$ determines on the one hand a Cuntz–Krieger algebra ${\mathcal{O}}_{A}$ with a distinguished abelian subalgebra ${\mathcal{D}}_{A}$ and a certain completely positive map $\unicode[STIX]{x1D70F}_{A}$ on ${\mathcal{O}}_{A}$. On the other hand, $(\mathsf{X}_{A},\unicode[STIX]{x1D70E}_{A})$ determines a groupoid ${\mathcal{G}}_{A}$ together with a certain homomorphism $\unicode[STIX]{x1D716}_{A}$ on ${\mathcal{G}}_{A}$. We show that each of these two sets of data completely characterizes the one-sided conjugacy class of $\mathsf{X}_{A}$. This strengthens a result of Cuntz and Krieger. We also exhibit an example of two irreducible shifts of finite type which are eventually conjugate but not conjugate. This provides a negative answer to a question of Matsumoto of whether eventual conjugacy implies conjugacy.

We realize Leavitt ultragraph path algebras as partial skew group rings. Using this realization we characterize artinian ultragraph path algebras and give simplicity criteria for these algebras.

Let $T_{1}$, $T_{2}$ be two Calderón–Zygmund operators and $T_{1,b}$ be the commutator of $T_{1}$ with symbol $b\in \text{BMO}(\mathbb{R}^{n})$. In this paper, by establishing new bilinear sparse dominations and a new weighted estimate for bilinear sparse operators, we prove that the composite operator $T_{1}T_{2}$ satisfies the following estimate: for $\unicode[STIX]{x1D706}>0$ and weight $w\in A_{1}(\mathbb{R}^{n})$,

$$\begin{eqnarray}\displaystyle & & \displaystyle w(\{x\in \mathbb{R}^{n}:\,|T_{1}T_{2}f(x)|>\unicode[STIX]{x1D706}\})\nonumber\\ \displaystyle & & \displaystyle \qquad \lesssim [w]_{A_{1}}[w]_{A_{\infty }}\log (\text{e}+[w]_{A_{\infty }})\int _{\mathbb{R}^{n}}\frac{|f(x)|}{\unicode[STIX]{x1D706}}\log \bigg(\text{e}+\frac{|f(x)|}{\unicode[STIX]{x1D706}}\bigg)w(x)\,dx,\nonumber\end{eqnarray}$$

$T_{1,b}T_{2}$

$$\begin{eqnarray}\displaystyle & & \displaystyle w(\{x\in \mathbb{R}^{n}:\,|T_{1,b}T_{2}f(x)|>\unicode[STIX]{x1D706}\})\nonumber\\ \displaystyle & & \displaystyle \qquad \lesssim [w]_{A_{1}}[w]_{A_{\infty }}^{2}\log (\text{e}+[w]_{A_{\infty }})\int _{\mathbb{R}^{n}}\frac{|f(x)|}{\unicode[STIX]{x1D706}}\log ^{2}\bigg(\text{e}+\frac{|f(x)|}{\unicode[STIX]{x1D706}}\bigg)w(x)\,dx.\nonumber\end{eqnarray}$$

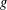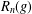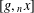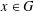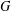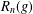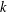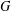Let $g$ be an element of a finite group $G$ and let $R_{n}(g)$ be the subgroup generated by all the right Engel values $[g,_{n}x]$ over $x\in G$. In the case when $G$ is soluble we prove that if, for some $n$, the Fitting height of $R_{n}(g)$ is equal to $k$, then $g$ belongs to the $(k+1)$th Fitting subgroup $F_{k+1}(G)$. 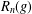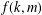For nonsoluble $G$, it is proved that if, for some $n$, the generalized Fitting height of $R_{n}(g)$ is equal to $k$, then $g$ belongs to the generalized Fitting subgroup $F_{f(k,m)}^{\ast }(G)$ with $f(k,m)$ depending only on $k$ and $m$, where $|g|$ is the product of $m$ primes counting multiplicities. It is also proved that if, for some $n$, the nonsoluble length of 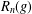$R_{n}(g)$ is equal to $k$, then $g$ belongs to a normal subgroup whose nonsoluble length is bounded in terms of $k$ and $m$. Earlier, similar generalizations of Baer’s theorem (which states that an Engel element of a finite group belongs to the Fitting subgroup) were obtained by the first two authors in terms of left Engel-type subgroups.

We improve some results in our paper [A. Languasco and A. Zaccagnini, ‘Short intervals asymptotic formulae for binary problems with prime powers’, J. Théor. Nombres Bordeaux30 (2018) 609–635] about the asymptotic formulae in short intervals for the average number of representations of integers of the forms $n=p_{1}^{\ell _{1}}+p_{2}^{\ell _{2}}$ and $n=p^{\ell _{1}}+m^{\ell _{2}}$, where $\ell _{1},\ell _{2}\geq 2$ are fixed integers, $p,p_{1},p_{2}$ are prime numbers and $m$ is an integer. We also remark that the techniques here used let us prove that a suitable asymptotic formula for the average number of representations of integers $n=\sum _{i=1}^{s}p_{i}^{\ell }$, where $s$, $\ell$ are two integers such that $2\leq s\leq \ell -1$, $\ell \geq 3$ and $p_{i}$, $i=1,\ldots ,s$, are prime numbers, holds in short intervals.

Vanishing cycles, introduced over half a century ago, are a fundamental tool for studying the topology of complex hypersurface singularity germs, as well as the change in topology of a degenerating family of projective manifolds. More recently, vanishing cycles have found deep applications in enumerative geometry, representation theory, applied algebraic geometry, birational geometry, etc. In this survey, we introduce vanishing cycles from a topological perspective and discuss some of their applications.

In this article, we establish a Lyapunov-type inequality for the following extremal Pucci’s equation:

$$\begin{eqnarray}\left\{\begin{array}{@{}ll@{}}{\mathcal{M}}_{\unicode[STIX]{x1D706},\unicode[STIX]{x1D6EC}}^{+}(D^{2}u)+b(x)|Du|+a(x)u=0 & \text{in}~\unicode[STIX]{x1D6FA},\\ u=0 & \text{on}~\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA},\end{array}\right.\end{eqnarray}$$

$\unicode[STIX]{x1D6FA}$

$\mathbb{R}^{N}$

$N\geq 2$