We single out a large class of groups 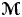${\rm {\boldsymbol {\mathscr {M}}}}$ for which the following unique prime factorization result holds: if $\Gamma _1,\ldots,\Gamma _n\in {\rm {\boldsymbol {\mathscr {M}}}}$ and $\Gamma _1\times \cdots \times \Gamma _n$ is measure equivalent to a product $\Lambda _1\times \cdots \times \Lambda _m$ of infinite icc groups, then $n \ge m$, and if $n = m$, then, after permutation of the indices, 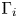$\Gamma _i$ is measure equivalent to 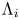$\Lambda _i$, for all 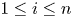$1\leq i\leq n$. This provides an analogue of Monod and Shalom's theorem [Orbit equivalence rigidity and bounded cohomology, Ann. of Math. 164 (2006), 825–878] for groups that belong to 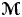${\rm {\boldsymbol {\mathscr {M}}}}$. Class ${\rm {\boldsymbol {\mathscr {M}}}}$ is constructed using groups whose von Neumann algebras admit an s-malleable deformation in the sense of Sorin Popa and it contains all icc non-amenable groups $\Gamma$ for which either (i) $\Gamma$ is an arbitrary wreath product group with amenable base or (ii) 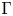$\Gamma$ admits an unbounded 1-cocycle into its left regular representation. Consequently, we derive several orbit equivalence rigidity results for actions of product groups that belong to 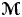${\rm {\boldsymbol {\mathscr {M}}}}$. Finally, for groups $\Gamma$ satisfying condition (ii), we show that all embeddings of group von Neumann algebras of non-amenable inner amenable groups into 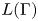$L(\Gamma )$ are ‘rigid’. In particular, we provide an alternative solution to a question of Popa that was recently answered by Ding, Kunnawalkam Elayavalli, and Peterson [Properly Proximal von Neumann Algebras, Preprint (2022), arXiv:2204.00517].

For an unramified reductive group, we determine the connected components of affine Deligne–Lusztig varieties in the affine flag variety. Based on work of Hamacher, Kim, and Zhou, this result allows us to verify, in the unramified group case, the He–Rapoport axioms, the almost product structure of Newton strata, and the precise description of isogeny classes predicted by the Langlands–Rapoport conjecture, for the Kisin–Pappas integral models of Shimura varieties of Hodge type with parahoric level structure.

Let $\textbf {G}$ be a simply connected semisimple algebraic group over a field of characteristic greater than the Coxeter number. We construct a monoidal action of the diagrammatic Hecke category on the principal block $\operatorname {Rep}_0(\textbf {G})$ of $\operatorname {Rep}(\textbf {G})$ by wall-crossing functors. This action was conjectured to exist by Riche and Williamson. Our method uses constructible sheaves and relies on Smith–Treumann theory.

Given a connected graph $H$ which is not a star, we show that the number of copies of $H$ in a dense uniformly random regular graph is asymptotically Gaussian, which was not known even for $H$ being a triangle. This addresses a question of McKay from the 2010 International Congress of Mathematicians. In fact, we prove that the behavior of the variance of the number of copies of $H$ depends in a delicate manner on the occurrence and number of cycles of $3,4,5$ edges as well as paths of $3$ edges in $H$. More generally, we provide control of the asymptotic distribution of certain statistics of bounded degree which are invariant under vertex permutations, including moments of the spectrum of a random regular graph. Our techniques are based on combining complex-analytic methods due to McKay and Wormald used to enumerate regular graphs with the notion of graph factors developed by Janson in the context of studying subgraph counts in $\mathbb {G}(n,p)$.

We classify fibrations of abstract $3$-regular GKM graphs over $2$-regular ones, and show that all fibrations satisfying the known necessary conditions for realizability are, in fact, realized as the projectivization of equivariant complex rank-$2$ vector bundles over quasitoric $4$-manifolds or $S^4$. We investigate the existence of invariant (stable) almost complex, symplectic, and Kähler structures on the total space. In this way, we obtain infinitely many Kähler manifolds with Hamiltonian non-Kähler actions in dimension $6$ with prescribed one-skeleton, in particular with a prescribed number of isolated fixed points.

We study the spaces of twisted conformal blocks attached to a $\Gamma$-curve $\Sigma$ with marked $\Gamma$-orbits and an action of $\Gamma$ on a simple Lie algebra $\mathfrak {g}$, where $\Gamma$ is a finite group. We prove that if $\Gamma$ stabilizes a Borel subalgebra of $\mathfrak {g}$, then the propagation theorem and factorization theorem hold. We endow a flat projective connection on the sheaf of twisted conformal blocks attached to a smooth family of pointed $\Gamma$-curves; in particular, it is locally free. We also prove that the sheaf of twisted conformal blocks on the stable compactification of Hurwitz stack is locally free. Let $\mathscr {G}$ be the parahoric Bruhat–Tits group scheme on the quotient curve $\Sigma /\Gamma$ obtained via the $\Gamma$-invariance of Weil restriction associated to $\Sigma$ and the simply connected simple algebraic group $G$ with Lie algebra $\mathfrak {g}$. We prove that the space of twisted conformal blocks can be identified with the space of generalized theta functions on the moduli stack of quasi-parabolic $\mathscr {G}$-torsors on $\Sigma /\Gamma$ when the level $c$ is divisible by $|\Gamma |$ (establishing a conjecture due to Pappas and Rapoport).