2.1. Simulation Condition

A prepulse of a heating laser is high intense (e.g., 10¹¹ Wcm⁻²) enough to create the plasma as the intensity of a main pulse is extremely high (e.g., 10¹⁹ Wcm⁻²) even if the contrast ratio is 10⁸ (Sunahara et al., Reference Sunahara, Johzaki, Nagatomo and Mima2012). Therefore, generation of a preformed plasma is unavoidable although some efforts are expended to avoid the preformed plasma generation (Sunahara et al., Reference Sunahara, Johzaki, Nagatomo and Mima2012; Sakagami et al., Reference Sakagami, Sunahara, Johzaki and Nagatomo2011).

Figure 1 shows (a) laser temporal profiles in four simulated cases and (b) two-dimensional profile of electron density of the target plasma. The gold-cone tip after prepulse irradiation is introduced as 36 μm thickness in x direction, 20 n _cr, real mass and Z = 40 plasma with preformed gold plasma, which has exponential profile of the scale length L = 4 μm with the density from 0.1to 20n _cr, where n _cr is the critical density. The density profile in y direction is uniform and the width of the target is 16 μm. Laser beam irradiates the target at normal incidence and its spatial beam profile in y direction is uniform. To investigate effects of laser temporal profile on fast electron generation, we use four different temporal profiles that are combination of two maximum intensities (1.0 × 10²⁰ and 2.5 × 10¹⁹ Wcm⁻²) and two pulse shapes (flattop and gaussian) as summarized in Table 1. Pulse lengths are adjusted to preserve the laser energy. The intensity in the case of the flattop pulses rises up as a gaussian with half-width at half maximum (HWHM) of 4.7 fs, is kept constant with its corresponding maximum intensity during 90 or 390 fs, and falls down as the gaussian with same HWHM. The former is called FH (flattop of high-intensity) and the latter FL (Flattop of Low-intensity). In other two cases, the intensity profile is gaussian with HWHM of 47 or 188 fs and the first is called GH (Gaussian of high-intensity) and the second GL (gaussian of low-intensity). Boundary conditions in x direction are reflection for particles and perfect absorption for electromagnetic waves. In y direction, periodic boundary conditions are applied for both particles and electromagnetic waves. Under these conditions, simulations are performed for 2000 fs and fast electrons that pass through the vertical line 5 μm behind the boundary between the preplasma and the flat profile plasma are observed. To ignore the circulation of fast electrons, fast electrons are artificially cooled down to initial temperature in the rear part, 6 μm from the rear edge of the plasma.

Fig. 1. (Color online) (a) Laser temporal profiles in four simulated cases and (b) two-dimensional profile of electron density of the target plasma, where blue line indicates the line for observation and blue shade in rear plasma represents artificially cooling region.

Table 1. Maximum intensities and temporal shapes of laser

2.2. Fast electron characteristics: Energy spectra

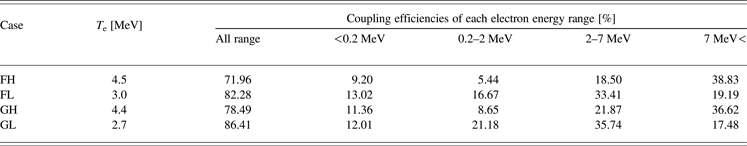
Time-integrated energy spectra of fast electrons for the four cases are shown in Figure 2. In both pulse shapes, low-energetic electrons (<7 MeV) decrease, and high-energetic electrons (>7 MeV) are more generated as the laser intensity becomes high. According to some scaling laws (Wilks et al., Reference Wilks, Kruer, Tabak and Langdon1992; Beg et al., Reference Beg, Bell, Dangor, Danson, Fews and Glinsky1997; Pukhov et al., Reference Pukhov, Sheng and Meyer-Ter-Vehn1999; Haines et al., Reference Haines, Wei, Beg and Stephens2009), the slope temperature of generated electrons is approximately proportional to the value between the square root and cube root of the laser intensity. Slope temperatures between 5 and 20 MeV in the four cases are summarized in Table 2. Ratios between the temperatures of high- and low-intensity lasers in the flattop and gaussian cases T _H/T _L are 1.5 and 1.63, respectively, and they approximately agree with that in scaling law of the cube root, namely (I _H/I _L)^1/3 = 1.59. Energy coupling efficiencies from the laser to observed electrons with energies of all range, less than 0.2, 0.2–2, 2–7 MeV, and more than 7 MeV are calculated and summarized in Table 2. Though the difference on coupling efficiencies to whole observed electrons among all cases is approximately 20% at most, there is not so much difference compared to that at range of each energy. Therefore, if the scaling law is applied to each cases, low-energy electrons are less generated, the number of high-energy electrons increases in the high-intensity case compared to the low-intensity case, and intersection points of electron energy spectra probably appear as shown in Figure 2. In the scaling law, generated fast electrons have the effective slope temperature, so, it is assumed that energy distribution function of these electrons is expressed by following equation:

Fig. 2. (Color online) Time-integrated energy spectra of fast electrons, where red and green lines mean high- and low-intensities and dashed and solid lines indicate flattop and gaussian cases, respectively.

Table 2. Slope temperatures and coupling efficiencies from laser to observed electrons with energies of all range, less than 0.2, 0.2–2, 2–7 MeV, and more than 7 MeV

(1)$$\,f \lpar E\rpar = A\; {\rm exp} \left({ - \displaystyle{E \over T}} \right)\comma \;$$

where A and T are normalized coefficient and the effective temperature, respectively. Total energy of these electrons E _tot is given by

(2)$$E_{\rm tot}=\int_0^\infty \, E f \lpar E\rpar {\rm d} E.$$

When it is assumed that total energies are the same on two cases namely case 1 and 2, the energy at the intersection point of two equations, E _1,2 is given by

(3)$$E_{1\comma 2}=\displaystyle{{T_1 T_2 } \over {T_1 - T_2 }} {\rm log} \left({\displaystyle{{T_1^2 } \over {T_2^2 }}} \right).$$

Then, we can derive intersection points among all cases using effective temperatures given in Table 2, i.e., E _FH,FL, E _GH,GL = 7.0, 6.8 MeV. Assuming same coupling efficiencies, calculated intersection points appear near 7 MeV in the scope of simulated intensities. Even if it takes different coupling efficiencies in consideration, only the term η₂/η₁ is multiplied to inside the logarithm in Eq. (3), where η is the coupling efficiency from the laser to electrons, and intersection points also appear near the 7 MeV. Therefore, energy spectra of generated fast electrons are approximately obtained using the scaling law if the coupling efficiencies from the laser to electrons are given.

When the cases of same maximum intensities are compared in Figure 2, the generation of high energy electrons is narrowly enhanced and that of low-energy electrons is barely weakened in the case of flattop compared to the gaussian case because the lower intensity laser in the gaussian case irradiates longer than that in the flattop case. The difference of 0.2–2 MeV electrons in Table 2 is especially large compared to others and these electrons efficiently heat the core. Therefore, the GL case is clearly the most suitable for fast ignition in those parameters when laser energy is same.

2.3. Fast electron characteristics: Divergence angle

It is important for a evaluation of core heating to investigate the divergence angle of fast electrons. Electron angular distribution is composed from observed electron data and HWHMs of the angular distribution in both cases are calculated and shown as afunction of electron energy in Figure 3. In both cases, the divergence angle in the high-intensity case is larger than that of the low-intensity case because high intensity laser generates large electron currents, they induce large magnetic fields, and the strong scattering of electrons occurs. Figure 4 shows time evolutions of maximum magnetic field in the cases of flattop and gaussian. Strong magnetic fields that easily bend the path of MeV electrons are observed in both high- and low-intensity cases. Although these magnetic fields scatter generated fast electrons, high energetic fast electrons are not affected so much because of the high energy. On the other hand, low-energetic electrons are enough to be affected and their divergence angles are similar in all cases, therefore, the divergence angle of 0–2 MeV electrons are almost the same among all cases. Furthermore, initially generated electrons has small divergence angle because of no magnetic fields. Therefore, divergence angle in the case of flattop becomes small compared to the Gaussian case because many electrons are initially generated.

Fig. 3. (Color online) HWHMs of angle as a function of electron energy in the cases of (a) flattop and (b) gaussian, where red and green lines indicate high- and low-intensities and dashed and solid lines indicate flattop and gaussian, respectively.

Fig. 4. (Color online) Time evolutions of maximum magnetic field inside the plasma in the cases of (a) flattop and (b) gaussian, where red and green lines indicate high- and low-intensities and dashed and solid lines indicate flattop and gaussian, respectively.

Consequently, divergence angles of high-energetic fast electrons vary with the laser intensity, however those of low-energetic fast electrons are similar in spite of the laser intensity because of large magnetic fields. From the standpoint of the fast ignition, there is no difference because the divergence angles of 0–2 MeV electrons that efficiently heat the core are almost the same, however, it is serious problem that the divergence angle is much large in whole simulated cases.

3.1. Simulation Condition

Figure 5 shows spatial profiles of laser in two simulated cases and two-dimensional profile of electron density of target plasma. Target conditions are similar to previous simulations, but some parameters are different. The thickness (in x direction) of the gold-cone tip is 15 μm because of the reduction of simulation time. The width (in y direction) of the target plasma is set to 70 μm that is more than three times as large as laser spot sizes. The temporal profile of the laser beam is flattop and the pulse length is semi-infinite. We use two spatial profiles, namely gaussian with the spot diameter of 10 μm and the intensity of 1 × 10²⁰ Wcm⁻² and gaussian with 20 μm and 5 × 10¹⁹ Wcm⁻² as shown in Figure 5. Spot diameters are adjusted to preserve the total laser energy. Each profile is named G10 and G20, respectively. Boundary conditions are reflection for particles and perfect absorption for electromagnetic waves in both x and y directions. As shown in Figure 5b, cooling region (blue shade) for avoiding the circulation of fast electrons are set in the regions of 10 < x < 15 and −30 < y < 30 μm, 0 < x < 10 and 30 < y < 35 μm, and 0 < x < 10 and −35 < y < −30 μm, where x = 0 μm is defined at boundary between the preformed plasma and the gold-cone tip and y = 0 μm at the center of the target. Under these conditions, simulations are performed for 200 fs and passing fast electrons are observed at the area of x = 5 μm and y = 0–25 μm.

Fig. 5. (Color online) Spatial profiles of laser in two simulated cases and two-dimensional profile of electron density of target plasma, where blue arrow indicate the line for observation and blue shade in rear plasma represents artificially cooling region.

3.2. Fast electron characteristics: Beam intensity

Figure 6 shows time-evolutions of the electron beam intensity at y = (a) 0–5, (b) 10–15, and (c) 20–25 μm. In both cases of G10 and G20, the electron beam intensity is high near the laser axis and becomes low as the laser intensity at the area for observation is lower. At y = 0–5 μm, the electron beam intensity in the G10 case exceeds that in the G20 case because the laser intensity of the G10 case at this region is higher than that of the G20 case as shown in Figure 5. At y = 20–25 μm, the electron beam intensity in the G20 case is higher than that in the G10 case, because the relationship of the laser intensity is reversed. However the difference of the electron beam intensity is not large compared to that of the laser intensity as most of electrons are come from the region where the laser intensity is high. In the G10 case, the time at which the electron beam intensity rises is later than that in the G20 case. It implies that observed electrons are come from another region, namely near the laser axis. At y = 10–15 μm, there are not much differences on time evolutions of the electron beam intensity and the rising time in the G10 case is also later than that in the G20 case. Consequently, electron beam intensities normally reflect the laser intensity in each area although the contribution from another area is not negligible.

Fig. 6. (Color online) Time-evolutions of the electron beam intensity at y = (a) 0–5, (b) 10–15, and (c) 20–25 μm, where red and green lines indicate G10 and G20 cases, respectively.

3.3. Fast electron characteristics: Energy spectra

Figure 7 shows time-integrated electron energy spectra in the G10 and G20 cases at y = (a) 0–5, (b) 10–15, (c) 20–25, and (d) 0–25 μm. At each area, the slope temperature in the G10 case is higher than that in the G20 case. As the observed area is far from the laser axis, number of fast electrons decreases. Its variation in the G10 case is the larger than that in the G20 case because the laser intensity in the G10 case is rapidly damped with the distance from the laser axis compared to the G20 case. At y = 0–25 μm, low-energetic electrons (<6.5 MeV) decrease and high-energetic electrons (>6.5 MeV) are more generated as the laser intensity becomes high. In Figure 7d, slope temperatures in the cases of G10 and G20 are 6.0 and 3.7 MeV, respectively. The estimated slope temperatures are between 1.5 and 15 MeV. The ratio of two slope temperature is 1.62 and it agree with scaling law of the cube root. If we pay attention to energy spectra of generated fast electrons, the G20 case is suitable for fast ignition because low-energetic electrons are much generated.

Fig. 7. (Color online) Time-integrated electron energy spectra at y = (a) 0–5, (b) 10–15, (c) 20–25, and (d) 0–25 μm, where red and green lines indicate G10 and G20 cases, respectively.

3.4. Fast electron characteristics: Divergence angle

To evaluate divergence angle, HWHMs of the angular distribution that is composed from observed electron data at y = 0–25 μm are calculated and shown as a function of electron energy in Figure 8. In both cases, HWHMs of high-energetic electrons (>10 MeV) are small compared to those of low-energetic electrons (<10 MeV). HWHMs in the G10 case are larger than those in the G20 case. Green et al. (Reference Green, Ovchinnikov, Evans, Akli, Azechi and Beg2008) reported that effects of focal spot are small and the divergence angle strongly depends on the laser intensity in 2008. Furthermore, according to Two dimensional PIC simulations in that research, divergence angles of whole observed electrons for different intensities of 1 × 10²⁰ and 2 × 10¹⁹ Wcm⁻² in the case of 16 μm focal spot diameter are 35 and 45, respectively. Our results are consistent with that research although we vary both parameters of focal spot diameter and laser intensity simultaneously because of the same laser energy. These results show that the G20 case is more suitable for fast ignition because of smaller divergence angle.

Fig. 8. (Color online) HWHMs of the angular distribution, where red and green lines indicate G10 and G20 cases, respectively.