Introduction
Since the middle of the 19th Century, the hydrothermal veins hosted within the metamorphic rocks belonging to the Apuan Alps Metamorphic Complex, northern Apennines, Italy, have been known for the occurrence of many thioantimonite and thioarsenite mineral species (the so-called ‘sulfosalts’, with trivalent Sb or As, respectively – Moëlo et al., Reference Moëlo, Makovicky, Mozgova, Jambor, Cook, Pring, Paar, Nickel, Graeser, Karup-Møller, Balić-Žunić, Mumme, Vurro, Topa, Bindi, Bente and Shimizu2008). On the contrary, antimonites are very rare and one of the few species so far identified is derbylite, reported from the Buca della Vena mine since the beginning of the 1980s (Mellini et al., Reference Mellini, Orlandi and Perchiazzi1983). Derbylite, ideally Fe3+4Ti4+3Sb3+O13(OH), was first found by Hussak and Prior (Reference Hussak and Prior1895) in the cinnabar-bearing gravels of the fazenda Três Cruzes, Tripuí (formerly Tripuhy), Ouro Preto, Minas Gerais, Brazil, and described by the same authors as a new mineral species two years later (Hussak and Prior, Reference Hussak and Prior1897). Its crystal structure was solved by Moore and Araki (Reference Moore and Araki1976) in the space group P21/m down to R 1 = 0.045. Mellini et al. (Reference Mellini, Orlandi and Perchiazzi1983) provided modern chemical data, suggesting the occurrence of the substitution mechanism 2Fe3+ = Fe2+ + Ti4+, and revising the chemical formula of derbylite to Fex2+Fe3+4–2xTi4+3+xSb3+O13(OH). Later, Mellini et al. (Reference Mellini, Orlandi and Vezzalini1986) discussed the occurrence of V3+ replacing Fe3+ in other samples from the Buca della Vena mine.
The mineralogical studies carried out since the end of the 2000s on the mineral assemblages from the Monte Arsiccio mine, a locality just a few km south-west with respect to Buca della Vena, in a similar geological setting, allowed an additional identification of derbylite from the Apuan Alps and a new finding of its arsenite analogue, graeserite, ideally Fe3+4Ti4+3As3+O13(OH). This latter mineral was first described by Krzemnicki and Reusser (1998) from the Binn Valley, Switzerland, and its crystal structure was solved by Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998). Graeserite is homeotypic with derbylite, showing a different space group symmetry, i.e., C2/m (assuming the standard setting).
The finding of derbylite and graeserite at the Monte Arsiccio mine has allowed us to refine the knowledge of the crystal-chemistry of these unusual Sb and As oxysalts, merging the chemical and structural data with Mössbauer spectroscopic information. In this paper, the results of this study are reported.
Experimental
Samples studied
Specimens of derbylite and graeserite were collected at the Sant'Olga and Sant'Anna levels of the Monte Arsiccio mine. In the former, derbylite (samples A–D) and graeserite (samples E–F) occur in veins hosted within pyrite- and magnetite-rich metadolostone, along with other rare minerals; in the latter, only graeserite was found (sample G), as porphyroblasts within schist. The samples studied (Fig. 1) are representative of some different mineral assemblages or morphologies shown by derbylite and graeserite from this locality. A description of the samples studied and the mineral association is given in Table 1. Sample A contains another antimonite, stibivanite, ideally V4+Sb3+2O5, which also occurs in sample F where it is associated with the new diarsenite mineral bianchiniite, ideally Ba2(TiV)(As2O5)2OF (Biagioni et al., Reference Biagioni, Pasero, Hålenius and Bosi2019).

Fig. 1. Selected samples of derbylite and graeserite from the Monte Arsiccio mine. (a) Black prismatic crystal of derbylite, deeply striated, with siderite, baryte and ‘hyalophane’. (b) Black acicular crystal of derbylite with magnetite, siderite, ‘hyalophane’ and quartz. (c) Aggregate of prismatic crystals of graeserite grown on ankerite. (d) Twinned crystals of graeserite with ‘hyalophane’, dolomite and siderite.
Table 1. Studied samples of derbylite (A–D) and graeserite (E–G).

Chemical data
Quantitative chemical data were collected on derbylite and graeserite samples using a Superprobe JEOL JXA 8200 electron microprobe at the “Eugen F. Stumpfl” laboratory, Leoben University, Austria. The following analytical conditions were used: wavelength-dispersive spectroscopy mode, accelerating voltage = 20 kV, beam current = 10 nA and beam size = 1 μm. The following standards (element, emission line) were used: rutile (TiKα), synthetic vanadium (VKα), chromite (CrKα), magnetite (FeKα), skutterudite (AsLα), stibnite (SbLα), baryte (BaLα) and galena (PbMα). The following diffracting crystals were selected: LIFH for Ti, V, Cr and Fe; TAP for As; PETJ for Ba; and PETH for Sb and Pb. Fluorine was sought but was found to be below the detection limit. The ZAF routine was applied for the correction of the raw data. Counting times were 20 s for peak and 10 s for left and right backgrounds. Chemical data are given in Table 2.
Table 2. Chemical composition (in wt.%) and chemical formulae (in atoms per formula unit, apfu, on the basis of Ti + V + Cr + Fe = 7 apfu) of derbylite (A–D) and graeserite (E–G) samples from the Monte Arsiccio mine.

n = Number of spot analyses; n.a. = not analysed.
Sample A: Fe2+0.67(Fe3+2.32V0.29Cr0.04)Ti3.67[(Sb0.70As0.26)(Ba0.02Pb0.01)]O12.94(OH)1.06
Sample B: Fe2+0.65(Fe3+2.53V0.16)Ti3.65[(Sb0.80As0.18)]O12.94(OH)1.06
Sample C: Fe2+0.67(Fe3+2.41V0.23Cr0.02)Ti3.67[(Sb0.76As0.21)(Ba0.04)]O13.00(OH)1.00
Sample D: Fe2+0.73(Fe3+2.30V0.21Cr0.03)Ti3.73[(Sb0.77As0.21)(Ba0.03Pb0.01)]O13.03(OH)0.97
Sample E: Fe2+0.52(Fe3+2.24V0.66Cr0.06)Ti3.52[(As0.64Sb0.29)(Ba0.25Pb0.01)]O13.27(OH)0.73
Sample F: Fe2+0.68(Fe3+2.03V0.57Cr0.05)Ti3.68[(As0.60Sb0.36)(Ba0.19Pb0.01)]O13.27(OH)0.73
Sample G: Fe2+0.62(Fe3+2.72V0.03)Ti3.62[(As0.87Sb0.07)(Ba0.19Pb0.08)]O13.38(OH)0.62
Mössbauer spectroscopy
The 57Fe Mössbauer spectra of two selected derbylite and graeserite samples (samples C and F, respectively, for which sufficient homogeneous material was available) were collected at room temperature in transmission mode using a 57Co (in Rh matrix) point source, with a nominal activity of 0.40 GBq (Swedish Museum of Natural History, Stockholm, Sweden). Absorbers were prepared by gently grinding sample material (in the range 0.1–0.2 mg), which was placed in a ~2 mm × 2 mm square on a strip of tape and positioned closely in front of the point source. The Mössbauer spectra were acquired over the velocity range ± 4 mm/s and were calibrated against α-Fe foil. The spectra, shown in Fig. 2, were fitted using the program MossA (Prescher et al., Reference Prescher, McCammon and Dubrovinsky2012). Hyperfine parameters are given in Table 3.

Fig. 2. Mössbauer spectra of derbylite (upper) and graeserite (lower).
Table 3. Hyperfine parameters for fitted doublets in the Mössbauer spectra of derbylite (sample C) and graeserite (sample F).

Uncertainties within brackets. χ 2 = 1.43 and 1.92 for derbylite and graeserite, respectively.
CS – Centre shift; QS –quadrupole splitting; FWHM – linewidth for full width at half maximum; Int – intensity.
Crystallography
Single-crystal X-ray diffraction data were collected on derbylite (sample C) and graeserite (samples F and G) using a Bruker Smart Breeze diffractometer equipped with an air-cooled Photon II CCD detector, with graphite-monochromatised MoKα radiation. The detector-to-crystal distance was set at 50 mm. Data were corrected for Lorentz-polarisation factors and absorption using the Apex3 package of software (Bruker AXS Inc., 2016).
Derbylite is monoclinic, space group P21/m, with unit-cell parameters a = 7.1690(3), b = 14.3515(7), c = 4.9867(2) Å, β = 104.820(3)° and V = 495.99(4) Å3. These values can be compared with those reported by Moore and Araki (Reference Moore and Araki1976), i.e. a = 7.160(1), b = 14.347(3), c = 4.970(1) Å, β = 104.61(2)° and V = 494.0(2) Å3; and Mellini et al. (Reference Mellini, Orlandi and Perchiazzi1983), i.e., a = 7.156(2), b = 14.354(4), c = 4.980(1) Å, β = 104.69(2)° and V = 494.8(2) Å3.
Unit-cell parameters of the two samples of graeserite are: a = 5.0225(7), b = 14.3114(18), c = 7.1743(9) Å, β = 104.878(3)° and V = 498.39(11) Å3 (sample F); and a = 5.0275(4), b = 14.2668(11), c = 7.1663(5) Å, β = 105.123(4)° and V = 496.21(7) Å3 (sample G). These values can be compared with those reported by Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998): a = 5.0053(4), b = 14.272(1), c = 7.1736(6) Å, β = 105.165(2)° and V = 494.61(7) Å3. The examination of systematic absences confirmed the space group C2/m for graeserite samples.
The crystal structures of derbylite and graeserite were refined using Shelxl-2018 (Sheldrick, Reference Sheldrick2015) starting from the atomic coordinates given by Moore and Araki (Reference Moore and Araki1976) and Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998) for derbylite and graeserite, respectively. Scattering curves for neutral atoms were taken from the International Tables for Crystallography (Wilson, Reference Wilson1992).
The first step of refinement of derbylite converged to R 1 = 17.8%, showing a high electron density residual corresponding to another position of Sb. After its addition, the R 1 dropped to 8.4%. The site scattering of the two Sb positions have been refined using the Sb vs. As scattering curves at the Sb(a) and Sb(b) sites, respectively, in order to take into account the occurrence of both Sb and As, as indicated by electron microprobe data, and constraining the sum of Sb and As to be 1 atom per formula unit (apfu). The site scattering at the four independent octahedral M(1–4) sites was refined using the Ti vs. Fe scattering curves. Actually, Moore and Araki (Reference Moore and Araki1976) refined the M(1) site as a pure Ti position. On the contrary, the relatively low U eq value suggested the occurrence of a heavier atom replacing Ti. Therefore, the refinement was improved to R 1 = 5.6%, lowered to 5.1% assuming an anisotropic model for both cations and anions. At this stage of refinement, a relatively high maximum close to (0, ¼, ¼) was observed. Adding this residual, interpreted as a partially occupied Ba position, the refinement converged to R 1 = 3.52% for 1955 reflections with F o > 4σ(F o) and 116 refined parameters.
The refinement of sample F of graeserite rapidly converged to R 1 = 8.6%, confirming the correctness of the structural model. The site scattering of the three independent M sites was freely refined using the scattering curves of Ti vs. Fe. Whereas M(1) was found fully occupied by Ti only, the M(2) and M(3) sites have a mixed (Ti,Fe) occupancy. The scattering curves of As vs. Sb were used for the As site, whereas the site occupancy at the Ba site was freely refined. The anisotropic model (with the only exception being represented by the Ba site that was refined isotropically) converged to R 1 = 4.04%. In the last stage of the refinement, the occurrence of an electron density residual close to the As site suggested a possible splitting of this position between As- and Sb-centred sites. The refinement finally converged to R 1 = 3.99% for 428 reflections with F o > 4σ(F o) and 68 refined parameters.
During the first step of the refinement of the crystal structure of sample G, the additional Ba position was removed. The refinement converged to a high R 1 value of 24.8%, showing a high maximum at coordinates (–½, ½, 0). Adding this maximum [labelled Ba(1)], the refinement converged to R 1 = 8.2%, with the occurrence of another significant residual close to Ba(1). The addition of this further maximum [initially labelled Ba(2)] lowered the R 1 value to 5.2%. The same site-scattering curves used for sample F were used. All three M sites revealed a mixed (Ti,Fe) occupancy. The structural model was refined anisotropically (except for the Ba sites) down to R 1 = 2.50%. Finally, the site occupancy of Ba(2) was modelled using the scattering curve of Pb vs. □, taking into account the asymmetric coordination of this atom, probably related to the lone-electron-pair activity of Pb2+; for this reason the site was relabelled as Pb(2). This improved the R 1 to a final value of 2.37% for 1081 reflections with F o > 4σ(F o) and 69 refined parameters.
Details of the intensity data collections and crystal structure refinements are given in Table 4. The crystallographic information files have been deposited with the Principal Editor of Mineralogical Magazine and are available as Supplementary material (see below).
Table 4. Crystal data and summary of parameters describing data collection and refinement for derbylite and graeserite.

Results and discussion
Chemical formula of derbylite and graeserite
Moore and Araki (Reference Moore and Araki1976) proposed the normalisation of the chemical formula of derbylite based on 27 negative charges (i.e. 13 O2– and one OH–) and eight cations. However, the occurrence of other elements like Ba and Pb poses some questions about the actual normalisation factor. Grey et al. (Reference Grey, Madsen and Harris1987) and Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998) showed that Ba and Pb occur in cuboctahedral cavities, in agreement with their larger ionic radii in six-fold coordination (1.35 and 1.19 Å, respectively – Shannon, Reference Shannon1976) compared with those of Fe2+ and Ti4+ (0.78 and 0.605 Å, respectively – Shannon, Reference Shannon1976).
Tomichite, (V,Fe)3+4Ti4+3As3+O13(OH), is an arsenite close to graeserite, which can contain minor Ba (Nickel and Grey, Reference Nickel and Grey1979; Grey et al., Reference Grey, Madsen and Harris1987). According to Grey et al. (Reference Grey, Madsen and Harris1987) the increasing Ba content in Ba-rich tomichite is coupled with the replacement of Ti4+ by (V,Fe)3+, probably according to the substitution 2Ti4+ + □ = 2(V,Fe)3+ + Ba2+. Grey et al. (Reference Grey, Madsen and Harris1987) adjusted the Fe2+/Fe3+ ratio in order to achieve the electrostatic balance, without any direct measurement of this ratio. Their chemical data apparently fit with this substitution mechanism, showing quite different Fe2+/Fe3+ ratios among the three samples studied. On this basis, they proposed the end-member composition Ba0.5(As2)0.5Ti2(V,Fe)5O13(OH) for Ba-rich tomichite. The relationships between Ti4+ and (Ba+Pb) apfu in the samples from Monte Arsiccio are shown in Fig. 3a. The samples with lowest (Ba+Pb) (derbylite samples) are relatively enriched in Ti4+, whereas the (Ba,Pb)-enriched compositions (graeserite samples) are slightly depleted in Ti4+. However, there is not a clear linear relationship between (Ba+Pb) and Ti apfu. According to Mellini et al. (Reference Mellini, Orlandi and Perchiazzi1983), the Ti content is also coupled with the Fe2+ content.

Fig. 3. Chemical variability in derbylite (light blue diamonds) and graeserite (red triangles) samples from the Monte Arsiccio mine.
Table 3 gives the results of Mössbauer spectroscopy performed on two selected samples of derbylite (sample C) and graeserite (sample F) from Monte Arsiccio. No significant differences between the two samples were observed as regards the Fe2+/Fe3+ atomic ratios, i.e. 0.19/0.81 in derbylite and 0.21/0.79 in graeserite. Within experimental uncertainties, the two samples have the same Fe2+/Fe3+ atomic ratio. Notwithstanding different Ba contents, the total amount of Ti is very similar (3.67 and 3.68 apfu for samples C and F, respectively), as well as the sum of trivalent metals (2.63 and 2.65 apfu for samples C and F, respectively). This seems to disagree with the hypothesis of Grey et al. (Reference Grey, Madsen and Harris1987). Moreover, the negative relation between ∑(Fe,V,Cr) vs. Ti occur in both derbylite and graeserite, as shown in Fig. 3b.
Barium and Pb are correlated positively with the As3+ content (Fig. 3c). This may be surprising, as Ba and As occupy the same cuboctahedral cavities. However, as discussed by Grey et al. (Reference Grey, Madsen and Harris1987) and Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998), the occurrence of Ba and Pb within these cavities seems to be coupled with the occurrence of two AsO3 groups within other cavities. Indeed, whereas derbylite has a very low Ba and Pb content, owing to the larger size of Sb, that does not allow the accommodation of two SbO3 groups within a cuboctahedral cavity, As minerals related to derbylite (e.g. graeserite and tomichite) show larger contents of divalent large-radius cations like Ba and Pb and the sum of (As+Sb) with (Ba+Pb) exceeds 1 apfu.
Arsenic and Sb are correlated negatively (Fig. 3d). Minor vacancies at the (As/Sb) sites have been observed both in derbylite and graeserite. Usually, they are up to 0.06 apfu. If they are real (and not the result of experimental uncertainties), they could be balanced through the substitution (As/Sb)3+ + 3O2– = □ + 3OH–.
On the basis of these considerations, the chemical formulae of derbylite and graeserite have been recalculated on the basis of (Fe2+ + Fe3+ + V3+ + Cr + Ti) = 7 apfu, distributing Fe2+ and Fe3+ on the basis of 24 positive charges and assuming the trivalent oxidation state for V. Hydroxyl groups were calculated on the basis of electrostatic neutrality, assuming the substitution mechanism (As/Sb)3+ + O2– = (Ba/Pb)2+ + OH– and (As/Sb)3+ + 3O2– = □ + 3OH–. The results are given in Table 2 and crystal-chemical formulae are reported in the footnote of the same Table.
It is interesting to compare the results of such a calculation procedure with the Fe2+/Fetot atomic ratios measured through Mössbauer spectroscopy (Table 3). The sample of derbylite (sample C) has a Fe2+/Fetot atomic ratio of 0.19(2), to be compared with 0.22 obtained through the recalculation procedure. In graeserite (sample F), an atomic ratio of 0.21(2) was measured, to be compared with a calculated value of 0.25. The two values are within the experimental uncertainties.
Crystal structure of derbylite and graeserite
In agreement with previous authors (e.g. Berlepsch and Armbruster, Reference Berlepsch and Armbruster1998), the crystal structure of derbylite and graeserite can be described as a tunnel structure, with the tunnel walls formed by α-PbO2-type double octahedral chains and the top and the bottom by V3O5-type octahedral columns (Fig. 4). Due to the different width of the V3O5-type columns, which varies between one and two octahedra, the tunnel width expands and contracts along its direction. In this way, cuboctahedral cavities can be identified, occupied by (As/Sb) or (Ba/Pb).

Fig. 4. The octahedral framework characterising derbylite and graeserite, as seen down a (a) and its constitutive V3O5-type (b) and α-PbO2-type (c) octahedral columns. The axial setting of graeserite was used.
Derbylite
Atomic coordinates, site occupancies, and displacement parameters of derbylite are given in Table 5, whereas selected bond distances are reported in Table 6. A comparison between refined and calculated site scatterings at the cation sites, along with proposed site populations and observed and calculated average distances are given in Table 7. Table 8 reports the bond-valence sums (BVS), calculated using the site populations given in Table 7 and the bond parameters of Brese and O'Keeffe (Reference Brese and O'Keeffe1991) for all the pair cation–anion, the only exception being Sb3+, for which the bond parameters of Mills et al. (Reference Mills, Christy, Chen and Raudsepp2009) were used.
Table 5. Atomic coordinates, site occupation factors (s.o.f.) and equivalent isotropic or isotropic (*) displacement parameters (Å2) for derbylite.

Table 6. Selected bond distances (in Å) for derbylite.

Table 7. Refined site scattering vs. calculated site scattering (in electrons), proposed site population, observed and calculated average distances (Å) for derbylite.

Note: average <M–O> distances for M(1–4) and Ba sites have been calculated using the ionic radii given by Shannon (Reference Shannon1976) and the bond parameters of Brese and O'Keeffe (Reference Brese and O'Keeffe1991) and Mills et al. (Reference Mills, Christy, Chen and Raudsepp2009) for As and Sb. For the partially occupied sites, a full occupancy is assumed for calculating the ideal <M–O> distances.
Table 8. Weighted bond-valence sums (in vu) for derbylite.

Note: bond parameters after Brese and O'Keeffe (1991), Sb–O bonds after Mills et al. (2009).
The octahedral framework is formed by four independent M(1–4) sites, whereas three partially filled sites occur in the cuboctahedral cavities. The M(1) octahedron is quite regular, with average bond distance of 1.992 Å (range 1.973–1.999 Å), to be compared with 1.962 Å reported by Moore and Araki (Reference Moore and Araki1976). In addition, the difference between the longest and shortest distance, Δd, is 0.026 Å and 0.061 Å for the samples from Monte Arsiccio and that studied by Moore and Araki (Reference Moore and Araki1976), respectively. It is well-known that Ti4+-centred octahedron are usually distorted, owing to the off-centre displacement of this cation (e.g. Megaw, Reference Megaw1968). Indeed, whereas refined site scattering (Table 7) suggested the replacement of Ti4+ by Fe in the sample from Monte Arsiccio, Moore and Araki (Reference Moore and Araki1976) reported a pure Ti occupancy for their sample. This effect is more clear at the M(3) and M(4) sites, having a mixed Ti/Fe3+ occupancy, and showing Δd values of 0.285 and 0.301 Å, respectively. The lower degree of distortion shown by M(1) suggests that minor V3+ (having an atomic number Z similar to that of Ti, i.e. 23 vs. 22) may be located at this site. The M(2)-centred octahedron has a pure Fe occupancy. The average bond distance (2.052 Å), as well as the bond-valence calculations (Table 8), suggest the mixed Fe2+/Fe3+ occupancy at this site. Considering the average bond distance and the ionic radii of Fe2+ and Fe3+ in octahedral coordination, using the values given by Shannon (Reference Shannon1976), an idealised site population of (Fe3+0.65Fe2+0.35) may be proposed. The total site scattering observed at the M(1–4) sites is 169.8 electrons per formula unit (epfu), comparable with 166.6 epfu calculated on the basis of electron microprobe data.
Mössbauer spectroscopy indicated that ferric and ferrous iron are both octahedrally coordinated. The half-band widths of the fitted quadrupole doublets indicate that Fe is located in more than one polyhedron each, but that the mean M–O distances and distortions are fairly similar. This contrasts with the higher distortion degree of M(3) and M(4) sites with respect to M(1) and M(2). However, it is likely that the distortion is due to Ti4+, whereas when these sites are occupied by Fe a more regular coordination occurs.
Cuboctahedral cavities host partially filled Sb(a), Sb(b) and Ba sites. Moore and Araki (Reference Moore and Araki1976) found only a fully occupied Sb site [i.e. Sb(a)], with average bond distance of 2.007 Å. On the contrary, the refinement of the crystal structure of the sample from Monte Arsiccio suggested the occurrence of two different Sb sites, Sb(a) and Sb(b). The former is occupied preferentially with respect to the latter. As bond distances do not suggest any preferential partitioning of Sb and As within one out of the two sites, these two elements were equally partitioned among Sb(a) and Sb(b) sites. Considering the refined site scattering at the two positions, the site population Sb0.63As0.17 and Sb0.13As0.04 are proposed for Sb(a) and Sb(b), respectively. The discrepancy between observed and theoretical BVS (Table 8) is probably due to the average nature of the ligand positions, related to the statistical occupancy of Sb and As between two different positions. The Ba site is located close to the centre of the cuboctahedral cavities. It has a twelve-fold coordination, with average bond distance 2.906 Å.
Graeserite
Atomic coordinates, site occupancies, and displacement parameters of the two samples of graeserite are given in Table 9, whereas selected bond distances are reported in Table 10.
Table 9. Atomic coordinates, site occupation factors (s.o.f.), and equivalent isotropic or isotropic(*) displacement parameters (Å2) for graeserite.

Table 10. Selected bond distances (in Å) for graeserite.

The octahedral framework is formed by three independent M(1–3) sites, whereas some partially filled sites occur in the cuboctahedral cavities. The M(1) octahedron is quite regular, with average bond distance of 1.981 Å (range 1.971–1.986 Å) and 1.972 Å (range 1.955–1.980 Å) for samples F and G, respectively. These values can be compared with the average distance reported by Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998), i.e., 1.984 Å. The distortion of this site is low, and comparable to that observed in derbylite. The M(2) site is the largest among octahedral sites and it has a mixed occupancy. Table 11 reports the proposed site population based on refined site scattering, electron microprobe data, and average bond distances. In both samples, a mixed (Ti,M 3+,Fe2+) occupancy was proposed, with M 3+ = V and Fe in samples F and G, respectively. M(2) is distorted, with Δd values of 0.238 and 0.252 Å for samples F and G. This distortion is comparable with that shown by the site M(3), i.e. 0.232 and 0.213 Å for samples F and G, respectively. The BVS at the M(1–3) sites agree with the theoretical values (Table 12). The total site scattering observed at the M(1–3) sites is 158.0 and 164.4 epfu for samples F and G, to be compared with 165.4 and 167.4 epfu calculated on the basis of electron microprobe data.
Table 11. Refined site scattering vs. calculated site scattering (in electrons), proposed site population, observed and calculated average distances (Å) for graeserite.
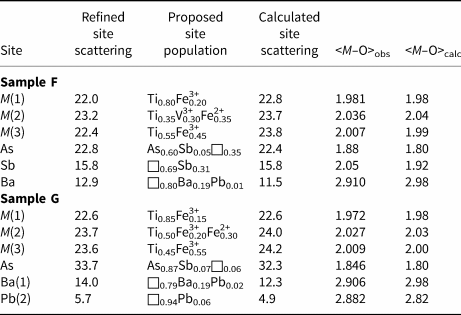
Note: average <M–O> distances for M(1–3), Ba, and Pb sites have been calculated using the ionic radii given by Shannon (Reference Shannon1976) and the bond parameters of Brese and O'Keeffe (Reference Brese and O'Keeffe1991) and Mills et al. (Reference Mills, Christy, Chen and Raudsepp2009) for As and Sb. For the partially occupied sites, a full occupancy is assumed for calculating the ideal <M–O> distances.
Table 12. Weighted bond-valence sums (in vu) for graeserite.

Note: bond parameters after Brese and O'Keeffe (1991) and S––O and Pb–O bonds after Mills et al. (2009) and Krivovichev (2012), respectively.
As in derbylite, Mössbauer spectroscopy indicated that ferric and ferrous iron are both octahedrally coordinated in graeserite. Similarly, the half-band widths of the fitted quadrupole doublets are rather high, indicating that Fe is located in more than one polyhedron each, but that the mean M–O distances and distortions are fairly similar.
Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998) reported the occurrence of an As and a Pb site within the cuboctahedral cavities of Pb-bearing graeserite. In samples F and G some differences can be observed. Sample F has significant Sb replacing As. Antimony forms longer distances with O than As and the crystal structure refinement showed the possible splitting of the As site into two sub-positions. One, more populated, has shorter distances and it is compatible with an As occupancy, with possibly a very minor replacement of Sb; the other has a lower occupancy and longer distances and it is interpreted as an Sb position. In sample G, As is strongly dominant over Sb and only one site was modelled. In both cases, the statistical nature of these positions results in longer than ideal average distances (Table 11). For instance, in sample G, having a nearly pure As occupancy, an average distance of 1.846 Å has been observed, definitely longer than the average As3+–O distance of 1.782 Å reported by Majzlan et al. (Reference Majzlan, Drahota, Filippi, Bowell, Alpers, Jamieson, Nordstrom and Majzlan2014). Longer than ideal distances are reported in other related minerals like tomichite and the arsenite–antimonite mineral hemloite, (Ti,V,Fe,Al)12(As,Sb)2O23(OH) (Grey et al., Reference Grey, Madsen and Harris1987; Harris et al., Reference Harris, Hoskins, Grey, Criddle and Stanley1989). Both samples contain Ba in detectable amounts, and sample G also shows the presence of minor Pb. Barium is twelve-fold coordinated and occurs close to or at the centre of the cuboctahedral cavities in samples F and G, respectively. On the contrary, sample G contains some Pb that was located in a site displaced from the centre, probably due to the lone-electron-pair activity of Pb2+. Its coordination is formed by five oxygen atoms, with distances shorter than 3 Å, and six additional longer bonds, between 3.09 and 3.18 Å, in agreement with Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998).
Metal partitioning among M sites
Taking into account the site multiplicity, the structural formula of derbylite and graeserite can be written (ignoring low-populated Ba/Pb sites) as M(1)M(2)2M(3)2M(4)2SbO13(OH) (Z = 2) and M(1)M(2)2M(3)4AsO12[O0.5(OH)0.5]2 (Z = 2), respectively.
Titanium (Z = 22), V (Z = 23) and Fe (Z = 26) have similar scattering factors and their accurate partitioning among the M sites of derbylite and graeserite is not an easy task. In addition, ionic radii of these transition elements in six-fold coordination are very similar. Following Shannon (Reference Shannon1976), the ionic radii are: Ti 0.605 Å, V3+ 0.640 Å, Fe3+ 0.645 Å and Fe2+ 0.78 Å. Only Fe2+ is significantly larger than the other cations.
In derbylite, the largest site is M(2) and Fe2+ is probably hosted at this position. M(1), M(3) and M(4) have similar average bond distances. M(1) is probably a mixed (Ti,Fe,V) site, whereas M(3) and M(4) are mixed (Ti,Fe) sites, with a higher Ti/Fe atomic ratio than that reported by Moore and Araki (Reference Moore and Araki1976), close to 1. The occurrence of (Fe,V)3+ at M(1) and the different Ti/Fe ratios at M(3) and M(4) with respect to the results of Moore and Araki (Reference Moore and Araki1976) may be related to the cross-substitution: M (1)Ti4+ + M (3),M(4)Fe3+ = M (1)(Fe,V)3+ + M (3),M(4)Ti4+, as well as to the substitution: M (2)Fe3+ + M (3),M(4)Fe3+ = M (2)Fe2+ + M (3),M(4)Ti4+. The cation sites of derbylite from Monte Arsiccio give the following formula: M (1)(Ti0.5Fe3+0.3V3+0.2)M (2)(Fe3+0.65Fe2+0.35)2M (3)(Ti0.8Fe3+0.2)2M (4)(Ti0.8Fe3+0.2)2. This can be compared with the formula proposed by Moore and Araki (Reference Moore and Araki1976), i.e., M (1)TiM (2)Fe3+2 M (3)(Ti0.57Fe3+0.43)2 M (4)(Ti0.43Fe3+0.57)2. Applying the two substitution mechanisms described above, the structural formula of the Monte Arsiccio sample can be simplified as M (1)TiM (2)Fe3+2 M (3),M(4)(Ti0.5Fe3+0.5)4. The M(3) and M(4) sites are virtually indistinguishable and can be considered as an aggregate site.
In graeserite, the M(1) site is probably a mixed (Ti,M 3+) site. In sample F, the refined site scattering at M(1) suggested a pure Ti occupancy. This is unlikely, because observed bond lengths suggested the occurrence of a larger cation replacing Ti. In Table 11, a site population with a mixed (Ti0.80Fe3+0.20) population is proposed. However, another possibility is represented by the occurrence of V3+ at this position. Vanadium has a scattering power very similar to that of Ti, and this could explain the observed site scattering; in addition, its ionic radius is similar to that of Fe3+. Consequently, an alternative site population may be proposed for the M(1) and M(2) sites: M (1)(Ti0.60V3+0.40) and M (2)(Ti0.45V3+0.10Fe3+0.10Fe2+0.35), resulting in a calculated site scattering of 22.4 and 23.9 electrons, and calculated <M–O> distances of 1.98 and 2.03 Å, respectively. These values are very similar to that reported in Table 11 for a slightly different cation distribution, indicating that a reliable metal partitioning among M sites can be very difficult. In V-poor sample G, mixed (Ti,Fe3+) and (Ti,Fe3+,Fe2+) populations at the M(1) and M(2) sites have been proposed. In both samples F and G, the M(3) site is a mixed (Ti,Fe3+) site, with a Ti/Fe atomic ratio close to 0.5. The M(1–3) sites give the formulae M (1)(Ti0.80Fe3+0.20)M (2)(Ti0.35V3+0.30Fe2+0.35)2 M (3)(Ti0.55Fe3+0.45)4 and M (1)(Ti0.85Fe3+0.15)M (2)(Ti0.50Fe3+0.20Fe2+0.30)2 M (3)(Ti0.45Fe3+0.55)4 for samples F and G, respectively. Also in these cases the same substitutions proposed above can be applied, in addition to the substitution V3+ = Fe3+. In both cases the formula approaches the composition M (1)TiM (2)Fe3+2 M (3),M(4)(Ti0.5Fe3+0.5)4.
In both derbylite and graeserite, the V3O5-type columns seems to have the more complex crystal-chemistry. Indeed, whereas its ideal cation composition is TiFe3+2, the actual chemistry is more complex, showing the occurrence of V3+ and Fe2+. On the contrary, the α-PbO2-type octahedral chains have a mixed (Ti,Fe) composition, with an ideal atomic ratio close to 1.
Hydroxyl group position and its relations with the cavity contents
The occurrence of OH groups in derbylite-related minerals has been assumed by several authors (e.g. Nickel and Grey, Reference Nickel and Grey1979; Mellini et al., Reference Mellini, Orlandi and Perchiazzi1983; Grey et al., Reference Grey, Madsen and Harris1987) on the basis of the structure solution of Moore and Araki (Reference Moore and Araki1976) but direct measurements of H2O have not been reported yet. Grey et al. (Reference Grey, Madsen and Harris1987) performed infrared spectroscopy and thermogravimetric analysis to confirm the occurrence of OH groups in Ba-rich tomichite. Both analyses were inconclusive, the former owing to the weakness and broadness of the stretching bands of O–H bonds observed at ~3400 cm–1, whereas the latter suffered the interference between the H2O loss and the As volatilisation. The most convincing data supporting the occurrence of OH groups are related to the solution and refinement of the crystal structures of derbylite-related minerals.
The examination of the BVS at the anion sites in derbylite from Monte Arsiccio (Table 8) shows the occurrence of a strongly underbonded anion, OH(8), having a BVS of 1.06 vu. As F is below the detection limit, this is the likely position of the OH group, in agreement with Moore and Araki (Reference Moore and Araki1976). Actually, this is the most frequent configuration and it is related to the occupancy of the Sb(a) position. When Sb(a) is vacant and Sb(b) is occupied, the likely position of OH group is at the O(1) site that becomes underbonded. In both cases, O⋅⋅⋅O distances compatible with H-bonds are formed with two O(4) sites, with OH(8)⋅⋅⋅O(4) and O(1)⋅⋅⋅O(4) of 2.983(3) and 2.875(3) Å, respectively.
In graeserite, the location of OH groups is not straightforward. Table 12 shows that the weighted BVS at the O sites show no significant underbonding for the O atoms; the underbonding of O(4) is not large enough to allow the proposal of the occurrence of OH groups at this position. However, a critical examination of these values is mandatory, in particular as regards the O(4) and O(5) sites. Indeed, the disordered nature of the sites occurring in the cuboctahedral cavities give rise to different local configurations, as exemplified in Fig. 5. It is worth noting that O(4), shared between consecutive cuboctahedral cavities, can be bonded to only one As3+ cation, in order to avoid its overbonding. The possible occupancy at the cuboctahedral cavities has been discussed by Grey et al. (Reference Grey, Madsen and Harris1987) and Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998). Whereas only one SbO3 group can be hosted within a cuboctahedral cavity, owing to steric reasons, one or two AsO3 groups can occupy one cavity. This is in agreement with the sum of (Ba/Pb) + (As/Sb) exceeding 1 apfu in As-dominant derbylite-related minerals.

Fig. 5. Hypothetical ordered sequences within the cuboctahedral cavities of graeserite. Symbols: large circle represents Ba; medium circle is As; and small circles are O sites. The O(5) site is highlighted and it is shown as light blue circles. For the sake of completeness, labels of the other O sites forming the cavities are shown in (a).
Oxygen atoms hosted at O(4) and O(5) are bonded to two M(3) sites and to one M(1) and two M(2) sites, respectively. Using this approach, the resulting BVS at O(4) and O(5) are ~0.9 and 1.3–1.4 vu, respectively. The actual BVS is then the result of the cation configuration in the cavities. There are three main possibilities (Fig. 5): (1) only one (As/Sb)O3 group occurs within a cavity; (2) two AsO3 groups occur within a cavity; and (3) a (Ba/Pb) cation occurs in the cavity. The first case is observed in derbylite. In this case, whereas O(4) is bonded to (As/Sb) atoms, achieving a BVS close to 2 vu, the two O(5) sites can be divided in one bonded to the (As/Sb) atoms and the other that is not bonded. In the former case, O(5) achieves a total BVS close to 2 vu and it is occupied by an O2– atom, whereas in the latter its BVS agrees with its occupancy by an OH group. In the second configuration, both O(4) and O(5) are always occupied by O2–, whereas in the third configuration, O(5) is occupied by OH groups. It is worth noting that the configuration (2) and (3) are closely related, because they allow the electrostatic neutrality of the chemical formula, through the substitution As3+ +O(5)O2– = (Ba/Pb)2+ + O(5)OH–. This mechanism has been proposed above, during the discussion of the chemical formula of derbylite and graeserite and it is supported by bond-valence arguments. The other mechanism, (As/Sb)3+ + 3O2– = □ + 3OH–, seems to play a very minor role in derbylite-related minerals and it will not be discussed here.
It is worth noting that the occurrence of (Ba/Pb) atoms could also be considered as a kind of ‘structural defect’ favouring a change in the ‘polarity’ of the (As/Sb)O3 trigonal pyramids. Indeed, two (AsO3) groups can decorate a (Ba/Pb)-filled cavity (Fig. 5a) or only one (AsO3) group can be bonded to an edge of the cuboctahedral cavity, in this approach allowing the change of the direction of the lone electron pairs of (As/Sb) atoms (Fig. 5b). This means the O(4) sites not bonded to (As/Sb) atoms are probably occupied by OH–. As a final consideration, it cannot be excluded that the local ordering could also influence the occupancy of nearby octahedral sites, adding further complexity to the real structure of graeserite.
Conclusion
The crystal-chemical characterisation of derbylite and graeserite is a further puzzle in the mineralogical knowledge of the hydrothermal ores from the Apuan Alps. Subtle variations in the As/Sb ratio in the crystallisation environments may favour the formation of derbylite (high Sb, low As) or graeserite (high As, low Sb). The role of Ba and minor Pb in the stabilisation of the latter mineral is not clear. However, it seems likely that the crystal structure of graeserite can be a host for minor large divalent cations (e.g. Ba2+ or Pb2+). The complex crystal-chemistry of the extraframework constituents (Sb, As, Ba and Pb) is probably at the origin of the satellite reflections reported by previous authors (e.g. Grey et al., Reference Grey, Madsen and Harris1987; Berlepsch and Armbruster, Reference Berlepsch and Armbruster1998) and the transition from the P21/m to the C2/m space group, as suggested by the pair tomichite–Ba-rich tomichite (Grey et al., Reference Grey, Madsen and Harris1987). Berlepsch and Armbruster (Reference Berlepsch and Armbruster1998) attributed the occurrence of an incommensurate superstructure to the presence of several residuals that were interpreted as disordered M sites. No such disorder was observed in the samples from the Monte Arsiccio mine, whereas some disorder was reported for sites hosted in the cuboctahedral cavities, as discussed above. This demands further investigation to unravel the short-range cation order within these cuboctahedral cavities.
In addition to the finding of derbylite and graeserite in hydrothermal veins, the finding of graeserite as porphyroblasts in schist from the Sant'Anna level suggests that this mineral may also occur as a metamorphic accessory phase in As-bearing environments. Its role in the possible release of As in the environment upon rock weathering is currently under further investigation.
Acknowledgements
Alois Lechner is thanked for providing us with the specimen of graeserite from the Sant'Anna level. This research was supported financially by the Ministero dell'Istruzione, Università e Ricerca through the project PRIN 2017 “TEOREM – deciphering geological processes using Terrestrial and Extraterrestrial ORE Minerals”, prot. 2017AK8C32, and from the University of Pisa through the project P.R.A. 2018-2019 “Georisorse e Ambiente” (Grant No. PRA_2018_41). The authors are grateful to the University Centrum for Applied Geosciences (UCAG) for the access to the E. F. Stumpfl electron microprobe laboratory. The careful revision of Yves Moëlo and two anonymous reviewers improved the manuscript.
Supplementary material
To view supplementary material for this article, please visit https://doi.org/10.1180/mgm.2020.67



















