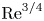…Oft turning others’ leaves, to see if thence would flow
Some fresh and fruitful showers upon my sunburn'd brain.
But words came halting forth, wanting invention's stay;
Invention, Nature's child, fled step-dame Study's blows;
And others’ feet still seemed but strangers in my way.
Thus great with child to speak and helpless in my throes,
Biting my truant pen, beating myself for spite,
“Fool,” said my Muse to me, “look in thy heart, and write.”
Sir Philip Sidney, Astrophil and Stella
Nothing is more usual and more natural for those, who pretend to discover any thing new to the world in philosophy and sciences, than to insinuate the praises of their own systems, by decrying all those, which have been advanced before them.
David Hume, A Treatise of Human Nature
1. Introduction
At times during the last two decades, watching furious debates about the theory of magnetohydrodynamic (MHD) turbulence raging over increasingly technical and/or unfalsifiable issues, or working hard on minute refinements to existing results, one might have been forgiven for gradually losing interest. Is MHD turbulence to follow hydrodynamic (isotropic, homogeneous, Kolmogorov) turbulence and become a boutique field, ever more disconnected from the excitements of ‘real’ physics? This perhaps is the fate of any successful theory (what more is there to be done?) or indeed of one that stalls for too long after initial breakthroughs (all the low-hanging fruit already picked?).
Most of the reasons for which I have found myself writing this piece with a degree of renewed enthusiasm emerged or crystallised in and since 2017. Enough has happened in these recent years for this text to be entirely different than it would have been had it been written before 2017; I do not think that the same could have been said during any of the 5, perhaps 10, years before that. The last significant conceptual breakthrough predating 2017 was the dynamic-alignment theory of Boldyrev (Reference Boldyrev2006) (see § 6.2), which updated the previous decade's paradigm-changing theory of Goldreich & Sridhar (Reference Goldreich and Sridhar1995) (§ 5) and was followed by a flurry of numerical activity, sustaining the field for nearly 10 years. Some of the furious debates alluded to above had to do with the validity of this work – but in the absence of a new idea as to what might be going on dynamically, the insistence in a series of papers by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b) that Boldyrev's theory failed at small scales (meeting with casual dismissal from Beresnyak's opponents and with amused indifference from the rest of the community) appeared doomed to be kicked into the long grass, waiting for ever bigger computers.Footnote 1
Simultaneously, the community has been showing increasing interest and investing increasing resources into studying the dissipation mechanisms in MHD turbulence – in particular, the role of spontaneously formed current sheets and associated local reconnection processes (this was pioneered a long time ago by Matthaeus & Lamkin Reference Matthaeus and Lamkin1986 and Politano, Pouquet & Sulem Reference Politano, Pouquet and Sulem1989, but has only recently bloomed into an active field: see references in § 7). The most intriguing question (which, however, remained mostly unasked – in print – until 2017) surely had to be this: if Boldyrev's MHD turbulence consisted of structures that were ever more aligned and so ever more sheet-like at small scales, was a scale eventually to be reached, given a broad enough inertial range, where these sheets would become too thin to stay stable and the reconnection processes known to disrupt such sheets would kick in?
Like Boldyrev's theory, the full/quantitative realisation that large-aspect-ratio current sheets cannot survive also dates back to the first decade of the century, if one accepts that the trigger was the paper by Loureiro, Schekochihin & Cowley (Reference Loureiro, Schekochihin and Cowley2007) on the plasmoid instability (see Appendix D.4.2; as always, in retrospect, one can identify early precursors, notably Bulanov, Syrovatskiǐ & Sakai Reference Bulanov, Syrovatskiǐ and Sakai1978; Bulanov, Sakai & Syrovatskii Reference Bulanov, Sakai and Syrovatskii1979, Biskamp Reference Biskamp1982, Reference Biskamp1986 and Tajima & Shibata Reference Tajima and Shibata1997). This, however, did not translate into a clear understanding of the disruption of dynamically forming sheets until the papers by Pucci & Velli (Reference Pucci and Velli2014) and Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) (which, in fact, had been around in preprint form since 2014). Once this result was out, it did not take long (even so, it took surprisingly long) to apply it to Boldyrev's aligning structures – it is this calculation (see § 7), published in the twin papers by Mallet, Schekochihin & Chandran (Reference Mallet, Schekochihin and Chandran2017b) and Loureiro & Boldyrev (Reference Loureiro and Boldyrev2017b), that, in my view, pushed the theory of MHD turbulence forward far enough that it is now both closer to a modicum of logical completeness and ripe for a review. The outcome appears to be that the Beresnyak vs. Boldyrev controversy is resolved (both are right, in a sense: see § 7.2.2), Kolmogorov's dissipation scale is back, in a somewhat peculiar way, reconnection and turbulence have joined hands, and the modellers hunting for current sheets have been vindicated and offered further scope for their modelling.
While emphasising this development as conceptually the most exciting amongst the recent ones, I will also take the opportunity presented by this review to discuss, in § 4 and Appendix A, my reservations about the standard version of weak Alfvén-wave turbulence theory and some ideas for how to fix (or interpret) it; to summarise, in § 6.4, what I view as a set of rather pretty new ideas on the intrinsically intermittent nature of aligned turbulence (Mallet, Schekochihin & Chandran Reference Mallet, Schekochihin and Chandran2015; Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016; Chandran et al. Reference Chandran, Schekochihin and Mallet2015; Mallet & Schekochihin Reference Mallet and Schekochihin2017); to explore some old ideas, and propose some new ones, on various imbalanced regimes of MHD turbulence (with cross-helicity, with residual magnetic energy, subviscous, decaying: see §§ 9–12 – in the case of decaying turbulence, reconnection has stolen the limelight again and some very neat new ideas have recently emerged); to offer an updated, if tentative, perspective on the saturated state of MHD dynamo – i.e., MHD turbulence with no mean field, which turns out also to be intertwined with reconnection (§ 13); and to advocate (in § 14) a number of lines of further investigation focusing on plasma effects – some of which have started emerging in a particularly intriguing way during the last few years. That over a half of this review is taken up by these sections, treating of open questions, old confusions, new speculative schemes, and promising directions for further forays into the unknown, should alert my reader to a very heavy caveat to my earlier claim about the ‘logical completeness’ of our current picture of MHD turbulence: this claim in fact only applies to forced, balanced, inertial-range turbulence, with collisional dissipation and in the presence of a strong mean field. To many disappointed readers, this will seem to be a rarefied version of MHD turbulence falling short of anything useful in any global context or indeed of appearing anywhere in reality. This is true, but only a few years ago, we did not even have a grip on that!
Because the subject of this review, if not exactly young, is still an active one and no one narrative has been settled as definitive, my exposition will be chronological, rather than logical, viz., I will discuss ideas that have proved to be wrong or incomplete before getting to those that as yet have not – not least because the latter were strongly influenced by, and would not have emerged without, the former. One day, there will be a much shorter story told in textbooks, with all intermediate steps forgotten. The erudites who already know this history, are uninterested in my prose and just want to skim the essential points and check out the new bits can start by reading §§ 6.4, 7.1–7.3, 9.6, 10.4, 11, 12.4, 13.3, and Appendices A.4, C, D.5.2, D.6.2–D.6.3, and D.7. In § 8, there is a summary and discussion of the main takeaways from the material covered up to that point.
Before proceeding, I would like, by way of a disclaimer, to stress the point that is already made in the title of this piece: this is a thoroughly biased review. Rather than merely recycling the truism that there is no such thing as an unbiased review of anything, I am apologising here for this one drawing particularly heavily on published papers in which I myself participated. I hope that I might nonetheless be forgiven on the grounds that the lion's share of the credit for those contributions in fact belongs to my co-authors. Leaving to more disengaged spectators the task of assigning to these works their true measure of (in)significance, perhaps as minor flecks of colour on the vast canvas of MHD turbulence theory, I will instead present this subject as I see it at the moment, with those flecks in the foreground.
PART ONE: A long road to Kolmogorov
Omnes autem, quae in rerum natura contingunt, mutationes ita sunt comparatae, ut si quid alicui rei accedit, id alteri derogetur. […] Quae naturae lex cum sit universalis, ideo etiam ad regulas motus extenditur…
M. V. Lomonosov, Letter to L. Euler, 5 July 1748 Footnote 2
2. K41, IK and GS95
The basic starting point for this discussion is to imagine a static, homogeneous plasma or, more generally, a conducting continuous medium, threaded by a uniform magnetic field. One can think of this situation as describing some local patch of a larger system, in which the magnetic field and other equilibrium parameters (density, pressure, flow velocity) are large-scale and structured in some system-dependent way. I am not going to be concerned (except much later, in §§ 12 and 13) with the question of what this large-scale structure is or how it is brought about – locally, it always looks like our homogeneous patch. Within this patch, I shall consider perturbations whose time and length scales are short compared to any length scales associated with that large-scale structure. Of course, such a local approximation is not entirely universal: I am putting aside the cases of strong shear, various stratified or rotating systems, etc. – or, to be precise, I am excluding from consideration perturbations that are sufficiently extended in space and/or time to ‘feel’ these background gradients. Arguably, in an ideal asymptotic world inhabited by theoretical physicists, one can always go to scales small enough for this restriction to be justified, without hitting dissipation/microphysical scales first (in a real world, this is, regrettably, not always true, but let us understand the asymptotically idealised reality first). The only large-scale feature that does not thus go away at small scales is the magnetic field. This is what makes MHD turbulence a priori different from, for example, rotating or stratified turbulence, which, at small enough scales, always reverts to the universal Kolmogorov state (see, e.g., Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011).
2.1. K41
Let us recall with maximum brevity what this Kolmogorov state is. Assume that energy is being pumped into the system at large scales and at some fixed rate ![]() $\varepsilon$. Then, in the inertial range (i.e., at small enough scales so the system is locally homogeneous but not small enough for viscosity or any other microphysics to matter yet), this same
$\varepsilon$. Then, in the inertial range (i.e., at small enough scales so the system is locally homogeneous but not small enough for viscosity or any other microphysics to matter yet), this same ![]() $\varepsilon$ is the constant energy flux from scale to scale. Assuming that the cascade (i.e., the passing of energy from scale to scale) is local, the energy spectrum is, by dimensional analysis,
$\varepsilon$ is the constant energy flux from scale to scale. Assuming that the cascade (i.e., the passing of energy from scale to scale) is local, the energy spectrum is, by dimensional analysis,
the famous Kolmogorov spectrum (Kolmogorov Reference Kolmogorov1941b; henceforth K41), or, in terms of typical velocity increments between points separated by a distance ![]() $\lambda$:
$\lambda$:
This is all obvious because the dimensions of the quantities involved are
where ![]() $U$ is a unit of velocity and
$U$ is a unit of velocity and ![]() $L$ of length. As we will be dealing with an incompressible medium (which is always achievable by going to small enough scales and so to sufficiently subsonic motions), its density is an irrelevant constant.Footnote 3
$L$ of length. As we will be dealing with an incompressible medium (which is always achievable by going to small enough scales and so to sufficiently subsonic motions), its density is an irrelevant constant.Footnote 3
2.2. IK
It was Kraichnan (Reference Kraichnan1965) who appears to have been the first to realise clearly the point made above about the irreducibility of the magnetic field. He therefore argued that, if the background uniform magnetic field ![]() $\boldsymbol {B}_0$, which in velocity units is called the Alfvén speed,
$\boldsymbol {B}_0$, which in velocity units is called the Alfvén speed,
(![]() $\rho _0$ is the mass density of the conducting medium), was to have a persistent (at small scales) role in the energy transfer from scale to scale, then the energy spectrum in the inertial range must be, again by dimensional analysis,
$\rho _0$ is the mass density of the conducting medium), was to have a persistent (at small scales) role in the energy transfer from scale to scale, then the energy spectrum in the inertial range must be, again by dimensional analysis,
This is known as the Iroshnikov–Kraichnan spectrum (henceforth IK; figure 1).Footnote 4 The scaling exponent was fixed by the requirement, put forward with the trademark combination of deep insight and slightly murky argumentation that one often finds in Kraichnan's papers, that the Alfvén time ![]() $\tau _{\mathrm {A}}\sim 1/kv_{\mathrm {A}}$ was the typical time during which interactions would occur (before build-up of correlations was arrested by perturbations propagating away from each other), so the energy flux had to be proportional to
$\tau _{\mathrm {A}}\sim 1/kv_{\mathrm {A}}$ was the typical time during which interactions would occur (before build-up of correlations was arrested by perturbations propagating away from each other), so the energy flux had to be proportional to ![]() $\tau _{\mathrm {A}}$ and, therefore, to
$\tau _{\mathrm {A}}$ and, therefore, to ![]() $1/v_{\mathrm {A}}$ – thus requiring them to enter in the combination
$1/v_{\mathrm {A}}$ – thus requiring them to enter in the combination ![]() $\varepsilon v _{\mathrm {A}}$.Footnote 5
$\varepsilon v _{\mathrm {A}}$.Footnote 5

Figure 1. IK and GS (photo of R. S. Iroshnikov courtesy of N. Lipunova and K. Bychkov, Sternberg Astronomical Institute; photo of R. H. Kraichnan courtesy of the AIP Emilio Segrè Visual Archives).
Kraichnan's prediction was viewed as self-evidently correct for 30 years, then wrong for 10 years (§ 5), then correct again (in a different sense) for another 10 years (§ 6), then had to be revised again, at small enough scales (§ 7). His own interpretation of it (which was also Iroshnikov's, arrived at independently) was certainly wrong, as it was based on the assumption – natural for a true Kolmogorovian susceptible to the great man's universalist notion of ‘restoration of symmetries’ at small scales, but, in retrospect, illogical in the context of proclaiming the unwaning importance of ![]() $\boldsymbol {B}_0$ at those same small scales – that turbulence sufficiently deep in the inertial range would be isotropic, i.e., that there is only one
$\boldsymbol {B}_0$ at those same small scales – that turbulence sufficiently deep in the inertial range would be isotropic, i.e., that there is only one ![]() $k$ to be used in the dimensional analysis. In fact, one both can and should argue that, a priori, there is a
$k$ to be used in the dimensional analysis. In fact, one both can and should argue that, a priori, there is a ![]() $k_{\parallel }$ and a
$k_{\parallel }$ and a ![]() $k_{\perp }$, which represent the variation of the turbulent fields along and across
$k_{\perp }$, which represent the variation of the turbulent fields along and across ![]() $\boldsymbol {B}_0$ and need not be the same. The presence of the dimensionless ratio
$\boldsymbol {B}_0$ and need not be the same. The presence of the dimensionless ratio ![]() $k_{\parallel }/k_{\perp }$ undermines the dimensional inevitability of (2.5) and opens up space for much theorising, inspired or otherwise.
$k_{\parallel }/k_{\perp }$ undermines the dimensional inevitability of (2.5) and opens up space for much theorising, inspired or otherwise.
2.3. GS95
Intuitively, in a strong magnetic field, perturbations with ![]() $k_{\parallel } \ll k_{\perp }$ should be more natural than isotropic ones, as the field is frozen into the motions but hard to bend. It turns out that MHD turbulence is indeed anisotropic in this way, at all scales, however small (see figure 2). This was realised quite early on, when the first, very tentative, experimental and numerical evidence started to be looked at (Robinson & Rusbridge Reference Robinson and Rusbridge1971; Montgomery & Turner Reference Montgomery and Turner1981; Shebalin, Matthaeus & Montgomery Reference Shebalin, Matthaeus and Montgomery1983), but, interestingly, it took more than a decade after that for the IK theory to be properly revised.
$k_{\parallel } \ll k_{\perp }$ should be more natural than isotropic ones, as the field is frozen into the motions but hard to bend. It turns out that MHD turbulence is indeed anisotropic in this way, at all scales, however small (see figure 2). This was realised quite early on, when the first, very tentative, experimental and numerical evidence started to be looked at (Robinson & Rusbridge Reference Robinson and Rusbridge1971; Montgomery & Turner Reference Montgomery and Turner1981; Shebalin, Matthaeus & Montgomery Reference Shebalin, Matthaeus and Montgomery1983), but, interestingly, it took more than a decade after that for the IK theory to be properly revised.

Figure 2. A visualisation of numerical RMHD turbulence, courtesy of A. Beresnyak (run R5 from Beresnyak Reference Beresnyak2012a, ![]() $1536^3$). The shades of grey represent the absolute value of
$1536^3$). The shades of grey represent the absolute value of ![]() $\boldsymbol {Z}_{\perp }^+ = \boldsymbol {u}_{\perp } + \boldsymbol {b}_{\perp }$ (see § 3).
$\boldsymbol {Z}_{\perp }^+ = \boldsymbol {u}_{\perp } + \boldsymbol {b}_{\perp }$ (see § 3).
Dynamically, the parallel variation (on scale ![]() $l_{\parallel }\sim k _{\parallel }^{-1}$) is associated with the propagation of Alfvén (Reference Alfvén1942) waves, the wave period (or ‘propagation time’) being
$l_{\parallel }\sim k _{\parallel }^{-1}$) is associated with the propagation of Alfvén (Reference Alfvén1942) waves, the wave period (or ‘propagation time’) being
and the perpendicular variation (on scale ![]() $\lambda \sim k _{\perp }^{-1}$) with nonlinear interactions, whose characteristic time is naïvely equal to the ‘eddy-turnover time’:
$\lambda \sim k _{\perp }^{-1}$) with nonlinear interactions, whose characteristic time is naïvely equal to the ‘eddy-turnover time’:
(we shall see in § 6 why this is naïve). Here and below, ![]() $\delta u_\lambda$ is used to represent the turbulent field on the grounds that, in Alfvénic perturbations,
$\delta u_\lambda$ is used to represent the turbulent field on the grounds that, in Alfvénic perturbations, ![]() $\delta u_\lambda \sim \delta b_\lambda$, where
$\delta u_\lambda \sim \delta b_\lambda$, where ![]() $\delta b$ is the magnetic perturbation in velocity units (see § 3 for a discussion with equations). Declaring the two times comparable at all scales was an inspired conjecture by Goldreich & Sridhar (Reference Goldreich and Sridhar1995, Reference Goldreich and Sridhar1997) (henceforth GS95; figure 1),Footnote 6 which has come to be known as the critical balance (CB). I shall discuss the physical reasons for it properly in §§ 4 and 5, but here let me just postulate it. Then, naturally, the ‘cascade time’ (i.e., the typical time to transfer energy from one perpendicular scale
$\delta b$ is the magnetic perturbation in velocity units (see § 3 for a discussion with equations). Declaring the two times comparable at all scales was an inspired conjecture by Goldreich & Sridhar (Reference Goldreich and Sridhar1995, Reference Goldreich and Sridhar1997) (henceforth GS95; figure 1),Footnote 6 which has come to be known as the critical balance (CB). I shall discuss the physical reasons for it properly in §§ 4 and 5, but here let me just postulate it. Then, naturally, the ‘cascade time’ (i.e., the typical time to transfer energy from one perpendicular scale ![]() $\lambda$ to the next) must be of the same order as either of the two other times:
$\lambda$ to the next) must be of the same order as either of the two other times:
If (2.7) is used for ![]() $\tau _{\mathrm {nl}}$, then (2.8) obviates the magnetic field and returns us to the K41 scaling (2.2), viz.,
$\tau _{\mathrm {nl}}$, then (2.8) obviates the magnetic field and returns us to the K41 scaling (2.2), viz.,
This anisotropic version of K41 is known as the Goldreich–Sridhar (or GS95) spectrum. Simultaneously, along the field,Footnote 7 the velocity increments must satisfy
 \begin{equation} \frac{\delta u_{l_{{\parallel}}}^2}{\tau_{\mathrm{c}}} \sim \varepsilon,\quad \tau_{\mathrm{c}}\sim\tau_{\mathrm{A}}\sim\frac{l_{{\parallel}}}{v_{\mathrm{A}}} \quad\Rightarrow\quad \delta u_{l_{{\parallel}}}\sim\left(\frac{\varepsilon l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}. \end{equation}
\begin{equation} \frac{\delta u_{l_{{\parallel}}}^2}{\tau_{\mathrm{c}}} \sim \varepsilon,\quad \tau_{\mathrm{c}}\sim\tau_{\mathrm{A}}\sim\frac{l_{{\parallel}}}{v_{\mathrm{A}}} \quad\Rightarrow\quad \delta u_{l_{{\parallel}}}\sim\left(\frac{\varepsilon l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}. \end{equation} Thus, ![]() $\boldsymbol {B}_0$'s influence does persist, but its size enters only the parallel scaling relations, not the perpendicular ones. Formally speaking, (2.9) is just the K41 dimensional argument for the perpendicular scale
$\boldsymbol {B}_0$'s influence does persist, but its size enters only the parallel scaling relations, not the perpendicular ones. Formally speaking, (2.9) is just the K41 dimensional argument for the perpendicular scale ![]() $\lambda$, with the CB conjecture used to justify not including
$\lambda$, with the CB conjecture used to justify not including ![]() $v_{\mathrm {A}}$ and
$v_{\mathrm {A}}$ and ![]() $l_{\parallel }$ amongst the local governing parameters. The assumption is that the sole role of
$l_{\parallel }$ amongst the local governing parameters. The assumption is that the sole role of ![]() $\boldsymbol {B}_0$ is to set the value of
$\boldsymbol {B}_0$ is to set the value of ![]() $l_{\parallel }$ for any given
$l_{\parallel }$ for any given ![]() $\lambda$: comparing (2.9) and (2.10), we get
$\lambda$: comparing (2.9) and (2.10), we get
Physically, this ![]() $l_{\parallel }$ is the distance that an Alfvénic pulse travels along the field, at speed
$l_{\parallel }$ is the distance that an Alfvénic pulse travels along the field, at speed ![]() $v_{\mathrm {A}}$, over the time
$v_{\mathrm {A}}$, over the time ![]() $\tau _{\mathrm {nl}}$, given by (2.7), that it takes a turbulent perturbation of size
$\tau _{\mathrm {nl}}$, given by (2.7), that it takes a turbulent perturbation of size ![]() $\lambda$ to break up nonlinearly. It is natural to argue, by causality, that this is the maximum distance over which any perturbation can remain correlated (Boldyrev Reference Boldyrev2005; Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011).
$\lambda$ to break up nonlinearly. It is natural to argue, by causality, that this is the maximum distance over which any perturbation can remain correlated (Boldyrev Reference Boldyrev2005; Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011).
This narrative arc brings us approximately to the state of affairs in the mid-1990s, although the GS95 theory did not really become mainstream until the early years of this century – and soon had to be revised. Before I move on to discussing this revision (§ 6) and the modern state of the subject, I would like to put the discussion of what happens dynamically and how CB is achieved on a slightly less hand-waving basis than I have done so far. Indeed, why critical balance? Pace the causality argument, which sets the maximum ![]() $l_{\parallel }$, why can
$l_{\parallel }$, why can ![]() $l_{\parallel }$ not be shorter? Is the nonlinear-time estimate (2.7), crucial for the scaling (2.9), justified? What happens dynamically?
$l_{\parallel }$ not be shorter? Is the nonlinear-time estimate (2.7), crucial for the scaling (2.9), justified? What happens dynamically?
From this point on, my exposition will be more sequential, I will avoid jumping ahead to the highlights and adopt a more systematic style, rederiving carefully some of the results reviewed in this section (an already well educated – or impatient – reader is welcome to skip or skim forward at her own pace).
3. Reduced MHD
The theoretical assumption (or numerical/observational evidence) that MHD turbulence consists of perturbations that have ![]() $k_{\perp }\gg k _{\parallel }$ but that their Alfvénic propagation remains important (so as to allow CB should the system want to be in it) leads to the following set of equations for these perturbations:
$k_{\perp }\gg k _{\parallel }$ but that their Alfvénic propagation remains important (so as to allow CB should the system want to be in it) leads to the following set of equations for these perturbations:
These are evolution equations for the Elsasser (Reference Elsasser1950) fields ![]() $\boldsymbol {Z}_{\perp }^\pm = \boldsymbol {u}_\perp \pm \boldsymbol {b}_{\perp }$, where
$\boldsymbol {Z}_{\perp }^\pm = \boldsymbol {u}_\perp \pm \boldsymbol {b}_{\perp }$, where ![]() $\boldsymbol {u}_{\perp }$ is the fluid velocity perpendicular to the equilibrium field
$\boldsymbol {u}_{\perp }$ is the fluid velocity perpendicular to the equilibrium field ![]() $\boldsymbol {B}_0$, and
$\boldsymbol {B}_0$, and ![]() $\boldsymbol {b}_{\perp }$ is the magnetic-field perturbation, also perpendicular to
$\boldsymbol {b}_{\perp }$ is the magnetic-field perturbation, also perpendicular to ![]() $\boldsymbol {B}_0$ and expressed in velocity units, i.e., scaled to
$\boldsymbol {B}_0$ and expressed in velocity units, i.e., scaled to ![]() $\sqrt {4\pi \rho _0}$. The total pressure
$\sqrt {4\pi \rho _0}$. The total pressure ![]() $p$ (which includes the magnetic pressure) is determined by the condition that
$p$ (which includes the magnetic pressure) is determined by the condition that ![]() $\boldsymbol {\nabla }_{\perp }\cdot \boldsymbol {Z}_{\perp }^\pm = 0$, enforcing the solenoidality of the magnetic field and the incompressibility of the motions, the latter achieved at small enough scales by small enough perturbations. Namely,
$\boldsymbol {\nabla }_{\perp }\cdot \boldsymbol {Z}_{\perp }^\pm = 0$, enforcing the solenoidality of the magnetic field and the incompressibility of the motions, the latter achieved at small enough scales by small enough perturbations. Namely, ![]() $p$ is the solution of
$p$ is the solution of
which amounts to multiplying the nonlinear term on the left-hand side of (3.1) by a projection operator in Fourier space. I have, for simplicity, taken the kinematic viscosity and magnetic diffusivity ![]() $\eta$ to be the same (but will relax this assumption from § 6.4 onwards). The last term in equation (3.1), the body force
$\eta$ to be the same (but will relax this assumption from § 6.4 onwards). The last term in equation (3.1), the body force ![]() $\boldsymbol {f}^\pm$, stands in for any energy-injection mechanism that this small-scale approximation might inherit from the non-universal large scales.
$\boldsymbol {f}^\pm$, stands in for any energy-injection mechanism that this small-scale approximation might inherit from the non-universal large scales.
The Reduced MHD equations (3.1–3.2) (RMHD, first proposed by Strauss Reference Strauss1976, but, as often happened in those days, also found independently by the Soviets, Kadomtsev & Pogutse Reference Kadomtsev and Pogutse1974), which also have a compact scalar form (see Appendix A.1), can be derived from the standard compressible MHD equations by ordering all perturbations of the equilibrium to be comparable to the Mach number and to ![]() $k_{\parallel }/k_{\perp }\ll 1$ and the rate of change of these perturbations to the Alfvén frequency
$k_{\parallel }/k_{\perp }\ll 1$ and the rate of change of these perturbations to the Alfvén frequency ![]() $k_{\parallel} v _{\mathrm {A}}$ (see Schekochihin & Cowley Reference Schekochihin and Cowley2007 or Schekochihin Reference Schekochihin2022; a number of similar, if ever so subtly different, schemes exist: see review by Oughton, Matthaeus & Dmitruk Reference Oughton, Matthaeus and Dmitruk2017 and references therein). These equations, apart from the visco-resistive terms, are, in fact, more general than the collisional MHD approximation and apply also to low-frequency, long-wavelength collisionless perturbations near a gyrotropic equilibrium (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015),Footnote 8 which makes them applicable to the solar wind (notable for being thoroughly measurable) and many other, more remote, astrophysical plasmas (only measurable with difficulty, but endlessly fascinating to large numbers of curious researchers in gainful employment).
$k_{\parallel} v _{\mathrm {A}}$ (see Schekochihin & Cowley Reference Schekochihin and Cowley2007 or Schekochihin Reference Schekochihin2022; a number of similar, if ever so subtly different, schemes exist: see review by Oughton, Matthaeus & Dmitruk Reference Oughton, Matthaeus and Dmitruk2017 and references therein). These equations, apart from the visco-resistive terms, are, in fact, more general than the collisional MHD approximation and apply also to low-frequency, long-wavelength collisionless perturbations near a gyrotropic equilibrium (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015),Footnote 8 which makes them applicable to the solar wind (notable for being thoroughly measurable) and many other, more remote, astrophysical plasmas (only measurable with difficulty, but endlessly fascinating to large numbers of curious researchers in gainful employment).
While, like any nonlinear equations of serious consequence, they are impossible to solve except in almost-trivial special cases, the RMHD equations possess a number of remarkable properties that form the basis for all theories of their turbulent solutions.
(i) The perturbations described by them, known as Alfvénic, are nonlinear versions of (packets of) Alfvén waves: perturbations of velocity and magnetic field transverse to ![]() $\boldsymbol {B}_0$ and propagating at speed
$\boldsymbol {B}_0$ and propagating at speed ![]() $v_{\mathrm {A}}$ along it (
$v_{\mathrm {A}}$ along it (![]() $\boldsymbol {Z}_{\perp }^+$ in the
$\boldsymbol {Z}_{\perp }^+$ in the ![]() $\boldsymbol {B}_0$ direction,
$\boldsymbol {B}_0$ direction, ![]() $\boldsymbol {Z}_{\perp }^-$ in the
$\boldsymbol {Z}_{\perp }^-$ in the ![]() $-\boldsymbol {B}_0$ direction). They are entirely decoupled from all other perturbations (compressive in the case of fluid MHD, kinetic for a collisionless plasma; see Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009 and Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015) and can be considered in isolation from them. If evolved via full compressible MHD equations, these Alfvénic perturbations do not generate motions or fields that violate the
$-\boldsymbol {B}_0$ direction). They are entirely decoupled from all other perturbations (compressive in the case of fluid MHD, kinetic for a collisionless plasma; see Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009 and Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015) and can be considered in isolation from them. If evolved via full compressible MHD equations, these Alfvénic perturbations do not generate motions or fields that violate the ![]() $k_{\parallel }\ll k _{\perp }$ assumption (e.g., higher-frequency fast MHD waves), so RMHD appears to be well posed in the sense that it does not break the assumptions that it is based on (this was checked numerically by Cho & Lazarian Reference Cho and Lazarian2002, Reference Cho and Lazarian2003, who trod in the footsteps of Matthaeus et al. Reference Matthaeus, Ghosh, Oughton and Roberts1996).
$k_{\parallel }\ll k _{\perp }$ assumption (e.g., higher-frequency fast MHD waves), so RMHD appears to be well posed in the sense that it does not break the assumptions that it is based on (this was checked numerically by Cho & Lazarian Reference Cho and Lazarian2002, Reference Cho and Lazarian2003, who trod in the footsteps of Matthaeus et al. Reference Matthaeus, Ghosh, Oughton and Roberts1996).
(ii) Only counterpropagating fields interact, so the nonlinearity vanishes if either ![]() $\boldsymbol {Z}_{\perp }^+ = 0$ or
$\boldsymbol {Z}_{\perp }^+ = 0$ or ![]() $\boldsymbol {Z}_{\perp }^-=0$, giving rise to the so-called Elsasser states (
$\boldsymbol {Z}_{\perp }^-=0$, giving rise to the so-called Elsasser states (![]() $\boldsymbol {u}_{\perp } = \mp \boldsymbol {b}_{\perp }$), exact nonlinear solutions that are arbitrary-amplitude, arbitrary-shape pulses travelling along
$\boldsymbol {u}_{\perp } = \mp \boldsymbol {b}_{\perp }$), exact nonlinear solutions that are arbitrary-amplitude, arbitrary-shape pulses travelling along ![]() $\boldsymbol {B}_0$ at the velocity
$\boldsymbol {B}_0$ at the velocity ![]() $\mp v _{\mathrm {A}}$.
$\mp v _{\mathrm {A}}$.
(iii) The energies of the two Elsasser fields are conserved individually (apart from any injection and dissipation terms), viz.,
The energy fluxes ![]() $\varepsilon ^\pm = \langle \boldsymbol {Z}_{\perp }^\pm \cdot \boldsymbol {f}^\pm \rangle$ need not be the same and their ratio
$\varepsilon ^\pm = \langle \boldsymbol {Z}_{\perp }^\pm \cdot \boldsymbol {f}^\pm \rangle$ need not be the same and their ratio ![]() $\varepsilon ^+/\varepsilon ^-$ is, in general, a parameter of the problem – when it is different from unity, the turbulence is called imbalanced (§ 9). Another way of framing (3.3) is by stating that RMHD has two invariants, the total energy and the cross-helicity:
$\varepsilon ^+/\varepsilon ^-$ is, in general, a parameter of the problem – when it is different from unity, the turbulence is called imbalanced (§ 9). Another way of framing (3.3) is by stating that RMHD has two invariants, the total energy and the cross-helicity:
respectively (so imbalanced turbulence is turbulence with non-zero cross-helicity). The name of the second invariant has topological origins, alluding, in incompressible 3D MHD, to conservation of linkages between flux tubes and vortex tubes; in the context of small Alfvénic perturbations of a strong uniform mean field ![]() $\boldsymbol {B}_0$, this does not appear to be a useful interpretation.
$\boldsymbol {B}_0$, this does not appear to be a useful interpretation.
(iv) The amplitudes ![]() $\boldsymbol {Z}_{\perp }^\pm$, time and the gradients can be arbitrarily but simultaneously rescaled:
$\boldsymbol {Z}_{\perp }^\pm$, time and the gradients can be arbitrarily but simultaneously rescaled: ![]() $\forall \epsilon$ and
$\forall \epsilon$ and ![]() $a$,
$a$,
This means that ![]() $\boldsymbol {Z}_{\perp }^\pm$ and
$\boldsymbol {Z}_{\perp }^\pm$ and ![]() $\nabla _{\parallel }$ are, formally speaking, infinitesimal compared to
$\nabla _{\parallel }$ are, formally speaking, infinitesimal compared to ![]() $v_{\mathrm {A}}$ and
$v_{\mathrm {A}}$ and ![]() $\nabla _{\perp }$, respectively (perpendicular and parallel distances in RMHD are measured ‘in different units’, as are the Alfvén speed and
$\nabla _{\perp }$, respectively (perpendicular and parallel distances in RMHD are measured ‘in different units’, as are the Alfvén speed and ![]() $\boldsymbol {Z}_{\perp }^\pm$). Any statistical scalings or heuristic theories must respect this symmetry (Beresnyak Reference Beresnyak2011, Reference Beresnyak2012a) – this requirement will feature prominently in § 6.4. Note that this symmetry implies that the parallel-to-perpendicular aspect ratio of the numerical box in simulations of RMHD is an arbitrary parameter.
$\boldsymbol {Z}_{\perp }^\pm$). Any statistical scalings or heuristic theories must respect this symmetry (Beresnyak Reference Beresnyak2011, Reference Beresnyak2012a) – this requirement will feature prominently in § 6.4. Note that this symmetry implies that the parallel-to-perpendicular aspect ratio of the numerical box in simulations of RMHD is an arbitrary parameter.
(v) Defining field increments
where ![]() ${\boldsymbol {\lambda }}$ is a point-separation vector in the perpendicular plane, assuming statistical isotropy in this plane and considering separations
${\boldsymbol {\lambda }}$ is a point-separation vector in the perpendicular plane, assuming statistical isotropy in this plane and considering separations ![]() $\lambda = |{\boldsymbol {\lambda }}|$ belonging to the inertial range (i.e., smaller than the energy-injection scale but greater than the viscous/resistive scale), one finds, in a statistical steady state,Footnote 9
$\lambda = |{\boldsymbol {\lambda }}|$ belonging to the inertial range (i.e., smaller than the energy-injection scale but greater than the viscous/resistive scale), one finds, in a statistical steady state,Footnote 9
where ![]() $\delta Z^\mp _L = \delta \boldsymbol {Z}^\mp _{\boldsymbol {\lambda }}\cdot {\boldsymbol {\lambda }}/\lambda$ is the ‘longitudinal’ increment. These exact laws are the RMHD version of the exact third-order laws that one always gets for turbulent systems with a convective nonlinearity, resembling the Kolmogorov (Reference Kolmogorov1941a) 4/5 law of hydrodynamic turbulence or (in fact, more closely) the Yaglom (Reference Yaglom1949) 4/3 law for a passive field (because in RMHD,
$\delta Z^\mp _L = \delta \boldsymbol {Z}^\mp _{\boldsymbol {\lambda }}\cdot {\boldsymbol {\lambda }}/\lambda$ is the ‘longitudinal’ increment. These exact laws are the RMHD version of the exact third-order laws that one always gets for turbulent systems with a convective nonlinearity, resembling the Kolmogorov (Reference Kolmogorov1941a) 4/5 law of hydrodynamic turbulence or (in fact, more closely) the Yaglom (Reference Yaglom1949) 4/3 law for a passive field (because in RMHD, ![]() $\boldsymbol {Z}_{\perp }^+$ advects
$\boldsymbol {Z}_{\perp }^+$ advects ![]() $\boldsymbol {Z}_{\perp }^-$ and vice versa). They were derived for incompressible MHD by Politano & Pouquet (Reference Politano and Pouquet1998a,Reference Politano and Pouquetb) assuming spatial isotropy and, isotropy having become untenable, adjusted to their RMHD form (3.7) by Boldyrev, Mason & Cattaneo (Reference Boldyrev, Mason and Cattaneo2009). They provide a useful (although not as restrictive as one might have hoped) analytical benchmark for any aspiring scaling theory of RMHD turbulence, weak or strong.
$\boldsymbol {Z}_{\perp }^-$ and vice versa). They were derived for incompressible MHD by Politano & Pouquet (Reference Politano and Pouquet1998a,Reference Politano and Pouquetb) assuming spatial isotropy and, isotropy having become untenable, adjusted to their RMHD form (3.7) by Boldyrev, Mason & Cattaneo (Reference Boldyrev, Mason and Cattaneo2009). They provide a useful (although not as restrictive as one might have hoped) analytical benchmark for any aspiring scaling theory of RMHD turbulence, weak or strong.
Everything in this review concerns turbulence that can be described by RMHD equations, with the following exceptions: parts of § 12, concerned with various types of decaying MHD turbulence, where energy-containing scales are the main object of study; § 13, which deals with turbulent dynamo – a situation in which ![]() $\boldsymbol {b}_{\perp }$ is emphatically not small compared to
$\boldsymbol {b}_{\perp }$ is emphatically not small compared to ![]() $\boldsymbol {B}_0$ (there is no
$\boldsymbol {B}_0$ (there is no ![]() $\boldsymbol {B}_0$) and so full MHD equations are needed; and § 14, where the limitations of the fluid description and the importance of kinetic effects are discussed.
$\boldsymbol {B}_0$) and so full MHD equations are needed; and § 14, where the limitations of the fluid description and the importance of kinetic effects are discussed.
4. Weak MHD turbulence
Most theory in physics is perturbation theory. In turbulence, the available perturbation theory is the ‘weak-turbulence’ (WT) approximation for wave-carrying systems. Its attraction is that it features a systematic derivation, an appealing interpretation of the turbulent system as a gas of weakly interacting quasiparticles, or ‘quantised’ waves, and quantitative predictions for spectra, or occupation numbers, of these waves (see textbooks by Zakharov, L'vov & Falkovich Reference Zakharov, L'vov and Falkovich1992, Nazarenko Reference Nazarenko2011, Schekochihin Reference Schekochihin2022, or, for a quick recap, Appendix A.2). Putting aside the question of whether the conditions necessary for it to hold are commonly (or ever) satisfied by natural turbulent systems, it is still interesting – and, arguably, also a matter of due diligence – to inquire whether such a regime, and such a theory, are relevant for our RMHD system. ‘Such a regime’ means small amplitudes – small enough for the nonlinear interactions to occur very slowly compared to wave motion. One can certainly imagine, at least in principle, driving an RMHD system in a WT way, very gently.
4.1. WT is irrelevant
On a broad-brush qualitative level, one can deal with this possibility as follows. Assume that in the energy-injection range, represented by some perpendicular scale ![]() $L_{\perp }$ and some parallel scale
$L_{\perp }$ and some parallel scale ![]() $L_{\parallel }=2\pi /k_{\parallel }$, Alfvén waves are generated with amplitudes
$L_{\parallel }=2\pi /k_{\parallel }$, Alfvén waves are generated with amplitudes ![]() $Z^\pm$ so small that
$Z^\pm$ so small that
If they are viewed as interacting quasiparticles (‘![]() $+$’ can only interact with ‘
$+$’ can only interact with ‘![]() $-$’, and vice versa), the momentum and energy conservation in a three-wave interaction require
$-$’, and vice versa), the momentum and energy conservation in a three-wave interaction require
 \begin{equation} \left.\begin{array}{ll@{}} & {\boldsymbol{p}} + {\boldsymbol{q}} = {\boldsymbol{k}},\\ & \omega_{\boldsymbol{p}}^\mp + \omega_{\boldsymbol{q}}^\pm = \omega_{\boldsymbol{k}}^\pm\quad\Rightarrow\quad -p_{{\parallel}} + q_{{\parallel}} = k_{{\parallel}} \end{array}\right\}\quad\Rightarrow\quad q_{{\parallel}} = k_{{\parallel}},\quad p_{{\parallel}} = 0. \end{equation}
\begin{equation} \left.\begin{array}{ll@{}} & {\boldsymbol{p}} + {\boldsymbol{q}} = {\boldsymbol{k}},\\ & \omega_{\boldsymbol{p}}^\mp + \omega_{\boldsymbol{q}}^\pm = \omega_{\boldsymbol{k}}^\pm\quad\Rightarrow\quad -p_{{\parallel}} + q_{{\parallel}} = k_{{\parallel}} \end{array}\right\}\quad\Rightarrow\quad q_{{\parallel}} = k_{{\parallel}},\quad p_{{\parallel}} = 0. \end{equation}
Thus, three-wave interaction in fact involves a wave (![]() ${\boldsymbol {q}}$) scattering off a 2D perturbation (
${\boldsymbol {q}}$) scattering off a 2D perturbation (![]() $p_{\parallel }=0$, not a wave) and becoming a wave (
$p_{\parallel }=0$, not a wave) and becoming a wave (![]() ${\boldsymbol {k}}$) with the same frequency (because
${\boldsymbol {k}}$) with the same frequency (because ![]() $k_{\parallel }=q_{\parallel }$) and a different perpendicular wavenumber (
$k_{\parallel }=q_{\parallel }$) and a different perpendicular wavenumber (![]() ${\boldsymbol {k}}_{\perp } = {\boldsymbol {p}}_{\perp } + {\boldsymbol {q}}_{\perp }$). Intuitively, there will be a cascade of the waves to higher
${\boldsymbol {k}}_{\perp } = {\boldsymbol {p}}_{\perp } + {\boldsymbol {q}}_{\perp }$). Intuitively, there will be a cascade of the waves to higher ![]() $k_{\perp }$. If the amplitude of the waves does not fall off with
$k_{\perp }$. If the amplitude of the waves does not fall off with ![]() $k_{\perp }$ faster than
$k_{\perp }$ faster than ![]() $k_{\perp }^{-1}$, which is equivalent to their energy spectrum being less steep than
$k_{\perp }^{-1}$, which is equivalent to their energy spectrum being less steep than ![]() $k_{\perp }^{-3}$, then the nonlinear-interaction time will become ever shorter with larger
$k_{\perp }^{-3}$, then the nonlinear-interaction time will become ever shorter with larger ![]() $k_{\perp }$, even as the waves’
$k_{\perp }$, even as the waves’ ![]() $k_{\parallel }$ and, therefore, their frequency stay the same. Eventually, at some perpendicular scale, which I shall call
$k_{\parallel }$ and, therefore, their frequency stay the same. Eventually, at some perpendicular scale, which I shall call ![]() $\lambda _{\mathrm {CB}}$, the condition
$\lambda _{\mathrm {CB}}$, the condition ![]() $\tau _{\mathrm {nl}}\gg \tau _{\mathrm {A}}$ will be broken, so we end up with
$\tau _{\mathrm {nl}}\gg \tau _{\mathrm {A}}$ will be broken, so we end up with ![]() $\tau _{\mathrm {nl}} \sim \tau _{\mathrm {A}}$ and can return to considerations of the strong-turbulence regime, critical balance, etc. Numerically, this transition was first captured quite recently, by Meyrand et al. (Reference Meyrand, Galtier and Kiyani2016), whose result is shown in figure 3.
$\tau _{\mathrm {nl}} \sim \tau _{\mathrm {A}}$ and can return to considerations of the strong-turbulence regime, critical balance, etc. Numerically, this transition was first captured quite recently, by Meyrand et al. (Reference Meyrand, Galtier and Kiyani2016), whose result is shown in figure 3.

Figure 3. (Decaying) MHD simulation of transition from weak to strong turbulence by Meyrand, Galtier & Kiyani (Reference Meyrand, Galtier and Kiyani2016): the upper panel shows the magnetic spectrum vs. ![]() $k_{\parallel }$ and
$k_{\parallel }$ and ![]() $k_{\perp }$ (where
$k_{\perp }$ (where ![]() $k_{\parallel }$ is along the global mean field), the lower one the same integrated over
$k_{\parallel }$ is along the global mean field), the lower one the same integrated over ![]() $k_{\parallel }$ and normalised by
$k_{\parallel }$ and normalised by ![]() $k_{\perp }^{3/2}$ (see § 6 for why
$k_{\perp }^{3/2}$ (see § 6 for why ![]() $k_{\perp }^{-3/2}$ rather than
$k_{\perp }^{-3/2}$ rather than ![]() $k_{\perp }^{-5/3}$). A transition manifestly occurs from a
$k_{\perp }^{-5/3}$). A transition manifestly occurs from a ![]() $k_{\perp }^{-2}$ to a
$k_{\perp }^{-2}$ to a ![]() $k_{\perp }^{-3/2}$ spectrum and, simultaneously, from a state with no
$k_{\perp }^{-3/2}$ spectrum and, simultaneously, from a state with no ![]() $k_{\parallel }$ cascade (and a relatively narrow-band parallel spectrum) to one consistent with a CB cascade (2D spectra of CB turbulence are worked out in Appendix C). (Reprinted with permission from Meyrand et al. Reference Meyrand, Galtier and Kiyani2016, copyright (2016) by the American Physical Society.)
$k_{\parallel }$ cascade (and a relatively narrow-band parallel spectrum) to one consistent with a CB cascade (2D spectra of CB turbulence are worked out in Appendix C). (Reprinted with permission from Meyrand et al. Reference Meyrand, Galtier and Kiyani2016, copyright (2016) by the American Physical Society.)
The transition scale ![]() $\lambda _{\mathrm {CB}}$ is easy to estimate without the need for a specific WT theory. In view of (4.2), weak interactions cannot increase the characteristic parallel scale of the perturbations, which therefore remains
$\lambda _{\mathrm {CB}}$ is easy to estimate without the need for a specific WT theory. In view of (4.2), weak interactions cannot increase the characteristic parallel scale of the perturbations, which therefore remains ![]() $L_{\parallel }$. Then
$L_{\parallel }$. Then ![]() $\lambda _{\mathrm {CB}}$ is the perpendicular scale corresponding to
$\lambda _{\mathrm {CB}}$ is the perpendicular scale corresponding to ![]() $l_{\parallel }=L_{\parallel }$ in (2.11), viz.,
$l_{\parallel }=L_{\parallel }$ in (2.11), viz.,
 \begin{equation} \lambda_{\mathrm{CB}} \sim \varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2}. \end{equation}
\begin{equation} \lambda_{\mathrm{CB}} \sim \varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2}. \end{equation}
In fact, one does not even need to invoke the GS95 CB curve (2.11), because (4.3) is the only dimensionally correct possibility if one asks for a scale that depends on ![]() $\varepsilon$ and
$\varepsilon$ and ![]() $\tau _{\mathrm {A}}\sim L _{\parallel }/v_{\mathrm {A}}$ only; that
$\tau _{\mathrm {A}}\sim L _{\parallel }/v_{\mathrm {A}}$ only; that ![]() $L_{\parallel }$ and
$L_{\parallel }$ and ![]() $v_{\mathrm {A}}$ must enter in this combination follows from the fact that
$v_{\mathrm {A}}$ must enter in this combination follows from the fact that ![]() $\nabla _{\parallel }$ and
$\nabla _{\parallel }$ and ![]() $v_{\mathrm {A}}$ only enter multiplying each other in the RMHD equations (3.1).
$v_{\mathrm {A}}$ only enter multiplying each other in the RMHD equations (3.1).
A reader who is both convinced by this argument and regards it as grounds for dismissing the WT regime as asymptotically irrelevant, can at this point skip to § 5. The rest of this section is for those restless souls who insist on worrying about what happens in weakly forced systems at ![]() $\lambda \gg \lambda _{\mathrm {CB}}$.
$\lambda \gg \lambda _{\mathrm {CB}}$.
4.2. A sketch of WT theory
A very simple heuristic WT calculation (Ng & Bhattacharjee Reference Ng and Bhattacharjee1997; Goldreich & Sridhar Reference Goldreich and Sridhar1997) – a useful and physically transparent shortcut, and a good starting point for discussion – goes as follows.
Imagine two counterpropagating Alfvénic structures of perpendicular size ![]() $\lambda$ and parallel coherence length
$\lambda$ and parallel coherence length ![]() $L_{\parallel }$ (which cannot change in WT, as per the argument in § 4.1) passing through each other and interacting weakly. Their transit time through each other is
$L_{\parallel }$ (which cannot change in WT, as per the argument in § 4.1) passing through each other and interacting weakly. Their transit time through each other is ![]() $\tau _{\mathrm {A}}\sim L _{\parallel }/v_{\mathrm {A}}$ and the change in their amplitudes during this time is
$\tau _{\mathrm {A}}\sim L _{\parallel }/v_{\mathrm {A}}$ and the change in their amplitudes during this time is
 \begin{equation} \varDelta(\delta Z_\lambda^\pm) \sim \delta Z_\lambda^\pm\frac{\tau_{\mathrm{A}}}{\tau_{\mathrm{nl}}^\pm} \sim \frac{\delta Z^+_\lambda\delta Z^-_\lambda}{\lambda}\,\tau_{\mathrm{A}}, \end{equation}
\begin{equation} \varDelta(\delta Z_\lambda^\pm) \sim \delta Z_\lambda^\pm\frac{\tau_{\mathrm{A}}}{\tau_{\mathrm{nl}}^\pm} \sim \frac{\delta Z^+_\lambda\delta Z^-_\lambda}{\lambda}\,\tau_{\mathrm{A}}, \end{equation}
assuming ![]() $\tau _{\mathrm {nl}}^\pm \sim \lambda /\delta Z^\mp _\lambda$. By definition of the WT regime,
$\tau _{\mathrm {nl}}^\pm \sim \lambda /\delta Z^\mp _\lambda$. By definition of the WT regime, ![]() $\tau _{\mathrm {nl}}^\pm \gg \tau _{\mathrm {A}}$, so the amplitude change in any one interaction is small,
$\tau _{\mathrm {nl}}^\pm \gg \tau _{\mathrm {A}}$, so the amplitude change in any one interaction is small, ![]() $\varDelta (\delta Z_\lambda ^\pm ) \ll \delta Z_\lambda ^\pm$, and many such interactions are needed in order to change the amplitude
$\varDelta (\delta Z_\lambda ^\pm ) \ll \delta Z_\lambda ^\pm$, and many such interactions are needed in order to change the amplitude ![]() $\delta Z_\lambda ^\pm$ by an amount comparable to itself, i.e., to ‘cascade’ the energy associated with scale
$\delta Z_\lambda ^\pm$ by an amount comparable to itself, i.e., to ‘cascade’ the energy associated with scale ![]() $\lambda$ to smaller scales. Suppose that interactions occur all the time and that the kicks (4.4) accumulate as a random walk. Then the cascade time is
$\lambda$ to smaller scales. Suppose that interactions occur all the time and that the kicks (4.4) accumulate as a random walk. Then the cascade time is ![]() $\tau _{\mathrm {c}}^\pm = N\tau _{\mathrm {A}}$ if after
$\tau _{\mathrm {c}}^\pm = N\tau _{\mathrm {A}}$ if after ![]() $N$ interactions the amplitude change is of order
$N$ interactions the amplitude change is of order ![]() $\delta Z_\lambda ^\pm$:
$\delta Z_\lambda ^\pm$:
 \begin{equation} \varDelta(\delta Z_\lambda^\pm)\sqrt{N}\sim \delta Z_\lambda^\pm \quad\Rightarrow\quad \frac{\tau_{\mathrm{A}}}{\tau_{\mathrm{nl}}^\pm}\sqrt{\frac{\tau_{\mathrm{c}}^\pm}{\tau_{\mathrm{A}}}} \sim 1 \quad\Rightarrow\quad \tau_{\mathrm{c}}^\pm \sim \frac{(\tau_{\mathrm{nl}}^\pm)^2}{\tau_{\mathrm{A}}}. \end{equation}
\begin{equation} \varDelta(\delta Z_\lambda^\pm)\sqrt{N}\sim \delta Z_\lambda^\pm \quad\Rightarrow\quad \frac{\tau_{\mathrm{A}}}{\tau_{\mathrm{nl}}^\pm}\sqrt{\frac{\tau_{\mathrm{c}}^\pm}{\tau_{\mathrm{A}}}} \sim 1 \quad\Rightarrow\quad \tau_{\mathrm{c}}^\pm \sim \frac{(\tau_{\mathrm{nl}}^\pm)^2}{\tau_{\mathrm{A}}}. \end{equation}The standard Kolmogorov constant-flux requirement gives
Assuming for the moment that ![]() $\varepsilon ^+\sim \varepsilon ^-$ and, therefore,
$\varepsilon ^+\sim \varepsilon ^-$ and, therefore, ![]() $\delta Z_\lambda ^+\sim \delta Z_\lambda ^-$, gets us the classic WT scalingFootnote 10
$\delta Z_\lambda ^+\sim \delta Z_\lambda ^-$, gets us the classic WT scalingFootnote 10
 \begin{equation} \delta Z_\lambda \sim \left(\frac{\varepsilon}{\tau_{\mathrm{A}}}\right)^{1/4}\lambda^{1/2} \quad\Leftrightarrow\quad E(k_{{\perp}})\sim \left(\frac{\varepsilon}{\tau_{\mathrm{A}}}\right)^{1/2}k_{{\perp}}^{{-}2}. \end{equation}
\begin{equation} \delta Z_\lambda \sim \left(\frac{\varepsilon}{\tau_{\mathrm{A}}}\right)^{1/4}\lambda^{1/2} \quad\Leftrightarrow\quad E(k_{{\perp}})\sim \left(\frac{\varepsilon}{\tau_{\mathrm{A}}}\right)^{1/2}k_{{\perp}}^{{-}2}. \end{equation}This scaling is indeed what one finds numerically (see figures 3 and 4) – it was first confirmed in early, semidirect simulations by Ng & Bhattacharjee (Reference Ng and Bhattacharjee1997) and Bhattacharjee & Ng (Reference Bhattacharjee and Ng2001), and then definitively by Perez & Boldyrev (Reference Perez and Boldyrev2008) and Boldyrev & Perez (Reference Boldyrev and Perez2009), leading the community to tick off WT as done and dusted.

Figure 4. Kinetic (![]() $E$, solid lines) and magnetic (
$E$, solid lines) and magnetic (![]() $M$, dotted lines with crosses) energy spectra for
$M$, dotted lines with crosses) energy spectra for ![]() $k_{\parallel }=0$ (red),
$k_{\parallel }=0$ (red), ![]() $k_{\parallel }=2\pi /L_{\parallel }$ (blue) and
$k_{\parallel }=2\pi /L_{\parallel }$ (blue) and ![]() $k_{\parallel }=4\pi /L_{\parallel }$ (green) from an unpublished weak RMHD turbulence simulation by Yousef & Schekochihin (Reference Yousef and Schekochihin2009). The box size was
$k_{\parallel }=4\pi /L_{\parallel }$ (green) from an unpublished weak RMHD turbulence simulation by Yousef & Schekochihin (Reference Yousef and Schekochihin2009). The box size was ![]() $(L_{\perp },L_{\parallel })$ in the perpendicular and parallel directions, respectively, and the forcing was narrow-band, at
$(L_{\perp },L_{\parallel })$ in the perpendicular and parallel directions, respectively, and the forcing was narrow-band, at ![]() $k_{\parallel }=2\pi /L_{\parallel }$ and
$k_{\parallel }=2\pi /L_{\parallel }$ and ![]() $k_{\perp } = (1,2)\times 2\pi /L_{\perp }$, deep in the WT regime (
$k_{\perp } = (1,2)\times 2\pi /L_{\perp }$, deep in the WT regime (![]() $L_{\perp }\gg \lambda _{\mathrm {CB}}$). WT spectra for the case of broad-band forcing can be found in Perez & Boldyrev (Reference Perez and Boldyrev2008) and Boldyrev & Perez (Reference Boldyrev and Perez2009) and are discussed in Appendix A.4.
$L_{\perp }\gg \lambda _{\mathrm {CB}}$). WT spectra for the case of broad-band forcing can be found in Perez & Boldyrev (Reference Perez and Boldyrev2008) and Boldyrev & Perez (Reference Boldyrev and Perez2009) and are discussed in Appendix A.4.
As anticipated in § 4.1, with the scaling (4.7), the ratio of the time scales can only stay small above a certain finite scale:
where ![]() $\lambda _{\mathrm {CB}}$ is transition scale anticipated in (4.3). For
$\lambda _{\mathrm {CB}}$ is transition scale anticipated in (4.3). For ![]() $\lambda \lesssim \lambda _{\mathrm {CB}}$, turbulence becomes strong and, presumably, critically balanced. Thus, the WT cascade, by transferring energy to smaller scales, where nonlinear times are shorter, saws the seeds of its own destruction.
$\lambda \lesssim \lambda _{\mathrm {CB}}$, turbulence becomes strong and, presumably, critically balanced. Thus, the WT cascade, by transferring energy to smaller scales, where nonlinear times are shorter, saws the seeds of its own destruction.
4.3. Imbalanced WT
What if ![]() $\varepsilon ^+\neq \varepsilon ^-$, viz., say,
$\varepsilon ^+\neq \varepsilon ^-$, viz., say, ![]() $\varepsilon ^+\gg \varepsilon ^-$? (If
$\varepsilon ^+\gg \varepsilon ^-$? (If ![]() $\varepsilon ^+>\varepsilon ^-$ but both are of the same order, arguably the results obtained for
$\varepsilon ^+>\varepsilon ^-$ but both are of the same order, arguably the results obtained for ![]() $\varepsilon ^+\sim \varepsilon ^-$ should still work, at least on the ‘twiddle’ level.) Alas, (4.6) is patently incapable of accommodating such a case, an embarrassment first noticed by Dobrowolny, Mangeney & Veltri (Reference Dobrowolny, Mangeney and Veltri1980), who were attempting an IK-style, isotropic (
$\varepsilon ^+\sim \varepsilon ^-$ should still work, at least on the ‘twiddle’ level.) Alas, (4.6) is patently incapable of accommodating such a case, an embarrassment first noticed by Dobrowolny, Mangeney & Veltri (Reference Dobrowolny, Mangeney and Veltri1980), who were attempting an IK-style, isotropic (![]() $L_{\parallel }\sim \lambda$), imbalanced theory – quite wrong, as we now know (§ 2.3), but they correctly identified the issue with the imbalanced regime. They concluded that no imbalanced stationary state was possible except a pure Elsasser state. This may be true for (certain types of) decaying turbulence (see § 12.7), but is certainly not a satisfactory conclusion for a forced case where
$L_{\parallel }\sim \lambda$), imbalanced theory – quite wrong, as we now know (§ 2.3), but they correctly identified the issue with the imbalanced regime. They concluded that no imbalanced stationary state was possible except a pure Elsasser state. This may be true for (certain types of) decaying turbulence (see § 12.7), but is certainly not a satisfactory conclusion for a forced case where ![]() $\varepsilon ^\pm$ are externally prescribed. Arguably, the inability to describe the imbalanced case casts a shadow of doubt also on the validity of the argument in § 4.2 for balanced WT, as introducing the imbalance just lifts a kind of ‘degeneracy’ and perhaps highlights a problem with the whole story.
$\varepsilon ^\pm$ are externally prescribed. Arguably, the inability to describe the imbalanced case casts a shadow of doubt also on the validity of the argument in § 4.2 for balanced WT, as introducing the imbalance just lifts a kind of ‘degeneracy’ and perhaps highlights a problem with the whole story.
A way out of this difficulty, various versions of which have been explored by Galtier et al. (Reference Galtier, Nazarenko, Newell and Pouquet2000), Lithwick & Goldreich (Reference Lithwick and Goldreich2003), and Chandran (Reference Chandran2008), is to accept (4.6) but notice that it allows the two Elsasser fields to have different scaling exponents, ![]() $\delta Z^\pm _\lambda \propto \lambda ^{\gamma ^\pm }$, as long as they satisfy
$\delta Z^\pm _\lambda \propto \lambda ^{\gamma ^\pm }$, as long as they satisfy ![]() $\gamma ^+ + \gamma ^- = 1$. The corresponding 2D spectra of the two fields are
$\gamma ^+ + \gamma ^- = 1$. The corresponding 2D spectra of the two fields are
because ![]() $\mu ^\pm = -2\gamma ^\pm -1$ and, WT permitting no changes in
$\mu ^\pm = -2\gamma ^\pm -1$ and, WT permitting no changes in ![]() $k_{\parallel }$, the scaling arguments of § 4.2 apply to each
$k_{\parallel }$, the scaling arguments of § 4.2 apply to each ![]() $k_{\parallel }$ individually. One may then declare that the difference between
$k_{\parallel }$ individually. One may then declare that the difference between ![]() $\varepsilon ^+$ and
$\varepsilon ^+$ and ![]() $\varepsilon ^-$ is hidden in the prefactors
$\varepsilon ^-$ is hidden in the prefactors ![]() $f^\pm (k_{\parallel })$, which are non-universal, inaccessible to ‘twiddle’ scaling arguments about local interactions in the WT inertial range, and have to be fixed from outside it. At the large-scale end, one has to decide whether the outer scales for the two Elsasser fields are the same or different (Chandran Reference Chandran2008) and whether it is the fluxes
$f^\pm (k_{\parallel })$, which are non-universal, inaccessible to ‘twiddle’ scaling arguments about local interactions in the WT inertial range, and have to be fixed from outside it. At the large-scale end, one has to decide whether the outer scales for the two Elsasser fields are the same or different (Chandran Reference Chandran2008) and whether it is the fluxes ![]() $\varepsilon ^\pm$ or the fields’ energies at the outer scale(s) that it makes better sense to consider prescribed. At the dissipation scale, one has the option of ‘pinning’ the spectra to the same value (an idea due to Grappin, Leorat & Pouquet Reference Grappin, Leorat and Pouquet1983 and revived by Lithwick & Goldreich Reference Lithwick and Goldreich2003), and it must also be decided whether the two fields are required to start feeling viscosity at the same scale or one can do so before the other (see discussion in Beresnyak & Lazarian Reference Beresnyak and Lazarian2008, and, for strong imbalanced turbulence, in § 9.6.4). If WT breaks down before the dissipation scale is reached, some other set of ad hoc arrangements is required (see, e.g., Chandran Reference Chandran2008). Typically, the outcome is that the stronger field has a steeper spectrum than the weaker field, but their scalings are non-universal, i.e., they depend on the particular set up of the problem, at both macro- and micro-scales.
$\varepsilon ^\pm$ or the fields’ energies at the outer scale(s) that it makes better sense to consider prescribed. At the dissipation scale, one has the option of ‘pinning’ the spectra to the same value (an idea due to Grappin, Leorat & Pouquet Reference Grappin, Leorat and Pouquet1983 and revived by Lithwick & Goldreich Reference Lithwick and Goldreich2003), and it must also be decided whether the two fields are required to start feeling viscosity at the same scale or one can do so before the other (see discussion in Beresnyak & Lazarian Reference Beresnyak and Lazarian2008, and, for strong imbalanced turbulence, in § 9.6.4). If WT breaks down before the dissipation scale is reached, some other set of ad hoc arrangements is required (see, e.g., Chandran Reference Chandran2008). Typically, the outcome is that the stronger field has a steeper spectrum than the weaker field, but their scalings are non-universal, i.e., they depend on the particular set up of the problem, at both macro- and micro-scales.
Another possibility is that (4.6) is wrong. Let me observe that the balanced (![]() $\varepsilon ^+\sim \varepsilon ^-$,
$\varepsilon ^+\sim \varepsilon ^-$, ![]() $\delta Z^+_\lambda \sim \delta Z^-_\lambda$) version of this scaling, i.e., the statement that the flux
$\delta Z^+_\lambda \sim \delta Z^-_\lambda$) version of this scaling, i.e., the statement that the flux ![]() $\varepsilon$ is proportional to the fourth power of the amplitude, is less likely to be wrong than any particular assignment of ‘
$\varepsilon$ is proportional to the fourth power of the amplitude, is less likely to be wrong than any particular assignment of ‘![]() $+$'s and ‘
$+$'s and ‘![]() $-$’s to these amplitudes: all it says is that the flux
$-$’s to these amplitudes: all it says is that the flux ![]() $\varepsilon$ is what it would have been in the case of strong interactions,
$\varepsilon$ is what it would have been in the case of strong interactions, ![]() $\sim \delta Z_\lambda ^2/\tau _{\mathrm {nl}}$ (cf. (2.9)), times the first power of the expansion parameter
$\sim \delta Z_\lambda ^2/\tau _{\mathrm {nl}}$ (cf. (2.9)), times the first power of the expansion parameter ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$, i.e., the lowest order that
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$, i.e., the lowest order that ![]() $\varepsilon$ can be in a perturbation expansion in that parameter. Thus, one may doubt the validity of (4.6) for the imbalanced regime without rejecting the numerically confirmed
$\varepsilon$ can be in a perturbation expansion in that parameter. Thus, one may doubt the validity of (4.6) for the imbalanced regime without rejecting the numerically confirmed ![]() $k_{\perp }^{-2}$ scaling of the balanced spectra. For example, in the (heuristic) scheme proposed by Schekochihin, Nazarenko & Yousef (Reference Schekochihin, Nazarenko and Yousef2012),
$k_{\perp }^{-2}$ scaling of the balanced spectra. For example, in the (heuristic) scheme proposed by Schekochihin, Nazarenko & Yousef (Reference Schekochihin, Nazarenko and Yousef2012),
which changes nothing for balanced WT, but leads to a very different situation in the imbalanced case than (4.6), allowing perfectly good ![]() $k_{\perp }^{-2}$ spectra for both fields.
$k_{\perp }^{-2}$ spectra for both fields.
I do not go through all this in detail because, the WT regime being largely irrelevant (§ 4.1), it would also, if it really were non-universal, not be very interesting. If it is universal and something like (4.10) holds, that is interesting, but I do not know how to make much progress beyond Schekochihin et al. (Reference Schekochihin, Nazarenko and Yousef2012), whose theory does not quite match simulations (see § 4.4). I also do not know how to construct a theory of imbalanced WT that would connect smoothly to any believable theory of strong imbalanced turbulence (e.g., one presented in § 9.6). An interested reader will find some further, equally unsatisfactory, observations in Appendix A.6.
4.4. 2D condensate
It follows from the discussion in § 4.1 that the WT approximation in its standard form cannot, in fact, work for the turbulence of Alfvén waves, at least not formally, because in every three-wave interaction, one of the three waves has ![]() $k_{\parallel }=0$, so is not a wave at all, but a zero-frequency 2D perturbation, for which the nonlinear interactions are the dominant influence. If such
$k_{\parallel }=0$, so is not a wave at all, but a zero-frequency 2D perturbation, for which the nonlinear interactions are the dominant influence. If such ![]() $k_{\parallel }=0$ perturbations are forbidden, i.e., if displacements vanish at infinity, one must consider four-wave interactions (i.e., go to next order in
$k_{\parallel }=0$ perturbations are forbidden, i.e., if displacements vanish at infinity, one must consider four-wave interactions (i.e., go to next order in ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$), which gives rise to an apparently legitimate WT state, different from (4.7) (Sridhar & Goldreich Reference Sridhar and Goldreich1994). There is no particular reason to think, however, that such a restriction on displacements is legitimate in a general physical situation (Ng & Bhattacharjee Reference Ng and Bhattacharjee1996) or, even if one starts with no energy at
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$), which gives rise to an apparently legitimate WT state, different from (4.7) (Sridhar & Goldreich Reference Sridhar and Goldreich1994). There is no particular reason to think, however, that such a restriction on displacements is legitimate in a general physical situation (Ng & Bhattacharjee Reference Ng and Bhattacharjee1996) or, even if one starts with no energy at ![]() $k_{\parallel }=0$, that such a state can be maintained, except in a box with field lines nailed down at the boundaries – failing such restrictions, a 2D ‘condensate’ must emerge (and does, in numerical simulations: see Boldyrev & Perez Reference Boldyrev and Perez2009, Wang, Boldyrev & Perez Reference Wang, Boldyrev and Perez2011, Meyrand, Kiyani & Galtier Reference Meyrand, Kiyani and Galtier2015; Meyrand et al. Reference Meyrand, Galtier and Kiyani2016, and figure 4).
$k_{\parallel }=0$, that such a state can be maintained, except in a box with field lines nailed down at the boundaries – failing such restrictions, a 2D ‘condensate’ must emerge (and does, in numerical simulations: see Boldyrev & Perez Reference Boldyrev and Perez2009, Wang, Boldyrev & Perez Reference Wang, Boldyrev and Perez2011, Meyrand, Kiyani & Galtier Reference Meyrand, Kiyani and Galtier2015; Meyrand et al. Reference Meyrand, Galtier and Kiyani2016, and figure 4).
Mathematically, this becomes quite obvious if we represent the solutions to (3.1) as
and separate the ![]() $k_{\parallel }=0$ modes from the rest:
$k_{\parallel }=0$ modes from the rest:
where ![]() $\hat {{\mathcal {P}}}$ is the projection operator that takes care of the pressure term (see (3.2)) and has been introduced for brevity; forcing and dissipation terms have been dropped. The first of these equations, (4.12), describes the condensate – two real fields
$\hat {{\mathcal {P}}}$ is the projection operator that takes care of the pressure term (see (3.2)) and has been introduced for brevity; forcing and dissipation terms have been dropped. The first of these equations, (4.12), describes the condensate – two real fields ![]() $\boldsymbol {Z}_0^\pm (x,y)$ advecting each other in the 2D plane while subject to an oscillating ‘force’ due to the mutual coupling of the Alfvén waves
$\boldsymbol {Z}_0^\pm (x,y)$ advecting each other in the 2D plane while subject to an oscillating ‘force’ due to the mutual coupling of the Alfvén waves ![]() $\boldsymbol {Z}^\pm _{k_{\parallel }}$. These Alfvén waves, described by (4.13), are advected by the 2D field and also by each other, but the latter interaction has an oscillating factor and vanishes in the WT approximation. Even if only the Alfvén waves are forced and the condensate is not, the condensate will nevertheless be built up.
$\boldsymbol {Z}^\pm _{k_{\parallel }}$. These Alfvén waves, described by (4.13), are advected by the 2D field and also by each other, but the latter interaction has an oscillating factor and vanishes in the WT approximation. Even if only the Alfvén waves are forced and the condensate is not, the condensate will nevertheless be built up.
Returning to three-wave interactions then (where one of the waves is not a wave), the traditional approach has been to ignore the inapplicability of the WT approximation to the ![]() $k_{\parallel }=0$ modes by conjecturing that the function
$k_{\parallel }=0$ modes by conjecturing that the function ![]() $f^\pm (k_{\parallel })$ in (4.9) is flat around
$f^\pm (k_{\parallel })$ in (4.9) is flat around ![]() $k_{\parallel }=0$ – the hypothesis of ‘spectral continuity’. One can then press on with putting MHD through the WT analytical grinder, find an evolution equation for the spectra and show that it has steady-state, constant-flux solutions of the form (4.9). This is what was done in the now-classic paper by Galtier et al. (Reference Galtier, Nazarenko, Newell and Pouquet2000) (see Appendices A.2 and A.3). In balanced turbulence, obviously,
$k_{\parallel }=0$ – the hypothesis of ‘spectral continuity’. One can then press on with putting MHD through the WT analytical grinder, find an evolution equation for the spectra and show that it has steady-state, constant-flux solutions of the form (4.9). This is what was done in the now-classic paper by Galtier et al. (Reference Galtier, Nazarenko, Newell and Pouquet2000) (see Appendices A.2 and A.3). In balanced turbulence, obviously, ![]() $\mu ^+=\mu ^-=2$, and we are back to (4.7); in imbalanced turbulence, one needs further scheming – see references in § 4.3.
$\mu ^+=\mu ^-=2$, and we are back to (4.7); in imbalanced turbulence, one needs further scheming – see references in § 4.3.
Nazarenko (Reference Nazarenko2007) argues that the hypothesis of spectral continuity is certainly false if the nonlinear broadening of the waves’ frequencies, of order ![]() $\tau _{\mathrm {nl}}^{-1}$, is smaller than the linear frequency associated with the spacing of the
$\tau _{\mathrm {nl}}^{-1}$, is smaller than the linear frequency associated with the spacing of the ![]() $k_{\parallel }$ ‘grid’ (
$k_{\parallel }$ ‘grid’ (![]() $=2\pi /L_{\parallel }$, the inverse parallel ‘box’ size) – i.e., if the Alfvénic perturbations at the longest finite parallel scale in the system are already in the WT limit (4.1),
$=2\pi /L_{\parallel }$, the inverse parallel ‘box’ size) – i.e., if the Alfvénic perturbations at the longest finite parallel scale in the system are already in the WT limit (4.1), ![]() $v_{\mathrm {A}}/L_{\parallel }\gg \tau _{\mathrm {nl}}^{-1}$. He is right. Figure 4 is taken from a (sadly, unpublished) numerical study of weak RMHD turbulence by Yousef & Schekochihin (Reference Yousef and Schekochihin2009), who forced Alfvén waves at
$v_{\mathrm {A}}/L_{\parallel }\gg \tau _{\mathrm {nl}}^{-1}$. He is right. Figure 4 is taken from a (sadly, unpublished) numerical study of weak RMHD turbulence by Yousef & Schekochihin (Reference Yousef and Schekochihin2009), who forced Alfvén waves at ![]() $k_{\parallel } = 2\pi /L_{\parallel }$, where
$k_{\parallel } = 2\pi /L_{\parallel }$, where ![]() $L_{\parallel }$ was the box size. It shows that, while the
$L_{\parallel }$ was the box size. It shows that, while the ![]() $k_{\perp }^{-2}$ scaling of the
$k_{\perp }^{-2}$ scaling of the ![]() $k_{\parallel }=2\pi /L_{\parallel }$ modes is undeniable, the spectra for the unforced modes (
$k_{\parallel }=2\pi /L_{\parallel }$ modes is undeniable, the spectra for the unforced modes (![]() $k_{\parallel } = 0$ and
$k_{\parallel } = 0$ and ![]() $k_{\parallel } =$ multiples of
$k_{\parallel } =$ multiples of ![]() $2\pi /L_{\parallel }$) are dramatically shallower. Similar spectra were reported by Bigot & Galtier (Reference Bigot and Galtier2011) and by Meyrand et al. (Reference Meyrand, Kiyani and Galtier2015). Qualitatively similar spectra (and a simple mechanism for how they might form) were also proposed by Schekochihin et al. (Reference Schekochihin, Nazarenko and Yousef2012) – but their theory fails quantitatively, with the spectra that it predicts for all unforced modes at least one power of
$2\pi /L_{\parallel }$) are dramatically shallower. Similar spectra were reported by Bigot & Galtier (Reference Bigot and Galtier2011) and by Meyrand et al. (Reference Meyrand, Kiyani and Galtier2015). Qualitatively similar spectra (and a simple mechanism for how they might form) were also proposed by Schekochihin et al. (Reference Schekochihin, Nazarenko and Yousef2012) – but their theory fails quantitatively, with the spectra that it predicts for all unforced modes at least one power of ![]() $k_{\perp }$ steeper than the numerical ones (e.g., their
$k_{\perp }$ steeper than the numerical ones (e.g., their ![]() $k_{\parallel }=0$ condensate has a
$k_{\parallel }=0$ condensate has a ![]() $\propto k_{\perp }^{-1}$ spectrum, while simulations suggest
$\propto k_{\perp }^{-1}$ spectrum, while simulations suggest ![]() $\propto k _{\perp }^0$).
$\propto k _{\perp }^0$).
Nazarenko (Reference Nazarenko2007) expects that the conventional WT theory should survive when ![]() $k_{\parallel} v _{\mathrm {A}}\gg \tau _{\mathrm {nl}}^{-1}\gg v _{\mathrm {A}}/L_{\parallel }$. This is a situation that should be realisable in a system that is weakly and randomly forced in a broad band of frequencies (and, therefore, parallel wavenumbers). In Appendix A.4, I discuss how, and in what sense, one might defend spectral continuity for such a system; I argue that the 2D condensate in this case is a strongly turbulent, critically balanced subsystem constantly fed by the weakly turbulent waves and developing a falsifiable set of scalings, which are, indeed, continuous with the WT scalings. While there are some indications (from the simulations by Wang et al. Reference Wang, Boldyrev and Perez2011; see Appendix A.5) that these scalings might be right, I have not seen spectral continuity corroborated numerically in a definitive fashion, as even Perez & Boldyrev (Reference Perez and Boldyrev2008) and Boldyrev & Perez (Reference Boldyrev and Perez2009), who took great care to force in a broad band of
$k_{\parallel} v _{\mathrm {A}}\gg \tau _{\mathrm {nl}}^{-1}\gg v _{\mathrm {A}}/L_{\parallel }$. This is a situation that should be realisable in a system that is weakly and randomly forced in a broad band of frequencies (and, therefore, parallel wavenumbers). In Appendix A.4, I discuss how, and in what sense, one might defend spectral continuity for such a system; I argue that the 2D condensate in this case is a strongly turbulent, critically balanced subsystem constantly fed by the weakly turbulent waves and developing a falsifiable set of scalings, which are, indeed, continuous with the WT scalings. While there are some indications (from the simulations by Wang et al. Reference Wang, Boldyrev and Perez2011; see Appendix A.5) that these scalings might be right, I have not seen spectral continuity corroborated numerically in a definitive fashion, as even Perez & Boldyrev (Reference Perez and Boldyrev2008) and Boldyrev & Perez (Reference Boldyrev and Perez2009), who took great care to force in a broad band of ![]() $k_{\parallel }$ to make sure the conventional WT theory did apply, saw a distinct dip in
$k_{\parallel }$ to make sure the conventional WT theory did apply, saw a distinct dip in ![]() $f^\pm (k_{\parallel })$ at
$f^\pm (k_{\parallel })$ at ![]() $k_{\parallel } = 0$, associated with an emergent condensate (which is magnetically dominated; see § 10.3 and Appendix A.5). The same was true in the decaying simulations of ?see the upper panel of figure 3) meyrand15,meyrand16, where an initial small-amplitude (and so WT-compliant) state had the choice to evolve towards a continuous parallel spectrum, but refused to do so, again developing a
$k_{\parallel } = 0$, associated with an emergent condensate (which is magnetically dominated; see § 10.3 and Appendix A.5). The same was true in the decaying simulations of ?see the upper panel of figure 3) meyrand15,meyrand16, where an initial small-amplitude (and so WT-compliant) state had the choice to evolve towards a continuous parallel spectrum, but refused to do so, again developing a ![]() $k_{\parallel }=0$ condensate with dramatically distinct properties, including a high degree of intermittency and a spectrum quite similar to figure 4.
$k_{\parallel }=0$ condensate with dramatically distinct properties, including a high degree of intermittency and a spectrum quite similar to figure 4.
Thus, the conventional WT theory is at best incomplete and at worst wrong. It is discussed further in Appendix A, where I review the WT's derivation, speculate about the structure of the condensate, and discuss a number of other WT-related issues. Here, having flagged these issues, I want to halt this digression into matters that are, arguably, of little impact, and move on to the physics-rich core of the MHD-turbulence theory.
5. Critical balance, parallel cascade, and anisotropy
5.1. Critical balance
Section 4 can be viewed as one long protracted justificatory piece in favour of critical balance: even if an ensemble of high-frequency Alfvén waves is stirred up very gently (![]() $\tau _{\mathrm {nl}}\gg \tau _{\mathrm {A}}$), it will, at small enough scales, get itself into the strong-turbulence regime (
$\tau _{\mathrm {nl}}\gg \tau _{\mathrm {A}}$), it will, at small enough scales, get itself into the strong-turbulence regime (![]() $\tau _{\mathrm {nl}}\sim \tau _{\mathrm {A}}$). The opposite limit, a 2D regime with
$\tau _{\mathrm {nl}}\sim \tau _{\mathrm {A}}$). The opposite limit, a 2D regime with ![]() $\tau _{\mathrm {nl}}\ll \tau _{\mathrm {A}}$, is unsustainable for the very simple reason of causality: as information in an RMHD system propagates along
$\tau _{\mathrm {nl}}\ll \tau _{\mathrm {A}}$, is unsustainable for the very simple reason of causality: as information in an RMHD system propagates along ![]() $\boldsymbol {B}_0$ at speed
$\boldsymbol {B}_0$ at speed ![]() $v_{\mathrm {A}}$, no structure longer than
$v_{\mathrm {A}}$, no structure longer than ![]() $l_{\parallel }\sim v _{\mathrm {A}}\tau _{\mathrm {nl}}$ can be kept coherent and so will break up (see Boldyrev Reference Boldyrev2005, Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011 and figure 5).
$l_{\parallel }\sim v _{\mathrm {A}}\tau _{\mathrm {nl}}$ can be kept coherent and so will break up (see Boldyrev Reference Boldyrev2005, Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011 and figure 5).

Figure 5. Critical balance in a (![]() $2+1$)D system supporting both nonlinearity and waves (RMHD).
$2+1$)D system supporting both nonlinearity and waves (RMHD).
It is worth mentioning in passing that the CB turns out to be a very robust feature of the turbulence in the following interesting sense. With a certain appropriate definition of ![]() $\tau _{\mathrm {nl}}$ (which will be explained in § 6.1), the ratio
$\tau _{\mathrm {nl}}$ (which will be explained in § 6.1), the ratio ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$ has been found (numerically) by Mallet et al. (Reference Mallet, Schekochihin and Chandran2015) to have a scale-invariant distribution (figure 6), a property that they dubbed refined critical balance (RCB). It gives a quantitative meaning to the somewhat vague statement
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}$ has been found (numerically) by Mallet et al. (Reference Mallet, Schekochihin and Chandran2015) to have a scale-invariant distribution (figure 6), a property that they dubbed refined critical balance (RCB). It gives a quantitative meaning to the somewhat vague statement ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}\sim 1$ – and becomes important in the (as it turns out, unavoidable) discussion of intermittency of MHD turbulence (§ 6.4.2).
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}\sim 1$ – and becomes important in the (as it turns out, unavoidable) discussion of intermittency of MHD turbulence (§ 6.4.2).

Figure 6. Refined critical balance: this figure, taken from Mallet et al. (Reference Mallet, Schekochihin and Chandran2015), shows the probability density function (PDF) of the ratio ![]() $\chi ^+=\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}^+$ with
$\chi ^+=\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}^+$ with ![]() $\tau _{\mathrm {nl}}^+$ defined by (6.4). In fact, 17 PDFs are plotted here, taken at different scales within an approximately decade-wide inertial range (this was a
$\tau _{\mathrm {nl}}^+$ defined by (6.4). In fact, 17 PDFs are plotted here, taken at different scales within an approximately decade-wide inertial range (this was a ![]() $1024^3$ RMHD simulation) – the corresponding lines are in colour shades from blue (smaller scales) to red (larger scales), but this is barely visible because the PDFs all collapse on top of each other. The inset shows that the self-similarity does not work if
$1024^3$ RMHD simulation) – the corresponding lines are in colour shades from blue (smaller scales) to red (larger scales), but this is barely visible because the PDFs all collapse on top of each other. The inset shows that the self-similarity does not work if ![]() $\tau _{\mathrm {nl}}^+$ is defined without the alignment angle (see § 6). (Reprinted from Mallet et al. Reference Mallet, Schekochihin and Chandran2015 by permission of the Royal Astronomical Society.)
$\tau _{\mathrm {nl}}^+$ is defined without the alignment angle (see § 6). (Reprinted from Mallet et al. Reference Mallet, Schekochihin and Chandran2015 by permission of the Royal Astronomical Society.)
5.2. Parallel cascade
The most straightforward – and the least controversial – consequence of CB is the scaling of parallel increments. I have already derived this result in (2.10), but let me now restate it using Elsasser fields. If it is the case that the nonlinear-interaction time and, therefore, the cascade time for ![]() $\boldsymbol {Z}_{\perp }^\pm$ are approximately the same as their propagation time
$\boldsymbol {Z}_{\perp }^\pm$ are approximately the same as their propagation time ![]() $\tau _{\mathrm {A}}\sim l _{\parallel }/v_{\mathrm {A}}$, then the parallel increments
$\tau _{\mathrm {A}}\sim l _{\parallel }/v_{\mathrm {A}}$, then the parallel increments ![]() $\delta Z_{l_{\parallel }}^\pm$ satisfy
$\delta Z_{l_{\parallel }}^\pm$ satisfy
 \begin{equation} \frac{(\delta Z_{l_{{\parallel}}}^\pm)^2}{\tau_{\mathrm{A}}} \sim \varepsilon^\pm\quad\Rightarrow\quad \delta Z^\pm_{l_{{\parallel}}} \sim \left(\frac{\varepsilon^\pm l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2} \quad\Leftrightarrow\quad E^\pm(k_{{\parallel}}) \sim \frac{\varepsilon^\pm}{v_{\mathrm{A}}}k_{{\parallel}}^{{-}2}. \end{equation}
\begin{equation} \frac{(\delta Z_{l_{{\parallel}}}^\pm)^2}{\tau_{\mathrm{A}}} \sim \varepsilon^\pm\quad\Rightarrow\quad \delta Z^\pm_{l_{{\parallel}}} \sim \left(\frac{\varepsilon^\pm l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2} \quad\Leftrightarrow\quad E^\pm(k_{{\parallel}}) \sim \frac{\varepsilon^\pm}{v_{\mathrm{A}}}k_{{\parallel}}^{{-}2}. \end{equation} Beresnyak (Reference Beresnyak2012a, Reference Beresnyak2015) gives two rather elegant (and related) arguments in favour of the scaling (5.1), alongside robust numerical evidence presented in the latter paper.Footnote 11 First, he argues that the scaling relation (5.1) can be obtained by dimensional analysis because the RMHD equations (3.1) stay invariant if ![]() $v_{\mathrm {A}}$ and
$v_{\mathrm {A}}$ and ![]() $1/k_{\parallel }$ are scaled simultaneously (see (3.5)) and so these two quantities must always appear in the combination
$1/k_{\parallel }$ are scaled simultaneously (see (3.5)) and so these two quantities must always appear in the combination ![]() $k_{\parallel} v _{\mathrm {A}}$ in scaling relations for any physical quantities – in the case of (5.1), energy, or field increment. Secondly, Beresnyak (Reference Beresnyak2015) notes that following the structure of the fluctuating field (calculating its increments) along the field line (in the positive
$k_{\parallel} v _{\mathrm {A}}$ in scaling relations for any physical quantities – in the case of (5.1), energy, or field increment. Secondly, Beresnyak (Reference Beresnyak2015) notes that following the structure of the fluctuating field (calculating its increments) along the field line (in the positive ![]() $\boldsymbol {B}_0$ direction) is the MHD equivalent of following its evolution forward (for
$\boldsymbol {B}_0$ direction) is the MHD equivalent of following its evolution forward (for ![]() $\boldsymbol {Z}_{\perp }^-$) or backward (for
$\boldsymbol {Z}_{\perp }^-$) or backward (for ![]() $\boldsymbol {Z}_{\perp }^+$) in time and it should, therefore, be possible to infer the parallel spectrum (5.1) from the Lagrangian frequency spectrum of the turbulence (as opposed to the Eulerian one, which is dominated by large-scale sweeping effects: see Lugones et al. Reference Lugones, Dmitruk, Mininni, Wan and Matthaeus2016, Reference Lugones, Dmitruk, Mininni, Pouquet and Matthaeus2019). Estimating the energy flux as the rate of change of energy in a fluid element in the Lagrangian frame (i.e., excluding sweeping by large eddies), one obtains (Landau & Lifshitz Reference Landau and Lifshitz1987; Corrsin Reference Corrsin1963)
$\boldsymbol {Z}_{\perp }^+$) in time and it should, therefore, be possible to infer the parallel spectrum (5.1) from the Lagrangian frequency spectrum of the turbulence (as opposed to the Eulerian one, which is dominated by large-scale sweeping effects: see Lugones et al. Reference Lugones, Dmitruk, Mininni, Wan and Matthaeus2016, Reference Lugones, Dmitruk, Mininni, Pouquet and Matthaeus2019). Estimating the energy flux as the rate of change of energy in a fluid element in the Lagrangian frame (i.e., excluding sweeping by large eddies), one obtains (Landau & Lifshitz Reference Landau and Lifshitz1987; Corrsin Reference Corrsin1963)
where ![]() $\delta Z_\tau ^\pm$ is the Lagrangian field increment over time interval
$\delta Z_\tau ^\pm$ is the Lagrangian field increment over time interval ![]() $\tau$. Then (5.1) is recovered from (5.2) by changing variables
$\tau$. Then (5.1) is recovered from (5.2) by changing variables ![]() $\omega =k_{\parallel} v _{\mathrm {A}}$ and letting
$\omega =k_{\parallel} v _{\mathrm {A}}$ and letting ![]() $E^\pm (\omega )\mathrm {d}\omega = E^\pm (k_{\parallel })\mathrm {d}k_{\parallel }$.
$E^\pm (\omega )\mathrm {d}\omega = E^\pm (k_{\parallel })\mathrm {d}k_{\parallel }$.
Thus, the parallel cascade and the associated scaling (5.1) appear to be a very simple and solid property of MHD turbulence. What happens in the perpendicular direction is a more complicated story.
5.3. Local, scale-dependent anisotropy
Using instead of the parallel increments the perpendicular ones ![]() $\delta Z^\pm _\lambda$ and substituting the nonlinear time
$\delta Z^\pm _\lambda$ and substituting the nonlinear time
for the cascade time, we recover (2.9):Footnote 12
 \begin{align} \frac{(\delta Z_\lambda^\pm)^2}{\tau_{\mathrm{nl}}^\pm} \sim \varepsilon^\pm &\quad\Rightarrow\quad \frac{\delta Z_\lambda^+}{\delta Z_\lambda^-}\sim\frac{\varepsilon^+}{\varepsilon^-},\quad \delta Z^\pm_\lambda \sim (\tilde\varepsilon^\pm\lambda)^{1/3}, \quad \tilde\varepsilon^\pm\equiv \frac{(\varepsilon^\pm)^2}{\varepsilon^\mp} \nonumber\\ &\quad\Rightarrow\quad E^\pm(k_{{\perp}}) \sim (\tilde\varepsilon^\pm)^{2/3}k_{{\perp}}^{{-}5/3}. \end{align}
\begin{align} \frac{(\delta Z_\lambda^\pm)^2}{\tau_{\mathrm{nl}}^\pm} \sim \varepsilon^\pm &\quad\Rightarrow\quad \frac{\delta Z_\lambda^+}{\delta Z_\lambda^-}\sim\frac{\varepsilon^+}{\varepsilon^-},\quad \delta Z^\pm_\lambda \sim (\tilde\varepsilon^\pm\lambda)^{1/3}, \quad \tilde\varepsilon^\pm\equiv \frac{(\varepsilon^\pm)^2}{\varepsilon^\mp} \nonumber\\ &\quad\Rightarrow\quad E^\pm(k_{{\perp}}) \sim (\tilde\varepsilon^\pm)^{2/3}k_{{\perp}}^{{-}5/3}. \end{align}
Treating ![]() $\delta Z^\pm _\lambda$ and
$\delta Z^\pm _\lambda$ and ![]() $\delta Z^\pm _{l_{\parallel }}$ as increments for the same structure, but measured across and along the field, and setting them equal to each other, we find a relationship between the parallel and perpendicular scales – the scale-dependent anisotropy (2.11):
$\delta Z^\pm _{l_{\parallel }}$ as increments for the same structure, but measured across and along the field, and setting them equal to each other, we find a relationship between the parallel and perpendicular scales – the scale-dependent anisotropy (2.11):
The fact of scale-dependent anisotropy of MHD turbulence (if, in retrospect, not with the same confidence as the scaling (5.5)) was confirmed numerically by Cho & Vishniac (Reference Cho and Vishniac2000) and Maron & Goldreich (Reference Maron and Goldreich2001) and, in a rare triumph of theory correctly anticipating measurement, observed in the solar wind by Horbury et al. (Reference Horbury, Forman and Oughton2008), followed by many others (e.g., Podesta Reference Podesta2009; Wicks et al. Reference Wicks, Horbury, Chen and Schekochihin2010; Luo & Wu Reference Luo and Wu2010; Chen et al. Reference Chen, Mallet, Yousef, Schekochihin and Horbury2011 – a complete list is impossible here as this has now become an industry, as successful ideas do; see Chen Reference Chen2016 for a review). Figure 7 shows some of the first of those results. An important nuance is that, in order to see scale-dependent anisotropy, one must measure the parallel correlations along the perturbed, rather than global, mean magnetic field.Footnote 13 The reason for this is as follows.

Figure 7. (a) Parallel (![]() $P_\parallel$) and perpendicular (
$P_\parallel$) and perpendicular (![]() $P_\perp$) spectra (Fourier and wavelet) of the magnetic fluctuations in the solar wind, measured by the Ulysses spacecraft and computed by Wicks et al. (Reference Wicks, Horbury, Chen and Schekochihin2010), with frequencies
$P_\perp$) spectra (Fourier and wavelet) of the magnetic fluctuations in the solar wind, measured by the Ulysses spacecraft and computed by Wicks et al. (Reference Wicks, Horbury, Chen and Schekochihin2010), with frequencies ![]() $f$ converted to wavenumbers
$f$ converted to wavenumbers ![]() $k$ using the Taylor hypothesis (reprinted from Wicks et al. Reference Wicks, Horbury, Chen and Schekochihin2010 by permission of the Royal Astronomical Society). (b) An earlier (historic, the first ever) measurement by Horbury, Forman & Oughton (Reference Horbury, Forman and Oughton2008) of the spectral index of these spectra as a function of angle to the local mean field (reprinted with permission from Horbury et al. Reference Horbury, Forman and Oughton2008, copyright (2008) by the American Physical Society).
$k$ using the Taylor hypothesis (reprinted from Wicks et al. Reference Wicks, Horbury, Chen and Schekochihin2010 by permission of the Royal Astronomical Society). (b) An earlier (historic, the first ever) measurement by Horbury, Forman & Oughton (Reference Horbury, Forman and Oughton2008) of the spectral index of these spectra as a function of angle to the local mean field (reprinted with permission from Horbury et al. Reference Horbury, Forman and Oughton2008, copyright (2008) by the American Physical Society).
Both the causality argument (Boldyrev Reference Boldyrev2005; Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011) and the Lagrangian-frequency one (Beresnyak Reference Beresnyak2015) that I invoked in §§ 5.1 and 5.2 to justify long parallel coherence lengths of the MHD fluctuations rely on the ability of Alfvénic perturbations to propagate along the magnetic field. Physically, a small such perturbation on any given scale does not know the difference between a larger perturbation on, say, a few times its scale, and the ‘true’ mean field (whatever that is, outside the ideal world of periodic simulation boxes). Thus, it will propagate along the local field and so it is along the local field that the arguments based on this propagation will apply. What if we instead measure correlations along the global mean field or, more generally, along some coarse-grained version of the exact field? Let that coarse-grained field be the average over all perpendicular scales at and below some ![]() $L_{\perp }$ (to get the global mean field, make
$L_{\perp }$ (to get the global mean field, make ![]() $L_{\perp }$ the outer scale). Define Elsasser-field increments between pairs of points separated by a vector
$L_{\perp }$ the outer scale). Define Elsasser-field increments between pairs of points separated by a vector ![]() ${\boldsymbol {l}}$,
${\boldsymbol {l}}$,
and consider ![]() ${\boldsymbol {l}}$ along the exact magnetic field vs.
${\boldsymbol {l}}$ along the exact magnetic field vs. ![]() ${\boldsymbol {l}}$ along our coarse-grained field. The perpendicular distance by which the latter vector will veer off the field line (figure 8) will be dominated by the magnetic perturbation at the largest scale that was not included in the coarse-grained field:
${\boldsymbol {l}}$ along our coarse-grained field. The perpendicular distance by which the latter vector will veer off the field line (figure 8) will be dominated by the magnetic perturbation at the largest scale that was not included in the coarse-grained field:
If we are trying to capture parallel correlations corresponding to perturbations with perpendicular scale ![]() $\lambda \ll L _{\perp }$, then, using CB,
$\lambda \ll L _{\perp }$, then, using CB, ![]() $l/v_{\mathrm {A}}\sim \tau _{\mathrm {nl}}$, and (5.3) with
$l/v_{\mathrm {A}}\sim \tau _{\mathrm {nl}}$, and (5.3) with ![]() $\delta Z_\lambda ^\pm \sim \delta b_\lambda$, we conclude that
$\delta Z_\lambda ^\pm \sim \delta b_\lambda$, we conclude that
i.e., in such a measurement, the parallel correlations are swamped by perpendicular decorrelation, unless, in fact, ![]() $\lambda \sim L_{\perp }$ or larger (there is no such problem with measuring perpendicular correlations: small changes in a separation vector
$\lambda \sim L_{\perp }$ or larger (there is no such problem with measuring perpendicular correlations: small changes in a separation vector ![]() ${\boldsymbol {l}}$ taken perpendicular to the global vs. exact field make no difference).
${\boldsymbol {l}}$ taken perpendicular to the global vs. exact field make no difference).

Figure 8. Measuring correlations along local vs. global mean field. True parallel correlations cannot be captured by a measurement along the global field ![]() $\boldsymbol {B}_0$ if the distance
$\boldsymbol {B}_0$ if the distance ![]() $\varDelta l_\perp$ (see (5.7)) by which the point-separation vector
$\varDelta l_\perp$ (see (5.7)) by which the point-separation vector ![]() ${\boldsymbol {l}}$ along
${\boldsymbol {l}}$ along ![]() $\boldsymbol {B}_0$ ‘slips’ off the exact field line (
$\boldsymbol {B}_0$ ‘slips’ off the exact field line (![]() $\boldsymbol {B}_0+\boldsymbol {b}_{\perp }$) is greater than the perpendicular decorrelation length
$\boldsymbol {B}_0+\boldsymbol {b}_{\perp }$) is greater than the perpendicular decorrelation length ![]() $\lambda$ between ‘neighbouring’ field lines.
$\lambda$ between ‘neighbouring’ field lines.
Consequently, the easiest practical way to extract correlations along the local field from either observed or numerically simulated turbulence (Chen et al. Reference Chen, Mallet, Yousef, Schekochihin and Horbury2011) is to measure field increments (5.6) for many different separation vectors ![]() ${\boldsymbol {l}}$ and to calculate for each such increment the angle between
${\boldsymbol {l}}$ and to calculate for each such increment the angle between ![]() ${\boldsymbol {l}}$ and the ‘local mean field’
${\boldsymbol {l}}$ and the ‘local mean field’ ![]() $\boldsymbol {B}_{\mathrm {loc}}$ defined as the arithmetic mean of the magnetic field measured at the two points involved:
$\boldsymbol {B}_{\mathrm {loc}}$ defined as the arithmetic mean of the magnetic field measured at the two points involved:
This amounts to coarse-graining the field always at the right scale (just) for the correlations that are being probed. One can then measure (for example) perpendicular and parallel structure functions as conditional averages:
and similarly for intermediate values of ![]() $\phi$ (as explained above, the difference between
$\phi$ (as explained above, the difference between ![]() $\boldsymbol {B}_0$ and
$\boldsymbol {B}_0$ and ![]() $\boldsymbol {B}_{\mathrm {loc}}$ matters only for small
$\boldsymbol {B}_{\mathrm {loc}}$ matters only for small ![]() $\phi$). Thus, in general, one measures
$\phi$). Thus, in general, one measures
Alternatively, in simulations, one can simply follow field lines to get ![]() $\delta Z^\pm _{l_{\parallel }}$ (Cho & Vishniac Reference Cho and Vishniac2000; Maron & Goldreich Reference Maron and Goldreich2001) or, as was initially done in the solar wind, use local wavelet spectra (Horbury et al. Reference Horbury, Forman and Oughton2008; Podesta Reference Podesta2009; Wicks et al. Reference Wicks, Horbury, Chen and Schekochihin2010).
$\delta Z^\pm _{l_{\parallel }}$ (Cho & Vishniac Reference Cho and Vishniac2000; Maron & Goldreich Reference Maron and Goldreich2001) or, as was initially done in the solar wind, use local wavelet spectra (Horbury et al. Reference Horbury, Forman and Oughton2008; Podesta Reference Podesta2009; Wicks et al. Reference Wicks, Horbury, Chen and Schekochihin2010).
It turns out (see references cited above and innumerable others) that, quite robustly, ![]() $\zeta _2(0)=1$, consistent with (5.1), whereas
$\zeta _2(0)=1$, consistent with (5.1), whereas ![]() $\zeta _2(90^\textrm {o})$ is typically between
$\zeta _2(90^\textrm {o})$ is typically between ![]() $2/3$ and
$2/3$ and ![]() $1/2$, i.e., between GS95 and IK, in the solar wind, and rather closer to
$1/2$, i.e., between GS95 and IK, in the solar wind, and rather closer to ![]() $1/2$ in numerical simulations – although this, as I will discuss in §§ 6.3 and 6.4, has been hotly disputed by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019).
$1/2$ in numerical simulations – although this, as I will discuss in §§ 6.3 and 6.4, has been hotly disputed by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019).
Thus, while little doubt remains about the reality of scale-dependent anisotropy (although not necessarily of the specific scaling (5.5)) and of the ![]() $k_{\parallel }^{-2}$ spectrum (5.1), both arising from the GS95 theory, the GS95 prediction for the perpendicular spectrum (5.4) has continued to be suspect and controversial.
$k_{\parallel }^{-2}$ spectrum (5.1), both arising from the GS95 theory, the GS95 prediction for the perpendicular spectrum (5.4) has continued to be suspect and controversial.
6. Dynamic alignment, perpendicular cascade, and intermittency
Whereas solar-wind turbulence observations were, for a period of time, viewed to be consistent with a ![]() $-5/3$ spectrum,Footnote 14 leading ultimately to the GS95 revision of the IK paradigm, high-resolution numerical simulations of forced, incompressible MHD turbulence, starting with Maron & Goldreich (Reference Maron and Goldreich2001) and Müller, Biskamp & Grappin (Reference Müller, Biskamp and Grappin2003), have consistently shown scaling exponents closer to
$-5/3$ spectrum,Footnote 14 leading ultimately to the GS95 revision of the IK paradigm, high-resolution numerical simulations of forced, incompressible MHD turbulence, starting with Maron & Goldreich (Reference Maron and Goldreich2001) and Müller, Biskamp & Grappin (Reference Müller, Biskamp and Grappin2003), have consistently shown scaling exponents closer to ![]() $-3/2$ (while strongly confirming the local anisotropy; see also Cho & Vishniac Reference Cho and Vishniac2000; Cho, Lazarian & Vishniac Reference Cho, Lazarian and Vishniac2002b). This undermined somewhat the then still young GS95 consensus and stimulated hard questioning of the assumptions underlying its treatment of nonlinear interactions. The winning idea turned out to be that the nonlinearity in MHD turbulence might be depleted in a scale-dependent way by some form of alignment between
$-3/2$ (while strongly confirming the local anisotropy; see also Cho & Vishniac Reference Cho and Vishniac2000; Cho, Lazarian & Vishniac Reference Cho, Lazarian and Vishniac2002b). This undermined somewhat the then still young GS95 consensus and stimulated hard questioning of the assumptions underlying its treatment of nonlinear interactions. The winning idea turned out to be that the nonlinearity in MHD turbulence might be depleted in a scale-dependent way by some form of alignment between ![]() $\boldsymbol {Z}_{\perp }^+$ and
$\boldsymbol {Z}_{\perp }^+$ and ![]() $\boldsymbol {Z}_{\perp }^-$ and/or, perhaps, between the magnetic and velocity fields. Maron & Goldreich (Reference Maron and Goldreich2001) commented in passing on field alignment in their simulations, and Beresnyak & Lazarian (Reference Beresnyak and Lazarian2006) focused on ‘polarisation alignment’ explicitly, putting it on the table as a key effect requiring revision of GS95.Footnote 15 The same possibility was mooted by Boldyrev (Reference Boldyrev2005), and a year later he came up with a very beautiful (if, as we will see in § 6.3, somewhat flawed) argument based on the idea of what he referred to as ‘dynamic alignment’ (Boldyrev Reference Boldyrev2006), which set the direction of the field for the subsequent 10 years and which I am now going to discuss.
$\boldsymbol {Z}_{\perp }^-$ and/or, perhaps, between the magnetic and velocity fields. Maron & Goldreich (Reference Maron and Goldreich2001) commented in passing on field alignment in their simulations, and Beresnyak & Lazarian (Reference Beresnyak and Lazarian2006) focused on ‘polarisation alignment’ explicitly, putting it on the table as a key effect requiring revision of GS95.Footnote 15 The same possibility was mooted by Boldyrev (Reference Boldyrev2005), and a year later he came up with a very beautiful (if, as we will see in § 6.3, somewhat flawed) argument based on the idea of what he referred to as ‘dynamic alignment’ (Boldyrev Reference Boldyrev2006), which set the direction of the field for the subsequent 10 years and which I am now going to discuss.
6.1. Alignment and anisotropy in the perpendicular plane
Let me, however, deviate from Boldyrev's original case for alignmentFootnote 16 and follow instead Chandran et al. (Reference Chandran, Schekochihin and Mallet2015) in positing that, as the two Elsasser fields advect each other, they will shear each other into an increasingly parallel configuration. As this occurs, the gradient of the advectee (say, ![]() $\boldsymbol {Z}_{\perp }^-$) in the direction of the advector (
$\boldsymbol {Z}_{\perp }^-$) in the direction of the advector (![]() $\boldsymbol {Z}_{\perp }^+$) will get smaller than the gradient of either of them in the direction transverse to both.
$\boldsymbol {Z}_{\perp }^+$) will get smaller than the gradient of either of them in the direction transverse to both.
In general, therefore, we must allow the possibility of a local anisotropy in the 2D plane perpendicular to the mean magnetic field (figure 9). If we do that, our ‘twiddle’ estimate of the nonlinear term in (3.1) becomes
where ![]() $\xi ^-$ is the scale of variation of
$\xi ^-$ is the scale of variation of ![]() $\boldsymbol {Z}_{\perp }^-$ (advectee) in the direction of
$\boldsymbol {Z}_{\perp }^-$ (advectee) in the direction of ![]() $\boldsymbol {Z}_{\perp }^+$ (advector), taken at scale
$\boldsymbol {Z}_{\perp }^+$ (advector), taken at scale ![]() $\lambda$, which is the scale of
$\lambda$, which is the scale of ![]() $\boldsymbol {Z}_{\perp }^\pm$'s variation in the direction perpendicular both to
$\boldsymbol {Z}_{\perp }^\pm$'s variation in the direction perpendicular both to ![]() $\boldsymbol {Z}_{\perp }^+$ and to
$\boldsymbol {Z}_{\perp }^+$ and to ![]() $\boldsymbol {B}_0$ (all interactions are still assumed local in scale). But, by elementary vector calculus,
$\boldsymbol {B}_0$ (all interactions are still assumed local in scale). But, by elementary vector calculus,
where ![]() $\theta _\lambda$ the angle between the two Elsasser fields taken at scale
$\theta _\lambda$ the angle between the two Elsasser fields taken at scale ![]() $\lambda$. Under the scheme whereby the two fields shear each other into alignment in an, on average, symmetric fashion, we have
$\lambda$. Under the scheme whereby the two fields shear each other into alignment in an, on average, symmetric fashion, we have ![]() $\xi ^+ \sim \xi ^-$ and
$\xi ^+ \sim \xi ^-$ and
i.e., ![]() $\sin \theta _\lambda$ is the aspect ratio of the field structures in the perpendicular 2D plane.Footnote 17
$\sin \theta _\lambda$ is the aspect ratio of the field structures in the perpendicular 2D plane.Footnote 17

Figure 9. Cartoon of a GS95 eddy (a) vs. a Boldyrev (Reference Boldyrev2006) aligned eddy (b). The latter has three scales: ![]() $l_{\parallel }\gg \xi \gg \lambda$ (along
$l_{\parallel }\gg \xi \gg \lambda$ (along ![]() $\boldsymbol {B}_0$, along
$\boldsymbol {B}_0$, along ![]() $\boldsymbol {b}_{\perp }$, and transverse to both). This picture is adapted from Boldyrev (Reference Boldyrev2006) (reprinted with permission from Boldyrev Reference Boldyrev2006, copyright (2006) by the American Physical Society). In the context of my discussion, the fluctuation direction should, in fact, be thought of as along
$\boldsymbol {b}_{\perp }$, and transverse to both). This picture is adapted from Boldyrev (Reference Boldyrev2006) (reprinted with permission from Boldyrev Reference Boldyrev2006, copyright (2006) by the American Physical Society). In the context of my discussion, the fluctuation direction should, in fact, be thought of as along ![]() $\boldsymbol {Z}_{\perp }^+$ or
$\boldsymbol {Z}_{\perp }^+$ or ![]() $\boldsymbol {Z}_{\perp }^-$ (see figure 38).
$\boldsymbol {Z}_{\perp }^-$ (see figure 38).
In view of all this, we ought to replace the estimate (5.3) of the nonlinear time with
If the last expression is correct, then the more aligned the two fields are the more the nonlinearity is reduced compared to the ‘naïve’GS95 estimate (5.3). The challenge is to work out the scale dependence of the reduction factor ![]() $\sin \theta _\lambda$, which is what Boldyrev (Reference Boldyrev2006) did.
$\sin \theta _\lambda$, which is what Boldyrev (Reference Boldyrev2006) did.
6.2. Boldyrev's alignment conjecture
A version of Boldyrev's argumentFootnote 18 is to conjecture that the fields want to be misaligned as little as possible and that this minimal degree of misalignment is set by a kind of uncertainty principle: since the direction of the local magnetic field along which these perturbations propagate can itself only be defined within a small angle ![]() $\sim \delta b_\lambda /v_{\mathrm {A}}$, the two Elsasser fields (or the velocity and the magnetic field) cannot be aligned any more precisely than this and so
$\sim \delta b_\lambda /v_{\mathrm {A}}$, the two Elsasser fields (or the velocity and the magnetic field) cannot be aligned any more precisely than this and so
Alignment and imbalance (local or global), and, therefore the relative magnitudes of magnetic, velocity, and Elsasser fields, can be linked in a non-trivial way, which is still a matter of some debate, but I do not wish be distracted and so will postpone the discussion of that topic to § 9. Till then, I shall assume everywhere that
This allows (6.5) to be combined with (6.4) and yield
The constancy of flux then implies immediatelyFootnote 19
In dimensional terms, this has brought us back to the IK spectrum (2.5), except the wavenumber is now the perpendicular wavenumber and both anisotropy and CB are retained, although the relationship between the parallel and perpendicular scales changes:
Since CB remains in force, the parallel cascade stays the same as described in § 5.2.
For imminent use in what follows, let us compute the extent of the inertial range that this aligned cascade is supposed to span. Comparing the nonlinear cascade time (6.7) with the Ohmic diffusion time (assuming, for convenience that the magnetic diffusivity ![]() $\eta$ is either the same or larger than the kinematic viscosity of our MHD fluid), we find
$\eta$ is either the same or larger than the kinematic viscosity of our MHD fluid), we find
 \begin{equation} \tau_{\mathrm{nl}} \sim \left(\frac{v_{\mathrm{A}}\lambda}{\varepsilon}\right)^{1/2}\ll \tau_{\eta} \sim \frac{\lambda^2}{\eta} \quad\Leftrightarrow\quad\lambda \gg \eta^{2/3}\left(\frac{v_{\mathrm{A}}}{\varepsilon}\right)^{1/3}\equiv\lambda_{\eta}, \end{equation}
\begin{equation} \tau_{\mathrm{nl}} \sim \left(\frac{v_{\mathrm{A}}\lambda}{\varepsilon}\right)^{1/2}\ll \tau_{\eta} \sim \frac{\lambda^2}{\eta} \quad\Leftrightarrow\quad\lambda \gg \eta^{2/3}\left(\frac{v_{\mathrm{A}}}{\varepsilon}\right)^{1/3}\equiv\lambda_{\eta}, \end{equation}
where ![]() $\lambda _{\eta }$ is the cutoff scale – the Kolmogorov scale for this turbulence. For comparison, note that the same calculation based on the GS95 scalings (5.3) and (5.4) gives
$\lambda _{\eta }$ is the cutoff scale – the Kolmogorov scale for this turbulence. For comparison, note that the same calculation based on the GS95 scalings (5.3) and (5.4) gives
where ![]() $\lambda _{\eta }^\mathrm {GS95}$ is the classic Kolmogorov scale.
$\lambda _{\eta }^\mathrm {GS95}$ is the classic Kolmogorov scale.
If one embraces (6.8), one could argue that Kraichnan's dimensional argument was actually right, but it should have been used with ![]() $k_{\perp }$, rather than with
$k_{\perp }$, rather than with ![]() $k$, because
$k$, because ![]() $k_{\parallel }$ is not a ‘nonlinear’ dimension. This is the style of reasoning that Kraichnan himself might have found attractive. We are about to see, however, that the result (6.8) also runs into serious trouble and needs revision.
$k_{\parallel }$ is not a ‘nonlinear’ dimension. This is the style of reasoning that Kraichnan himself might have found attractive. We are about to see, however, that the result (6.8) also runs into serious trouble and needs revision.
6.3. Plot thickens
This is a very appealing theory, whose main conclusions were rapidly confirmed by a programme of numerical simulations undertaken by Boldyrev's group – in particular, the angle between velocity and magnetic field, measured in a certain opportune way,Footnote 20 was reported to scale according to ![]() $\theta _\lambda \propto \lambda ^{1/4}$, as implied by (6.8) and (6.5) (Mason, Cattaneo & Boldyrev Reference Mason, Cattaneo and Boldyrev2006, Reference Mason, Cattaneo and Boldyrev2008; Mason et al. Reference Mason, Perez, Cattaneo and Boldyrev2011, Reference Mason, Perez, Boldyrev and Cattaneo2012; Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2012, Reference Perez, Mason, Boldyrev and Cattaneo2014b). The same papers confirmed the earlier numerical claims that the spectrum of MHD turbulence indeed scaled as
$\theta _\lambda \propto \lambda ^{1/4}$, as implied by (6.8) and (6.5) (Mason, Cattaneo & Boldyrev Reference Mason, Cattaneo and Boldyrev2006, Reference Mason, Cattaneo and Boldyrev2008; Mason et al. Reference Mason, Perez, Cattaneo and Boldyrev2011, Reference Mason, Perez, Boldyrev and Cattaneo2012; Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2012, Reference Perez, Mason, Boldyrev and Cattaneo2014b). The same papers confirmed the earlier numerical claims that the spectrum of MHD turbulence indeed scaled as ![]() $k_{\perp }^{-3/2}$ (figure 10a). However, the legitimacy of this conclusion was contested by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019), who had, in fact, been first to focus on the alignment effect numerically (Beresnyak & Lazarian Reference Beresnyak and Lazarian2006), but disputed that the numerical spectra exhibiting it were converged and argued that systematic convergence tests in fact favoured a trend towards a
$k_{\perp }^{-3/2}$ (figure 10a). However, the legitimacy of this conclusion was contested by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019), who had, in fact, been first to focus on the alignment effect numerically (Beresnyak & Lazarian Reference Beresnyak and Lazarian2006), but disputed that the numerical spectra exhibiting it were converged and argued that systematic convergence tests in fact favoured a trend towards a ![]() $k_{\perp }^{-5/3}$ spectrum at asymptotically small scales. His point was that convergence of spectra with increasing resolution ought to be checked from the dissipative end of the inertial interval and that rescaling the spectra in his simulations to the Kolmogorov scale (6.11) gave a better data collapse than rescaling them to Boldyrev's cutoff scale (6.10) (figure 10(b); to be precise, in Beresnyak Reference Beresnyak2014b, he reports that the best numerical convergence obtained by this method is to
$k_{\perp }^{-5/3}$ spectrum at asymptotically small scales. His point was that convergence of spectra with increasing resolution ought to be checked from the dissipative end of the inertial interval and that rescaling the spectra in his simulations to the Kolmogorov scale (6.11) gave a better data collapse than rescaling them to Boldyrev's cutoff scale (6.10) (figure 10(b); to be precise, in Beresnyak Reference Beresnyak2014b, he reports that the best numerical convergence obtained by this method is to ![]() $k_{\perp }^{-1.7}$). Despite the sound and fury of the ensuing debate about the quality of the two competing sets of numerics (Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2014a; Beresnyak Reference Beresnyak2013, Reference Beresnyak2014a), it would not necessarily be obvious to anyone who took a look at their papers that their raw numerical results themselves were in fact all that different – certainly not as different as the interpretation of these results by their authors. Without dwelling on either, however, let me focus instead on a conceptual wrinkle in Boldyrev's original argument that Beresnyak (Reference Beresnyak2011) spotted and that cannot be easily dismissed.
$k_{\perp }^{-1.7}$). Despite the sound and fury of the ensuing debate about the quality of the two competing sets of numerics (Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2014a; Beresnyak Reference Beresnyak2013, Reference Beresnyak2014a), it would not necessarily be obvious to anyone who took a look at their papers that their raw numerical results themselves were in fact all that different – certainly not as different as the interpretation of these results by their authors. Without dwelling on either, however, let me focus instead on a conceptual wrinkle in Boldyrev's original argument that Beresnyak (Reference Beresnyak2011) spotted and that cannot be easily dismissed.

Figure 10. The best-resolved currently available spectra of RMHD turbulence. (a) From simulations by Perez et al. (Reference Perez, Mason, Boldyrev and Cattaneo2012) (their figure 1), with Laplacian viscosity and resolution up to ![]() $2048^2\times 512$. (b) From simulations by Beresnyak (Reference Beresnyak2014b) (his figure 1, © AAS, reproduced with permission), with Laplacian viscosity (a) and with fourth-order hyperviscosity (b); the resolution for the three spectra is
$2048^2\times 512$. (b) From simulations by Beresnyak (Reference Beresnyak2014b) (his figure 1, © AAS, reproduced with permission), with Laplacian viscosity (a) and with fourth-order hyperviscosity (b); the resolution for the three spectra is ![]() $1024^3$,
$1024^3$, ![]() $2048^3$ and
$2048^3$ and ![]() $4096^3$. His spectra are rescaled to Kolmogorov scale (6.11) (which he denotes
$4096^3$. His spectra are rescaled to Kolmogorov scale (6.11) (which he denotes ![]() $\eta$). He finds poorer convergence (see his figure 2) when he rescales to Boldyrev's scale (6.24). Perez et al. (Reference Perez, Mason, Boldyrev and Cattaneo2012) appear to get a somewhat better outcome (see their figure 8) if they plot their spectra vs.
$\eta$). He finds poorer convergence (see his figure 2) when he rescales to Boldyrev's scale (6.24). Perez et al. (Reference Perez, Mason, Boldyrev and Cattaneo2012) appear to get a somewhat better outcome (see their figure 8) if they plot their spectra vs. ![]() $k_{\perp }\lambda _{\eta }$ where
$k_{\perp }\lambda _{\eta }$ where ![]() $\lambda _{\eta }$ is given by (6.24) with
$\lambda _{\eta }$ is given by (6.24) with ![]() $\lambda _{\mathrm {CB}}$ computed in each simulation as the normalisation constant in the scaling (6.22) of
$\lambda _{\mathrm {CB}}$ computed in each simulation as the normalisation constant in the scaling (6.22) of ![]() $\sin \theta _\lambda$ (in their analysis, however, this is the angle between velocity and magnetic perturbations, not the Elsasser fields).
$\sin \theta _\lambda$ (in their analysis, however, this is the angle between velocity and magnetic perturbations, not the Elsasser fields).
In the RMHD limit (whose applicability to MHD turbulence at sufficiently small scales we have no reason to doubt), ![]() $\delta b_\lambda /v_{\mathrm {A}}$ is an arbitrarily small quantity, and so must then be, according to (6.5), the alignment angle
$\delta b_\lambda /v_{\mathrm {A}}$ is an arbitrarily small quantity, and so must then be, according to (6.5), the alignment angle ![]() $\sin \theta _\lambda$. Introducing such a large depletion of the nonlinearity into (3.1) would abolish it completely in the RMHD ordering and render the system linear. The only way to keep the nonlinearity while assuming a small angle
$\sin \theta _\lambda$. Introducing such a large depletion of the nonlinearity into (3.1) would abolish it completely in the RMHD ordering and render the system linear. The only way to keep the nonlinearity while assuming a small angle ![]() $\theta _\lambda$ is to take the angle to be small but still ordered as unity in the RMHD ordering – in other words, it cannot scale with
$\theta _\lambda$ is to take the angle to be small but still ordered as unity in the RMHD ordering – in other words, it cannot scale with ![]() $\epsilon$ under the RMHD rescaling symmetry (3.5). The same rescaling symmetry implies that any physical scaling that involves
$\epsilon$ under the RMHD rescaling symmetry (3.5). The same rescaling symmetry implies that any physical scaling that involves ![]() $v_{\mathrm {A}}$ and
$v_{\mathrm {A}}$ and ![]() $l_{\parallel }$ (and no other scales) must involve them in the combination
$l_{\parallel }$ (and no other scales) must involve them in the combination ![]() $l_{\parallel }/v_{\mathrm {A}}$ (see § 5.2 and Beresnyak Reference Beresnyak2012a), which (6.9) manifestly does not. All this flies in the face of the fact that a substantial body of numerical evidence supporting aligned MHD turbulence was obtained by means of RMHD simulations (Mason et al. Reference Mason, Perez, Cattaneo and Boldyrev2011, Reference Mason, Perez, Boldyrev and Cattaneo2012; Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2012; Beresnyak Reference Beresnyak2012a; Mallet et al. Reference Mallet, Schekochihin and Chandran2015, Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016) – complemented by explicit evidence that full MHD simulations produce quantitatively the same alignment – so the standard recourse to casting a cloud of suspicion on the validity of an asymptotic approximation is not available in this case.
$l_{\parallel }/v_{\mathrm {A}}$ (see § 5.2 and Beresnyak Reference Beresnyak2012a), which (6.9) manifestly does not. All this flies in the face of the fact that a substantial body of numerical evidence supporting aligned MHD turbulence was obtained by means of RMHD simulations (Mason et al. Reference Mason, Perez, Cattaneo and Boldyrev2011, Reference Mason, Perez, Boldyrev and Cattaneo2012; Perez et al. Reference Perez, Mason, Boldyrev and Cattaneo2012; Beresnyak Reference Beresnyak2012a; Mallet et al. Reference Mallet, Schekochihin and Chandran2015, Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016) – complemented by explicit evidence that full MHD simulations produce quantitatively the same alignment – so the standard recourse to casting a cloud of suspicion on the validity of an asymptotic approximation is not available in this case.
In a further blow to the conjecture (6.5), it turns out that the alignment angle between the Elsasser fields at any given scale is anticorrelated with their amplitudes (Mallet et al. Reference Mallet, Schekochihin and Chandran2015), supporting the view that the dynamical alignment is indeed dynamical, being brought about by the mutual shearing of the Elsasser fields (Chandran et al. Reference Chandran, Schekochihin and Mallet2015), rather than by the uncertainty principle (6.5) (which would imply, presumably, a positive correlation between ![]() $\theta _\lambda$ and
$\theta _\lambda$ and ![]() $\delta Z_\lambda$).
$\delta Z_\lambda$).
The numerical evidence in favour of alignment appears to be real and solid.Footnote 21 While numerical simulations at currently feasible resolutions cannot definitively verify or falsify Beresnyak's expectation that alignment is but a transient feature that disappears at asymptotically small scales, they certainly show aligned, locally 3D-anisotropic turbulence over a respectable inertial subrange at least one order of magnitude wide, and probably two. This is approaching the kind of scale separations that actually exist in nature, e.g., in the solar wind (where the evidence for a ![]() $k_{\perp }^{-3/2}$ spectrum has also been firming up: see, e.g., Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020), and we cannot be casually dismissive of a physical regime, even if transient, that occupies most of the phase space that we are able to probe!
$k_{\perp }^{-3/2}$ spectrum has also been firming up: see, e.g., Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020), and we cannot be casually dismissive of a physical regime, even if transient, that occupies most of the phase space that we are able to probe!
6.4. Revised model of aligned MHD turbulence
6.4.1. Dimensional and RMHD-symmetry constraints
Let me make the restrictions implied by Beresnyak's objection more explicit. Under the RMHD rescaling symmetry (3.5),
(note that ![]() $\varepsilon$ is the energy flux whereas
$\varepsilon$ is the energy flux whereas ![]() $\epsilon$ is the scaling factor). Therefore, the scaling relation (6.8) becomes
$\epsilon$ is the scaling factor). Therefore, the scaling relation (6.8) becomes ![]() $\epsilon \delta Z_\lambda \sim \epsilon ^{3/4}(\varepsilon v _{\mathrm {A}}\lambda )^{1/4}$, which is obviously a contradiction. Indeed, trialling
$\epsilon \delta Z_\lambda \sim \epsilon ^{3/4}(\varepsilon v _{\mathrm {A}}\lambda )^{1/4}$, which is obviously a contradiction. Indeed, trialling
and mandating both the symmetry (6.12) and dimensional consistency, one finds that the GS95 solution (5.4), ![]() $\nu =0$ and
$\nu =0$ and ![]() $\gamma =\mu =1/3$, is the only possibility, which was Beresnyak's point.
$\gamma =\mu =1/3$, is the only possibility, which was Beresnyak's point.
It seems obvious that the only way to rescue alignment is to allow another parameter – and the (almost) obvious choice is ![]() $L_{\parallel }$, the parallel outer scale, which transforms as
$L_{\parallel }$, the parallel outer scale, which transforms as ![]() $L_{\parallel }\to (a/\epsilon )L_{\parallel }$. Then
$L_{\parallel }\to (a/\epsilon )L_{\parallel }$. Then
where the second expression is the result of imposing on the first the RMHD symmetry (6.12) and dimensional correctness; ![]() $\delta =0$ returns us to GS95.Footnote 22 The same argument applied to the scaling of
$\delta =0$ returns us to GS95.Footnote 22 The same argument applied to the scaling of ![]() $l_{\parallel }$ with
$l_{\parallel }$ with ![]() $\varepsilon$,
$\varepsilon$, ![]() $v_{\mathrm {A}}$,
$v_{\mathrm {A}}$, ![]() $\lambda$ and
$\lambda$ and ![]() $L_{\parallel }$ gives
$L_{\parallel }$ gives
where ![]() $\sigma$ is a free parameter. Note that both (6.14) and (6.15) manifestly contain the parallel scales and
$\sigma$ is a free parameter. Note that both (6.14) and (6.15) manifestly contain the parallel scales and ![]() $v_{\mathrm {A}}$ in the solely allowed combinations
$v_{\mathrm {A}}$ in the solely allowed combinations ![]() $l_{\parallel }/v_{\mathrm {A}}$ and
$l_{\parallel }/v_{\mathrm {A}}$ and ![]() $L_{\parallel }/v_{\mathrm {A}}$. A reassuring consistency check is to ask what perpendicular scale
$L_{\parallel }/v_{\mathrm {A}}$. A reassuring consistency check is to ask what perpendicular scale ![]() $\lambda =L_{\perp }$ corresponds to
$\lambda =L_{\perp }$ corresponds to ![]() $l_{\parallel }=L_{\parallel }$: this turns out to be
$l_{\parallel }=L_{\parallel }$: this turns out to be
 \begin{equation} L_{{\perp}} \sim \varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2} \sim \lambda_{\mathrm{CB}}, \end{equation}
\begin{equation} L_{{\perp}} \sim \varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2} \sim \lambda_{\mathrm{CB}}, \end{equation}
the very same ![]() $\lambda _{\mathrm {CB}}$, given by (4.3), at which weak turbulence becomes strong – thus seamlessly connecting any strong-turbulence theory expressed by (6.14) and (6.15) with the WT cascade discussed in § 4.Footnote 23 Notably, if we applied such a test to (6.9), we would find the price of consistency to be
$\lambda _{\mathrm {CB}}$, given by (4.3), at which weak turbulence becomes strong – thus seamlessly connecting any strong-turbulence theory expressed by (6.14) and (6.15) with the WT cascade discussed in § 4.Footnote 23 Notably, if we applied such a test to (6.9), we would find the price of consistency to be ![]() $L_{\perp }=L_{\parallel }$, which is allowed but does not have to be the case in MHD and certainly cannot be the case in RMHD.
$L_{\perp }=L_{\parallel }$, which is allowed but does not have to be the case in MHD and certainly cannot be the case in RMHD.
Finally, since the parallel-cascade scaling (5.1) remains beyond reasonable doubt and, as can be readily checked, respects the rescaling symmetry (3.5) (Beresnyak Reference Beresnyak2015), combining it with (6.15) and (6.14) fixes
Alas, CB does not help with determining ![]() $\delta$ because, in aligned turbulence, the nonlinear time (6.4) contains the unknown scale
$\delta$ because, in aligned turbulence, the nonlinear time (6.4) contains the unknown scale ![]() $\xi$, or, equivalently, the alignment angle
$\xi$, or, equivalently, the alignment angle ![]() $\theta _\lambda \sim \lambda /\xi$. If we did know
$\theta _\lambda \sim \lambda /\xi$. If we did know ![]() $\delta$, CB would let us determine this angle:
$\delta$, CB would let us determine this angle:
where ![]() $\lambda _{\mathrm {CB}}$ is given by (6.16). The answer that we want to get – keeping Boldyrev's scalings of everything with
$\lambda _{\mathrm {CB}}$ is given by (6.16). The answer that we want to get – keeping Boldyrev's scalings of everything with ![]() $\lambda$ but not with
$\lambda$ but not with ![]() $\varepsilon$ or
$\varepsilon$ or ![]() $v_{\mathrm {A}}$ – requires
$v_{\mathrm {A}}$ – requires
Then, instead of (6.8), we end up with
 \begin{equation} \delta Z_\lambda \sim \varepsilon^{3/8}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/8}\lambda^{1/4},\quad l_{{\parallel}} \sim \varepsilon^{{-}1/4}v_{\mathrm{A}}^{3/4}L_{{\parallel}}^{1/4}\lambda^{1/2},\quad \sin\theta_\lambda \sim \varepsilon^{{-}1/8}\left(\frac{v_{\mathrm{A}}}{L_{{\parallel}}}\right)^{3/8}\lambda^{1/4}, \end{equation}
\begin{equation} \delta Z_\lambda \sim \varepsilon^{3/8}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/8}\lambda^{1/4},\quad l_{{\parallel}} \sim \varepsilon^{{-}1/4}v_{\mathrm{A}}^{3/4}L_{{\parallel}}^{1/4}\lambda^{1/2},\quad \sin\theta_\lambda \sim \varepsilon^{{-}1/8}\left(\frac{v_{\mathrm{A}}}{L_{{\parallel}}}\right)^{3/8}\lambda^{1/4}, \end{equation}and the dissipation cutoff scale (6.10) is corrected as follows:
 \begin{equation} \tau_{\mathrm{nl}} \sim \left(\frac{L_{{\parallel}}}{\varepsilon v_{\mathrm{A}}}\right)^{1/4}\lambda^{1/2} \ll \tau_{\eta} \sim \frac{\lambda^2}{\eta}\quad\Leftrightarrow\quad \lambda \gg \eta^{2/3}\left(\frac{L_{{\parallel}}}{\varepsilon v_{\mathrm{A}}}\right)^{1/6}\equiv\lambda_{\eta}. \end{equation}
\begin{equation} \tau_{\mathrm{nl}} \sim \left(\frac{L_{{\parallel}}}{\varepsilon v_{\mathrm{A}}}\right)^{1/4}\lambda^{1/2} \ll \tau_{\eta} \sim \frac{\lambda^2}{\eta}\quad\Leftrightarrow\quad \lambda \gg \eta^{2/3}\left(\frac{L_{{\parallel}}}{\varepsilon v_{\mathrm{A}}}\right)^{1/6}\equiv\lambda_{\eta}. \end{equation}
Note that, since ![]() $\lambda _{\eta }\propto \eta ^{2/3}$ still, this does not address Beresnyak's numerical evidence on the convergence of the spectra (§ 6.3) – I shall come back to this problem in § 7.
$\lambda _{\eta }\propto \eta ^{2/3}$ still, this does not address Beresnyak's numerical evidence on the convergence of the spectra (§ 6.3) – I shall come back to this problem in § 7.
For future convenience, let me recast the scalings (6.20) in a somewhat simpler form:
 \begin{equation} \delta Z_\lambda \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/4},\quad \frac{l_{{\parallel}}}{L_{{\parallel}}} \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/2},\quad \sin\theta_\lambda \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/4}. \end{equation}
\begin{equation} \delta Z_\lambda \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/4},\quad \frac{l_{{\parallel}}}{L_{{\parallel}}} \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/2},\quad \sin\theta_\lambda \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/4}. \end{equation}Defining the magnetic Reynolds number based on the CB scale (6.16) and the fluctuation amplitude at this scale,
allows the dissipation scale (6.21) to be recast as follows:
 \begin{equation} \frac{\lambda_{\eta}}{\lambda_{\mathrm{CB}}} \sim \left(\frac{\mathrm{Rm}}{1+\mathrm{Pm}}\right)^{{-}2/3} = \widetilde{\mathrm{Re}}^{{-}2/3},\quad \mathrm{Pm} = \frac{\nu}{\eta},\quad \widetilde{\mathrm{Re}} = \frac{\delta Z_{\lambda_{\mathrm{CB}}}\lambda_{\mathrm{CB}}}{\nu+\eta}. \end{equation}
\begin{equation} \frac{\lambda_{\eta}}{\lambda_{\mathrm{CB}}} \sim \left(\frac{\mathrm{Rm}}{1+\mathrm{Pm}}\right)^{{-}2/3} = \widetilde{\mathrm{Re}}^{{-}2/3},\quad \mathrm{Pm} = \frac{\nu}{\eta},\quad \widetilde{\mathrm{Re}} = \frac{\delta Z_{\lambda_{\mathrm{CB}}}\lambda_{\mathrm{CB}}}{\nu+\eta}. \end{equation}
I have restored the possibility that viscosity ![]() $\nu$ might be larger than the magnetic diffusivity
$\nu$ might be larger than the magnetic diffusivity ![]() $\eta$: if that is the case, one must replace the latter with the former in the calculation of the dissipative cutoff, whereas if
$\eta$: if that is the case, one must replace the latter with the former in the calculation of the dissipative cutoff, whereas if ![]() $\mathrm {Pm}\lesssim 1$, it does not matter, hence the appearance of the magnetic Prandtl number
$\mathrm {Pm}\lesssim 1$, it does not matter, hence the appearance of the magnetic Prandtl number ![]() $\mathrm {Pm}$ in the combination
$\mathrm {Pm}$ in the combination ![]() $(1+\mathrm {Pm})$.
$(1+\mathrm {Pm})$.
Yet another way to write the first of the scaling relations (6.20) is
This is effectively the prediction for the spectrum that Perez et al. (Reference Perez, Mason, Boldyrev and Cattaneo2012, Reference Perez, Mason, Boldyrev and Cattaneo2014b) used in their numerical convergence studies. Thus, they and I are on the same page as to what the spectrum of aligned turbulence is expected to be, although the question remains why it should be that if Boldyrev's uncertainty principle (6.5) can no longer be used.
A set of RMHD-compatible scalings (6.20), or (6.25), is also effectively what was deduced by Chandran et al. (Reference Chandran, Schekochihin and Mallet2015) and by Mallet & Schekochihin (Reference Mallet and Schekochihin2017) from a set of plausible conjectures about the dynamics and statistics of RMHD turbulence (they did not explicitly discuss the issue of the RMHD rescaling symmetry, but used normalisations that enforced it automatically). The two papers differed in their strategy for determining the exponent ![]() $\delta$; my exposition here will be a ‘heuristic’ version of Mallet & Schekochihin (Reference Mallet and Schekochihin2017).
$\delta$; my exposition here will be a ‘heuristic’ version of Mallet & Schekochihin (Reference Mallet and Schekochihin2017).
6.4.2. Intermittency matters!
The premise of both Chandran et al. (Reference Chandran, Schekochihin and Mallet2015) and Mallet & Schekochihin (Reference Mallet and Schekochihin2017) is that in order to determine the scalings of everything, including the energy spectrum, one must have a working model of intermittency, i.e., of the way in which fluctuation amplitudes and their scale lengths in all three directions – ![]() $\lambda$,
$\lambda$, ![]() $\xi$ and
$\xi$ and ![]() $l_{\parallel }$ – are distributed in a turbulent MHD system. It may be disturbing to the reader, or viewed by her as an unnecessary complication, that we must involve ‘rare’ events – as this is what the theory of intermittency is about – in the mundane business of the scaling of the energy spectra, which are usually viewed as made up from the more ‘typical’ fluctuations. These doubts might be alleviated by the following observation. The appearance of the outer scale
$l_{\parallel }$ – are distributed in a turbulent MHD system. It may be disturbing to the reader, or viewed by her as an unnecessary complication, that we must involve ‘rare’ events – as this is what the theory of intermittency is about – in the mundane business of the scaling of the energy spectra, which are usually viewed as made up from the more ‘typical’ fluctuations. These doubts might be alleviated by the following observation. The appearance of the outer scale ![]() $L_{\parallel }$ in (6.14) suggests that the self-similarity is broken – this is somewhat analogous to what happens in hydrodynamic turbulence, where corrections to the K41 scaling (2.2) come in as powers of
$L_{\parallel }$ in (6.14) suggests that the self-similarity is broken – this is somewhat analogous to what happens in hydrodynamic turbulence, where corrections to the K41 scaling (2.2) come in as powers of ![]() $\lambda /L$ (Kolmogorov Reference Kolmogorov1962; Frisch Reference Frisch1995). We may view
$\lambda /L$ (Kolmogorov Reference Kolmogorov1962; Frisch Reference Frisch1995). We may view ![]() $\delta$ as just such a correction to the self-similar GS95 result, and alignment as the physical mechanism whereby this intermittency correction arises. The main difference with the hydrodynamic case is that
$\delta$ as just such a correction to the self-similar GS95 result, and alignment as the physical mechanism whereby this intermittency correction arises. The main difference with the hydrodynamic case is that ![]() $\delta$ is not all that small (MHD turbulence is ‘more intermittent’ than the hydrodynamic one), the mechanism responsible for it has important consequences (§ 7), and so we care.
$\delta$ is not all that small (MHD turbulence is ‘more intermittent’ than the hydrodynamic one), the mechanism responsible for it has important consequences (§ 7), and so we care.
I shall forgo a detailed discussion of the intermittency model that Mallet & Schekochihin (Reference Mallet and Schekochihin2017) proposed; for my purposes here, a vulgarised version of their argument will suffice. They consider the turbulent field as an ensemble of structures, or fluctuations, each of which has some amplitude and three scales: parallel ![]() $l_{\parallel }$, perpendicular
$l_{\parallel }$, perpendicular ![]() $\lambda$ and fluctuation-direction
$\lambda$ and fluctuation-direction ![]() $\xi$ (they call this the ‘RMHD ensemble’). They make certain conjectures about the joint probability distribution of these quantities, which then allow them to fix scalings. The most crucial (and perhaps also the most arbitrary) of these conjectures is, effectively, that for all structures,
$\xi$ (they call this the ‘RMHD ensemble’). They make certain conjectures about the joint probability distribution of these quantities, which then allow them to fix scalings. The most crucial (and perhaps also the most arbitrary) of these conjectures is, effectively, that for all structures, ![]() $l_{\parallel }\sim \lambda ^\alpha$ with the same exponent
$l_{\parallel }\sim \lambda ^\alpha$ with the same exponent ![]() $\alpha$, i.e., that the quantity
$\alpha$, i.e., that the quantity ![]() $l_{\parallel }/\lambda ^\alpha$ has a scale-invariant distribution (this appears to be confirmed by numerical evidence: see figure 11a). They then determine the exponent
$l_{\parallel }/\lambda ^\alpha$ has a scale-invariant distribution (this appears to be confirmed by numerical evidence: see figure 11a). They then determine the exponent ![]() $\alpha$ by considering ‘the most intense structures’Footnote 24 – because it is possible to work out what the probability of encountering them is as a function both of
$\alpha$ by considering ‘the most intense structures’Footnote 24 – because it is possible to work out what the probability of encountering them is as a function both of ![]() $\lambda$ and of
$\lambda$ and of ![]() $l_{\parallel }$.
$l_{\parallel }$.

Figure 11. (a) Probability distribution of ![]() $l_{\parallel }/\lambda ^{1/2}$ in a
$l_{\parallel }/\lambda ^{1/2}$ in a ![]() $1024^3$ RMHD simulation (the shades of colour from blue to red correspond to PDFs at increasing scales within the inertial range). This plot is taken from Mallet & Schekochihin (Reference Mallet and Schekochihin2017) (where the reader will find a discussion – somewhat inconclusive – of the slope of this PDF) and illustrates how good (or otherwise) is the assumption that
$1024^3$ RMHD simulation (the shades of colour from blue to red correspond to PDFs at increasing scales within the inertial range). This plot is taken from Mallet & Schekochihin (Reference Mallet and Schekochihin2017) (where the reader will find a discussion – somewhat inconclusive – of the slope of this PDF) and illustrates how good (or otherwise) is the assumption that ![]() $l_{\parallel }/\lambda ^\alpha$ has a scale-invariant distribution (the assumption is not as good as RCB, illustrated in figure 6 based on data from the same simulation). (b) Joint probability distribution for the length
$l_{\parallel }/\lambda ^\alpha$ has a scale-invariant distribution (the assumption is not as good as RCB, illustrated in figure 6 based on data from the same simulation). (b) Joint probability distribution for the length ![]() $l_{\parallel }$ and width
$l_{\parallel }$ and width ![]() $\xi$ (in my notation) of the most intense dissipative structures (adapted from Zhdankin, Boldyrev & Uzdensky Reference Zhdankin, Boldyrev and Uzdensky2016b). This shows that
$\xi$ (in my notation) of the most intense dissipative structures (adapted from Zhdankin, Boldyrev & Uzdensky Reference Zhdankin, Boldyrev and Uzdensky2016b). This shows that ![]() $\xi \propto l _{\parallel }$, in line with (6.29). (Reprinted from Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b with the permission of AIP Publishing.) Independent simulations by J. M. Stone (private communication, 2018) support this scaling.
$\xi \propto l _{\parallel }$, in line with (6.29). (Reprinted from Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b with the permission of AIP Publishing.) Independent simulations by J. M. Stone (private communication, 2018) support this scaling.
They then conjecture that the most intense structures in the RMHD ensemble are sheets transverse to the local perpendicular direction. Therefore, if one looks for their probability (filling fraction) in any perpendicular plane as a function of the perpendicular scale ![]() $\lambda$, one expects it to scale as
$\lambda$, one expects it to scale as
If, on the other hand, one is interested in their filling fraction in the plane locally tangent to a flux sheet (i.e., defined by the local mean field and the direction of the fluctuation vector), it is
The next conjecture is the ‘refined critical balance’ (RCB, already advertised in § 5.1), stating that not only is ![]() $\tau _{\mathrm {nl}}\sim \tau _{\mathrm {A}}$ in some vague ‘typical’ sense, but the quantity
$\tau _{\mathrm {nl}}\sim \tau _{\mathrm {A}}$ in some vague ‘typical’ sense, but the quantity
has a scale-invariant distribution in the RMHD ensemble – this was discovered by Mallet et al. (Reference Mallet, Schekochihin and Chandran2015) to be satisfied with truly remarkable accuracy in numerically simulated RMHD turbulence (figure 6).Footnote 25 If this is true for all structures, it is true for the most intense ones – and a further assumption about those is that their amplitude ![]() $\delta Z_{\mathrm {max}}$ is not a function of scale but is simply equal to some typical outer-scale value (i.e., the most intense structures are formed by the largest perturbations collapsing, or being sheared, into sheets without breaking up into smaller perturbations; see Chandran et al. Reference Chandran, Schekochihin and Mallet2015). This, together with (6.27), implies that for those structures,
$\delta Z_{\mathrm {max}}$ is not a function of scale but is simply equal to some typical outer-scale value (i.e., the most intense structures are formed by the largest perturbations collapsing, or being sheared, into sheets without breaking up into smaller perturbations; see Chandran et al. Reference Chandran, Schekochihin and Mallet2015). This, together with (6.27), implies that for those structures,
(Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b confirm that ![]() $\xi \propto l _{\parallel }$ for the most intense dissipative structures: see figure 11b). Comparing (6.29) with (6.26), we conclude that
$\xi \propto l _{\parallel }$ for the most intense dissipative structures: see figure 11b). Comparing (6.29) with (6.26), we conclude that ![]() $l_{\parallel }\propto \lambda ^{1/2}$ for the most intense structures and, therefore, for everyone else – by the conjecture of scale invariance of
$l_{\parallel }\propto \lambda ^{1/2}$ for the most intense structures and, therefore, for everyone else – by the conjecture of scale invariance of ![]() $l_{\parallel }/\lambda ^\alpha$, where we now know that
$l_{\parallel }/\lambda ^\alpha$, where we now know that ![]() $\alpha =1/2$. Comparing this with (6.15), we see that
$\alpha =1/2$. Comparing this with (6.15), we see that ![]() $\alpha = 2(1-\sigma )/3$, whence
$\alpha = 2(1-\sigma )/3$, whence
the latter by virtue of (6.17). Q.e.d.: we now have the scalings (6.20).
I do not know if the reader will find this quasi-intuitive argument more (or less) convincing than the formal-looking conjectures and corollaries in Mallet & Schekochihin (Reference Mallet and Schekochihin2017). There is no need to repeat their algebra here, but hopefully the above sheds some (flickering) light – if not, perhaps a better argument will be invented one day, but all I can recommend for now is reading their paper. Notably, in their more formal treatment, not just the energy spectrum but the two-point structure functions of all orders are predicted – and turn out to be a decent fit to numerical data as it currently stands.Footnote 26 The same is true about the model proposed in the earlier paper by Chandran et al. (Reference Chandran, Schekochihin and Mallet2015). Their approach is based on a much more enthusiastic engagement with dynamics: a careful analysis of how aligned and non-aligned structures might form and interact. They get ![]() $\delta \approx 0.108$, which leads to
$\delta \approx 0.108$, which leads to ![]() $\delta Z_\lambda \propto \lambda ^{0.26}$ – not a great deal of difference with (6.20), considering that all of this is very far from being exact science. Their approach does have the distinction, however, of emphasising particularly strongly the dynamic nature of the dynamic alignment, which arises as Elsasser fields shear each other into sheet-like structures.
$\delta Z_\lambda \propto \lambda ^{0.26}$ – not a great deal of difference with (6.20), considering that all of this is very far from being exact science. Their approach does have the distinction, however, of emphasising particularly strongly the dynamic nature of the dynamic alignment, which arises as Elsasser fields shear each other into sheet-like structures.
6.5. 3D anisotropy
Before moving on, I would like to re-emphasise the 3D anisotropy of the aligned MHD turbulence – and the fact that this anisotropy is local, associated at every point with the three directions that themselves depend on the fluctuating fields: parallel to the magnetic field (![]() $l_{\parallel }$), along the vector direction of the perturbed field
$l_{\parallel }$), along the vector direction of the perturbed field ![]() $\boldsymbol {Z}_{\perp }^\mp$ that advects the field
$\boldsymbol {Z}_{\perp }^\mp$ that advects the field ![]() $\boldsymbol {Z}_{\perp }^\pm$ whose correlations we are measuring (
$\boldsymbol {Z}_{\perp }^\pm$ whose correlations we are measuring (![]() $\xi$), and the third direction perpendicular to the other two (
$\xi$), and the third direction perpendicular to the other two (![]() $\lambda$). This local 3D anisotropy is measurableFootnote 27 and has indeed been observed both in the solar wind (Chen et al. Reference Chen, Mallet, Schekochihin, Horbury, Wicks and Bale2012; Verdini et al. Reference Verdini, Grappin, Alexandrova and Lion2018, Reference Verdini, Grappin, Alexandrova, Franci, Landi, Matteini and Papini2019) and in numerical simulations (Verdini & Grappin Reference Verdini and Grappin2015; Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016) – both are illustrated by figure 12. The main point of discrepancy between the true and virtual reality is the scale dependence of the anisotropy: confirmed solidly in simulations but only very tentatively in the solar wind (see footnote 21). However, progress never stops, and one can hope for better missions (Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) and even more sophisticated analysis.
$\lambda$). This local 3D anisotropy is measurableFootnote 27 and has indeed been observed both in the solar wind (Chen et al. Reference Chen, Mallet, Schekochihin, Horbury, Wicks and Bale2012; Verdini et al. Reference Verdini, Grappin, Alexandrova and Lion2018, Reference Verdini, Grappin, Alexandrova, Franci, Landi, Matteini and Papini2019) and in numerical simulations (Verdini & Grappin Reference Verdini and Grappin2015; Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016) – both are illustrated by figure 12. The main point of discrepancy between the true and virtual reality is the scale dependence of the anisotropy: confirmed solidly in simulations but only very tentatively in the solar wind (see footnote 21). However, progress never stops, and one can hope for better missions (Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) and even more sophisticated analysis.

Figure 12. Locally 3D-anisotropic structures in the (a) solar wind (from Verdini et al. Reference Verdini, Grappin, Alexandrova and Lion2018 © AAS, reproduced with permission) and (b) numerical simulations (here ![]() $l_{\parallel }$ is normalised to
$l_{\parallel }$ is normalised to ![]() $L_{\parallel }/2\pi$ and
$L_{\parallel }/2\pi$ and ![]() $\lambda$ and
$\lambda$ and ![]() $\xi$ to
$\xi$ to ![]() $L_{\perp }/2\pi$, hence apparent isotropy at the outer scale). These are surfaces of constant second-order structure function of the magnetic field (a) or one of the Elsasser fields (b). The three images correspond to successively smaller fluctuations and so successively smaller scales (only the last of the three is firmly in the universal inertial range). In both cases, the emergence of statistics with
$L_{\perp }/2\pi$, hence apparent isotropy at the outer scale). These are surfaces of constant second-order structure function of the magnetic field (a) or one of the Elsasser fields (b). The three images correspond to successively smaller fluctuations and so successively smaller scales (only the last of the three is firmly in the universal inertial range). In both cases, the emergence of statistics with ![]() $l_{\parallel }\gg \xi \gg \lambda$ is manifest. In the solar wind, the route that turbulence takes to this aligned state appears to depend quite strongly on the solar-wind expansion, which distorts magnetic-field component in the radial direction compared to the azimuthal ones (Verdini & Grappin Reference Verdini and Grappin2015; Vech & Chen Reference Vech and Chen2016). The data shown in panel (a) was carefully selected to minimise this effect; without such selection, one sees structures most strongly elongated in the
$l_{\parallel }\gg \xi \gg \lambda$ is manifest. In the solar wind, the route that turbulence takes to this aligned state appears to depend quite strongly on the solar-wind expansion, which distorts magnetic-field component in the radial direction compared to the azimuthal ones (Verdini & Grappin Reference Verdini and Grappin2015; Vech & Chen Reference Vech and Chen2016). The data shown in panel (a) was carefully selected to minimise this effect; without such selection, one sees structures most strongly elongated in the ![]() $\xi$ direction at the larger scales (
$\xi$ direction at the larger scales (![]() $\xi >l_{\parallel }>\lambda$), although they too tend to the universal aligned regime at smaller scales (see Chen et al. Reference Chen, Mallet, Schekochihin, Horbury, Wicks and Bale2012, where 3D-anisotropy plots like ones shown here first appeared).
$\xi >l_{\parallel }>\lambda$), although they too tend to the universal aligned regime at smaller scales (see Chen et al. Reference Chen, Mallet, Schekochihin, Horbury, Wicks and Bale2012, where 3D-anisotropy plots like ones shown here first appeared).
The scaling of the energy spectrum in the parallel direction (§ 5.2) was arguably the most robust and uncontroversial of the results reviewed thus far. We then occupied ourselves with the scalings of the Elsasser-field increments and of ![]() $l_{\parallel }$ vs. the perpendicular scale
$l_{\parallel }$ vs. the perpendicular scale ![]() $\lambda$, culminating in § 6.4 with a theory that one (hopefully) can believe in. The scalings with the third, fluctuation-direction coordinate
$\lambda$, culminating in § 6.4 with a theory that one (hopefully) can believe in. The scalings with the third, fluctuation-direction coordinate ![]() $\xi$ are very easy to obtain because the nonlinear time of the aligned cascade (6.4) has the same dependence on
$\xi$ are very easy to obtain because the nonlinear time of the aligned cascade (6.4) has the same dependence on ![]() $\xi$ as it did on
$\xi$ as it did on ![]() $\lambda$ in the unaligned, GS95 theory: see (5.3). Therefore,
$\lambda$ in the unaligned, GS95 theory: see (5.3). Therefore,
 \begin{equation} \delta Z_\xi \sim (\varepsilon\xi)^{1/3},\quad \xi \sim \varepsilon^{1/8}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/8}\lambda^{3/4} \sim \lambda_{\mathrm{CB}}^{1/4}\lambda^{3/4}, \end{equation}
\begin{equation} \delta Z_\xi \sim (\varepsilon\xi)^{1/3},\quad \xi \sim \varepsilon^{1/8}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/8}\lambda^{3/4} \sim \lambda_{\mathrm{CB}}^{1/4}\lambda^{3/4}, \end{equation}
with the latter formula following from (6.3) and (6.20) or (6.22). Thus, Elsasser fields’ spectra have exponents ![]() $-2$ in the
$-2$ in the ![]() $l_{\parallel }$ direction,
$l_{\parallel }$ direction, ![]() $-3/2$ in the
$-3/2$ in the ![]() $\lambda$ direction and
$\lambda$ direction and ![]() $-5/3$ in the
$-5/3$ in the ![]() $\xi$ direction (see the
$\xi$ direction (see the ![]() $n=2$ exponents in figure 13a). Let me note in passing that the ‘Kolmogorov’ scaling (6.31) will play a key part in my discussion, in Appendix D.7, of why the Lazarian & Vishniac (Reference Lazarian and Vishniac1999) notion of ‘stochastic reconnection’does not automatically invalidate the aligned cascade and return us to GS95, as an erudite reader might have been worried it would – the idea is that the Richardson (Reference Richardson1926) (super)diffusion of Lagrangian trajectories in a turbulent field is always determined by the
$n=2$ exponents in figure 13a). Let me note in passing that the ‘Kolmogorov’ scaling (6.31) will play a key part in my discussion, in Appendix D.7, of why the Lazarian & Vishniac (Reference Lazarian and Vishniac1999) notion of ‘stochastic reconnection’does not automatically invalidate the aligned cascade and return us to GS95, as an erudite reader might have been worried it would – the idea is that the Richardson (Reference Richardson1926) (super)diffusion of Lagrangian trajectories in a turbulent field is always determined by the ![]() $\xi$-dependent scaling (6.31), regardless of the nature of the cascade in
$\xi$-dependent scaling (6.31), regardless of the nature of the cascade in ![]() $\lambda$.
$\lambda$.

Figure 13. Scaling exponents of the structure functions in RMHD turbulence simulated by Mallet et al. (Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016) (the plot is reproduced from Mallet & Schekochihin Reference Mallet and Schekochihin2017). (a) Structure functions of the Elsasser-field increments (5.6): by definition, ![]() $\langle |\delta \boldsymbol {Z}_{\boldsymbol {l}}^+|^n\rangle \propto l^{\zeta _n}$ and
$\langle |\delta \boldsymbol {Z}_{\boldsymbol {l}}^+|^n\rangle \propto l^{\zeta _n}$ and ![]() $\zeta _n^\perp$,
$\zeta _n^\perp$, ![]() $\zeta _n^\mathrm {fluc}$,
$\zeta _n^\mathrm {fluc}$, ![]() $\zeta _n^\parallel$ are exponents for
$\zeta _n^\parallel$ are exponents for ![]() $l=\lambda$,
$l=\lambda$, ![]() $\xi$,
$\xi$, ![]() $l_{\parallel }$, respectively (i.e., all structure functions are conditional on the point separation being in one of the three directions of local 3D anisotropy; see §§ 5.3 and 6.5). The solid lines are for a
$l_{\parallel }$, respectively (i.e., all structure functions are conditional on the point separation being in one of the three directions of local 3D anisotropy; see §§ 5.3 and 6.5). The solid lines are for a ![]() $1024^3$ simulation (with hyperviscosity), the dashed ones are for a
$1024^3$ simulation (with hyperviscosity), the dashed ones are for a ![]() $512^3$ simulation, indicating how converged, or otherwise, the exponents are, and the dotted lines, in both (a) and (b), are the theoretical model by Mallet & Schekochihin (Reference Mallet and Schekochihin2017). (b) Similarly defined structure functions of the velocity (solid lines) and magnetic-field (dashed lines) increments from the same
$512^3$ simulation, indicating how converged, or otherwise, the exponents are, and the dotted lines, in both (a) and (b), are the theoretical model by Mallet & Schekochihin (Reference Mallet and Schekochihin2017). (b) Similarly defined structure functions of the velocity (solid lines) and magnetic-field (dashed lines) increments from the same ![]() $1024^3$ simulation. The magnetic field is ‘more intermittent’ than the Elsasser fields and the latter more so than velocity. An early (possibly first) numerical measurement of this kind, highlighting the differences between scalings of different fields and in different local directions, was done by Cho, Lazarian & Vishniac (Reference Cho, Lazarian and Vishniac2003).
$1024^3$ simulation. The magnetic field is ‘more intermittent’ than the Elsasser fields and the latter more so than velocity. An early (possibly first) numerical measurement of this kind, highlighting the differences between scalings of different fields and in different local directions, was done by Cho, Lazarian & Vishniac (Reference Cho, Lazarian and Vishniac2003).
A good way of thinking physically of the inevitability of 3D anisotropy is to note that, from (6.4) and CB,
i.e., ![]() $\xi$ is the typical displacement of a fluid element and also the typical perpendicular distance a field line wanders within a structure coherent on the parallel scale
$\xi$ is the typical displacement of a fluid element and also the typical perpendicular distance a field line wanders within a structure coherent on the parallel scale ![]() $l_{\parallel }$. Fluctuations must therefore preserve coherence in their own direction at least on the scale
$l_{\parallel }$. Fluctuations must therefore preserve coherence in their own direction at least on the scale ![]() $\xi$. They are not constrained in this way in the third direction
$\xi$. They are not constrained in this way in the third direction ![]() $\lambda$, and the fluctuation direction itself has an angular uncertainty of the order of the angle
$\lambda$, and the fluctuation direction itself has an angular uncertainty of the order of the angle ![]() $\theta _\lambda$ between the two fields, so it makes sense that the aspect ratio of the structures in the perpendicular plane should satisfy (6.3).
$\theta _\lambda$ between the two fields, so it makes sense that the aspect ratio of the structures in the perpendicular plane should satisfy (6.3).
The dependence of the anisotropy on the local direction of the fluctuating fields makes the connection between anisotropy, alignment and intermittency more obvious: when we follow perturbed field lines to extract parallel correlations or measure one Elsasser field's decorrelation along the direction of another Elsasser field, we are clearly not calculating second-order statistics in the strict sense – and so, in formal terms, local scale-dependent anisotropy always involves correlation functions of (all) higher orders.Footnote 28 Thus, it makes a certain natural sense to speak of the alignment-induced departure of MHD-turbulence spectrum from the Kolmogorovian GS95 scaling and of the 3D anisotropy of the underlying fluctuation field as an intermittency effect, as I have done here.
6.6. Higher-order statistics
In several places (e.g., in §§ 5.3 and 6.4.2), I have brushed against the more formal task of the intermittency theory – the calculation of the scaling exponents of higher-order structure functions or, equivalently, of the probability distributions of field increments – and recoiled every time, opting for ‘twiddle’ algebra and statements about spectra. A fair amount of information on these matters is available from simulations and from the solar-wind measurements: what intermittency looks like in the former is illustrated by figure 13 (a survey of previous measurements of structure functions, both in simulations and in the solar wind, can be found in Chandran et al. Reference Chandran, Schekochihin and Mallet2015). Some of what is known is perhaps understood, but much remains a mystery: for example, we do not know why the higher-order scaling exponents are generally not the same for velocity, Elsasser and magnetic fields, with the latter ‘more intermittent’ than the former, as is evident in figure 13(b) (in § 10.4, I will moot a possible connexion between intermittency and negative ‘residual energy’ – an asymmetry between the magnetic and velocity spectra seen both in numerical simulations and in the solar wind – but I do not know how to translate this into anything resembling figure 13b).
Interesting as it is, I will now leave the problem of higher-order statistics alone. We know from the (ongoing) history of hydrodynamic-turbulence theory that once this becomes the unsolved problem that everyone is working on, the scope for abstract theorising expands to fill all available space (and time) while attention paid by the outside world diminishes.Footnote 29 This said, I hasten to dispel any possible impression that I do not consider intermittency of MHD turbulence an important problem: in fact, as I have argued above, intermittency as a physical phenomenon appears to be so inextricably hard-wired into the structure of MHD turbulence that any workable theory of the latter has to be a theory of its intermittency.
Finally, let me jump ahead of myself and mention also that we know nothing at all of the intermittency in ‘tearing-dominated turbulence’, which is about to be introduced (§ 7), and very little of the intermittency in the variants of MHD turbulence surveyed in §§ 9–13. In particular, the relationship between intermittency and Elsasser imbalance, local or global, appears to me to be a promising object for theoreticians’ scrutiny (see § 9.1).
7. MHD turbulence meets reconnection
Finally, we wonder if it is possible that Sweet's mechanism might modify somewhat the diffusion and dissipation of the magnetic field in hydromagnetic turbulence.
Last sentence of Parker (Reference Parker1957)
If we accept that MHD turbulence in the inertial range – or, at least, in some subrange of the inertial range immediately below the outer scale – has a tendency to organise itself into fettuccine-like structures whose aspect ratio in the 2D plane perpendicular to the mean magnetic field increases as their size decreases, we are opting for a state of affairs that is not sustainable asymptotically, at ever smaller scales. These structures are mini-sheets, and sheets in MHD tend to be tearing-unstable. Thus, just like WT, strong aligned turbulence too carries in it the seeds of its own destruction, making an eventual transition to some new state inevitable at sufficiently small scales.Footnote 30
The notion that current sheets will spontaneously form in a turbulent MHD fluid is not new (Matthaeus & Lamkin Reference Matthaeus and Lamkin1986; Politano et al. Reference Politano, Pouquet and Sulem1989) and the phenomenology of these structures has been studied (numerically) quite extensively, e.g., by Servidio et al. (Reference Servidio, Matthaeus, Shay, Cassak and Dmitruk2009, Reference Servidio, Matthaeus, Shay, Dmitruk, Cassak and Wan2010, Reference Servidio, Dmitruk, Greco, Wan, Donato, Cassak, Shay, Carbone and Matthaeus2011a,Reference Servidio, Greco, Matthaeus, Osman and Dmitrukb) in 2D and by Zhdankin et al. (Reference Zhdankin, Uzdensky, Perez and Boldyrev2013, Reference Zhdankin, Boldyrev, Perez and Tobias2014); Zhdankin, Uzdensky & Boldyrev (Reference Zhdankin, Uzdensky and Boldyrev2015); Zhdankin et al. (Reference Zhdankin, Boldyrev and Uzdensky2016b) in 3D (see also Wan et al. Reference Wan, Rappazzo, Matthaeus, Servidio and Oughton2014), while solar-wind measurements (e.g., Retinò et al. Reference Retinò, Sundkvist, Vaivads, Mozer, André and Owen2007; Sundkvist et al. Reference Sundkvist, Retinò, Vaivads and Bale2007; Osman et al. Reference Osman, Matthaeus, Gosling, Greco, Servidio, Hnat, Chapman and Phan2014; Greco et al. Reference Greco, Perri, Servidio, Yordanova and Veltri2016) provided motivation and, perhaps, vindication. However, theoretical discussion of these results appeared to focus on the association between current sheets in MHD turbulence and its intermittent nature, identifying spontaneously forming current sheets as the archetypal intermittent events – and effectively segregating this topic from the traditional questions about the energy spectrum and the ‘typical’ structures believed to be responsible for it, viz., Alfvénic perturbations, aligned or otherwise.
In fact, as I argued in §§ 6.4.2 and 6.5, it is impossible to separate the physics of alignment from that of intermittency. Dynamic alignment produces sheet-like, or ‘proto-sheet’, structures that measurably affect the energy spectrum but are also the intermittent fluctuations that can perhaps collapse into proper current sheets. The likelihood that they will do so – or, more generally, that aligned structures can survive at all – hinges on whether the nonlinear cascade time ![]() $\tau _{\mathrm {nl}}$ at a given scale
$\tau _{\mathrm {nl}}$ at a given scale ![]() $\lambda$ is longer or shorter than the typical time scale on which a tearing mode can be triggered, leading to the break up of the dynamically forming sheets into islands (Uzdensky & Loureiro Reference Uzdensky and Loureiro2016). Since the growth rate of the tearing mode in resistive MHD is limited by resistivity and would be zero in the limit of infinitely small
$\lambda$ is longer or shorter than the typical time scale on which a tearing mode can be triggered, leading to the break up of the dynamically forming sheets into islands (Uzdensky & Loureiro Reference Uzdensky and Loureiro2016). Since the growth rate of the tearing mode in resistive MHD is limited by resistivity and would be zero in the limit of infinitely small ![]() $\eta$, the aligned turbulent cascade should be safe from tearing above a certain scale that must be proportional to some positive power of
$\eta$, the aligned turbulent cascade should be safe from tearing above a certain scale that must be proportional to some positive power of ![]() $\eta$. However, this need not be the same as Boldyrev's cutoff scale (6.21) that arises from the competition between the cascade rate and vanilla Ohmic (or viscous) diffusion (
$\eta$. However, this need not be the same as Boldyrev's cutoff scale (6.21) that arises from the competition between the cascade rate and vanilla Ohmic (or viscous) diffusion (![]() $\tau _{\mathrm {nl}}$ vs.
$\tau _{\mathrm {nl}}$ vs. ![]() $\tau _{\eta }$) – so, at the very least, the cutoff scale of the aligned cascade may not be what one might have thought it was, and what happens below that scale may be more interesting than the usual dull exponential petering out of the energy spectrum in the dissipation range.
$\tau _{\eta }$) – so, at the very least, the cutoff scale of the aligned cascade may not be what one might have thought it was, and what happens below that scale may be more interesting than the usual dull exponential petering out of the energy spectrum in the dissipation range.
This possibility was explored by Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b) and Loureiro & Boldyrev (Reference Loureiro and Boldyrev2017b) (unaware of each other's converging preoccupations), leading to a new scaling for the aligned cascade's cutoff and to a new model for the tail end of the MHD turbulence spectrum – mitigating some of the unsatisfactory features of the aligned-turbulence paradigm and thus providing a kind of glossy finish to the overall picture (despite their rather esoteric nature, the two papers appear to have become instant classics – so much so as to merit logarithmic corrections: Comisso et al. Reference Comisso, Huang, Lingam, Hirvijoki and Bhattacharjee2018). While the key idea in the two papers was the same, their takes on its consequences for the ‘tearing-mediated turbulence’ were somewhat different – here I will follow Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b), but present their results in a somewhat simpler, if less general, form.Footnote 31
Before I proceed, let me alert my erudite reader that the profound alteration of the MHD cascade by reconnection that I will discuss here has nothing at all to do with the concept of ‘stochastic reconnection’ in MHD turbulence associated with the work of Lazarian, Vishniac, Eyink, and their co-workers – this is explained carefully in § 8.3.3 and in Appendix D.7.
7.1. Disruption by tearing
The scale at which the aligned structures will be disrupted by tearing can be estimated very easily by comparing the nonlinear time (6.4) of the aligned cascade with the growth time of the fastest tearing mode that can be triggered in an MHD sheet of a given transverse scale ![]() $\lambda$. That this growth time is a good estimate for the time that reconnection needs to break up a sheet forming as a result of ideal-MHD dynamics is not quite as obvious as it might appear, but it is true and was carefully shown to be so by Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016). The maximum tearing growth rate is
$\lambda$. That this growth time is a good estimate for the time that reconnection needs to break up a sheet forming as a result of ideal-MHD dynamics is not quite as obvious as it might appear, but it is true and was carefully shown to be so by Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016). The maximum tearing growth rate is
How to derive this is reviewed in Appendix D.1 (see (D32)). Here ![]() $v_{\mathrm {A}y}$ is the Alfvén speed associated with the perturbed magnetic field that reverses at scale
$v_{\mathrm {A}y}$ is the Alfvén speed associated with the perturbed magnetic field that reverses at scale ![]() $\lambda$,
$\lambda$, ![]() $S_\lambda$ is the corresponding Lundquist number and
$S_\lambda$ is the corresponding Lundquist number and ![]() $\mathrm {Pm}$ is the magnetic Prandtl number, which only matters if the viscosity
$\mathrm {Pm}$ is the magnetic Prandtl number, which only matters if the viscosity ![]() $\nu$ is larger than the magnetic diffusivity
$\nu$ is larger than the magnetic diffusivity ![]() $\eta$. In application to our aligned Alfvénic structures, we should replace
$\eta$. In application to our aligned Alfvénic structures, we should replace ![]() $v_{\mathrm {A}y}\sim \delta Z_\lambda$. Then, using the scalings (6.22) to work out
$v_{\mathrm {A}y}\sim \delta Z_\lambda$. Then, using the scalings (6.22) to work out ![]() $\tau _{\mathrm {nl}}$, we find that the aligned cascade is faster than tearing as long as
$\tau _{\mathrm {nl}}$, we find that the aligned cascade is faster than tearing as long as
where ![]() $\mathrm {Rm} \sim S_{\lambda _{\mathrm {CB}}}$, as defined in (6.23), and the scale
$\mathrm {Rm} \sim S_{\lambda _{\mathrm {CB}}}$, as defined in (6.23), and the scale ![]() $\lambda _{\mathrm {D}}$ will henceforth be referred to as the disruption scale. At scales
$\lambda _{\mathrm {D}}$ will henceforth be referred to as the disruption scale. At scales ![]() $\lambda \lesssim \lambda _{\mathrm {D}}$, aligned sheet-like structures can no longer retain their integrity against the onslaught of tearing.Footnote 32
$\lambda \lesssim \lambda _{\mathrm {D}}$, aligned sheet-like structures can no longer retain their integrity against the onslaught of tearing.Footnote 32
7.1.1. Some reservations and limitations
Let me observe parenthetically that one can entertain legitimate reservations about the validity of (7.1) and other formulae based on laminar-tearing theory in a noisy, turbulent environment (see, e.g., the discussion and references in footnote 103). Here suffice it to say, that at scales where ![]() $\gamma \ll \tau _{\mathrm {nl}}^{-1}$, the laminar formulae are, of course, unjustified, and at scales where
$\gamma \ll \tau _{\mathrm {nl}}^{-1}$, the laminar formulae are, of course, unjustified, and at scales where ![]() $\gamma \gg \tau _{\mathrm {nl}}^{-1}$, if such situations existed, they would be perfectly fine, modulo the issue of flows, which I will explain in a moment.Footnote 33 The disruption scale
$\gamma \gg \tau _{\mathrm {nl}}^{-1}$, if such situations existed, they would be perfectly fine, modulo the issue of flows, which I will explain in a moment.Footnote 33 The disruption scale ![]() $\lambda _{\mathrm {D}}$ is where
$\lambda _{\mathrm {D}}$ is where ![]() $\gamma \sim \tau _{\mathrm {nl}}^{-1}$, so the laminar-tearing formulae are presumably only order-unity wrong, which I acknowledge by using ‘
$\gamma \sim \tau _{\mathrm {nl}}^{-1}$, so the laminar-tearing formulae are presumably only order-unity wrong, which I acknowledge by using ‘![]() $\sim$’ instead of ‘
$\sim$’ instead of ‘![]() $=$’ everywhere.
$=$’ everywhere.
Another legitimate reservation concerns application to aligned structures formed by the MHD cascade of the tearing theory derived for a magnetostatic equilibrium. Indeed, these structures are not purely magnetic sheets, but rather Elsasser ones, i.e., they have local shear flows superimposed on them. These flows are likely somewhat sub-Alfvénic (the reasons and evidence for that are explained in § 10.4), but, technically speaking, it has not been shown that they are sub-Alfvénic enough to justify application of the no-flow tearing-mode theory or even that they cannot fatally stabilise tearing (some further discussion and references on tearing with flows can be found at the end of Appendix D.4.2). Since the shear rate in these flows is no larger than ![]() $\tau _{\mathrm {nl}}^{-1}$, I think that this difficulty is a technical one, rather than a deal breaker, i.e., that what I am doing here is again only order-unity wrong (cf. Tolman, Loureiro & Uzdensky Reference Tolman, Loureiro and Uzdensky2018), but I cannot prove it rigorously. If this admission has not discouraged the reader, let us proceed.
$\tau _{\mathrm {nl}}^{-1}$, I think that this difficulty is a technical one, rather than a deal breaker, i.e., that what I am doing here is again only order-unity wrong (cf. Tolman, Loureiro & Uzdensky Reference Tolman, Loureiro and Uzdensky2018), but I cannot prove it rigorously. If this admission has not discouraged the reader, let us proceed.
The disruption scale ![]() $\lambda _{\mathrm {D}}$, upon comparison with the putative resistive cutoff (6.24) of the aligned cascade turns out to supersede it provided
$\lambda _{\mathrm {D}}$, upon comparison with the putative resistive cutoff (6.24) of the aligned cascade turns out to supersede it provided ![]() $\mathrm {Pm}$ is not too large:
$\mathrm {Pm}$ is not too large:
 \begin{equation} \frac{\lambda_{\mathrm{D}}}{\lambda_{\eta}} \sim \left[\frac{\mathrm{Rm}}{(1+\mathrm{Pm})^{10}}\right]^{2/21}\gg1. \end{equation}
\begin{equation} \frac{\lambda_{\mathrm{D}}}{\lambda_{\eta}} \sim \left[\frac{\mathrm{Rm}}{(1+\mathrm{Pm})^{10}}\right]^{2/21}\gg1. \end{equation}
In view of the ridiculous exponents involved, this means that in a system with even moderately large ![]() $\mathrm {Pm}$ and/or not a truly huge
$\mathrm {Pm}$ and/or not a truly huge ![]() $\mathrm {Rm}$, the aligned cascade will happily make it to the dissipation cutoff (6.24) and no further chapters are necessary in this story.Footnote 34 However, I do want to tell the story in full and so will focus on situations in which the condition (7.3) is satisfied.
$\mathrm {Rm}$, the aligned cascade will happily make it to the dissipation cutoff (6.24) and no further chapters are necessary in this story.Footnote 34 However, I do want to tell the story in full and so will focus on situations in which the condition (7.3) is satisfied.
7.1.2. Debris of disruption
I shall turn to the question of what happens at scales below ![]() $\lambda _{\mathrm {D}}$ in § 7.2, but to do that, it is necessary first to ask what becomes of the aligned structures that are disrupted at
$\lambda _{\mathrm {D}}$ in § 7.2, but to do that, it is necessary first to ask what becomes of the aligned structures that are disrupted at ![]() $\lambda _{\mathrm {D}}$.
$\lambda _{\mathrm {D}}$.
The tearing instability that disrupts them, the so-called Coppi mode, or (the fastest-growing) resistive internal kink mode (Coppi et al. Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976), has the wavenumber (see (D32))
 \begin{equation} k_* \sim \frac{1}{\lambda}\,S_{\lambda}^{{-}1/4}(1+\mathrm{Pm})^{1/8} \sim\frac{1}{\lambda_{\mathrm{CB}}} \mathrm{Rm}^{{-}1/4}(1+\mathrm{Pm})^{1/8}\left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{{-}21/16}, \end{equation}
\begin{equation} k_* \sim \frac{1}{\lambda}\,S_{\lambda}^{{-}1/4}(1+\mathrm{Pm})^{1/8} \sim\frac{1}{\lambda_{\mathrm{CB}}} \mathrm{Rm}^{{-}1/4}(1+\mathrm{Pm})^{1/8}\left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{{-}21/16}, \end{equation}
where (6.22) has been used to substitute for ![]() $\delta Z_\lambda$ inside
$\delta Z_\lambda$ inside ![]() $S_\lambda$. Therefore, at the disruption scale (
$S_\lambda$. Therefore, at the disruption scale (![]() $\lambda =\lambda _{\mathrm {D}}$),
$\lambda =\lambda _{\mathrm {D}}$),
If referred to the length of the sheet ![]() $\xi _{\mathrm {D}}$, which depends on
$\xi _{\mathrm {D}}$, which depends on ![]() $\lambda _{\mathrm {D}}$ via (6.31), this wavenumber gives us an estimate for the number of islands in the growing perturbation:
$\lambda _{\mathrm {D}}$ via (6.31), this wavenumber gives us an estimate for the number of islands in the growing perturbation:
As this is always large (or at least ![]() $\gtrsim 1$), the mode fits comfortably into the sheet that it is trying to disrupt.Footnote 35
$\gtrsim 1$), the mode fits comfortably into the sheet that it is trying to disrupt.Footnote 35
What happens to these islands? When the tearing mode enters the nonlinear regime, the island width is (see Appendix D.2)
which is smaller than ![]() $\lambda _{\mathrm {D}}$ and so, technically speaking, the aligned structures need not be destroyed by these islands. Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) (followed by Mallet et al. Reference Mallet, Schekochihin and Chandran2017b and by Loureiro & Boldyrev Reference Loureiro and Boldyrev2017b) argue that, after the tearing mode goes nonlinear, the islands will grow further while the
$\lambda _{\mathrm {D}}$ and so, technically speaking, the aligned structures need not be destroyed by these islands. Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) (followed by Mallet et al. Reference Mallet, Schekochihin and Chandran2017b and by Loureiro & Boldyrev Reference Loureiro and Boldyrev2017b) argue that, after the tearing mode goes nonlinear, the islands will grow further while the ![]() $X$-points between them collapse into current sheets – all of that on the same time scale (7.1) as the mode grew. It seems intuitive that, in order to break up the aligned structure (ideal-MHD sheet) that spawned them, the islands would have to get to
$X$-points between them collapse into current sheets – all of that on the same time scale (7.1) as the mode grew. It seems intuitive that, in order to break up the aligned structure (ideal-MHD sheet) that spawned them, the islands would have to get to ![]() $w\sim \lambda _{\mathrm {D}}$ (Uzdensky & Loureiro Reference Uzdensky and Loureiro2016). If they did this in a cross-section-area-preserving way, then they would be circular at the point of disruption: from (7.7),
$w\sim \lambda _{\mathrm {D}}$ (Uzdensky & Loureiro Reference Uzdensky and Loureiro2016). If they did this in a cross-section-area-preserving way, then they would be circular at the point of disruption: from (7.7), ![]() $w k_*^{-1}\sim \lambda _{\mathrm {D}}^2$. Such a set of islands (flux ropes) would be isotropic in the perpendicular plane. Another simplistic model is to imagine their width grow due to further reconnection while their length remains the same, viz.,
$w k_*^{-1}\sim \lambda _{\mathrm {D}}^2$. Such a set of islands (flux ropes) would be isotropic in the perpendicular plane. Another simplistic model is to imagine their width grow due to further reconnection while their length remains the same, viz., ![]() $\sim k_*^{-1}$ (simplistic because the lengthening of the inter-island current sheets is ignored). The aspect ratio of such a flux rope once it reached the width
$\sim k_*^{-1}$ (simplistic because the lengthening of the inter-island current sheets is ignored). The aspect ratio of such a flux rope once it reached the width ![]() $\lambda _{\mathrm {D}}$ would be
$\lambda _{\mathrm {D}}$ would be
where ![]() $N$ is given by (7.6). This is a degree of alignment preserved, but reduced by a (asymptotically large) factor of
$N$ is given by (7.6). This is a degree of alignment preserved, but reduced by a (asymptotically large) factor of ![]() $N$. At the extreme end of the range of possibilities is the view of Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017) that, in fact, islands of size (7.7) are already sufficient to break up the aligned structure, so alignment is not abruptly reduced by tearing disruption. Both this idea and some nuances about the
$N$. At the extreme end of the range of possibilities is the view of Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017) that, in fact, islands of size (7.7) are already sufficient to break up the aligned structure, so alignment is not abruptly reduced by tearing disruption. Both this idea and some nuances about the ![]() $X$-point collapse are discussed further in § 7.4.1. The key point for us here is that at the disruption scale, the aligned structures that cascade down from the inertial range are broken up by reconnection into flux ropes that are underaligned and may even be isotropic in the perpendicular plane. They are a starting point for a new kind of cascade, which I shall now proceed to consider.
$X$-point collapse are discussed further in § 7.4.1. The key point for us here is that at the disruption scale, the aligned structures that cascade down from the inertial range are broken up by reconnection into flux ropes that are underaligned and may even be isotropic in the perpendicular plane. They are a starting point for a new kind of cascade, which I shall now proceed to consider.
7.2. Tearing-mediated turbulence
If you accept the argument that the disruption by tearing of an aligned structure at the scale ![]() $\lambda _{\mathrm {D}}$ leads to its break-up into a number of flux ropes of width
$\lambda _{\mathrm {D}}$ leads to its break-up into a number of flux ropes of width ![]() $\sim \lambda _{\mathrm {D}}$, then the natural conclusion is that
$\sim \lambda _{\mathrm {D}}$, then the natural conclusion is that ![]() $\lambda _{\mathrm {D}}$ now becomes a kind of ‘outer scale’ for a new cascade. There need not be anything particularly different about this cascade compared to the standard aligned cascade except the alignment angle may now be reset to a greater value. As the disruption-scale structures interact with each other and break up into smaller structures, the latter should develop the same tendency to align as their inertial-range predecessors did. For a while, the structures in this new cascade are safe from tearing as their aspect ratio is not large enough, but eventually (i.e., at small enough scales), they too will become sufficiently aligned to be broken up by tearing modes. This leads to another disruption, another iteration of an aligned ‘mini-cascade’, and so on. Thus, if we rename our critical-balance scale
$\lambda _{\mathrm {D}}$ now becomes a kind of ‘outer scale’ for a new cascade. There need not be anything particularly different about this cascade compared to the standard aligned cascade except the alignment angle may now be reset to a greater value. As the disruption-scale structures interact with each other and break up into smaller structures, the latter should develop the same tendency to align as their inertial-range predecessors did. For a while, the structures in this new cascade are safe from tearing as their aspect ratio is not large enough, but eventually (i.e., at small enough scales), they too will become sufficiently aligned to be broken up by tearing modes. This leads to another disruption, another iteration of an aligned ‘mini-cascade’, and so on. Thus, if we rename our critical-balance scale ![]() $\lambda _{\mathrm {CB}}=\lambda _0$, the disruption scale
$\lambda _{\mathrm {CB}}=\lambda _0$, the disruption scale ![]() $\lambda _{\mathrm {D}}=\lambda _1$, and call the subsequent disruption scales
$\lambda _{\mathrm {D}}=\lambda _1$, and call the subsequent disruption scales ![]() $\lambda _n$, we can think of the MHD cascade as consisting of a sequence of aligned cascades interrupted by disruption episodes. I shall calculate
$\lambda _n$, we can think of the MHD cascade as consisting of a sequence of aligned cascades interrupted by disruption episodes. I shall calculate ![]() $\lambda _n$ and the spectral exponents for this new, composite tearing-mediated cascade in § 7.2.1, the final dissipation cutoff in § 7.2.2, the cascade's alignment properties in § 7.2.3, and its parallel scalings, again determined by CB, in § 7.2.4.
$\lambda _n$ and the spectral exponents for this new, composite tearing-mediated cascade in § 7.2.1, the final dissipation cutoff in § 7.2.2, the cascade's alignment properties in § 7.2.3, and its parallel scalings, again determined by CB, in § 7.2.4.
7.2.1. Mini-cascades and the spectrum of tearing-mediated turbulence
Let us calculate the disruption scales ![]() $\lambda _n$, following Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b). Since the ‘mini-cascades’ that connect them are just the same as the aligned cascade whose disruption we analysed in § 7.1, we can use (7.2) to deduce a recursion relation
$\lambda _n$, following Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b). Since the ‘mini-cascades’ that connect them are just the same as the aligned cascade whose disruption we analysed in § 7.1, we can use (7.2) to deduce a recursion relation
(remembering that ![]() $\mathrm {Rm}$ was defined as the Lundquist number at scale
$\mathrm {Rm}$ was defined as the Lundquist number at scale ![]() $\lambda _{\mathrm {CB}}=\lambda _0$). To work out the Lundquist number
$\lambda _{\mathrm {CB}}=\lambda _0$). To work out the Lundquist number ![]() $S_{\lambda _n}$ at scale
$S_{\lambda _n}$ at scale ![]() $\lambda _n$, notice that, if the alignment angle
$\lambda _n$, notice that, if the alignment angle ![]() $\theta _{\lambda }$ just below
$\theta _{\lambda }$ just below ![]() $\lambda _n$ is increased, there must be a downward jump in the amplitude of the turbulent fluctuations at
$\lambda _n$ is increased, there must be a downward jump in the amplitude of the turbulent fluctuations at ![]() $\lambda _n$: indeed, the nonlinear time (6.4) shortens compared to what it was in the aligned cascade just above
$\lambda _n$: indeed, the nonlinear time (6.4) shortens compared to what it was in the aligned cascade just above ![]() $\lambda _n$, and the cascade accelerates. I shall consider the extreme possibility that the alignment is reset to being order unity, as this will effectively bracket the range of outcomes for the scalings of this cascade. Since it still has to carry the same energy flux, we have, for amplitudes just below the disruption scale (
$\lambda _n$, and the cascade accelerates. I shall consider the extreme possibility that the alignment is reset to being order unity, as this will effectively bracket the range of outcomes for the scalings of this cascade. Since it still has to carry the same energy flux, we have, for amplitudes just below the disruption scale (![]() $\lambda _n^-$),
$\lambda _n^-$),
 \begin{equation} \frac{\bigl(\delta Z_{\lambda_n^-}\bigr)^3}{\lambda_n}\sim\varepsilon \quad\Rightarrow\quad \delta Z_{\lambda_n^-} \sim (\varepsilon\lambda_n)^{1/3}, \end{equation}
\begin{equation} \frac{\bigl(\delta Z_{\lambda_n^-}\bigr)^3}{\lambda_n}\sim\varepsilon \quad\Rightarrow\quad \delta Z_{\lambda_n^-} \sim (\varepsilon\lambda_n)^{1/3}, \end{equation}
just a Kolmogorov (or GS95) scaling. Therefore, the Lundquist number of the ![]() $n$-th mini-cascade is
$n$-th mini-cascade is
 \begin{equation} S_{\lambda_n}\sim \frac{\delta Z_{\lambda_n^-}\lambda_n}{\eta} \sim \frac{\varepsilon^{1/3}\lambda_n^{4/3}}{\eta} \sim \mathrm{Rm}\left(\frac{\lambda_n}{\lambda_{\mathrm{CB}}}\right)^{4/3}. \end{equation}
\begin{equation} S_{\lambda_n}\sim \frac{\delta Z_{\lambda_n^-}\lambda_n}{\eta} \sim \frac{\varepsilon^{1/3}\lambda_n^{4/3}}{\eta} \sim \mathrm{Rm}\left(\frac{\lambda_n}{\lambda_{\mathrm{CB}}}\right)^{4/3}. \end{equation}In combination with (7.9), this gives us
I shall return to this obviously suggestive (Kolmogorov!) scaling in § 7.2.2.
In the picture that I have just painted, the cascade in the tearing-mediated range looks like a ladder (figure 14), with amplitude dropping at each successive disruption scale as structures become unaligned (or less aligned, in which case the the steps of the ladder are less tall). In between the disruption scales, there are aligned ‘mini-cascades’ of the same kind as the original one discussed in § 6.4, with ![]() $k_{\perp }^{-3/2}$ spectra. This means that the overall scaling of the turbulent fluctuation amplitudes can be constrained between their scaling just below each disruption scale (
$k_{\perp }^{-3/2}$ spectra. This means that the overall scaling of the turbulent fluctuation amplitudes can be constrained between their scaling just below each disruption scale (![]() $\lambda _n^-$), given by (7.10), and just above it (
$\lambda _n^-$), given by (7.10), and just above it (![]() $\lambda _n^+$). The latter scaling, for the amplitudes of the structures just before they get disrupted can be inferred from the fact that for these structures, the tearing growth rate (7.1) must be the same as the nonlinear interaction (cascade) rate: letting
$\lambda _n^+$). The latter scaling, for the amplitudes of the structures just before they get disrupted can be inferred from the fact that for these structures, the tearing growth rate (7.1) must be the same as the nonlinear interaction (cascade) rate: letting ![]() $v_{\mathrm {A}y}\sim \delta Z_{\lambda _n^+}$ in (7.1), we get
$v_{\mathrm {A}y}\sim \delta Z_{\lambda _n^+}$ in (7.1), we get
and, therefore,
 \begin{align} \frac{\bigl(\delta Z_{\lambda_n^+}\bigr)^2}{\tau_{\mathrm{nl}}} \sim \varepsilon\quad\Rightarrow\quad \delta Z_{\lambda_n^+} &\sim \varepsilon^{2/5}\eta^{{-}1/5}(1+\mathrm{Pm})^{1/10}\lambda_n^{3/5} \nonumber\\ &\sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4} \left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{3/5}. \end{align}
\begin{align} \frac{\bigl(\delta Z_{\lambda_n^+}\bigr)^2}{\tau_{\mathrm{nl}}} \sim \varepsilon\quad\Rightarrow\quad \delta Z_{\lambda_n^+} &\sim \varepsilon^{2/5}\eta^{{-}1/5}(1+\mathrm{Pm})^{1/10}\lambda_n^{3/5} \nonumber\\ &\sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4} \left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{3/5}. \end{align}The last expression puts this result explicitly in contact with the inertial-range scaling (6.22). Thus, the tearing-mediated-range spectrum is (Mallet et al. Reference Mallet, Schekochihin and Chandran2017b)Footnote 36
Since the ![]() $-11/5$ upper envelope is steeper than the
$-11/5$ upper envelope is steeper than the ![]() $-5/3$ lower one, the two converge and eventually meet at
$-5/3$ lower one, the two converge and eventually meet at
which is, of course, the scale (7.12) in the limit ![]() $n\to \infty$. For
$n\to \infty$. For ![]() $\mathrm {Pm}\gg 1$, this will, in fact, be superseded by the Kolmogorov cutoff to be derived in § 7.2.2.
$\mathrm {Pm}\gg 1$, this will, in fact, be superseded by the Kolmogorov cutoff to be derived in § 7.2.2.

Figure 14. Spectrum of MHD turbulence and transition to tearing-mediated cascade (see (7.15)) (adapted from Mallet et al. Reference Mallet, Schekochihin and Chandran2017b). The width of the tearing-mediated range is, of course, exaggerated in this cartoon. The spectral slopes of the ‘mini-cascades’ between ![]() $\lambda _n^{-1}$ and
$\lambda _n^{-1}$ and ![]() $\lambda _{n+1}^{-1}$ are all
$\lambda _{n+1}^{-1}$ are all ![]() $k_{\perp }^{-3/2}$, but the overall envelope is
$k_{\perp }^{-3/2}$, but the overall envelope is ![]() $k_{\perp }^{-11/5}$. Note that, modulo
$k_{\perp }^{-11/5}$. Note that, modulo ![]() $\mathrm {Pm}$ dependence, the disrupted aligned cascade and a putative unaligned GS95
$\mathrm {Pm}$ dependence, the disrupted aligned cascade and a putative unaligned GS95 ![]() $k_{\perp }^{-5/3}$ spectrum, starting at
$k_{\perp }^{-5/3}$ spectrum, starting at ![]() $\lambda _{\mathrm {CB}}$, terminate at the same, Kolmogorov, scale (7.19).
$\lambda _{\mathrm {CB}}$, terminate at the same, Kolmogorov, scale (7.19).
While in the above construction, the tearing-mediated-range spectrum is pictured as a succession of ‘steps’ representing the ‘mini-cascades’ that connect the successive disruption scales (figure 14), the reality will almost certainly look more like some overall power-law spectrum with a slope for which the upper ![]() $-11/5$ bound (7.15) seems to be a good estimate. Indeed, the tearing disruptions will be happening within intermittently distributed aligned structures of different amplitudes and sizes, on which the disruption scales will depend (Mallet et al. Reference Mallet, Schekochihin and Chandran2017b). Thus, each scale
$-11/5$ bound (7.15) seems to be a good estimate. Indeed, the tearing disruptions will be happening within intermittently distributed aligned structures of different amplitudes and sizes, on which the disruption scales will depend (Mallet et al. Reference Mallet, Schekochihin and Chandran2017b). Thus, each scale ![]() $\lambda _n$ will in fact be smeared over some range and, as the successive intervals
$\lambda _n$ will in fact be smeared over some range and, as the successive intervals ![]() $(\lambda _n,\lambda _{n+1})$ become narrower, this smear can easily exceed their width. Pending a detailed theory of intermittency in the tearing-mediated range, perhaps the best way to think of the spectrum and other scalings in this range is, therefore, in a ‘coarse-grained’ sense, focusing on the characteristic dependence of all interesting quantities on
$(\lambda _n,\lambda _{n+1})$ become narrower, this smear can easily exceed their width. Pending a detailed theory of intermittency in the tearing-mediated range, perhaps the best way to think of the spectrum and other scalings in this range is, therefore, in a ‘coarse-grained’ sense, focusing on the characteristic dependence of all interesting quantities on ![]() $\lambda _n$, treated as a continuous variable.
$\lambda _n$, treated as a continuous variable.
7.2.2. Restoration of Kolmogorov cutoff
To calculate the dissipative cutoff for the tearing-mediated cascade, one balances the larger of the viscous and resistive diffusion rates with the nonlinear cascade rate (7.13) (this is the longest of the nonlinear time scales involved): using (7.14), one gets
For ![]() $\mathrm {Pm}\lesssim 1$, this is the same scale as (7.16), where the
$\mathrm {Pm}\lesssim 1$, this is the same scale as (7.16), where the ![]() $-11/5$ and
$-11/5$ and ![]() $-5/3$ scalings meet; for
$-5/3$ scalings meet; for ![]() $\mathrm {Pm}\gg 1$, (7.17) is reached before (7.16). The condition for the range
$\mathrm {Pm}\gg 1$, (7.17) is reached before (7.16). The condition for the range ![]() $[\lambda _{\mathrm {D}},\lambda _{\eta }^\mathrm {K}]$ to be non-empty is
$[\lambda _{\mathrm {D}},\lambda _{\eta }^\mathrm {K}]$ to be non-empty is
 \begin{equation} \frac{\lambda_{\mathrm{D}}}{\lambda_{\eta}^\mathrm{tearing}}\sim\left[\frac{\mathrm{Rm}}{(1+\mathrm{Pm})^{10}}\right]^{5/28}\gg1. \end{equation}
\begin{equation} \frac{\lambda_{\mathrm{D}}}{\lambda_{\eta}^\mathrm{tearing}}\sim\left[\frac{\mathrm{Rm}}{(1+\mathrm{Pm})^{10}}\right]^{5/28}\gg1. \end{equation}This is less stringent than (7.3), so will always be satisfied if the disruption occurs in the first place.
The good news (or, at any rate, the news) is that Kolmogorov's scaling of the dissipative cutoff is rehabilitated for ![]() $\mathrm {Pm}\lesssim 1$. Notably, it is not quite rehabilitated for
$\mathrm {Pm}\lesssim 1$. Notably, it is not quite rehabilitated for ![]() $\mathrm {Pm}\gg 1$, but that is likely an illusion. Indeed, while viscosity destroys velocities, aligned magnetic structures survive unscathed (cf. § 11), so tearing can keep going in the viscously dominated regime. If it breaks up the aligned structures at the scale
$\mathrm {Pm}\gg 1$, but that is likely an illusion. Indeed, while viscosity destroys velocities, aligned magnetic structures survive unscathed (cf. § 11), so tearing can keep going in the viscously dominated regime. If it breaks up the aligned structures at the scale ![]() $\lambda _{\eta }^\mathrm {tearing}$ into flux ropes of the same width and lesser alignment, the turnover time of these structures will be shorter than the viscous-diffusion time – in just the same way as the debris of disruption had shorter turnover times than their mother sheets in § 7.2.1 – and the tearing-mediate cascade can continue. A rough estimate of how small the debris have to get to be killed completely by diffusion is to set their Lundquist number (7.11) obtained on the assumption of complete lack of alignment to
$\lambda _{\eta }^\mathrm {tearing}$ into flux ropes of the same width and lesser alignment, the turnover time of these structures will be shorter than the viscous-diffusion time – in just the same way as the debris of disruption had shorter turnover times than their mother sheets in § 7.2.1 – and the tearing-mediate cascade can continue. A rough estimate of how small the debris have to get to be killed completely by diffusion is to set their Lundquist number (7.11) obtained on the assumption of complete lack of alignment to ![]() $S_{\lambda _n}\sim 1+\mathrm {Pm}$ (in other words, the cutoff occurs when either
$S_{\lambda _n}\sim 1+\mathrm {Pm}$ (in other words, the cutoff occurs when either ![]() $\mathrm {Rm}$ or
$\mathrm {Rm}$ or ![]() $\mathrm {Re}$ associated with the
$\mathrm {Re}$ associated with the ![]() $\lambda _n$-scale structures is order unity). This gives
$\lambda _n$-scale structures is order unity). This gives
 \begin{equation} \lambda_{\eta}^\mathrm{K} \sim \lambda_{\mathrm{CB}} \left(\frac{\mathrm{Rm}}{1+\mathrm{Pm}}\right)^{{-}3/4} = \lambda_{\mathrm{CB}}\widetilde{\mathrm{Re}}^{{-}3/4} \sim \frac{(\nu+\eta)^{3/4}}{\varepsilon^{1/4}}, \end{equation}
\begin{equation} \lambda_{\eta}^\mathrm{K} \sim \lambda_{\mathrm{CB}} \left(\frac{\mathrm{Rm}}{1+\mathrm{Pm}}\right)^{{-}3/4} = \lambda_{\mathrm{CB}}\widetilde{\mathrm{Re}}^{{-}3/4} \sim \frac{(\nu+\eta)^{3/4}}{\varepsilon^{1/4}}, \end{equation}
where ![]() $\widetilde {\mathrm {Re}}$, defined in (6.24), is
$\widetilde {\mathrm {Re}}$, defined in (6.24), is ![]() $\mathrm {Rm}$ when
$\mathrm {Rm}$ when ![]() $\mathrm {Pm}\lesssim 1$ and
$\mathrm {Pm}\lesssim 1$ and ![]() $\mathrm {Re}$ when
$\mathrm {Re}$ when ![]() $\mathrm {Pm}\gg 1$. This is the proper, classic Kolmogorov scale.
$\mathrm {Pm}\gg 1$. This is the proper, classic Kolmogorov scale.
It is interesting to recall that it is the Kolmogorov scaling at (and of) the dissipation scale that was the strongest claim made by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019) on the basis of a convergence study of his numerical spectra (see § 6.3 and figure 10b). While he inferred from that an interpretation of these spectra as showing a ![]() $-5/3$ scaling in the inertial range, it is their convergence at the dissipative end of the resolved range that appeared to be the least negotiable feature of his work. He may well have been right. Indeed, his largest simulations (see figure 10) fall somewhere in between the condition (7.18) (
$-5/3$ scaling in the inertial range, it is their convergence at the dissipative end of the resolved range that appeared to be the least negotiable feature of his work. He may well have been right. Indeed, his largest simulations (see figure 10) fall somewhere in between the condition (7.18) (![]() $\lambda _{\mathrm {D}}/\lambda _{\eta }^\mathrm {K} \gtrsim 3$ would require perhaps
$\lambda _{\mathrm {D}}/\lambda _{\eta }^\mathrm {K} \gtrsim 3$ would require perhaps ![]() $\mathrm {Rm}\gtrsim 10^3$ at
$\mathrm {Rm}\gtrsim 10^3$ at ![]() $\mathrm {Pm}\sim 1$) and the more stringent condition (7.3) needed to stop Boldyrev's cutoff (6.24) from taking over (
$\mathrm {Pm}\sim 1$) and the more stringent condition (7.3) needed to stop Boldyrev's cutoff (6.24) from taking over (![]() $\lambda _{\mathrm {D}}/\lambda _{\eta } \gtrsim 3$ if
$\lambda _{\mathrm {D}}/\lambda _{\eta } \gtrsim 3$ if ![]() $\mathrm {Rm}\gtrsim 10^5$). Thus,
$\mathrm {Rm}\gtrsim 10^5$). Thus, ![]() $\lambda _{\mathrm {D}}$ in Beresnyak's simulations could not have been more than a factor of order unity larger than the dissipation scale. An optimist might argue that this could have been just about enough to pick up the Kolmogorov scaling of the latter.
$\lambda _{\mathrm {D}}$ in Beresnyak's simulations could not have been more than a factor of order unity larger than the dissipation scale. An optimist might argue that this could have been just about enough to pick up the Kolmogorov scaling of the latter.
7.2.3. Alignment in the tearing-mediated range
The structures corresponding to the lower (GS95) envelope (7.10) are unaligned, whereas the alignment corresponding to the upper envelope (7.14) is the tightest alignment sustainable in the tearing-mediated range and achieved by each aligned ‘mini-cascade’ just before it is disrupted by tearing at the scale ![]() $\lambda _n$. This is (cf. Boldyrev & Loureiro Reference Boldyrev and Loureiro2017)
$\lambda _n$. This is (cf. Boldyrev & Loureiro Reference Boldyrev and Loureiro2017)
 \begin{equation} \sin\theta_{\lambda_n^+} \sim \frac{\lambda_n/\delta Z_{\lambda_n^+}}{\tau_{\mathrm{nl}}} \sim \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{{-}4/5}. \end{equation}
\begin{equation} \sin\theta_{\lambda_n^+} \sim \frac{\lambda_n/\delta Z_{\lambda_n^+}}{\tau_{\mathrm{nl}}} \sim \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{{-}4/5}. \end{equation}Equivalently, the fluctuation-direction coherence scale is
 \begin{equation} \xi_n \sim \frac{\lambda_n}{\sin\theta_{\lambda_n^+}}\sim \lambda_{\mathrm{CB}} \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{3/4}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{9/5}. \end{equation}
\begin{equation} \xi_n \sim \frac{\lambda_n}{\sin\theta_{\lambda_n^+}}\sim \lambda_{\mathrm{CB}} \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{3/4}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{9/5}. \end{equation}
The corresponding spectral exponent is again ![]() $-5/3$, which is automatically the case given the definitions of
$-5/3$, which is automatically the case given the definitions of ![]() $\tau _{\mathrm {nl}}$,
$\tau _{\mathrm {nl}}$, ![]() $\theta _\lambda$ and
$\theta _\lambda$ and ![]() $\xi$ (see (6.4) and § 6.5).
$\xi$ (see (6.4) and § 6.5).
Thus, the smallest possible alignment angle, having reached its minimum at ![]() $\lambda _{\mathrm {D}}$, gets larger through the tearing-mediated range, according to (7.20). It becomes order unity at the scale (7.16), which is the same as the Kolmogorov cutoff (7.19) for
$\lambda _{\mathrm {D}}$, gets larger through the tearing-mediated range, according to (7.20). It becomes order unity at the scale (7.16), which is the same as the Kolmogorov cutoff (7.19) for ![]() $\mathrm {Pm}\lesssim 1$ and a bit smaller than it for
$\mathrm {Pm}\lesssim 1$ and a bit smaller than it for ![]() $\mathrm {Pm}\gg 1$ (but in fact there is no more alignment below the Kolmogorov cutoff).
$\mathrm {Pm}\gg 1$ (but in fact there is no more alignment below the Kolmogorov cutoff).
To the (doubtful) extent that existing numerical evidence can be considered to be probing this regime, perhaps we can take heart from the numerical papers by both Beresnyak and by Boldyrev's group cited in § 6.3 all reporting that alignment fades away at the small-scale end of the inertial range – although this may also be just a banal effect of the numerical resolution cutoff.
7.2.4. Parallel cascade in the tearing-mediated range
As ever, CB should be an enduring feature of our turbulence. This means that the parallel spectrum (5.1) will not notice the disruption scale and blithely extend all the way through the tearing-mediated range. Since the unaligned (or less aligned) flux ropes produced in the wake of the disruption of aligned structures have a shorter decorrelation time than their aligned progenitors, they should break up in the parallel direction (cf. Zhou, Loureiro & Uzdensky Reference Zhou, Loureiro and Uzdensky2020 and § 12.6). The resulting parallel coherence scale, the same as the scale (5.5) in the GS95 theory, is the lower bound on ![]() $l_{\parallel }$ at each
$l_{\parallel }$ at each ![]() $\lambda _n$. The upper bound can be inferred by equating the nonlinear time (7.13) at
$\lambda _n$. The upper bound can be inferred by equating the nonlinear time (7.13) at ![]() $\lambda _n$ to the Alfvén time
$\lambda _n$ to the Alfvén time ![]() $l_{\parallel }/v_{\mathrm {A}}$. The result is
$l_{\parallel }/v_{\mathrm {A}}$. The result is
 \begin{equation} v_{\mathrm{A}}\varepsilon^{{-}1/3}\lambda_n^{2/3}\lesssim l_{{\parallel}}\lesssim v_{\mathrm{A}}\varepsilon^{{-}1/5}\eta^{{-}2/5}(1+\mathrm{Pm})^{1/5}\lambda_n^{6/5} \sim L_{{\parallel}}\left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/2}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{6/5}. \end{equation}
\begin{equation} v_{\mathrm{A}}\varepsilon^{{-}1/3}\lambda_n^{2/3}\lesssim l_{{\parallel}}\lesssim v_{\mathrm{A}}\varepsilon^{{-}1/5}\eta^{{-}2/5}(1+\mathrm{Pm})^{1/5}\lambda_n^{6/5} \sim L_{{\parallel}}\left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/2}\left(\frac{\lambda_n}{\lambda_{\mathrm{D}}}\right)^{6/5}. \end{equation}
Thus, the upper bound on the parallel anisotropy ![]() $l_{\parallel }/\lambda$ decreases with scale in this range (turbulence becomes less anisotropic).
$l_{\parallel }/\lambda$ decreases with scale in this range (turbulence becomes less anisotropic).
7.3. Is this a falsifiable theory?
With considerable difficulty.
Numerically, anything like a definite confirmation of the tearing disruption (§ 7.1) and the existence of a tearing-mediated cascade (§ 7.2) requires formidably large simulations: the condition (7.3) demands ![]() $\mathrm {Rm}\sim 10^5$ at least (estimated via the frivolous but basically sound principle that the smallest large number is 3) – and probably quite a bit larger if one is to see the scaling of the tearing-mediated-range spectrum (7.15). However, as was mooted above, an optimist might find cause for optimism in the evidence of the MHD turbulence cutoff seen in simulations appearing to obey the Kolmogorov scaling (7.19) (see discussion in § 7.2.2) or in the numerically measured alignment petering out at the small-scale end of the inertial range, as required by § 7.2.3. While the trouble to which I have gone to keep track of the
$\mathrm {Rm}\sim 10^5$ at least (estimated via the frivolous but basically sound principle that the smallest large number is 3) – and probably quite a bit larger if one is to see the scaling of the tearing-mediated-range spectrum (7.15). However, as was mooted above, an optimist might find cause for optimism in the evidence of the MHD turbulence cutoff seen in simulations appearing to obey the Kolmogorov scaling (7.19) (see discussion in § 7.2.2) or in the numerically measured alignment petering out at the small-scale end of the inertial range, as required by § 7.2.3. While the trouble to which I have gone to keep track of the ![]() $\mathrm {Pm}$ dependence of the tearing-mediated-range quantities did not yield anything qualitatively spectacular, there is perhaps an opportunity here for numerical tests: e.g., can one obtain Boldyrev's scaling (6.24) of the dissipation cutoff in the limit of moderate
$\mathrm {Pm}$ dependence of the tearing-mediated-range quantities did not yield anything qualitatively spectacular, there is perhaps an opportunity here for numerical tests: e.g., can one obtain Boldyrev's scaling (6.24) of the dissipation cutoff in the limit of moderate ![]() $\mathrm {Rm}$ and large
$\mathrm {Rm}$ and large ![]() $\mathrm {Pm}$? – which, in view of (7.3), is unlikely to need to be very large to take over and shut down tearing.
$\mathrm {Pm}$? – which, in view of (7.3), is unlikely to need to be very large to take over and shut down tearing.
One way to circumvent the need for getting into a hyper-asymptotic regime is to simulate directly the dynamics of structures that resemble Alfvénic sheets deep in the inertial range. Such a study by Walker, Boldyrev & Loureiro (Reference Walker, Boldyrev and Loureiro2018), in 2D, of the decay of an Alfvénic ‘eddy’ highly anisotropic in the perpendicular plane, has shown it breaking up promisingly into plasmoids and giving rise to a steeper spectrum than exhibited by a larger-![]() $\mathrm {Rm}$ case where tearing was too slow. Dong et al. (Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018) went further and actually demonstrated a spectral break at the disruption scale and a
$\mathrm {Rm}$ case where tearing was too slow. Dong et al. (Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018) went further and actually demonstrated a spectral break at the disruption scale and a ![]() $k_{\perp }^{-11/5}$ spectrum below it, with sheets in a turbulent system very vividly breaking up into plasmoids (figure 15) – but still in 2D. So far so good, but the real prize is always for 3D. The present review has spent so long going through revisions that 3D results, of which I originally wrote in remote future tense, are in fact now on the brink of arriving, again due to Dong et al. (Reference Dong, Wang, Comisso, Huang and Bhattacharjee2021), who have performed the mother of all MHD simulations (with
$k_{\perp }^{-11/5}$ spectrum below it, with sheets in a turbulent system very vividly breaking up into plasmoids (figure 15) – but still in 2D. So far so good, but the real prize is always for 3D. The present review has spent so long going through revisions that 3D results, of which I originally wrote in remote future tense, are in fact now on the brink of arriving, again due to Dong et al. (Reference Dong, Wang, Comisso, Huang and Bhattacharjee2021), who have performed the mother of all MHD simulations (with ![]() $\mathrm {Rm}$ comfortably over
$\mathrm {Rm}$ comfortably over ![]() $10^5$) and are claiming to see a ‘subinertial range’ with a
$10^5$) and are claiming to see a ‘subinertial range’ with a ![]() $-11/5$ spectral slope, as per (7.15); the spectral break where this scaling starts appears to be in the right place,
$-11/5$ spectral slope, as per (7.15); the spectral break where this scaling starts appears to be in the right place, ![]() $\lambda _{\mathrm {D}}\propto \mathrm {Rm}^{-4/7}$ as per (7.2). If I read their results correctly (based on C. Dong's presentation at the 2021 APS DPP Meeting), hallelujah.
$\lambda _{\mathrm {D}}\propto \mathrm {Rm}^{-4/7}$ as per (7.2). If I read their results correctly (based on C. Dong's presentation at the 2021 APS DPP Meeting), hallelujah.

Figure 15. A snapshot of current density (![]() $j_z$) from a 2D,
$j_z$) from a 2D, ![]() $\mathrm {Rm}=10^6$ (64,000
$\mathrm {Rm}=10^6$ (64,000![]() $^2$) MHD simulation by Dong et al. (Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018) (reprinted with permission from Dong et al. Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018, copyright (2018) by the American Physical Society; I am grateful to C. Dong for letting me have the original figure file). Zoomed areas show sheets broken up into plasmoids. The 3D versions of these visualisations reported by Dong et al. (Reference Dong, Wang, Comisso, Huang and Bhattacharjee2021) look generally messier, with islands less clearly delineated (they look a bit like the flux ropes in figure 50), but not, at first glance, qualitatively different in a paradigm-changing way.
$^2$) MHD simulation by Dong et al. (Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018) (reprinted with permission from Dong et al. Reference Dong, Wang, Huang, Comisso and Bhattacharjee2018, copyright (2018) by the American Physical Society; I am grateful to C. Dong for letting me have the original figure file). Zoomed areas show sheets broken up into plasmoids. The 3D versions of these visualisations reported by Dong et al. (Reference Dong, Wang, Comisso, Huang and Bhattacharjee2021) look generally messier, with islands less clearly delineated (they look a bit like the flux ropes in figure 50), but not, at first glance, qualitatively different in a paradigm-changing way.
Observationally, our best bet for fine measurements of turbulence is the solar wind and the terrestrial magnetosphere (e.g., the magnetosheath). However, these are collisionless environments, so, before any triumphs of observational confirmation can be celebrated, all the resistive reconnection physics on which the tearing-mediated-range cascade depends needs to be amended for the cornucopia of kinetic effects that await at the small-scale end of the cascade (see § 14.1). Once the tearing disruption in MHD was proposed, such generalisations were the ripe, low-hanging fruit quite a lot of which was immediately picked (Mallet, Schekochihin & Chandran Reference Mallet, Schekochihin and Chandran2017a; Loureiro & Boldyrev Reference Loureiro and Boldyrev2017a; Boldyrev & Loureiro Reference Boldyrev and Loureiro2019; see also Loureiro & Boldyrev Reference Loureiro and Boldyrev2018, where these ideas were ported to pair plasmas).
Pending all this validation and verification, the tearing-mediated cascade remains a beautiful fantasy – but one must be grateful that after half a century of scrutiny, MHD turbulence still has such gifts to offer.
7.4. Tearing disruption, plasmoid chains, fast reconnection, and reconnection-driven turbulence
This section deals with what many readers might feel are fairly esoteric details. They are right – and so skipping straight to § 8 will not subtract much from their experience.
In what until recently was a separate strand of research, much interest in the reconnection community has focused on stochastic plasmoid chains that arise in current sheets susceptible to the plasmoid instability (a subspecies of tearing), where a lively population of islands (plasmoids) are born, grow, travel along the sheet with Alfvénic outflows, occasionally eat each other (coalesce),Footnote 37 and, as shown by Uzdensky, Loureiro & Schekochihin (Reference Uzdensky, Loureiro and Schekochihin2010), cause reconnection in the sheet that they inhabit to be fast,Footnote 38 meaning independent of ![]() $\eta$ as
$\eta$ as ![]() $\eta \to +0$ (a derivation of the plasmoid instability, a long list of references on stochastic plasmoid chains, and, in figure 46, an example of one, can be found in Appendix D.4.2; the Uzdensky et al. Reference Uzdensky, Loureiro and Schekochihin2010 argument is reproduced in Appendix D.6). A stochastic chain can be viewed as a kind of ‘1D turbulence’, and has some distinctive statistical properties (see Appendices D.6.1 and D.6.2). Should one imagine the disrupted aligned structures spawning multiple instances of such a turbulence, and does the simple theory presented in § 7.2 describe this situation or does it need to be revised to represent a superposition of many fast-reconnecting, plasmoid-infested sheets (as attempted in three different ways by Loureiro & Boldyrev Reference Loureiro and Boldyrev2017b, Reference Loureiro and Boldyrev2020 and Tenerani & Velli Reference Tenerani and Velli2020b)?
$\eta \to +0$ (a derivation of the plasmoid instability, a long list of references on stochastic plasmoid chains, and, in figure 46, an example of one, can be found in Appendix D.4.2; the Uzdensky et al. Reference Uzdensky, Loureiro and Schekochihin2010 argument is reproduced in Appendix D.6). A stochastic chain can be viewed as a kind of ‘1D turbulence’, and has some distinctive statistical properties (see Appendices D.6.1 and D.6.2). Should one imagine the disrupted aligned structures spawning multiple instances of such a turbulence, and does the simple theory presented in § 7.2 describe this situation or does it need to be revised to represent a superposition of many fast-reconnecting, plasmoid-infested sheets (as attempted in three different ways by Loureiro & Boldyrev Reference Loureiro and Boldyrev2017b, Reference Loureiro and Boldyrev2020 and Tenerani & Velli Reference Tenerani and Velli2020b)?
7.4.1. Nature of tearing disruption
In considering this problem, I first want to return to the question of what the ‘disruption’ of the aligned structures actually consists of. There are two lines of thinking on this, articulated most explicitly in the papers by Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b) and Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017), of which I have so far stuck with the former. Namely, at the end of § 7.1, I followed Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016), Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b) and Loureiro & Boldyrev (Reference Loureiro and Boldyrev2017b) in invoking the collapse of the ![]() $X$-points separating the tearing-mode islands as a means of consummating the disruption of the aligned structure – the theory of the tearing-mediated cascade in § 7.2 was then presented as a corollary of this view.
$X$-points separating the tearing-mode islands as a means of consummating the disruption of the aligned structure – the theory of the tearing-mediated cascade in § 7.2 was then presented as a corollary of this view.
The collapse of inter-island ![]() $X$-points is actually the first step in the formation of a stochastic chain. A nuance that I have previously elided is that comparing the
$X$-points is actually the first step in the formation of a stochastic chain. A nuance that I have previously elided is that comparing the ![]() $X$-point collapse rate (which is
$X$-point collapse rate (which is ![]() $\sim$ the tearing growth rate
$\sim$ the tearing growth rate ![]() $\gamma$) with the growth rate of a secondary tearing instability of the same
$\gamma$) with the growth rate of a secondary tearing instability of the same ![]() $X$-point shows that, at asymptotically large Lundquist numbers, the latter is always greater than the former. Therefore, the collapse may itself be disrupted by tearing, producing more islands and more
$X$-point shows that, at asymptotically large Lundquist numbers, the latter is always greater than the former. Therefore, the collapse may itself be disrupted by tearing, producing more islands and more ![]() $X$-points, followed by the collapse of those, also disrupted, and so on. My take on this recursive tearing is presented in Appendix D.5.2 (alongside a review of prior literature, starting with Shibata & Tanuma Reference Shibata and Tanuma2001). I argue there that the smaller-scale islands that are produced in this process are not energetically relevant and so we need not worry about including them to amend the ‘one-level’ scenario of tearing disruption presented in § 7.1.
$X$-points, followed by the collapse of those, also disrupted, and so on. My take on this recursive tearing is presented in Appendix D.5.2 (alongside a review of prior literature, starting with Shibata & Tanuma Reference Shibata and Tanuma2001). I argue there that the smaller-scale islands that are produced in this process are not energetically relevant and so we need not worry about including them to amend the ‘one-level’ scenario of tearing disruption presented in § 7.1.
The recursive tearing proceeds until inter-island current sheets are short enough to be stable, at which point the true nonlinear plasmoid chain can form, involving not just multiple tearings, but also nonlinear plasmoid growth by reconnection, their coalescence, and ejection from the sheet. While the multiscale statistics of such a chain may be different from that of a tearing-mediated cascade that I described in § 7.2, I assumed there, implicitly, that the chain could not survive for a long time, if at all: indeed, the characteristic time scale of the process of fully forming the sheet out of an aligned structure is the tearing time (![]() $\sim \gamma ^{-1}$), and the time to break apart that aligned structure by ideal-MHD dynamics is of the same order (
$\sim \gamma ^{-1}$), and the time to break apart that aligned structure by ideal-MHD dynamics is of the same order (![]() $\sim \tau _{\mathrm {nl}}$). So it seems that the ‘mother sheet’ (collapsed aligned structure) should break apart entirely shortly after (or even before) fully forming and release its plasmoids (flux ropes) into the general turbulent wilderness, where they are free to interact with each other or with anything else that comes along, and are thus no different from turbulent fluctuations of a particular size generically splashing around in a large nonlinear system. This gives rise to the ‘mini-cascades’ in § 7.2, with the overall
$\sim \tau _{\mathrm {nl}}$). So it seems that the ‘mother sheet’ (collapsed aligned structure) should break apart entirely shortly after (or even before) fully forming and release its plasmoids (flux ropes) into the general turbulent wilderness, where they are free to interact with each other or with anything else that comes along, and are thus no different from turbulent fluctuations of a particular size generically splashing around in a large nonlinear system. This gives rise to the ‘mini-cascades’ in § 7.2, with the overall ![]() $k_{\perp }^{-11/5}$ spectral envelope (7.15).
$k_{\perp }^{-11/5}$ spectral envelope (7.15).
Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017) also derive the ![]() $k_{\perp }^{-11/5}$ spectrum by using (7.13) as the operational prescription for the cascade time (
$k_{\perp }^{-11/5}$ spectrum by using (7.13) as the operational prescription for the cascade time (![]() $\tau _{\mathrm {nl}}\sim \gamma ^{-1}$ at each scale in the tearing-mediated range). However, they have a different narrative about what happens dynamically: they do not believe that inter-island
$\tau _{\mathrm {nl}}\sim \gamma ^{-1}$ at each scale in the tearing-mediated range). However, they have a different narrative about what happens dynamically: they do not believe that inter-island ![]() $X$-points ever collapse, but that, rather, the tearing mode upsets alignment by order unity, changing the effective nonlinear cascade rate to the tearing rate. This is based on the (correct) observation that the alignment angle at the disruption scale (7.2)
$X$-points ever collapse, but that, rather, the tearing mode upsets alignment by order unity, changing the effective nonlinear cascade rate to the tearing rate. This is based on the (correct) observation that the alignment angle at the disruption scale (7.2)
is the same (at least for ![]() $\mathrm {Pm}\lesssim 1$) as the angular distortion of the field line caused by the tearing perturbation at the onset of the nonlinear regime: indeed, using (7.7) and (7.4) at
$\mathrm {Pm}\lesssim 1$) as the angular distortion of the field line caused by the tearing perturbation at the onset of the nonlinear regime: indeed, using (7.7) and (7.4) at ![]() $\lambda =\lambda _{\mathrm {D}}$,
$\lambda =\lambda _{\mathrm {D}}$,
They think that this is enough to make the aligned structure ‘cascade’, in some unspecified manner, without much reconnection, production of flux ropes, etc. In Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020), they revise their view a little and allow that, since the collapse time, the tearing time and, therefore, the cascade time are comparable to each other, some aligned structures might, in fact, collapse into proper reconnecting sheets.Footnote 39
It is hard to say whether the two pictures outlined above represent a disagreement in substance or merely in the style of presentation. While in the Mallet et al. (Reference Mallet, Schekochihin and Chandran2017b) interpretation, the collapse of the inter-island ![]() $X$-points is the way in which the distortion of alignment caused by tearing leads to faster nonlinear break-up of the aligned structures, Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020) think this is not necessary but does happen with some finite probability. This might not be sufficiently quantifiable a difference to be testable.
$X$-points is the way in which the distortion of alignment caused by tearing leads to faster nonlinear break-up of the aligned structures, Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020) think this is not necessary but does happen with some finite probability. This might not be sufficiently quantifiable a difference to be testable.
7.4.2. Onset of fast reconnection
What may be consequential physically, however, is the onset of fast reconnection in those aligned, tearing structures that do manage to collapse into proper sheets. Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020) conjecture that when that happens, one should start worrying about the resulting reconnecting sheets making a difference to the nature of the tearing-mediated (now reconnection-mediated) turbulence.Footnote 40 In order for such a transition to be realisable, ![]() $\mathrm {Rm}$ must be large enough for the characteristic time of fast reconnection to be shorter than the cascade time:
$\mathrm {Rm}$ must be large enough for the characteristic time of fast reconnection to be shorter than the cascade time:
where the reconnection time has been estimated as the time for all of the flux in a magnetic structure of scale ![]() $\lambda$ to be reconnected, and
$\lambda$ to be reconnected, and ![]() $\epsilon _{\mathrm {rec}}\sim 10^{-2}(1+\mathrm {Pm})^{-1/2}$ is the dimensionless fast-reconnection rate in a Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010) plasmoid chain (see Appendix D.6), or some modified version of it appropriate for a turbulent environment. The estimate (7.25) simply says that if the alignment angle (inverse aspect ratio) of a structure manages to become smaller than the reconnection rate, such a structure might be capable of becoming a fast-reconnecting sheet.
$\epsilon _{\mathrm {rec}}\sim 10^{-2}(1+\mathrm {Pm})^{-1/2}$ is the dimensionless fast-reconnection rate in a Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010) plasmoid chain (see Appendix D.6), or some modified version of it appropriate for a turbulent environment. The estimate (7.25) simply says that if the alignment angle (inverse aspect ratio) of a structure manages to become smaller than the reconnection rate, such a structure might be capable of becoming a fast-reconnecting sheet.
Let us recall that the alignment angle decreases with ![]() $\lambda$ according to (6.22) in an aligned MHD cascade, reaches its minimum at the disruption scale
$\lambda$ according to (6.22) in an aligned MHD cascade, reaches its minimum at the disruption scale ![]() $\lambda _{\mathrm {D}}$ (given by (7.2)), and then increases with decreasing
$\lambda _{\mathrm {D}}$ (given by (7.2)), and then increases with decreasing ![]() $\lambda$ according to (7.20) in a tearing-mediated cascade. The condition (7.25) is realisable if
$\lambda$ according to (7.20) in a tearing-mediated cascade. The condition (7.25) is realisable if
 \begin{equation} \sin\theta_{\lambda_{\mathrm{D}}}\sim \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4} \lesssim \epsilon_{\mathrm{rec}} \quad\Leftrightarrow\quad \mathrm{Rm} \gtrsim \epsilon_{\mathrm{rec}}^{{-}7}(1+\mathrm{Pm})^{{-}1/2} \sim 10^{14}(1+\mathrm{Pm})^3. \end{equation}
\begin{equation} \sin\theta_{\lambda_{\mathrm{D}}}\sim \left(\frac{\lambda_{\mathrm{D}}}{\lambda_{\mathrm{CB}}}\right)^{1/4} \lesssim \epsilon_{\mathrm{rec}} \quad\Leftrightarrow\quad \mathrm{Rm} \gtrsim \epsilon_{\mathrm{rec}}^{{-}7}(1+\mathrm{Pm})^{{-}1/2} \sim 10^{14}(1+\mathrm{Pm})^3. \end{equation}
Obviously, this is never going to be numerically (or indeed experimentally) achievable for resistive MHD – unless ![]() $\epsilon _{\mathrm {rec}}$ is substantially enhanced in a turbulent setting (Loureiro & Boldyrev Reference Loureiro and Boldyrev2020 show that this ‘nonlinear-reconnecting’ regime might also be more easily accessible in certain kinetic settings).
$\epsilon _{\mathrm {rec}}$ is substantially enhanced in a turbulent setting (Loureiro & Boldyrev Reference Loureiro and Boldyrev2020 show that this ‘nonlinear-reconnecting’ regime might also be more easily accessible in certain kinetic settings).
In the asymptotic world where the condition (7.25) can be realised, it will be realised for ![]() $\lambda \in [\lambda _{\mathrm {rec}}^<,\lambda _{\mathrm {rec}}^>]$, with the two scales lying below and above
$\lambda \in [\lambda _{\mathrm {rec}}^<,\lambda _{\mathrm {rec}}^>]$, with the two scales lying below and above ![]() $\lambda _{\mathrm {D}}$: using (6.22) and (7.20) for the alignment angle in the aligned and tearing-mediated cascades, respectively, one gets
$\lambda _{\mathrm {D}}$: using (6.22) and (7.20) for the alignment angle in the aligned and tearing-mediated cascades, respectively, one gets
One might argue that ![]() $\lambda _{\mathrm {rec}}^>$ is irrelevant because at that scale ideal nonlinear interactions are faster than tearing, so the instability that assists in the formation of a plasmoid chain is too slow to get it going before the energy cascades to small scales (if this is not true, then reconnection-mediated turbulence sets in at
$\lambda _{\mathrm {rec}}^>$ is irrelevant because at that scale ideal nonlinear interactions are faster than tearing, so the instability that assists in the formation of a plasmoid chain is too slow to get it going before the energy cascades to small scales (if this is not true, then reconnection-mediated turbulence sets in at ![]() $\lambda _{\mathrm {rec}}^>$, an
$\lambda _{\mathrm {rec}}^>$, an ![]() $\mathrm {Rm}$-independent scale, putting a hard lower bound on the allowed alignment angles,
$\mathrm {Rm}$-independent scale, putting a hard lower bound on the allowed alignment angles, ![]() $\sin \theta _\lambda \gtrsim \epsilon _{\mathrm {rec}}$, and vindicating Beresnyak Reference Beresnyak2019). In contrast, the interval
$\sin \theta _\lambda \gtrsim \epsilon _{\mathrm {rec}}$, and vindicating Beresnyak Reference Beresnyak2019). In contrast, the interval ![]() $[\lambda _{\mathrm {D}},\lambda _{\mathrm {rec}}^<]$ is tearing-dominated, so plasmoid chains could be on the cards.
$[\lambda _{\mathrm {D}},\lambda _{\mathrm {rec}}^<]$ is tearing-dominated, so plasmoid chains could be on the cards.
What might turbulence in such a situation look like? To get some idea of that, it is perhaps wise to start with numerical evidence about turbulence in individual, ‘stand-alone’ plasmoid chains – a subject on which copious literature exists, quoted in Appendix D.4.2.
7.4.3. Reconnection-driven turbulence
Much of that literature describes 2D simulations, but there is a handful of papers dedicated to unstable sheets in 3D. In all of these 3D numerical experiments, a large-scale reconnecting configuration – a macroscopic sheet – was set up as an initial condition and/or driven by inflows/outflows from/to the boundaries of the domain, then went violently unstable, much more so than in 2D, and ended up looking like a strip of vigorous turbulence, rather than a quasi-1D chain (see Appendix D.6.3 for citations and further discussion). There does not appear to be any reason for such a configuration to stay together without external help, so it is likely that what we are witnessing in these numerical simulations is a version of a tearing-mediated cascading event prolonged by the bespoke numerical set-up and thus guaranteed to go into the fast-reconnecting regime.
If this is true, then such reconnection-driven MHD turbulence and turbulence in a homogeneous box into which energy is injected by a body force are different only inasmuch as any two different outer-scale, system-specific arrangements for stirring up turbulence are different. In the spirit of universality, it is hard to believe that small patches of a turbulent sheet would look any different in close-up than a generic box of MHD turbulence. One can imagine, however, that, due to the macroscopic ‘reconnection driving’ of the turbulence in a sheet, the turbulent cascade starts off at the outer scale already in a highly aligned, tearing-dominated, regime (Walker et al. Reference Walker, Boldyrev and Loureiro2018 was an explicit attempt to exploit this idea). Indeed, both Bárta et al. (Reference Bárta, Büchner, Karlický and Skála2011) and Huang & Bhattacharjee (Reference Huang and Bhattacharjee2016) see spectra somewhat steeper than ![]() $-2$, perhaps consistent with
$-2$, perhaps consistent with ![]() $-11/5 = -2.2$ (or with the
$-11/5 = -2.2$ (or with the ![]() $k_{\perp }^{-2}$ spectrum derived in Appendix D.6.2). In contrast, Beresnyak (Reference Beresnyak2017) and Kowal et al. (Reference Kowal, Falceta-Gonçalves, Lazarian and Vishniac2017) report small-scale statistics very similar to those found in standard MHD turbulence. Tenerani & Velli (Reference Tenerani and Velli2020b) find the same at a sufficient distance from the neutral line, whereas close to it, they see interesting anisotropic scalings dependent on the (component of) the field and the direction in which its variation is probed vis-à-vis the orientation of the sheet.
$k_{\perp }^{-2}$ spectrum derived in Appendix D.6.2). In contrast, Beresnyak (Reference Beresnyak2017) and Kowal et al. (Reference Kowal, Falceta-Gonçalves, Lazarian and Vishniac2017) report small-scale statistics very similar to those found in standard MHD turbulence. Tenerani & Velli (Reference Tenerani and Velli2020b) find the same at a sufficient distance from the neutral line, whereas close to it, they see interesting anisotropic scalings dependent on the (component of) the field and the direction in which its variation is probed vis-à-vis the orientation of the sheet.
Moving from turbulence in one sheet to an ensemble of turbulent sheets, Tenerani & Velli (Reference Tenerani and Velli2020b) speculate about the spectrum of a turbulence entirely dominated by reconnecting sheets filling a scale-dependent fraction of the volume, and arrive at ![]() $k_{\perp }^{-11/5}$ by an entirely different route, perhaps a coincidence. Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020), in pursuit of the same idea, amend
$k_{\perp }^{-11/5}$ by an entirely different route, perhaps a coincidence. Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020), in pursuit of the same idea, amend ![]() $k_{\perp }^{-11/5}$ to
$k_{\perp }^{-11/5}$ to ![]() $k_{\perp }^{-12/5}$ just by assuming the volume-filling fraction
$k_{\perp }^{-12/5}$ just by assuming the volume-filling fraction ![]() $\propto \lambda$ (sheets) for energy at every scale
$\propto \lambda$ (sheets) for energy at every scale ![]() $\lambda$ in an otherwise standard tearing-mediated cascade.
$\lambda$ in an otherwise standard tearing-mediated cascade.
This is the current state of affairs. I do not have a definitive contribution to make to the (still wide open) theory of reconnection-driven turbulence. In Appendix D.6.2, I show, following Bárta et al. (Reference Bárta, Skála, Karlický and Büchner2012) and Loureiro (Reference Loureiro2016), how to get a ![]() $k_{\perp }^{-2}$ spectrum for a stochastic plasmoid chain envisioned by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010). I then argue tentatively, in Appendix D.6.3, that if plasmoids (in 3D, flux ropes) all go unstable and thus drive small-scale turbulence, that turbulence should look like regular (possibly tearing-mediated) MHD turbulence, but with a very broad driving range featuring a
$k_{\perp }^{-2}$ spectrum for a stochastic plasmoid chain envisioned by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010). I then argue tentatively, in Appendix D.6.3, that if plasmoids (in 3D, flux ropes) all go unstable and thus drive small-scale turbulence, that turbulence should look like regular (possibly tearing-mediated) MHD turbulence, but with a very broad driving range featuring a ![]() $k_{\perp }^{-1}$ spectrum, in which turbulent motions are forced with an alignment angle independent of scale and equal to the (dimensionless) fast-reconnection rate
$k_{\perp }^{-1}$ spectrum, in which turbulent motions are forced with an alignment angle independent of scale and equal to the (dimensionless) fast-reconnection rate ![]() $\epsilon _{\mathrm {rec}}$. I have no evidence to back this up.
$\epsilon _{\mathrm {rec}}$. I have no evidence to back this up.
Finally, let me also flag here the possibility that a good example of reconnection-driven turbulence may be the inertial-range turbulence in magnetically dominated decaying MHD systems, where decay is controlled by reconnection in current sheets that separate outer-scale relaxed structures (see § 12; the discussion of spectra is in § 12.8). Another (although more uncertain) such example may be the saturated state of the turbulent dynamo (§ 13) if the scheme mooted in § 13.4.4 turns out to have merit.
I will let the subject drop at this point, with the parting message that the last word has not been written on the intermittency effects and the role of fast plasmoid reconnection in tearing-mediated turbulence.
8. Halfway summary
For the beginning is thought to be more than half of the whole, and many of the questions we ask are cleared up by it.
Aristotle, Nicomachean Ethics (translated by W. D. Ross)
8.1. Is this the end of the road?
It never quite is (see §§ 7.4.2–7.4.3 and the second part of this review, starting from § 9), but the basic story looks roughly complete for the first time in years, at least as far as forced, balanced RMHD turbulence is concerned. The principle of critical balance gave us a sound ideology for dealing with anisotropic turbulence in a system that supports propagation of waves (§ 5) and a firm prescription for the parallel spectrum (§ 5.2). The aligned cascade (§ 6) produced a plausible prediction for the perpendicular spectrum but used to have an air of unfinished business about it, both in the sense that it gave rise to a state that appeared unsustainable at asymptotically small scales and in view of the objections, physical and numerical, raised by Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019). With the revised interpretation of alignment as an intermittency effect (§ 6.4) and with the tearing-mediated cascade (§ 7) connecting the inertial-range, aligned cascade to the Kolmogorov cutoff (7.19), these issues appear to be satisfactorily resolved. In what is also an aesthetically pleasing development, the tearing-mediated cascade has emerged as an ingenious way in which MHD turbulence contrives to thermalise its energy while shedding the excessive alignment that ideal-MHD dynamics could not help producing in the inertial range. This development joins together in a most definite way the physics of turbulence and reconnection – arguably, this was always inevitable, but it is good that we now appear to have some grip on what happens specifically.
Before I move on to the miscellany of Part II, let me make a few comments about the robustness of the general picture presented above and its connection to other schools of thought on MHD turbulence.
8.2. What can go wrong?
It is only fair to spell out explicitly what is settled and what can go catastrophically wrong with this entire picture.
The principle of critical balance and, therefore, the theory of the parallel cascade (§ 5) are, in my view, quite safe. They are straightforward physically and have been quite convincingly verified both observationally and numerically (pace the ‘waves vs. structures’ confusion: see § 8.3.1). The CB approach also appears to offer an attractive and credible strategy for dealing with turbulence in wave-carrying systems other than MHD, in both plasmas and hydrodynamics (e.g., Cho & Lazarian Reference Cho and Lazarian2004; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Reference Schekochihin, Parker, Highcock, Dellar, Dorland and Hammett2016; Schekochihin, Kawazura & Barnes Reference Schekochihin, Kawazura and Barnes2019; Nazarenko & Schekochihin Reference Nazarenko and Schekochihin2011; Barnes, Parra & Schekochihin Reference Barnes, Parra and Schekochihin2011; Boldyrev et al. Reference Boldyrev, Horaites, Xia and Perez2013; Chen & Boldyrev Reference Chen and Boldyrev2017; Passot, Sulem & Tassi Reference Passot, Sulem and Tassi2017; Loureiro & Boldyrev Reference Loureiro and Boldyrev2018; Avsarkisov Reference Avsarkisov2020; Adkins et al. Reference Adkins, Schekochihin, Ivanov and Roach2022; Skoutnev Reference Skoutnev2022), further bolstering its claim to being a universal physical principle.
In contrast, the aligned perpendicular cascade (§ 6), its tearing disruption (§ 7.1) and replacement by a tearing-mediated cascade (§ 7.2), which have formed the bulk of my story so far, are hotly debated concepts. Arguably, it is still subject to verification (requiring currently inaccessible resolutions) that alignment is not a transient, large-scale feature, as Beresnyak (Reference Beresnyak2019) would have it. It seems to me that we do know, however, that if we stir up unaligned turbulence, it will get aligned at smaller scales (see numerical studies cited in § 6.3), so its possible transient nature can only be due to some secondary instability of the aligned structures. The picture presented above relied on this being the tearing instability – but it is not entirely impossible that it is, in fact, an ideal MHD instability, e.g., some version of Kelvin–Helmholtz (KH) instability. The difference is that tearing required resistivity and so the disruption scale ![]() $\lambda _{\mathrm {D}}\propto \eta ^{4/7}$ was asymptotically separated from the outer scale
$\lambda _{\mathrm {D}}\propto \eta ^{4/7}$ was asymptotically separated from the outer scale ![]() $\lambda _{\mathrm {CB}}$ (see (7.2)), whereas the KH instability would kick in at some
$\lambda _{\mathrm {CB}}$ (see (7.2)), whereas the KH instability would kick in at some ![]() $\lambda \sim$ a finite fraction of
$\lambda \sim$ a finite fraction of ![]() $\lambda _{\mathrm {CB}}$. The usual expectation is that the KH instability is quenched by the magnetic field (and indeed hence perhaps the statistical preponderance of current sheets over shear layers; see § 10.4), but this can in principle turn out not to be enough. If it does, ideal MHD will take care of limiting alignment, without alignment there will be no need for, or dynamical tendency to, the tearing-mediated remedy to it at small scales, and, presumably, we will be back to GS95, in which case I apologise to my readers for having wasted their time (this scenario is explained in another language at end of § 8.3.3 and at the beginning of Appendix D.7.4).
$\lambda _{\mathrm {CB}}$. The usual expectation is that the KH instability is quenched by the magnetic field (and indeed hence perhaps the statistical preponderance of current sheets over shear layers; see § 10.4), but this can in principle turn out not to be enough. If it does, ideal MHD will take care of limiting alignment, without alignment there will be no need for, or dynamical tendency to, the tearing-mediated remedy to it at small scales, and, presumably, we will be back to GS95, in which case I apologise to my readers for having wasted their time (this scenario is explained in another language at end of § 8.3.3 and at the beginning of Appendix D.7.4).
8.3. What is lost in translation?
This section is devoted to misunderstandings and arguments at cross purposes. As any vibrant, fast-evolving field, MHD turbulence is a subject talked about in many languages, and differences in vocabulary are sometimes mistaken for fundamental disagreements.
8.3.1. Waves vs. structures
In the minds of some enthusiasts of current sheets (or, generally, of ‘coherent structures’) in MHD turbulence, the distinction between the sheets (‘structures’) and critically balanced Alfvénic perturbations (‘waves’) has become a dichotomy between two allegedly incompatible paradigms of how energy is dissipated in MHD turbulence – in strongly dissipative structures or via wave damping. This is a misunderstanding that appears to be based on the incorrect perception of CB-based theories as requiring turbulence to be an ensemble of random-phased Alfvén waves, similar to WT (§ 4). No dichotomy, and certainly no mutual exclusivity, between waves and structures, in fact, exists: while Alfvénic perturbations retain certain properties associated with the linear-wave response, their turbulence is strong (which is the whole point of the CB principle) and the tendency to form sheets dynamic (mutual shearing of Elsasser fields: see Chandran et al. Reference Chandran, Schekochihin and Mallet2015; Howes Reference Howes2016). This nonlinear, intermittent dynamics perpendicular to ![]() $\boldsymbol {B}$ produces ‘structures’, while the linear wave-propagation physics gives them coherence along
$\boldsymbol {B}$ produces ‘structures’, while the linear wave-propagation physics gives them coherence along ![]() $\boldsymbol {B}$, via CB as a causality constraint (§ 5.1).
$\boldsymbol {B}$, via CB as a causality constraint (§ 5.1).
In the recent literature, the most systematic, and sensible, discussion of the ‘waves vs. structures’ issue can be found in Grošelj et al. (Reference Grošelj, Chen, Mallet, Samtaney, Schneider and Jenko2019) (although their focus is on the kinetic, rather than MHD, range of scales); the transition – and key differences – between wave turbulence and CB turbulence are illustrated very vividly in Meyrand et al. (Reference Meyrand, Galtier and Kiyani2016) (already referred to in § 4).
8.3.2. Cellularisation of turbulence
The interest in dissipative structures has its origin in the long history of thinking about MHD turbulence (and generally turbulence) in a language that is, at first glance, very different from the one in which the preceding sections of this review have been written. This line of thinking dates back to Montgomery, Turner & Vahala (Reference Montgomery, Turner and Vahala1978, Reference Montgomery, Turner and Vahala1979) and Matthaeus & Montgomery (Reference Matthaeus and Montgomery1980), who looked for thermal-equilibrium states in MHD turbulence, subject to conservation of various secondary invariants – e.g., in 3D, helicity and cross-helicity – and conjectured that MHD turbulence would tend to such equilibria patch-wise in space. By a classic variational argument, it is possible show that nonlinear interactions would be suppressed in the regions – ‘cells’ – of fixed helicity, which would host force-free magnetic fields (Taylor Reference Taylor1974). A fixed cross-helicity would push velocity and magnetic field into alignment with each other (which does indeed happen in decaying MHD turbulence: see, e.g., Matthaeus et al. Reference Matthaeus, Pouquet, Mininni, Dmitruk and Breech2008); if they were also equal to each other in amplitude, the result would be a pure Elsasser, non-interacting state. Global force-free or Elsasser states are usually not achievable (e.g., because the conserved net helicity and/or cross-helicity of the system are zero), so the cells would be separated by boundaries where intense nonlinear dynamics and hence dissipation would take place (e.g., current sheets). That then would be the structure of a turbulent state – relaxed cells plus nonlinear, dissipative structures on their boundaries (non-volume-filling, so intermittent).
A recent review of this philosophy, from an original source and with copious references, is Matthaeus et al. (Reference Matthaeus, Wan, Servidio, Greco, Osman, Oughton and Dmitruk2015) (a similar thinking, mostly applied to hydrodynamic turbulence, is reviewed, in a fascinatingly idiosyncratic way, by Levich Reference Levich2009). While this approach is most natural in the context of decaying (freely relaxing) turbulence (and does indeed work there: see § 12; vivid illustrations of this ‘cellularisation’ of turbulence are figures 25 and 26), similar ideas have been mooted with regard to inertial-range statistics of forced turbulence: see, e.g., the discussion in § 9.1 of Elsasser-balanced MHD turbulence proving to be a patchwork of locally imbalanced, heavily cross-helical regions (figure 17). Dynamic alignment à la Boldyrev (§ 6), its salient effect being the local reduction of nonlinearity in the MHD inertial range, was also originally argued by him (Boldyrev Reference Boldyrev2006) to have to do with conservation and cascading of cross-helicity (see footnote 16).
Thus, perhaps cellularisation and the critically balanced, aligned dynamics that I have described above are, in fact, the same thing said in two different languages. A clear translation between the two is, however, still to be articulated, especially the kind of translation that would add something genuinely new to our understanding of the subject.
8.3.3. Stochastic reconnection
I have touted the joining of MHD turbulence and reconnection theories as a key outcome of the developments described in §§ 6–7, but of course there exists another school of thought for which this very connection has been the defining mantra for many years, but which has been almost entirely decoupled from those developments. This school of thought, vaguely anticipated already by Matthaeus & Lamkin (Reference Matthaeus and Lamkin1985, Reference Matthaeus and Lamkin1986) (naturally so, given reconnection's role in the cellularisation picture discussed in § 8.3.2), was properly launched by Lazarian & Vishniac (Reference Lazarian and Vishniac1999) when they put forward the notion of ‘stochastic reconnection’. Appendix D.7 is my attempt to review this topic (in which task I was greatly helped by its interpretation by Eyink, Lazarian & Vishniac Reference Eyink, Lazarian and Vishniac2011) and its connection to, and lack of contradiction with, the main narrative presented above. Here I shall try to summarise it very briefly.
The notion of turbulent viscosity has been a mainstay of the theory of hydrodynamic turbulence for nearly as long as this theory has existed (see, e.g., the textbook by Davidson Reference Davidson2015). The idea is simply (but not trivially) that if we ‘coarse-grain’ the velocity field at some scale ![]() $\lambda$ in the middle of the inertial range (or even at the outer scale), the effect of all the motions at scales smaller than
$\lambda$ in the middle of the inertial range (or even at the outer scale), the effect of all the motions at scales smaller than ![]() $\lambda$ can be modelled, very crudely but surprisingly adequately, by an effective viscosity
$\lambda$ can be modelled, very crudely but surprisingly adequately, by an effective viscosity ![]() $\sim \delta u_\lambda \lambda$. This is because the hydrodynamic cascade is local and (in 3D) direct, so motions at scale
$\sim \delta u_\lambda \lambda$. This is because the hydrodynamic cascade is local and (in 3D) direct, so motions at scale ![]() $\lambda$ are broken up at the rate
$\lambda$ are broken up at the rate ![]() $\delta u_\lambda /\lambda = (\delta u_\lambda \lambda )/\lambda ^2$, which can be viewed as a renormalised diffusion of momentum. The rate at which momentum and energy are removed from scale
$\delta u_\lambda /\lambda = (\delta u_\lambda \lambda )/\lambda ^2$, which can be viewed as a renormalised diffusion of momentum. The rate at which momentum and energy are removed from scale ![]() $\lambda$ is entirely independent of the true molecular viscosity. In a similar way, an MHD ‘eddy’ at the outer scale or in the inertial range (but not, according to § 7, in the tearing-mediated range) will lose both its momentum and its magnetic flux on a time scale (
$\lambda$ is entirely independent of the true molecular viscosity. In a similar way, an MHD ‘eddy’ at the outer scale or in the inertial range (but not, according to § 7, in the tearing-mediated range) will lose both its momentum and its magnetic flux on a time scale (![]() $\tau _{\mathrm {nl}}$, much discussed in the above) that is entirely independent of the true viscosity and resistivity of the MHD fluid (Eyink Reference Eyink2015 argues this with careful attention to detail). Thus, just like there is a turbulent viscosity, there is also a turbulent resistivity, and so a turbulent reconnection with an
$\tau _{\mathrm {nl}}$, much discussed in the above) that is entirely independent of the true viscosity and resistivity of the MHD fluid (Eyink Reference Eyink2015 argues this with careful attention to detail). Thus, just like there is a turbulent viscosity, there is also a turbulent resistivity, and so a turbulent reconnection with an ![]() $\eta$-independent effective rate. This has some interesting and non-trivial consequences, both physical and mathematical, for a number of problems involving reconnection (see Appendix D.7, the review by Lazarian et al. Reference Lazarian, Eyink, Jafari, Kowal, Li, Xu and Vishniac2020, and references in both), but it does not mean that reconnection does not need
$\eta$-independent effective rate. This has some interesting and non-trivial consequences, both physical and mathematical, for a number of problems involving reconnection (see Appendix D.7, the review by Lazarian et al. Reference Lazarian, Eyink, Jafari, Kowal, Li, Xu and Vishniac2020, and references in both), but it does not mean that reconnection does not need ![]() $\eta$ or small scales – just that those small scales are reached at an
$\eta$ or small scales – just that those small scales are reached at an ![]() $\eta$-independent rate.
$\eta$-independent rate.
If the turbulent resistivity were isotropic in the plane perpendicular to the mean magnetic field, no alignment effect would be possible because the aligned structures would not need tearing to break up, and we would be back to § 8.2 – no alignment, no tearing-mediated cascade, no need for this review. That is, essentially, the view of Lazarian et al. (Reference Lazarian, Eyink, Jafari, Kowal, Li, Xu and Vishniac2020). I cannot prove that this is wrong, but I think that it is unlikely (especially given the numerical evidence announced by Dong et al. Reference Dong, Wang, Comisso, Huang and Bhattacharjee2021; see § 7.3). In Appendix D.7.4, I show why it is not logically inevitable, i.e., how stochastic reconnection à la Lazarian, Vishniac, and Eyink can be reconciled with an aligned cascade.
PART TWO: Imbalances and loose ends
As we know,
There are known knowns.
There are things we know we know.
We also know
There are known unknowns.
That is to say
We know there are some things
We do not know.
But there are also unknown unknowns,
The ones we don't know
We don't know.
D. H. Rumsfeld,Footnote 41
U.S. Department of Defense News Briefing,
12 February 2002
In the remainder of this review, I will survey some of what has been done, what remains to be done, and what, in my view, is worth doing regarding the regimes of MHD turbulence in which there is an imbalance either between the energies of the two Elsasser fields or between the kinetic and magnetic energy. Such situations are relevant – and indeed often more relevant – in many astrophysical contexts, but remain much less (or even less) well understood, than the nice, if somewhat fictional, case in which one can just assume ![]() $\delta Z_\lambda ^+\sim \delta Z_\lambda ^-\sim \delta b_\lambda \sim \delta u_\lambda$. Not only the cases of Elsasser (§ 9) and Alfvénic (§ 10) imbalance can be put in this class but also the distinct regimes of MHD turbulence that arise below the viscous scale (assuming large
$\delta Z_\lambda ^+\sim \delta Z_\lambda ^-\sim \delta b_\lambda \sim \delta u_\lambda$. Not only the cases of Elsasser (§ 9) and Alfvénic (§ 10) imbalance can be put in this class but also the distinct regimes of MHD turbulence that arise below the viscous scale (assuming large ![]() $\mathrm {Pm}$; § 11), or when the turbulence is allowed to decay freely (§ 12), or when no mean field is imposed (the saturated MHD dynamo; § 13). For the reader's reference, §§ 9.6, 10.4, 11, 12.6.2, and 13.3–13.4 contain some new results and arguments that have not been published elsewhere.
$\mathrm {Pm}$; § 11), or when the turbulence is allowed to decay freely (§ 12), or when no mean field is imposed (the saturated MHD dynamo; § 13). For the reader's reference, §§ 9.6, 10.4, 11, 12.6.2, and 13.3–13.4 contain some new results and arguments that have not been published elsewhere.
9. Imbalanced MHD turbulence
9.1. Imbalance global and local
Since both incompressible MHD and RMHD conserve two invariants – the total energy and cross-helicity – each of the two Elsasser fields ![]() $\boldsymbol {Z}_{\perp }^\pm$ has its own conserved energy (see (3.3)). The energy fluxes
$\boldsymbol {Z}_{\perp }^\pm$ has its own conserved energy (see (3.3)). The energy fluxes ![]() $\varepsilon ^\pm$ of these fields are, therefore, independent parameters of MHD turbulence. Setting them equal to each other makes arguments simpler, but does not, in general, correspond to physical reality, for a number of reasons.
$\varepsilon ^\pm$ of these fields are, therefore, independent parameters of MHD turbulence. Setting them equal to each other makes arguments simpler, but does not, in general, correspond to physical reality, for a number of reasons.
First, everyone's favourite case of directly measurable MHD turbulence is the solar wind, where the Alfvénic perturbations propagating away from the Sun are launched from the Sun (Roberts et al. Reference Roberts, Goldstein, Klein and Matthaeus1987), while the counterpropagating ones have to be supplied by some mechanism that is still under discussion and probably involves Alfvén-wave reflection as plasma density decreases outwards from the Sun (see Chandran & Perez Reference Chandran and Perez2019 and references therein). The counterpropagating component is usually energetically smaller, especially in the fast wind (Bruno & Carbone Reference Bruno and Carbone2013; Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020).
Secondly – and, for a theoretical physicist interested in universality, more importantly – it is an intrinsic property of MHD turbulence to develop regions of local imbalance. This can be understood dynamically as a desire to evolve towards an Elsasser state, ![]() $\boldsymbol {Z}_{\perp }^+=0$ or
$\boldsymbol {Z}_{\perp }^+=0$ or ![]() $\boldsymbol {Z}_{\perp }^-=0$, which is an exact solution of RMHD equations (confirmed in simulations of decaying RMHD turbulence; see § 12.7), or statistically as a tendency for the local dissipation rates
$\boldsymbol {Z}_{\perp }^-=0$, which is an exact solution of RMHD equations (confirmed in simulations of decaying RMHD turbulence; see § 12.7), or statistically as a tendency for the local dissipation rates ![]() $\varepsilon ^\pm$ to fluctuate in space – a mainstay of intermittency theories since Landau's famous objection (see Frisch Reference Frisch1995) to Kolmogorov (Reference Kolmogorov1941b) and the latter's response in the form of the refined similarity hypothesis, accepting a fluctuating
$\varepsilon ^\pm$ to fluctuate in space – a mainstay of intermittency theories since Landau's famous objection (see Frisch Reference Frisch1995) to Kolmogorov (Reference Kolmogorov1941b) and the latter's response in the form of the refined similarity hypothesis, accepting a fluctuating ![]() $\varepsilon$ (Kolmogorov Reference Kolmogorov1962) (the theories of intermittency for balanced MHD turbulence proposed by Chandran et al. Reference Chandran, Schekochihin and Mallet2015 and Mallet & Schekochihin Reference Mallet and Schekochihin2017, skimmed through in § 6.4.2, were based on the same premise). In this context, a complete intermittency theory for MHD turbulence must incorporate whatever local modification (if any) of the MHD cascade is caused by
$\varepsilon$ (Kolmogorov Reference Kolmogorov1962) (the theories of intermittency for balanced MHD turbulence proposed by Chandran et al. Reference Chandran, Schekochihin and Mallet2015 and Mallet & Schekochihin Reference Mallet and Schekochihin2017, skimmed through in § 6.4.2, were based on the same premise). In this context, a complete intermittency theory for MHD turbulence must incorporate whatever local modification (if any) of the MHD cascade is caused by ![]() $\varepsilon ^+\neq \varepsilon ^-$, something that no existing theory has as yet accomplished or attempted. Another influential school of thought on the root causes of local imbalance connects it to a tendency for the cross-helicity to be less vigorously cascaded than energy, leading to local enhancements of
$\varepsilon ^+\neq \varepsilon ^-$, something that no existing theory has as yet accomplished or attempted. Another influential school of thought on the root causes of local imbalance connects it to a tendency for the cross-helicity to be less vigorously cascaded than energy, leading to local enhancements of ![]() $\boldsymbol {u}_{\perp }\cdot \boldsymbol {b}_{\perp }$ (see footnotes 15 and 16 and references therein).
$\boldsymbol {u}_{\perp }\cdot \boldsymbol {b}_{\perp }$ (see footnotes 15 and 16 and references therein).
That an intimate connection must exist between any verifiable theory of MHD turbulence and local imbalance is well illustrated (in figure 16) by the following piece of observational analysis, rather noteworthy, in my (not impartial) view. Wicks et al. (Reference Wicks, Roberts, Mallet, Schekochihin, Horbury and Chen2013b) took a series of measurements by Wind spacecraft of magnetic and velocity perturbations in fast solar wind and sorted them according to the amount of imbalance, both Elsasser and Alfvénic (§ 10), at each scale. They then computed structure functions conditional on these imbalances. While the majority of perturbations were imbalanced one way or the other (or both), there was a subpopulation with ![]() $\delta Z^+_\lambda \sim \delta Z^-_\lambda \sim \delta b_\lambda \sim \delta u_\lambda$. Interestingly, the structure function restricted to this subpopulation had what seemed to be a robust GS95 scaling (corresponding to a
$\delta Z^+_\lambda \sim \delta Z^-_\lambda \sim \delta b_\lambda \sim \delta u_\lambda$. Interestingly, the structure function restricted to this subpopulation had what seemed to be a robust GS95 scaling (corresponding to a ![]() $k_{\perp }^{-5/3}$ spectrum), even though the structure functions of the imbalanced perturbations were consistent with Boldyrev's
$k_{\perp }^{-5/3}$ spectrum), even though the structure functions of the imbalanced perturbations were consistent with Boldyrev's ![]() $k_{\perp }^{-3/2}$ aligned-cascade scaling and indeed exhibited some alignment, unlike the GS95 population (although not necessarily an alignment with the theoretically desirable scale dependence: see footnote 21 and Wicks et al. Reference Wicks, Mallet, Horbury, Chen, Schekochihin and Mitchell2013a; Podesta & Borovsky Reference Podesta and Borovsky2010 reported analogous results, conditioning on the presence of cross-helicity only). It is important to recognise that imbalance and alignment of Elsasser fields do not automatically imply each other, so balanced fluctuations are not absolutely required to be unaligned, or aligned fluctuations to be imbalanced (see Appendix B.1). However, as I argued in § 6.4.2, dynamical alignment is an intermittency effect and so there may be a correlation between the emergence of imbalanced patches at ever smaller scales and Elsasser fields shearing each other into alignment (cf. Chandran et al. Reference Chandran, Schekochihin and Mallet2015).
$k_{\perp }^{-3/2}$ aligned-cascade scaling and indeed exhibited some alignment, unlike the GS95 population (although not necessarily an alignment with the theoretically desirable scale dependence: see footnote 21 and Wicks et al. Reference Wicks, Mallet, Horbury, Chen, Schekochihin and Mitchell2013a; Podesta & Borovsky Reference Podesta and Borovsky2010 reported analogous results, conditioning on the presence of cross-helicity only). It is important to recognise that imbalance and alignment of Elsasser fields do not automatically imply each other, so balanced fluctuations are not absolutely required to be unaligned, or aligned fluctuations to be imbalanced (see Appendix B.1). However, as I argued in § 6.4.2, dynamical alignment is an intermittency effect and so there may be a correlation between the emergence of imbalanced patches at ever smaller scales and Elsasser fields shearing each other into alignment (cf. Chandran et al. Reference Chandran, Schekochihin and Mallet2015).

Figure 16. (a) Distribution of normalised cross-helicity (![]() $\sigma _{\mathrm {c}}$) and residual energy (
$\sigma _{\mathrm {c}}$) and residual energy (![]() $\sigma _{\mathrm {r}}$) (defined in (B4)) in an interval of fast-solar-wind data taken by Wind spacecraft and analysed by Wicks et al. (Reference Wicks, Roberts, Mallet, Schekochihin, Horbury and Chen2013b), from whose paper both plots in this figure are taken (© AAS, reproduced with permission). (b) Structure functions corresponding to the total energy (sum of kinetic and magnetic) conditioned on values of
$\sigma _{\mathrm {r}}$) (defined in (B4)) in an interval of fast-solar-wind data taken by Wind spacecraft and analysed by Wicks et al. (Reference Wicks, Roberts, Mallet, Schekochihin, Horbury and Chen2013b), from whose paper both plots in this figure are taken (© AAS, reproduced with permission). (b) Structure functions corresponding to the total energy (sum of kinetic and magnetic) conditioned on values of ![]() $\sigma _{\mathrm {c}}$ and
$\sigma _{\mathrm {c}}$ and ![]() $\sigma _{\mathrm {r}}$ and corresponding to Regions 1 (balanced), 2 (Elsasser-imbalanced), and 3 (Alfvénically imbalanced towards magnetic perturbations), indicated in panel (a). The
$\sigma _{\mathrm {r}}$ and corresponding to Regions 1 (balanced), 2 (Elsasser-imbalanced), and 3 (Alfvénically imbalanced towards magnetic perturbations), indicated in panel (a). The ![]() $f^{-2/3}$ slope corresponds to a
$f^{-2/3}$ slope corresponds to a ![]() $k_{\perp }^{-5/3}$ spectrum, the
$k_{\perp }^{-5/3}$ spectrum, the ![]() $f^{-1/2}$ slope to a
$f^{-1/2}$ slope to a ![]() $k_{\perp }^{-3/2}$ one.
$k_{\perp }^{-3/2}$ one.
Intuitively then, since patches of imbalance are locally ubiquitous even in globally balanced turbulence (Perez & Boldyrev Reference Perez and Boldyrev2009; see figure 17) and since the theory of balanced turbulence described in § 6.4 incorporates intermittency effects in the form of alignment, we might expect that this allows for local imbalance – and, therefore, that mildly imbalanced turbulence might look largely similar to the balanced one. Indeed, how would perturbations in the middle of inertial range ‘know’ that the local imbalance they ‘see’ is local rather than global? Obviously, on average, there will not be an imbalance and so the results for ![]() $\delta Z_\lambda$ that one derives for balanced turbulence (§§ 6 and 7) are effectively averaged over the statistics of the stronger and weaker Elsasser fields – which of
$\delta Z_\lambda$ that one derives for balanced turbulence (§§ 6 and 7) are effectively averaged over the statistics of the stronger and weaker Elsasser fields – which of ![]() $\delta Z^+_\lambda$ and
$\delta Z^+_\lambda$ and ![]() $\delta Z^-_\lambda$ is which, depends on time and space.
$\delta Z^-_\lambda$ is which, depends on time and space.

Figure 17. Cosine of the angle between increments ![]() $\delta \boldsymbol {u}_{\boldsymbol {\lambda }}$ and
$\delta \boldsymbol {u}_{\boldsymbol {\lambda }}$ and ![]() $\delta \boldsymbol {b}_{\boldsymbol {\lambda }}$ in the
$\delta \boldsymbol {b}_{\boldsymbol {\lambda }}$ in the ![]() $(x,y)$ plane, for
$(x,y)$ plane, for ![]() $\lambda = L_{\perp }/6$ (left) and
$\lambda = L_{\perp }/6$ (left) and ![]() $\lambda = L_{\perp }/12$ (right, corresponding to the region demarcated by the white square within the left panel) in a balanced RMHD simulation by Perez & Boldyrev (Reference Perez and Boldyrev2009) (reprinted with permission from Perez & Boldyrev Reference Perez and Boldyrev2009, copyright (2009) by the American Physical Society). Since
$\lambda = L_{\perp }/12$ (right, corresponding to the region demarcated by the white square within the left panel) in a balanced RMHD simulation by Perez & Boldyrev (Reference Perez and Boldyrev2009) (reprinted with permission from Perez & Boldyrev Reference Perez and Boldyrev2009, copyright (2009) by the American Physical Society). Since ![]() $\delta \boldsymbol {u}_{\boldsymbol {\lambda }}\cdot \delta \boldsymbol {b}_{\boldsymbol {\lambda }} =\left (|\delta \boldsymbol {Z}^+_{\boldsymbol {\lambda }}|^2 - |\delta \boldsymbol {Z}^-_{\boldsymbol {\lambda }}|^2\right )/4$, this is an illustration of patchy local imbalance, as well as of local alignment between the velocity and magnetic field.
$\delta \boldsymbol {u}_{\boldsymbol {\lambda }}\cdot \delta \boldsymbol {b}_{\boldsymbol {\lambda }} =\left (|\delta \boldsymbol {Z}^+_{\boldsymbol {\lambda }}|^2 - |\delta \boldsymbol {Z}^-_{\boldsymbol {\lambda }}|^2\right )/4$, this is an illustration of patchy local imbalance, as well as of local alignment between the velocity and magnetic field.
If we now allow ![]() $\varepsilon ^+>\varepsilon ^-$ on average, it becomes reasonable to expect
$\varepsilon ^+>\varepsilon ^-$ on average, it becomes reasonable to expect ![]() $\delta Z^+_\lambda > \delta Z^-_\lambda$ nearly everywhere or, at least, typically – unless
$\delta Z^+_\lambda > \delta Z^-_\lambda$ nearly everywhere or, at least, typically – unless ![]() $\varepsilon ^+/\varepsilon ^-$ is close enough to unity that fluctuations of local imbalance overwhelm the overall global one. In the latter case, presumably the global imbalance does not matter – at any rate, in the balanced considerations of §§ 6 and 7, we only ever required
$\varepsilon ^+/\varepsilon ^-$ is close enough to unity that fluctuations of local imbalance overwhelm the overall global one. In the latter case, presumably the global imbalance does not matter – at any rate, in the balanced considerations of §§ 6 and 7, we only ever required ![]() $\varepsilon ^+\sim \varepsilon ^-$, rather than
$\varepsilon ^+\sim \varepsilon ^-$, rather than ![]() $\varepsilon ^+=\varepsilon ^-$ exactly. What I am driving at here, perhaps with too much faffing about, is the rather obvious point that it is only the limit of strong imbalance,
$\varepsilon ^+=\varepsilon ^-$ exactly. What I am driving at here, perhaps with too much faffing about, is the rather obvious point that it is only the limit of strong imbalance, ![]() $\varepsilon ^+\gg \varepsilon ^-$, that can be expected to be physically distinct, in a qualitative manner, from the balanced regime.
$\varepsilon ^+\gg \varepsilon ^-$, that can be expected to be physically distinct, in a qualitative manner, from the balanced regime.
9.2. Numerical and observational evidence
As usual, it is this most interesting limit that is also the hardest to resolve numerically and so we have little definitive information as to what happens in the strongly imbalanced regime. As in the case of the spectra of balanced turbulence, the debate about the numerical evidence regarding the imbalanced cascade and its correct theoretical interpretation has been dominated by the antagonistic symbiosis of Beresnyak and Boldyrev, so it is from their papers (Beresnyak & Lazarian Reference Beresnyak and Lazarian2008, Reference Beresnyak and Lazarian2009b, Reference Beresnyak and Lazarian2010; Beresnyak Reference Beresnyak2019; Perez & Boldyrev Reference Perez and Boldyrev2009, Reference Perez and Boldyrev2010a,Reference Perez and Boldyrevb) that I derive much of the information reviewed below. Perez & Boldyrev (Reference Perez and Boldyrev2010a,Reference Perez and Boldyrevb) argue that large imbalances are unresolvable and refuse to simulate them. Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b, Reference Beresnyak and Lazarian2010) do not necessarily disagree with this, but believe that useful things can still be learned from strongly imbalanced simulations, even if imperfectly resolved. Based (mostly) on both groups’ simulations, imbalanced MHD turbulence appears to exhibit the following distinctive features (which I recount with a degree of confidence as they have been reproduced in two sets of independent, unpublished RMHD simulations by Mallet & Schekochihin Reference Mallet and Schekochihin2011 and by Meyrand & Squire Reference Meyrand and Squire2020).
(i) The stronger field has a steeper spectrum than the weaker one, with the former steeper and the latter shallower than the standard balanced-case spectra (figure 18a). However, it is fairly certain that these spectra are not converged with resolution: as resolution is increased, the tendency appears to be for the spectral slopes to get closer to each other, both when the imbalance is weak (Perez & Boldyrev Reference Perez and Boldyrev2010a) and when it is strong (Mallet & Schekochihin Reference Mallet and Schekochihin2011). This led Perez & Boldyrev (Reference Perez and Boldyrev2010a) to argue that numerical evidence was consistent with the two fields having the same spectral slope in the asymptotic limit of infinite Reynolds numbers. There is no agreement as to whether the two fields’ spectra might be ‘pinned’ (i.e., equal) to each other at the dissipation scale: ‘yes’, it seems, in weakly imbalanced simulations of Perez & Boldyrev (Reference Perez and Boldyrev2010a), ‘no’ in the strongly imbalanced ones of Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) and Meyrand & Squire (Reference Meyrand and Squire2020).Footnote 42 In fact, in the latter studies, the dissipation scales of the two fields do not appear to be the same: larger for the stronger field, smaller for the weaker field (see figure 18a and figure 35c).

Figure 18. A typical MHD simulation with large imbalance: (a) spectra, (b) anisotropy, ![]() $l_{\parallel }^\pm$ vs.
$l_{\parallel }^\pm$ vs. ![]() $\lambda$. These plots are adapted from Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) (© AAS, reproduced with permission). Mallet & Schekochihin (Reference Mallet and Schekochihin2011) and Meyrand & Squire (Reference Meyrand and Squire2020) have qualitatively similar results (although the difference in slopes between the weaker and the stronger fields’ spectra is much smaller in the higher-resolution simulations of Meyrand & Squire Reference Meyrand and Squire2020 – see an example of that in figure 35c, taken from Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021).
$\lambda$. These plots are adapted from Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) (© AAS, reproduced with permission). Mallet & Schekochihin (Reference Mallet and Schekochihin2011) and Meyrand & Squire (Reference Meyrand and Squire2020) have qualitatively similar results (although the difference in slopes between the weaker and the stronger fields’ spectra is much smaller in the higher-resolution simulations of Meyrand & Squire Reference Meyrand and Squire2020 – see an example of that in figure 35c, taken from Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021).
(ii) The ratio of stronger to weaker field's energies, a crude outer-scale quantity that Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008, Reference Beresnyak and Lazarian2009b, Reference Beresnyak and Lazarian2010) argue (reasonably, in my view) to be more likely to be numerically converged than inertial-range scalings, scales very strongly with ![]() $\varepsilon ^+/\varepsilon ^-$: it increases at least as fast as
$\varepsilon ^+/\varepsilon ^-$: it increases at least as fast as
 \begin{equation} \frac{\langle|\boldsymbol{Z}_{{\perp}}^+|^2\rangle}{\langle|\boldsymbol{Z}_{{\perp}}^-|^2\rangle} \sim \left(\frac{\varepsilon^+}{\varepsilon^-}\right)^2 \end{equation}
\begin{equation} \frac{\langle|\boldsymbol{Z}_{{\perp}}^+|^2\rangle}{\langle|\boldsymbol{Z}_{{\perp}}^-|^2\rangle} \sim \left(\frac{\varepsilon^+}{\varepsilon^-}\right)^2 \end{equation}
and possibly faster (which is inconsistent with the theory of Perez & Boldyrev Reference Perez and Boldyrev2009, another casus belli for the two groups; see § 9.4). Mallet & Schekochihin (Reference Mallet and Schekochihin2011, see figure 19) and Meyrand & Squire (Reference Meyrand and Squire2020) found the same scaling in their simulations for values of ![]() $\varepsilon ^+/\varepsilon ^-$ up to 10 (simulations with much higher imbalance are numerically suspect). In fact, early numerical evidence for (9.1) appears already in the (decaying) simulations of Verma et al. (Reference Verma, Roberts, Goldstein, Ghosh and Stribling1996) (see § 12.7).
$\varepsilon ^+/\varepsilon ^-$ up to 10 (simulations with much higher imbalance are numerically suspect). In fact, early numerical evidence for (9.1) appears already in the (decaying) simulations of Verma et al. (Reference Verma, Roberts, Goldstein, Ghosh and Stribling1996) (see § 12.7).

Figure 19. Scalings found in (unpublished) ![]() $512^3$ RMHD numerical simulations by Mallet & Schekochihin (Reference Mallet and Schekochihin2011): perpendicular (parallel) spectral indices
$512^3$ RMHD numerical simulations by Mallet & Schekochihin (Reference Mallet and Schekochihin2011): perpendicular (parallel) spectral indices ![]() $\mu _\perp$ (
$\mu _\perp$ (![]() $\mu _\parallel$) (inferred from structure functions calculated as explained in § 5.3) for both fields, denoted by the
$\mu _\parallel$) (inferred from structure functions calculated as explained in § 5.3) for both fields, denoted by the ![]() $\pm$ superscripts. In terms of the scaling exponents
$\pm$ superscripts. In terms of the scaling exponents ![]() $\gamma ^\pm _{\perp,\parallel }$ of the field increments (
$\gamma ^\pm _{\perp,\parallel }$ of the field increments (![]() $\delta Z^\pm _\lambda \propto \lambda ^{\gamma ^\pm _\perp }$,
$\delta Z^\pm _\lambda \propto \lambda ^{\gamma ^\pm _\perp }$, ![]() $\delta Z^\pm _{l_{\parallel }}\propto l _{\parallel }^{\gamma ^\pm _\parallel }$), these are
$\delta Z^\pm _{l_{\parallel }}\propto l _{\parallel }^{\gamma ^\pm _\parallel }$), these are ![]() $\mu ^\pm _{\perp,\parallel } = -2\gamma ^\pm _{\perp,\parallel } - 1$. The last column shows the overall Elsasser ratio
$\mu ^\pm _{\perp,\parallel } = -2\gamma ^\pm _{\perp,\parallel } - 1$. The last column shows the overall Elsasser ratio ![]() $R_{\mathrm {E}}=\langle |\boldsymbol {Z}_{\perp }^+|^2\rangle /\langle |\boldsymbol {Z}_{\perp }^-|^2\rangle$. The parallel scalings of the weaker field were converged with resolution, while the perpendicular scalings of the stronger (weaker) field at
$R_{\mathrm {E}}=\langle |\boldsymbol {Z}_{\perp }^+|^2\rangle /\langle |\boldsymbol {Z}_{\perp }^-|^2\rangle$. The parallel scalings of the weaker field were converged with resolution, while the perpendicular scalings of the stronger (weaker) field at ![]() $\varepsilon ^+/\varepsilon ^- = 10$ became shallower (steeper) as resolution was increased from
$\varepsilon ^+/\varepsilon ^- = 10$ became shallower (steeper) as resolution was increased from ![]() $256^3$ to
$256^3$ to ![]() $512^3$ to
$512^3$ to ![]() $1024^2\times 512$; the parallel scaling of the stronger field also appeared to become shallower. Simulations with
$1024^2\times 512$; the parallel scaling of the stronger field also appeared to become shallower. Simulations with ![]() $\varepsilon ^+/\varepsilon ^- = 100, 1000$ should be viewed as numerically suspect.
$\varepsilon ^+/\varepsilon ^- = 100, 1000$ should be viewed as numerically suspect.
(iii) According to Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008, Reference Beresnyak and Lazarian2009b) and Meyrand & Squire (Reference Meyrand and Squire2020), the stronger field is less anisotropic than the weaker one, in the sense that ![]() $l_{\parallel }^+< l_{\parallel }^-$ and
$l_{\parallel }^+< l_{\parallel }^-$ and ![]() $l_{\parallel }^+$ drops faster with
$l_{\parallel }^+$ drops faster with ![]() $\lambda$ than
$\lambda$ than ![]() $l_{\parallel }^-$ (figure 18b). Beresnyak (Reference Beresnyak2019) notes that this is true in his simulations even though he forces the two fields with the same parallel scale, i.e., given an opportunity to keep
$l_{\parallel }^-$ (figure 18b). Beresnyak (Reference Beresnyak2019) notes that this is true in his simulations even though he forces the two fields with the same parallel scale, i.e., given an opportunity to keep ![]() $l_{\parallel }^+=l_{\parallel }^-$, the system refuses to do so.
$l_{\parallel }^+=l_{\parallel }^-$, the system refuses to do so.
(iv) Mallet & Schekochihin (Reference Mallet and Schekochihin2011, see figure 19) found that the parallel spectrum of the weaker field (measured via its local-field-parallel structure function, as in § 5.3) was very robustly ![]() $k_{\parallel }^{-2}$ – to be precise, the exponent varied between
$k_{\parallel }^{-2}$ – to be precise, the exponent varied between ![]() $-1.9$ and
$-1.9$ and ![]() $-2.1$, but in a manner that evinced no systematic dependence on
$-2.1$, but in a manner that evinced no systematic dependence on ![]() $\varepsilon ^+/\varepsilon ^-$. For the stronger field, they found a gradual steepening of the parallel spectrum with higher imbalance.
$\varepsilon ^+/\varepsilon ^-$. For the stronger field, they found a gradual steepening of the parallel spectrum with higher imbalance.
(v) Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) found that the alignment angle between the Elsasser fields, defined as ![]() $\sin \theta$ in (B1), with numerator and denominator averaged separately, decreased with scale roughly as
$\sin \theta$ in (B1), with numerator and denominator averaged separately, decreased with scale roughly as ![]() $\lambda ^{0.1}$, independently of the degree of imbalance. Mallet & Schekochihin (Reference Mallet and Schekochihin2011) measured the same exponent, quite robustly for a wide range of imbalances, but noticed also that the scaling exponent depended on the definition of the ‘alignment angle’: e.g., if root-mean-square numerator and denominator were used, the scaling was
$\lambda ^{0.1}$, independently of the degree of imbalance. Mallet & Schekochihin (Reference Mallet and Schekochihin2011) measured the same exponent, quite robustly for a wide range of imbalances, but noticed also that the scaling exponent depended on the definition of the ‘alignment angle’: e.g., if root-mean-square numerator and denominator were used, the scaling was ![]() $\lambda ^{0.2\dots 0.25}$, closer to the familiar theoretical result (6.20). This is not special to the imbalanced cascades – the same is true in balanced turbulence (Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016).
$\lambda ^{0.2\dots 0.25}$, closer to the familiar theoretical result (6.20). This is not special to the imbalanced cascades – the same is true in balanced turbulence (Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016).
(vi) The observational picture is only just emerging. A steeper scaling for the stronger field noted in item (i) appears to be consistent with the structure functions measured in the fast solar wind by, e.g., Wicks et al. (Reference Wicks, Horbury, Chen and Schekochihin2011), although, besides this, they also exhibit low Alfvén ratio (see § 10), which simulations do not, and a rather-hard-to-interpret (or, possibly, to trust) scale dependence of the anisotropy. In contrast, Podesta & Borovsky (Reference Podesta and Borovsky2010) report a scale-independent Elsasser ratio and ![]() $k^{-3/2}$ spectra for both fields in a number of reasonably imbalanced cases of solar-wind turbulence at 1 AU. The same result has been reported by Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) from the very recent measurements by the Parker Solar Probe made closer to the Sun, where the imbalance gets larger (
$k^{-3/2}$ spectra for both fields in a number of reasonably imbalanced cases of solar-wind turbulence at 1 AU. The same result has been reported by Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) from the very recent measurements by the Parker Solar Probe made closer to the Sun, where the imbalance gets larger (![]() $\langle |\boldsymbol {Z}^+|^2\rangle /\langle |\boldsymbol {Z}^-|^2\rangle \approx 15$) – this may be damning for any theory or simulation where the two fields’ spectra scale differently, at least insomuch as these theories or simulations aspire to apply to the solar wind.
$\langle |\boldsymbol {Z}^+|^2\rangle /\langle |\boldsymbol {Z}^-|^2\rangle \approx 15$) – this may be damning for any theory or simulation where the two fields’ spectra scale differently, at least insomuch as these theories or simulations aspire to apply to the solar wind.
(vii) As the solar wind offers practically the only chance of observational testing of theory – a chance greatly enhanced by the launch of the Parker Solar Probe – there is a growing industry of direct numerical modelling of the generation of inward-propagating (![]() $\boldsymbol {Z}^-$) perturbations by reflection of the outward-propagating ones (
$\boldsymbol {Z}^-$) perturbations by reflection of the outward-propagating ones (![]() $\boldsymbol {Z}^+$), which is what is supposed to happen in the expanding solar wind. The latest and most sophisticated study of this kind is Chandran & Perez (Reference Chandran and Perez2019) (who also provide an excellent overview of previous work). Their results appear to be quite different from the idealised periodic-box, artificially-forced-turbulence studies discussed above: the stronger field's spectrum is actually shallower than the weaker one's (sometimes as shallow as
$\boldsymbol {Z}^+$), which is what is supposed to happen in the expanding solar wind. The latest and most sophisticated study of this kind is Chandran & Perez (Reference Chandran and Perez2019) (who also provide an excellent overview of previous work). Their results appear to be quite different from the idealised periodic-box, artificially-forced-turbulence studies discussed above: the stronger field's spectrum is actually shallower than the weaker one's (sometimes as shallow as ![]() $k^{-1}$), but both asymptote towards
$k^{-1}$), but both asymptote towards ![]() $k^{-3/2}$ with increasing heliocentric distance – good news for modelling, in view of what Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) have found. Chandran & Perez (Reference Chandran and Perez2019) acknowledge, however, that they can break these results by fiddling with how their turbulence is forced in the photosphere. Thus, the nature of large-scale energy injection appears to matter,Footnote 43 at least at finite resolutions, perhaps reinforcing the doubts expressed above about the convergence of even the more idealised simulations.
$k^{-3/2}$ with increasing heliocentric distance – good news for modelling, in view of what Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) have found. Chandran & Perez (Reference Chandran and Perez2019) acknowledge, however, that they can break these results by fiddling with how their turbulence is forced in the photosphere. Thus, the nature of large-scale energy injection appears to matter,Footnote 43 at least at finite resolutions, perhaps reinforcing the doubts expressed above about the convergence of even the more idealised simulations.
For a short while still, the field appears set to remain open to enterprising theoreticians.
9.3. Lithwick et al. (Reference Lithwick, Goldreich and Sridhar2007)
In what follows, in view of the discussion in § 6.1 and in Appendix B.2, I shall stick with my use of the Elsasser-field alignment angle ![]() $\theta$ in the expression (6.4) for
$\theta$ in the expression (6.4) for ![]() $\tau _{\mathrm {nl}}^{\pm }$. This angle is obviously the same for both fields, so
$\tau _{\mathrm {nl}}^{\pm }$. This angle is obviously the same for both fields, so
 \begin{equation} \tau_{\mathrm{nl}}^\pm \sim \frac{\lambda}{\delta Z^\mp_\lambda\sin\theta_\lambda}\quad\Rightarrow\quad \frac{\tau_{\mathrm{nl}}^+}{\tau_{\mathrm{nl}}^-} \sim \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} > 1, \end{equation}
\begin{equation} \tau_{\mathrm{nl}}^\pm \sim \frac{\lambda}{\delta Z^\mp_\lambda\sin\theta_\lambda}\quad\Rightarrow\quad \frac{\tau_{\mathrm{nl}}^+}{\tau_{\mathrm{nl}}^-} \sim \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} > 1, \end{equation}i.e., the cascade of the stronger field is slower (because it is advected by the weaker field). Assuming nevertheless that both cascades are strong, we infer immediately
 \begin{equation} \frac{(\delta Z^\pm_\lambda)^2}{\tau_{\mathrm{nl}}^\pm} \sim \varepsilon^\pm\quad\Rightarrow\quad \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} \sim \frac{\varepsilon^+}{\varepsilon^-}. \end{equation}
\begin{equation} \frac{(\delta Z^\pm_\lambda)^2}{\tau_{\mathrm{nl}}^\pm} \sim \varepsilon^\pm\quad\Rightarrow\quad \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} \sim \frac{\varepsilon^+}{\varepsilon^-}. \end{equation}
Thus, the two fields’ increments have the same scaling with ![]() $\lambda$ (the same
$\lambda$ (the same ![]() $k_{\perp }$ spectra) and the ratio of their energies is
$k_{\perp }$ spectra) and the ratio of their energies is ![]() $\sim (\varepsilon ^+/\varepsilon ^-)^2$, in agreement with (9.1). This is the conclusion at which Lithwick et al. (Reference Lithwick, Goldreich and Sridhar2007, henceforth LGS07) arrived – they considered unaligned GS95-style turbulence (
$\sim (\varepsilon ^+/\varepsilon ^-)^2$, in agreement with (9.1). This is the conclusion at which Lithwick et al. (Reference Lithwick, Goldreich and Sridhar2007, henceforth LGS07) arrived – they considered unaligned GS95-style turbulence (![]() $\sin \theta \sim 1$), but that does not affect (9.3) (note that this result already appeared in (5.4)).
$\sin \theta \sim 1$), but that does not affect (9.3) (note that this result already appeared in (5.4)).
Things are, however, not as straightforward as they might appear. LGS07 point out that it is, in fact, counterintuitive that the weaker ![]() $\delta Z_\lambda ^-$ perturbation, which is distorted by
$\delta Z_\lambda ^-$ perturbation, which is distorted by ![]() $\delta Z_\lambda ^+$ on a shorter time scale
$\delta Z_\lambda ^+$ on a shorter time scale ![]() $\tau _{\mathrm {nl}}^-$, can nevertheless coherently distort
$\tau _{\mathrm {nl}}^-$, can nevertheless coherently distort ![]() $\delta Z_\lambda ^+$ for a longer time
$\delta Z_\lambda ^+$ for a longer time ![]() $\tau _{\mathrm {nl}}^+$. Their solution to this is to argue that, while the weaker field is strongly distorted in space by the stronger one, it remains correlated in time for as long as the stronger field does (coherence time of the long-correlated advector inherited by the advectee). In other words, during its (long) correlation time
$\tau _{\mathrm {nl}}^+$. Their solution to this is to argue that, while the weaker field is strongly distorted in space by the stronger one, it remains correlated in time for as long as the stronger field does (coherence time of the long-correlated advector inherited by the advectee). In other words, during its (long) correlation time ![]() $\tau _{\mathrm {nl}}^+$, the stronger field (in its reference frame travelling at
$\tau _{\mathrm {nl}}^+$, the stronger field (in its reference frame travelling at ![]() $v_{\mathrm {A}}$) sees a weak field that has been rendered multiscale by the spatial variation of the stronger field, but remains approximately constant for a time
$v_{\mathrm {A}}$) sees a weak field that has been rendered multiscale by the spatial variation of the stronger field, but remains approximately constant for a time ![]() $\tau _{\mathrm {nl}}^+$ and so can keep distorting the stronger field in a time-coherent way.
$\tau _{\mathrm {nl}}^+$ and so can keep distorting the stronger field in a time-coherent way.
This argument can only work, it seems, if the long-term coherence of the weaker field is not upset by the way in which it is forced, so one must assume that it is forced at the outer scale with the same (long!) correlation time as the cascade time of the stronger field, in the Alfvénic frame of the latter. Chandran & Perez (Reference Chandran and Perez2019) dub this the ‘coherence assumption’. While hard to justify in general,Footnote 44 it is great for them as, in their model, the weaker field is generated by the reflection of the stronger one as the latter propagates outwards in an expanding solar wind, and so one should indeed expect the two fields to be tightly correlated at the outer scale. Their endorsement of LGS07 – perhaps with an amendment that ![]() $\theta _\lambda$ should have some scaling with
$\theta _\lambda$ should have some scaling with ![]() $\lambda$ determined by alignment/intermittency (§ 6.4.2) – isbacked up by their numerical results, where both fields’ spectra approach
$\lambda$ determined by alignment/intermittency (§ 6.4.2) – isbacked up by their numerical results, where both fields’ spectra approach ![]() $k_{\perp }^{-3/2}$ (and their alignment increases) with increasing heliocentric distance.
$k_{\perp }^{-3/2}$ (and their alignment increases) with increasing heliocentric distance.
9.4. Perez & Boldyrev (Reference Perez and Boldyrev2009)
Perez & Boldyrev (Reference Perez and Boldyrev2009) disagree with the entire approach leading to (9.3): they think that the two Elsasser fields should have two different alignment angles ![]() $\theta ^\pm _\lambda$, both small, and posit that those ought to be the angles that they make with the velocity field.Footnote 45 Why that should be the case they do not explain, but if one takes their word for it, then (as is obvious from the geometry in figure 38)
$\theta ^\pm _\lambda$, both small, and posit that those ought to be the angles that they make with the velocity field.Footnote 45 Why that should be the case they do not explain, but if one takes their word for it, then (as is obvious from the geometry in figure 38)
 \begin{equation} \delta Z^+_\lambda\sin\theta^+_\lambda \sim \delta Z^-_\lambda\sin\theta^-_\lambda \quad\Rightarrow\quad \tau_{\mathrm{nl}}^+ \sim \tau_{\mathrm{nl}}^- \sim \frac{\lambda}{\delta Z^\pm_\lambda\sin\theta^\pm_\lambda} \quad\Rightarrow\quad \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} \sim \sqrt{\frac{\varepsilon^+}{\varepsilon^-}}. \end{equation}
\begin{equation} \delta Z^+_\lambda\sin\theta^+_\lambda \sim \delta Z^-_\lambda\sin\theta^-_\lambda \quad\Rightarrow\quad \tau_{\mathrm{nl}}^+ \sim \tau_{\mathrm{nl}}^- \sim \frac{\lambda}{\delta Z^\pm_\lambda\sin\theta^\pm_\lambda} \quad\Rightarrow\quad \frac{\delta Z^+_\lambda}{\delta Z^-_\lambda} \sim \sqrt{\frac{\varepsilon^+}{\varepsilon^-}}. \end{equation}
The last result follows from the first relation in (9.3) with ![]() $\tau _{\mathrm {nl}}^+\sim \tau _{\mathrm {nl}}^-$. The equality of cascade times also conveniently spares them having to deal with the issue, discussed above, of long-time correlatedness, or otherwise, of the weaker field (or with
$\tau _{\mathrm {nl}}^+\sim \tau _{\mathrm {nl}}^-$. The equality of cascade times also conveniently spares them having to deal with the issue, discussed above, of long-time correlatedness, or otherwise, of the weaker field (or with ![]() $l_{\parallel }^+\neq l _{\parallel }^-$; see § 9.5).
$l_{\parallel }^+\neq l _{\parallel }^-$; see § 9.5).
Perez & Boldyrev (Reference Perez and Boldyrev2009, Reference Perez and Boldyrev2010a,Reference Perez and Boldyrevb) are not forthcoming with any detailed tests of this scheme (viz., either of the details of alignment or of the energy-ratio scaling), while Beresnyak & Lazarian (Reference Beresnyak and Lazarian2010) present numerical results that contradict very strongly the expectation of the energy ratio scaling as ![]() $\varepsilon ^+/\varepsilon ^-$ (as implied by (9.4)) and possibly support
$\varepsilon ^+/\varepsilon ^-$ (as implied by (9.4)) and possibly support ![]() $(\varepsilon ^+/\varepsilon ^-)^2$ (i.e., (9.3)).Footnote 46 Perez & Boldyrev (Reference Perez and Boldyrev2010b) reply that (9.4) should only be expected to hold for local fluctuating values of the amplitudes and of
$(\varepsilon ^+/\varepsilon ^-)^2$ (i.e., (9.3)).Footnote 46 Perez & Boldyrev (Reference Perez and Boldyrev2010b) reply that (9.4) should only be expected to hold for local fluctuating values of the amplitudes and of ![]() $\varepsilon ^\pm$ and not for their box averages. It is not impossible that this could make a difference for cases of weak imbalance (
$\varepsilon ^\pm$ and not for their box averages. It is not impossible that this could make a difference for cases of weak imbalance (![]() $\varepsilon ^+/\varepsilon ^-\sim 1$), with local fluctuations of energy fluxes superseding the overall imbalance, although it seems to me that if it does, we are basically dealing with balanced turbulence anyway: I do not see any fundamental physical difference between
$\varepsilon ^+/\varepsilon ^-\sim 1$), with local fluctuations of energy fluxes superseding the overall imbalance, although it seems to me that if it does, we are basically dealing with balanced turbulence anyway: I do not see any fundamental physical difference between ![]() $\varepsilon ^+=\varepsilon ^-$ and
$\varepsilon ^+=\varepsilon ^-$ and ![]() $\varepsilon ^+\sim \varepsilon ^-$ on the level of ‘twiddle’ arguments by which everything is done in these theories. At strong imbalance, (9.3) seems to work better (Beresnyak & Lazarian Reference Beresnyak and Lazarian2009b, Reference Beresnyak and Lazarian2010) for the overall energy ratio, but not for spectra, which do not have the same slope (figure 18a). Perez & Boldyrev (Reference Perez and Boldyrev2010a,Reference Perez and Boldyrevb) argue that such cases in fact cannot be properly resolved, the limiting factor being the weaker field providing too slow a nonlinearity to compete with dissipation and produce a healthy inertial range. If so, the interesting case is inaccessible and the accessible case is uninteresting, we know nothing.
$\varepsilon ^+\sim \varepsilon ^-$ on the level of ‘twiddle’ arguments by which everything is done in these theories. At strong imbalance, (9.3) seems to work better (Beresnyak & Lazarian Reference Beresnyak and Lazarian2009b, Reference Beresnyak and Lazarian2010) for the overall energy ratio, but not for spectra, which do not have the same slope (figure 18a). Perez & Boldyrev (Reference Perez and Boldyrev2010a,Reference Perez and Boldyrevb) argue that such cases in fact cannot be properly resolved, the limiting factor being the weaker field providing too slow a nonlinearity to compete with dissipation and produce a healthy inertial range. If so, the interesting case is inaccessible and the accessible case is uninteresting, we know nothing.
9.5. Parallel scales and two flavours of CB
By the CB conjecture (§ 5.1), the parallel coherence lengths of the two fields are, in the ‘naïve’ theory leading to (9.3),
 \begin{equation} l_{{\parallel}}^\pm \sim v_{\mathrm{A}}\tau_{\mathrm{nl}}^\pm \quad\Rightarrow\quad \frac{l_{{\parallel}}^+}{l_{{\parallel}}^-} \sim \frac{\varepsilon^+}{\varepsilon^-} > 1, \end{equation}
\begin{equation} l_{{\parallel}}^\pm \sim v_{\mathrm{A}}\tau_{\mathrm{nl}}^\pm \quad\Rightarrow\quad \frac{l_{{\parallel}}^+}{l_{{\parallel}}^-} \sim \frac{\varepsilon^+}{\varepsilon^-} > 1, \end{equation}
whereas in the Perez & Boldyrev (Reference Perez and Boldyrev2009) theory (9.4), the equality of cascade times implies ![]() $l_{\parallel }^+\sim l _{\parallel }^-$, end of story. LGS07 argue that, in fact, also (9.5) should be replaced by
$l_{\parallel }^+\sim l _{\parallel }^-$, end of story. LGS07 argue that, in fact, also (9.5) should be replaced by
because ![]() $\boldsymbol {Z}_{\perp }^+$ perturbations separated by distance
$\boldsymbol {Z}_{\perp }^+$ perturbations separated by distance ![]() $l_{\parallel }^-$ in the parallel direction are advected by completely spatially decorrelated
$l_{\parallel }^-$ in the parallel direction are advected by completely spatially decorrelated ![]() $\boldsymbol {Z}_{\perp }^-$ perturbations, which would then imprint their parallel coherence length on their stronger cousins (the parallel coherence length of the short-correlated advector imprinted on the advectee).
$\boldsymbol {Z}_{\perp }^-$ perturbations, which would then imprint their parallel coherence length on their stronger cousins (the parallel coherence length of the short-correlated advector imprinted on the advectee).
Furthermore, if one accepts the LGS07 argument that the correlation time of the ![]() $\boldsymbol {Z}_{\perp }^-$ field is
$\boldsymbol {Z}_{\perp }^-$ field is ![]() $\tau _{\mathrm {nl}}^+$, not
$\tau _{\mathrm {nl}}^+$, not ![]() $\tau _{\mathrm {nl}}^-$ (see § 9.3), then
$\tau _{\mathrm {nl}}^-$ (see § 9.3), then ![]() $l_{\parallel }^-\sim v _{\mathrm {A}}\tau _{\mathrm {nl}}^-$ must be justified not by temporal (causal) decorrelation but by the weaker field being spatially distorted beyond recognition on the scale
$l_{\parallel }^-\sim v _{\mathrm {A}}\tau _{\mathrm {nl}}^-$ must be justified not by temporal (causal) decorrelation but by the weaker field being spatially distorted beyond recognition on the scale ![]() $l_{\parallel }^-$, even if remaining temporally coherent. This is more or less what Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008) call ‘propagation CB’ (the other CB being ‘causality CB’). They note that the typical uncertainty in the parallel gradient of any fluctuating field at scale
$l_{\parallel }^-$, even if remaining temporally coherent. This is more or less what Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008) call ‘propagation CB’ (the other CB being ‘causality CB’). They note that the typical uncertainty in the parallel gradient of any fluctuating field at scale ![]() $\lambda$ is
$\lambda$ is
In balanced turbulence, ![]() $\delta k _{\parallel }^{-1}\sim v _{\mathrm {A}}\tau _{\mathrm {nl}} \sim l_{\parallel }$ (cf. (6.32)), so this is just a consistency check. In imbalanced turbulence,
$\delta k _{\parallel }^{-1}\sim v _{\mathrm {A}}\tau _{\mathrm {nl}} \sim l_{\parallel }$ (cf. (6.32)), so this is just a consistency check. In imbalanced turbulence,
where ![]() $\tau _{\mathrm {nl}}^-$, given by (9.2), is the spatial-distortion time of
$\tau _{\mathrm {nl}}^-$, given by (9.2), is the spatial-distortion time of ![]() $\delta Z^-_\lambda$, not necessarily its correlation time. The parallel scale of any field will be the shorter of
$\delta Z^-_\lambda$, not necessarily its correlation time. The parallel scale of any field will be the shorter of ![]() $\delta k _{\parallel }^{-1}$ and whatever is implied by the causality CB. In the LGS07 theory, the latter is
$\delta k _{\parallel }^{-1}$ and whatever is implied by the causality CB. In the LGS07 theory, the latter is ![]() $v_{\mathrm {A}}\tau _{\mathrm {nl}}^+$ for both fields. Since
$v_{\mathrm {A}}\tau _{\mathrm {nl}}^+$ for both fields. Since ![]() $\tau _{\mathrm {nl}}^+\gg \tau _{\mathrm {nl}}^-$, we must set
$\tau _{\mathrm {nl}}^+\gg \tau _{\mathrm {nl}}^-$, we must set ![]() $l_{\parallel }^+ \sim l_{\parallel }^- \sim \delta k _{\parallel }^{-1}$, which is the same as (9.6).
$l_{\parallel }^+ \sim l_{\parallel }^- \sim \delta k _{\parallel }^{-1}$, which is the same as (9.6).
Thus, we end up with both Elsasser fields having ![]() $\tau _{\mathrm {A}} \sim l_{\parallel }^-/v_{\mathrm {A}}$ that is smaller than their correlation time
$\tau _{\mathrm {A}} \sim l_{\parallel }^-/v_{\mathrm {A}}$ that is smaller than their correlation time ![]() $\tau _{\mathrm {nl}}^+$ (even though the weaker field has a shorter spatial distortion time
$\tau _{\mathrm {nl}}^+$ (even though the weaker field has a shorter spatial distortion time ![]() $\tau _{\mathrm {nl}}^-\sim \tau _{\mathrm {A}}$), but their cascades are nevertheless strong. Whatever you think of the merits of the above arguments, neither (9.5) nor (9.6) appear to be consistent with any of the cases reported by Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b), weakly or strongly imbalanced, which all have
$\tau _{\mathrm {nl}}^-\sim \tau _{\mathrm {A}}$), but their cascades are nevertheless strong. Whatever you think of the merits of the above arguments, neither (9.5) nor (9.6) appear to be consistent with any of the cases reported by Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b), weakly or strongly imbalanced, which all have ![]() $l_{\parallel }^+< l_{\parallel }^-$ (see, e.g., figure 18b). No other numerical evidence on the parallel scales in imbalanced turbulence is, as far as I know, available in print.
$l_{\parallel }^+< l_{\parallel }^-$ (see, e.g., figure 18b). No other numerical evidence on the parallel scales in imbalanced turbulence is, as far as I know, available in print.
9.6. Towards a new theory of imbalanced MHD turbulence
The Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008) argument was, in fact, more complicated than presented in § 9.5, because they did not agree with LGS07 about the long correlation time of the weaker field, assumed the stronger field to be weakly, rather than strongly, turbulent, and were keen to accommodate ![]() $l_{\parallel }^+ < l_{\parallel }^-$. Their key innovation was to allow interactions to be non-local. I will not review their theory here, because it depends on a number of ad hoc choices that I do not know how to justify, and does not, as far as I can tell, lead to a fully satisfactory set of predictions, but I would like to seize on their idea of non-locality of interactions, although in a way that is somewhat different from theirs. The resulting scheme captures most of the properties of imbalanced turbulence observed in numerical simulations (§ 9.2) and reduces to the already established theory for the balanced case when
$l_{\parallel }^+ < l_{\parallel }^-$. Their key innovation was to allow interactions to be non-local. I will not review their theory here, because it depends on a number of ad hoc choices that I do not know how to justify, and does not, as far as I can tell, lead to a fully satisfactory set of predictions, but I would like to seize on their idea of non-locality of interactions, although in a way that is somewhat different from theirs. The resulting scheme captures most of the properties of imbalanced turbulence observed in numerical simulations (§ 9.2) and reduces to the already established theory for the balanced case when ![]() $\varepsilon ^+/\varepsilon ^-\sim 1$, so perhaps it deserves at least some benefit of the doubt.
$\varepsilon ^+/\varepsilon ^-\sim 1$, so perhaps it deserves at least some benefit of the doubt.
9.6.1. Two semi-local cascades
Let me assume a priori that, as suggested by numerics (Beresnyak & Lazarian Reference Beresnyak and Lazarian2009b; Beresnyak Reference Beresnyak2019), ![]() $l_{\parallel }^+\lll _{\parallel }^-$ in the inertial range, viz.,
$l_{\parallel }^+\lll _{\parallel }^-$ in the inertial range, viz.,
 \begin{equation} \frac{l_{{\parallel}\lambda}^+}{l_{{\parallel}\lambda}^-} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^\alpha, \end{equation}
\begin{equation} \frac{l_{{\parallel}\lambda}^+}{l_{{\parallel}\lambda}^-} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^\alpha, \end{equation}
where ![]() $\alpha > 0$ and
$\alpha > 0$ and ![]() $L_{\perp }$ is the perpendicular outer scale (so the two Elsasser fields are assumed to have the same parallel correlation length,
$L_{\perp }$ is the perpendicular outer scale (so the two Elsasser fields are assumed to have the same parallel correlation length, ![]() $L_{\parallel }$, at the outer scale – e.g., by being forced that way, as was done by Beresnyak Reference Beresnyak2019).
$L_{\parallel }$, at the outer scale – e.g., by being forced that way, as was done by Beresnyak Reference Beresnyak2019).
This implies that, at the same ![]() $\lambda$, the stronger field
$\lambda$, the stronger field ![]() $\delta Z_\lambda ^+$ oscillates much faster than the weaker field
$\delta Z_\lambda ^+$ oscillates much faster than the weaker field ![]() $\delta Z_\lambda ^-$. I shall assume therefore that the interaction between the two fields local to the scale
$\delta Z_\lambda ^-$. I shall assume therefore that the interaction between the two fields local to the scale ![]() $\lambda$ is not efficient: even though
$\lambda$ is not efficient: even though ![]() $\delta Z_\lambda ^-$ is buffeted quite vigorously by the stronger field
$\delta Z_\lambda ^-$ is buffeted quite vigorously by the stronger field ![]() $\delta Z_\lambda ^+$, most of this cancels out. Rather than attempting to pick up a contribution arising for the resulting weak interaction, let me instead posit that the dominant, strong nonlinear distortion of
$\delta Z_\lambda ^+$, most of this cancels out. Rather than attempting to pick up a contribution arising for the resulting weak interaction, let me instead posit that the dominant, strong nonlinear distortion of ![]() $\delta Z_\lambda ^-$ will be due to the stronger field
$\delta Z_\lambda ^-$ will be due to the stronger field ![]() $\delta Z_{\lambda '}^+$ at a scale
$\delta Z_{\lambda '}^+$ at a scale ![]() $\lambda ' > \lambda$ such that
$\lambda ' > \lambda$ such that
In other words, the interaction is non-local in ![]() $\lambda$ but local in
$\lambda$ but local in ![]() $l_{\parallel }$.Footnote 47 The constancy of the flux of the weaker field then requires
$l_{\parallel }$.Footnote 47 The constancy of the flux of the weaker field then requires
 \begin{equation} \frac{\left(\delta Z_\lambda^-\right)^2\delta Z_{\lambda'}^+}{\xi_{\lambda'}} \sim \varepsilon^-, \end{equation}
\begin{equation} \frac{\left(\delta Z_\lambda^-\right)^2\delta Z_{\lambda'}^+}{\xi_{\lambda'}} \sim \varepsilon^-, \end{equation}
where ![]() $\xi _{\lambda '}$ has been introduced to account for a possible depletion of the nonlinearity due to alignment:
$\xi _{\lambda '}$ has been introduced to account for a possible depletion of the nonlinearity due to alignment:
In the absence of alignment, ![]() $\beta = 1$. For aligned, balanced, locally cascading (
$\beta = 1$. For aligned, balanced, locally cascading (![]() $\lambda ' \sim \lambda$) turbulence,
$\lambda ' \sim \lambda$) turbulence, ![]() $\beta = 3/4$ (see (6.31)). By the usual CB argument, the parallel coherence scale of the weaker field is
$\beta = 3/4$ (see (6.31)). By the usual CB argument, the parallel coherence scale of the weaker field is
Note that, in the terminology of § 9.5, this is both the causality CB and the propagation CB, because ![]() $\delta k _{\parallel }$ for
$\delta k _{\parallel }$ for ![]() $\delta Z_\lambda ^-$ is determined by the propagation of the latter along the ‘local mean field’
$\delta Z_\lambda ^-$ is determined by the propagation of the latter along the ‘local mean field’ ![]() $\delta b_{\lambda '}$ (see (9.7)).
$\delta b_{\lambda '}$ (see (9.7)).
Now consider the cascading of the stronger field by the weaker one. Since ![]() $l_{\parallel \lambda }^+ \ll l_{\parallel \lambda }^-$, the
$l_{\parallel \lambda }^+ \ll l_{\parallel \lambda }^-$, the ![]() $\delta Z_\lambda ^-$ fluctuations are, from the point of view of the
$\delta Z_\lambda ^-$ fluctuations are, from the point of view of the ![]() $\delta Z_\lambda ^+$ ones, slow and quasi-2D, and so the weaker field can cascade the strong one locally, in the same way as it does in any of the theories described above:
$\delta Z_\lambda ^+$ ones, slow and quasi-2D, and so the weaker field can cascade the strong one locally, in the same way as it does in any of the theories described above:
Causality CB would imply ![]() $l_{\parallel \lambda }^+\sim v_{\mathrm {A}}\xi _\lambda /\delta Z_\lambda ^-$, but that is long compared to
$l_{\parallel \lambda }^+\sim v_{\mathrm {A}}\xi _\lambda /\delta Z_\lambda ^-$, but that is long compared to ![]() $\delta k _{\parallel }^{-1}$ given by (9.7), so I shall use propagation CB instead, just like LGS07 and Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008) did:
$\delta k _{\parallel }^{-1}$ given by (9.7), so I shall use propagation CB instead, just like LGS07 and Beresnyak & Lazarian (Reference Beresnyak and Lazarian2008) did:
Reassuringly, this choice immediately clicks into consistency with the requirement of parallel locality (9.10) if ![]() $l_{\parallel \lambda }^-$ is given by (9.13).
$l_{\parallel \lambda }^-$ is given by (9.13).
There are two nuances here. First, in order for the ![]() $\delta Z_\lambda ^-$ field to be able to distort
$\delta Z_\lambda ^-$ field to be able to distort ![]() $\delta Z_\lambda ^+$ according to (9.14), it needs to remain coherent for a time
$\delta Z_\lambda ^+$ according to (9.14), it needs to remain coherent for a time ![]() $\sim \xi _\lambda /\delta Z_\lambda ^-$. To make it do so, let me invoke the LGS07 argument already rehearsed in § 9.3: according to (9.11),
$\sim \xi _\lambda /\delta Z_\lambda ^-$. To make it do so, let me invoke the LGS07 argument already rehearsed in § 9.3: according to (9.11), ![]() $\delta Z_\lambda ^-$ stays coherent as long as
$\delta Z_\lambda ^-$ stays coherent as long as ![]() $\delta Z_{\lambda '}^+$ does, which, according to (9.14) with
$\delta Z_{\lambda '}^+$ does, which, according to (9.14) with ![]() $\lambda =\lambda '$, is
$\lambda =\lambda '$, is ![]() $\xi _{\lambda '}/\delta Z_{\lambda '}^-$ – long enough!
$\xi _{\lambda '}/\delta Z_{\lambda '}^-$ – long enough!
Secondly, in (9.14), I used the same fluctuation-direction scale ![]() $\xi _\lambda$ as in (9.11), except at
$\xi _\lambda$ as in (9.11), except at ![]() $\lambda$, rather than at
$\lambda$, rather than at ![]() $\lambda '$. This may be a somewhat simplistic treatment of alignment in local vs. non-local interactions, but I do not know how to do better, and the scalings that I get this way will have all the right properties. A reader who finds this unconvincing may assume
$\lambda '$. This may be a somewhat simplistic treatment of alignment in local vs. non-local interactions, but I do not know how to do better, and the scalings that I get this way will have all the right properties. A reader who finds this unconvincing may assume ![]() $\xi _\lambda \sim \lambda$ and treat what follows as a GS95-style theory that ignores alignment altogether.
$\xi _\lambda \sim \lambda$ and treat what follows as a GS95-style theory that ignores alignment altogether.
To summarise, I am considering here an imbalanced turbulence that consists of two ‘semi-local’ cascades: that of the stronger field, local in ![]() $\lambda$ but not in
$\lambda$ but not in ![]() $l_{\parallel }$, and that of the weaker one, local in
$l_{\parallel }$, and that of the weaker one, local in ![]() $l_{\parallel }$ but not in
$l_{\parallel }$ but not in ![]() $\lambda$ (figure 20).
$\lambda$ (figure 20).

Figure 20. Cartoon of the spectra of imbalanced turbulence. The interactions are shown by arrows: red (advection of the weaker field by the stronger field, non-local in ![]() $\lambda$ but local in
$\lambda$ but local in ![]() $l_{\parallel }$) and blue (advection of the stronger field by the weaker field, local in
$l_{\parallel }$) and blue (advection of the stronger field by the weaker field, local in ![]() $\lambda$ but non-localin
$\lambda$ but non-localin ![]() $l_{\parallel }$). The inset shows the parallel scales
$l_{\parallel }$). The inset shows the parallel scales ![]() $l_{\parallel }^\pm$ vs. the perpendicular scale
$l_{\parallel }^\pm$ vs. the perpendicular scale ![]() $\lambda$.
$\lambda$.
9.6.2. Perpendicular spectra
In view of (9.13) and (9.15), (9.11) can be rewritten as follows:
 \begin{equation} \frac{\left(\delta Z_\lambda^-\right)^2\delta Z_\lambda^+}{\xi_\lambda} \sim \varepsilon^-\frac{\xi_{\lambda'}\delta Z_\lambda^+}{\xi_\lambda\delta Z_{\lambda'}^+} \sim \varepsilon^-\frac{l_{{\parallel}\lambda}^-}{l_{{\parallel}\lambda}^+} \sim \varepsilon^-\left(\frac{\lambda}{L_{{\perp}}}\right)^{-\alpha}, \end{equation}
\begin{equation} \frac{\left(\delta Z_\lambda^-\right)^2\delta Z_\lambda^+}{\xi_\lambda} \sim \varepsilon^-\frac{\xi_{\lambda'}\delta Z_\lambda^+}{\xi_\lambda\delta Z_{\lambda'}^+} \sim \varepsilon^-\frac{l_{{\parallel}\lambda}^-}{l_{{\parallel}\lambda}^+} \sim \varepsilon^-\left(\frac{\lambda}{L_{{\perp}}}\right)^{-\alpha}, \end{equation}the last step being a recapitulation of the assumption (9.9). Dividing (9.14) by (9.16), one gets
 \begin{equation} \frac{\delta Z_\lambda^+}{\delta Z_\lambda^-} \sim \frac{\varepsilon^+}{\varepsilon^-}\left(\frac{\lambda}{L_{{\perp}}}\right)^\alpha. \end{equation}
\begin{equation} \frac{\delta Z_\lambda^+}{\delta Z_\lambda^-} \sim \frac{\varepsilon^+}{\varepsilon^-}\left(\frac{\lambda}{L_{{\perp}}}\right)^\alpha. \end{equation}
Thus, the ratio of the energies at the outer scale (![]() $\lambda =L_{\perp }$) is
$\lambda =L_{\perp }$) is ![]() $(\varepsilon ^+/\varepsilon ^-)^2$, likely the correct scaling (see § 9.2, item (ii) and § 9.4), and the spectrum of the stronger field is steeper than that of the weaker field, also in agreement with numerics (§ 9.2, item (i)).
$(\varepsilon ^+/\varepsilon ^-)^2$, likely the correct scaling (see § 9.2, item (ii) and § 9.4), and the spectrum of the stronger field is steeper than that of the weaker field, also in agreement with numerics (§ 9.2, item (i)).
Now, by using (9.14), (9.17) and the alignment assumption (9.12), it becomes possible to determine the scalings of both fields:
 \begin{equation} \delta Z_\lambda^+ \sim \left[\frac{(\varepsilon^+)^2}{\varepsilon^-}L_{{\perp}}\right]^{1/3} \left(\frac{\lambda}{L_{{\perp}}}\right)^{(\beta + \alpha)/3},\qquad \delta Z_\lambda^- \sim \left[\frac{(\varepsilon^-)^2}{\varepsilon^+}L_{{\perp}}\right]^{1/3} \left(\frac{\lambda}{L_{{\perp}}}\right)^{(\beta - 2\alpha)/3}. \end{equation}
\begin{equation} \delta Z_\lambda^+ \sim \left[\frac{(\varepsilon^+)^2}{\varepsilon^-}L_{{\perp}}\right]^{1/3} \left(\frac{\lambda}{L_{{\perp}}}\right)^{(\beta + \alpha)/3},\qquad \delta Z_\lambda^- \sim \left[\frac{(\varepsilon^-)^2}{\varepsilon^+}L_{{\perp}}\right]^{1/3} \left(\frac{\lambda}{L_{{\perp}}}\right)^{(\beta - 2\alpha)/3}. \end{equation}Comparing the first of these with (9.11), one can also work out how non-local the interactions are:
 \begin{equation} \frac{\lambda}{\lambda'} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{3\alpha/(2\beta-\alpha)}. \end{equation}
\begin{equation} \frac{\lambda}{\lambda'} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{3\alpha/(2\beta-\alpha)}. \end{equation} With ![]() $\alpha =0$ and
$\alpha =0$ and ![]() $\beta =1$ in (9.18), we are back to GS95 (§ 5.3), whereas with
$\beta =1$ in (9.18), we are back to GS95 (§ 5.3), whereas with ![]() $\alpha = 0$ and
$\alpha = 0$ and ![]() $\beta =3/4$, we recover the aligned theory of § 6.4.
$\beta =3/4$, we recover the aligned theory of § 6.4.
9.6.3. Parallel spectra
Now, from (9.15), (9.12), (9.18), and (9.9), the parallel scales are
 \begin{equation} \frac{l_{{\parallel}\lambda}^+}{L_{{\parallel}}} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{(2\beta-\alpha)/3},\qquad \frac{l_{{\parallel}\lambda}^-}{L_{{\parallel}}} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{2(\beta-2\alpha)/3}, \end{equation}
\begin{equation} \frac{l_{{\parallel}\lambda}^+}{L_{{\parallel}}} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{(2\beta-\alpha)/3},\qquad \frac{l_{{\parallel}\lambda}^-}{L_{{\parallel}}} \sim \left(\frac{\lambda}{L_{{\perp}}}\right)^{2(\beta-2\alpha)/3}, \end{equation}where the parallel outer scale is (cf. (6.16))
 \begin{equation} L_{{\parallel}} = v_{\mathrm{A}} L_{{\perp}}^{2/3}\left[\frac{(\varepsilon^+)^2}{\varepsilon^-}\right]^{{-}1/3}. \end{equation}
\begin{equation} L_{{\parallel}} = v_{\mathrm{A}} L_{{\perp}}^{2/3}\left[\frac{(\varepsilon^+)^2}{\varepsilon^-}\right]^{{-}1/3}. \end{equation}Combining (9.20) with (9.18) gives us the parallel scalings of the field increments:
 \begin{equation} \delta Z_{l_{{\parallel}}}^+ \sim \left[\frac{(\varepsilon^+)^2L_{{\parallel}}}{\varepsilon^-v_{\mathrm{A}}}\right]^{1/2} \left(\frac{l_{{\parallel}}}{L_{{\parallel}}}\right)^{(\beta + \alpha)/(2\beta - \alpha)},\qquad \delta Z_{l_{{\parallel}}}^- \sim \left(\frac{\varepsilon^-l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}. \end{equation}
\begin{equation} \delta Z_{l_{{\parallel}}}^+ \sim \left[\frac{(\varepsilon^+)^2L_{{\parallel}}}{\varepsilon^-v_{\mathrm{A}}}\right]^{1/2} \left(\frac{l_{{\parallel}}}{L_{{\parallel}}}\right)^{(\beta + \alpha)/(2\beta - \alpha)},\qquad \delta Z_{l_{{\parallel}}}^- \sim \left(\frac{\varepsilon^-l_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}. \end{equation}
Whereas the stronger field's scaling is (for small ![]() $\alpha$, slightly) steeper than
$\alpha$, slightly) steeper than ![]() $l_{\parallel }^{1/2}$, the weaker one's is exactly that, corresponding to a
$l_{\parallel }^{1/2}$, the weaker one's is exactly that, corresponding to a ![]() $k_{\parallel }^{-2}$ spectrum, as is indeed seen in numerical simulations (§ 9.2, item (iv) and figure 19). This makes sense because the weaker field was assumed to have a local parallel cascade with the usual CB conjecture, so the standard arguments for its parallel spectrum given in § 5.2 remain valid.
$k_{\parallel }^{-2}$ spectrum, as is indeed seen in numerical simulations (§ 9.2, item (iv) and figure 19). This makes sense because the weaker field was assumed to have a local parallel cascade with the usual CB conjecture, so the standard arguments for its parallel spectrum given in § 5.2 remain valid.
9.6.4. Pinning
It turns out that it is possible to determine ![]() $\alpha$ by considering what happens at the dissipation scale(s). The dissipation cutoffs
$\alpha$ by considering what happens at the dissipation scale(s). The dissipation cutoffs ![]() $\lambda _{\eta }^\pm$ for the two Elsasser fields can be worked out by balancing their fluxes with their dissipation rates:
$\lambda _{\eta }^\pm$ for the two Elsasser fields can be worked out by balancing their fluxes with their dissipation rates:
 \begin{equation} \varepsilon^\pm \sim \frac{\nu + \eta}{\left(\lambda_{\eta}^\pm\right)^2}\left(\delta Z^\pm_{\lambda_{\eta}^\pm}\right)^2. \end{equation}
\begin{equation} \varepsilon^\pm \sim \frac{\nu + \eta}{\left(\lambda_{\eta}^\pm\right)^2}\left(\delta Z^\pm_{\lambda_{\eta}^\pm}\right)^2. \end{equation}
Using (9.18) to work out the field amplitudes at ![]() $\lambda _{\eta }^\pm$, one gets
$\lambda _{\eta }^\pm$, one gets
 \begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}} \sim \left(\frac{\varepsilon^-}{\varepsilon^+}\widetilde{\mathrm{Re}}\right)^{{-}1.5/(3-\beta-\alpha)}, \qquad \frac{\lambda_{\eta}^-}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta+2\alpha)}, \qquad \widetilde{\mathrm{Re}} = \frac{\delta Z^+_{L_{{\perp}}}L_{{\perp}}}{\nu + \eta}, \end{equation}
\begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}} \sim \left(\frac{\varepsilon^-}{\varepsilon^+}\widetilde{\mathrm{Re}}\right)^{{-}1.5/(3-\beta-\alpha)}, \qquad \frac{\lambda_{\eta}^-}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta+2\alpha)}, \qquad \widetilde{\mathrm{Re}} = \frac{\delta Z^+_{L_{{\perp}}}L_{{\perp}}}{\nu + \eta}, \end{equation}
where, as before, ![]() $\widetilde {\mathrm {Re}}$ is the smaller of
$\widetilde {\mathrm {Re}}$ is the smaller of ![]() $\mathrm {Re}$ and
$\mathrm {Re}$ and ![]() $\mathrm {Rm}$. There are two possibilities: either
$\mathrm {Rm}$. There are two possibilities: either ![]() $\lambda _{\eta }^+ < \lambda _{\eta }^-$ or
$\lambda _{\eta }^+ < \lambda _{\eta }^-$ or ![]() $\lambda _{\eta }^+ > \lambda _{\eta }^-$. The first of these is, in fact, impossible: if the weaker field is cut off at
$\lambda _{\eta }^+ > \lambda _{\eta }^-$. The first of these is, in fact, impossible: if the weaker field is cut off at ![]() $\lambda _{\eta }^-$, there is nothing to cascade the stronger field at
$\lambda _{\eta }^-$, there is nothing to cascade the stronger field at ![]() $\lambda < \lambda _{\eta }^-$ (locally in
$\lambda < \lambda _{\eta }^-$ (locally in ![]() $\lambda$, as I assumed in § 9.6.1). The second possibility is
$\lambda$, as I assumed in § 9.6.1). The second possibility is ![]() $\lambda _{\eta }^+ > \lambda _{\eta }^-$. Since the weaker field is cascaded by the stronger one non-locally, a self-consistent situation would be one in which
$\lambda _{\eta }^+ > \lambda _{\eta }^-$. Since the weaker field is cascaded by the stronger one non-locally, a self-consistent situation would be one in which ![]() $\lambda _{\eta }^+$ were the scale
$\lambda _{\eta }^+$ were the scale ![]() $\lambda '$ corresponding to
$\lambda '$ corresponding to ![]() $\lambda =\lambda _{\eta }^-$. Using (9.19), we must therefore ‘pin’ the dissipation scales together in the following way:
$\lambda =\lambda _{\eta }^-$. Using (9.19), we must therefore ‘pin’ the dissipation scales together in the following way:
 \begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}}\sim\left(\frac{\lambda_{\eta}^-}{L_{{\perp}}}\right)^{2(\beta-2\alpha)/(2\beta-\alpha)} \quad\Rightarrow\quad 1 -\frac{2(\beta-2\alpha)(3-\beta-\alpha)}{(2\beta-\alpha)(3-\beta+2\alpha)} = \frac{\ln(\varepsilon^+{/}\varepsilon^-)}{\ln\widetilde{\mathrm{Re}}}. \end{equation}
\begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}}\sim\left(\frac{\lambda_{\eta}^-}{L_{{\perp}}}\right)^{2(\beta-2\alpha)/(2\beta-\alpha)} \quad\Rightarrow\quad 1 -\frac{2(\beta-2\alpha)(3-\beta-\alpha)}{(2\beta-\alpha)(3-\beta+2\alpha)} = \frac{\ln(\varepsilon^+{/}\varepsilon^-)}{\ln\widetilde{\mathrm{Re}}}. \end{equation}
Assuming ![]() $\alpha \ll 1$, we get
$\alpha \ll 1$, we get
Thus, indeed, ![]() $\alpha \to 0$ as
$\alpha \to 0$ as ![]() $\widetilde {\mathrm {Re}}\to \infty$, but very slowly, with very large
$\widetilde {\mathrm {Re}}\to \infty$, but very slowly, with very large ![]() $\widetilde {\mathrm {Re}}$ needed to achieve a modicum of asymptoticity at larger imbalances. For the record, this implies, from (9.24),
$\widetilde {\mathrm {Re}}$ needed to achieve a modicum of asymptoticity at larger imbalances. For the record, this implies, from (9.24),
 \begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta)}\left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{0.5(9+\beta)/(9-\beta^2)}, \qquad \frac{\lambda_{\eta}^-}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta)}\left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{2\beta/(9-\beta^2)}. \end{equation}
\begin{equation} \frac{\lambda_{\eta}^+}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta)}\left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{0.5(9+\beta)/(9-\beta^2)}, \qquad \frac{\lambda_{\eta}^-}{L_{{\perp}}} \sim \widetilde{\mathrm{Re}}^{{-}1.5/(3-\beta)}\left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{2\beta/(9-\beta^2)}. \end{equation}
It actually does appear to be the case that ![]() $\lambda _{\eta }^+>\lambda _{\eta }^-$ in figure 18(a) (Beresnyak & Lazarian Reference Beresnyak and Lazarian2009b) and figure 35(c) (Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021) – perhaps the strongest evidence that we have of the non-locality of imbalanced cascades.
$\lambda _{\eta }^+>\lambda _{\eta }^-$ in figure 18(a) (Beresnyak & Lazarian Reference Beresnyak and Lazarian2009b) and figure 35(c) (Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021) – perhaps the strongest evidence that we have of the non-locality of imbalanced cascades.
While what I have proposed above is a kind of ‘pinning’, it is not the conventional ‘pinning’ that means equating the amplitudes of the two fields to each other at the dissipation scale – one of the tenets of the theory of weak imbalanced turbulence (§ 4.3). Indeed, (9.23) implies that the ratio of the Elsasser amplitudes at their respective dissipation scales is
 \begin{equation} \frac{\delta Z^+_{\lambda_{\eta}^+}}{\delta Z^-_{\lambda_{\eta}^-}} \sim \sqrt{\frac{\varepsilon^+}{\varepsilon^-}}\frac{\lambda_{\eta}^+}{\lambda_{\eta}^-} \sim \left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{(3+\beta/2)/(3+\beta)}, \end{equation}
\begin{equation} \frac{\delta Z^+_{\lambda_{\eta}^+}}{\delta Z^-_{\lambda_{\eta}^-}} \sim \sqrt{\frac{\varepsilon^+}{\varepsilon^-}}\frac{\lambda_{\eta}^+}{\lambda_{\eta}^-} \sim \left(\frac{\varepsilon^+}{\varepsilon^-}\right)^{(3+\beta/2)/(3+\beta)}, \end{equation}
where I have used (9.27), valid in the limit ![]() $\widetilde {\mathrm {Re}}\to \infty$. Notably, the amplitude ratio is independent of
$\widetilde {\mathrm {Re}}\to \infty$. Notably, the amplitude ratio is independent of ![]() $\widetilde {\mathrm {Re}}$ in this limit, but is not equal to
$\widetilde {\mathrm {Re}}$ in this limit, but is not equal to ![]() $(\varepsilon ^+/\varepsilon ^-)^{1/2}$, as one might have concluded from (9.23) for
$(\varepsilon ^+/\varepsilon ^-)^{1/2}$, as one might have concluded from (9.23) for ![]() $\lambda _{\eta }^+=\lambda _{\eta }^-$ (as Beresnyak & Lazarian Reference Beresnyak and Lazarian2008 did).
$\lambda _{\eta }^+=\lambda _{\eta }^-$ (as Beresnyak & Lazarian Reference Beresnyak and Lazarian2008 did).
Arguably, this is a rather attractive theory: asymptotically, the spectra are parallel, interactions are local, etc., but in any finite-width inertial range, there are finite-![]() $\widetilde {\mathrm {Re}}$ logarithmic corrections to scalings, locality, etc., accounting for all of the distinctive features of imbalanced turbulence seen in non-asymptotic simulations (§ 9.2).
$\widetilde {\mathrm {Re}}$ logarithmic corrections to scalings, locality, etc., accounting for all of the distinctive features of imbalanced turbulence seen in non-asymptotic simulations (§ 9.2).
9.6.5. Alignment, intermittency, reconnection
Like in balanced turbulence, alignment is likely related to intermittency in imbalanced turbulence as well. Since, for imbalanced turbulence, we are still litigating such basic things as spectra, there is not much we know about its intermittency – and I do not propose to engage with this topic here any more than I have done already with a few throw-away comments in § 9.1. The argument in § 6.4.2 that led to ![]() $\beta =3/4$ depended on assumptions about the most intense structures being sheets and on the ‘refined critical balance’ (Mallet et al. Reference Mallet, Schekochihin and Chandran2015, see figure 6). It seems a worthwhile project to check whether, and in what sense, these features survive in imbalanced turbulence.
$\beta =3/4$ depended on assumptions about the most intense structures being sheets and on the ‘refined critical balance’ (Mallet et al. Reference Mallet, Schekochihin and Chandran2015, see figure 6). It seems a worthwhile project to check whether, and in what sense, these features survive in imbalanced turbulence.
Since reconnection playing an important role at the small-scale end of the inertial range depended on alignment, the equivalent of § 7 for imbalanced turbulence must wait for a better understanding of alignment. If tearing disruption does occur at some scale in (strongly) imbalanced turbulence, the pinning scheme proposed in § 9.6.4 has to be redesigned. Incidentally, it also has to be redesigned (according to Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021, redesigned quite dramatically) for natural plasmas like the solar wind, where the cutoff of the RMHD inertial range is accomplished by kinetic effects rather than by Laplacian viscosity – but these matters are outside the scope of this review (see §§ 14.1 and 14.2).
10. Residual energy in MHD turbulence
10.1. Observational and numerical evidence
Going back to figure 16(a), we see that real MHD turbulence observed in the solar wind is distributed between cases with a local Elsasser imbalance (cross-helicity) and those with an Alfvénic one – the latter in favour of the magnetic field. Thus, the imbalanced cascades are only half of the story. According to the second relation in (B6), in imbalanced turbulence (![]() $|\sigma _{\mathrm {c}}|\approx 1$), it is a geometric inevitability that
$|\sigma _{\mathrm {c}}|\approx 1$), it is a geometric inevitability that ![]() $|\sigma _{\mathrm {r}}|\ll 1$, as illustrated by figure 16(a) and confirmed directly in the statistical study of solar-wind data by Bowen et al. (Reference Bowen, Mallet, Bonnell and Bale2018). In contrast, when the cross-helicity is not large (i.e., when
$|\sigma _{\mathrm {r}}|\ll 1$, as illustrated by figure 16(a) and confirmed directly in the statistical study of solar-wind data by Bowen et al. (Reference Bowen, Mallet, Bonnell and Bale2018). In contrast, when the cross-helicity is not large (i.e., when ![]() $\sigma _{\mathrm {c}}$ is not close to
$\sigma _{\mathrm {c}}$ is not close to ![]() $\pm 1$), there is flexibility for the perturbations to have finite residual energy: in the event,
$\pm 1$), there is flexibility for the perturbations to have finite residual energy: in the event, ![]() $\sigma _{\mathrm {r}}<0$. The definitive observational paper on this is Chen et al. (Reference Chen, Bale, Salem and Maruca2013), confirming negative
$\sigma _{\mathrm {r}}<0$. The definitive observational paper on this is Chen et al. (Reference Chen, Bale, Salem and Maruca2013), confirming negative ![]() $\sigma _{\mathrm {r}}$ over a large data set obtained in the solar wind. They also report that residual energy has a spectrum consistent with
$\sigma _{\mathrm {r}}$ over a large data set obtained in the solar wind. They also report that residual energy has a spectrum consistent with ![]() $k_{\perp }^{-2}$ or perhaps a little shallower, but certainly steeper than either the kinetic- or magnetic-energy spectra: the scalings of all three are reproduced in figure 21. This seems to be in agreement with earlier observational and numerical evidence (Müller & Grappin Reference Müller and Grappin2005; Boldyrev et al. Reference Boldyrev, Perez, Borovsky and Podesta2011, figure 22).
$k_{\perp }^{-2}$ or perhaps a little shallower, but certainly steeper than either the kinetic- or magnetic-energy spectra: the scalings of all three are reproduced in figure 21. This seems to be in agreement with earlier observational and numerical evidence (Müller & Grappin Reference Müller and Grappin2005; Boldyrev et al. Reference Boldyrev, Perez, Borovsky and Podesta2011, figure 22).

Figure 21. Spectra of magnetic (red), kinetic (blue), total (black) and residual (green) energies measured by Chen et al. (Reference Chen, Bale, Salem and Maruca2013) (figures taken from Chen Reference Chen2016): (a) typical spectra; (b) average spectral indices vs. normalised cross-helicity ![]() $\sigma _{\mathrm {c}}$ (defined in (B4)).
$\sigma _{\mathrm {c}}$ (defined in (B4)).

Figure 22. Spectra of total, magnetic, kinetic ((a), solid, dashed and dot-dashed lines, respectively, compensated by ![]() $k_{\perp }^{3/2}$) and residual energy ((b), compensated by
$k_{\perp }^{3/2}$) and residual energy ((b), compensated by ![]() $k_{\perp }^{1.9}$) in an RMHD simulation by Boldyrev et al. (Reference Boldyrev, Perez, Borovsky and Podesta2011) (© AAS, reproduced with permission). Beresnyak (Reference Beresnyak2014b) does a convergence study by rescaling to the dissipative cutoff and finds instead a
$k_{\perp }^{1.9}$) in an RMHD simulation by Boldyrev et al. (Reference Boldyrev, Perez, Borovsky and Podesta2011) (© AAS, reproduced with permission). Beresnyak (Reference Beresnyak2014b) does a convergence study by rescaling to the dissipative cutoff and finds instead a ![]() $k_{\perp }^{-1.7}$ scaling to be the best fit to the residual-energy spectrum (see his figure 3), so it is possible that what is displayed here is a large-scale transient.
$k_{\perp }^{-1.7}$ scaling to be the best fit to the residual-energy spectrum (see his figure 3), so it is possible that what is displayed here is a large-scale transient.
There are two ways in which a shade of legitimate doubt extends over both numerical and observational evidence quoted above.
(i) Beresnyak (Reference Beresnyak2014b), analysing his (largest-ever) simulations, reports that the residual energy at the small-scale end of its spectrum scales approximately as ![]() $k_{\perp }^{-1.7}$, same as his kinetic- and magnetic-energy spectra. His conclusion is that residual energy is merely a scale-independent finite fraction
$k_{\perp }^{-1.7}$, same as his kinetic- and magnetic-energy spectra. His conclusion is that residual energy is merely a scale-independent finite fraction ![]() $\approx 0.15$ of the total energy.
$\approx 0.15$ of the total energy.
(ii) Solar wind's expansion with heliocentric distance and the resulting reflection of the outward-propagating Elsasser field leads to an increase in the negative residual energy, which has nothing to do with nonlinear interactions in the locally homogeneous turbulence (see, e.g., Perez & Chandran Reference Perez and Chandran2013). The steepening of the magnetic field's spectrum compared to velocity's due to this effect is captured quite clearly in, e.g., the expanding-box simulations of Squire, Chandran & Meyrand (Reference Squire, Chandran and Meyrand2020), to whose paper I also refer the reader requiring further space-physics references on this subject. In assessing solar-wind measurements for evidence of residual energy in MHD turbulence, it may be non-trivial to separate this expansion effect from the organic local tendency of the nonlinear interactions to favour negative residual energy.
Alas, nothing is ever clear-cut in this subject, but let me press on. Obviously, it cannot be true at asymptotically small scales that, as the solar-wind data suggests, the magnetic- and kinetic-energy spectra scale as ![]() $k_{\perp }^{-5/3}$ and
$k_{\perp }^{-5/3}$ and ![]() $k_{\perp }^{-3/2}$, respectively, while their difference scales as
$k_{\perp }^{-3/2}$, respectively, while their difference scales as ![]() $k_{\perp }^{-2}$ – the
$k_{\perp }^{-2}$ – the ![]() $\boldsymbol {b}$ and
$\boldsymbol {b}$ and ![]() $\boldsymbol {u}$ spectra must meet somewhere, as they indeed do in figure 21(a). The residual energy appears to peter out at the same scale (although that is also where the noise effects kick in) – but it would not be asymptotically impossible for it to retain the
$\boldsymbol {u}$ spectra must meet somewhere, as they indeed do in figure 21(a). The residual energy appears to peter out at the same scale (although that is also where the noise effects kick in) – but it would not be asymptotically impossible for it to retain the ![]() $k_{\perp }^{-2}$ scaling as a subdominant correction to approximately equipartitioned
$k_{\perp }^{-2}$ scaling as a subdominant correction to approximately equipartitioned ![]() $\boldsymbol {b}$ and
$\boldsymbol {b}$ and ![]() $\boldsymbol {u}$ spectra (as suggested by Boldyrev et al. Reference Boldyrev, Perez, Borovsky and Podesta2011) – or perhaps to parallel, finitely offset ones (à la Beresnyak Reference Beresnyak2014b). I will discuss a plausible origin for such a correction in § 10.4, but first some history.
$\boldsymbol {u}$ spectra (as suggested by Boldyrev et al. Reference Boldyrev, Perez, Borovsky and Podesta2011) – or perhaps to parallel, finitely offset ones (à la Beresnyak Reference Beresnyak2014b). I will discuss a plausible origin for such a correction in § 10.4, but first some history.
10.2. Old theories
The first awakening of the MHD turbulence community to the turbulence's tendency for residual-energy generation dates back to the dawn of time (Pouquet, Frisch & Leorat Reference Pouquet, Frisch and Leorat1976; Grappin et al. Reference Grappin, Frisch, Pouquet and Leorat1982, Reference Grappin, Leorat and Pouquet1983), when theories and simulations based on isotropic EDQNMFootnote 48 closure models of MHD turbulence predicted a negative residual energy (i.e., an excess of magnetic energy) scaling as a ![]() $k^{-2}$ correction to the dominant
$k^{-2}$ correction to the dominant ![]() $k^{-3/2}$ IK spectrum (see § 2.2). While the isotropic IK theory certainly cannot be relevant to MHD turbulence with a strong mean field (see § 2), the modern evidence (§ 10.1) looks very much like those old results, with
$k^{-3/2}$ IK spectrum (see § 2.2). While the isotropic IK theory certainly cannot be relevant to MHD turbulence with a strong mean field (see § 2), the modern evidence (§ 10.1) looks very much like those old results, with ![]() $k$ replaced by
$k$ replaced by ![]() $k_{\perp }$. This led Müller & Grappin (Reference Müller and Grappin2005) to claim a degree of vindication for the EDQNM-based theory. This vindication cannot, however, be any stronger than the vindication of IK provided by Boldyrev's theory (§ 6.2) and its variants (§ 6.4): same scaling, different physics.
$k_{\perp }$. This led Müller & Grappin (Reference Müller and Grappin2005) to claim a degree of vindication for the EDQNM-based theory. This vindication cannot, however, be any stronger than the vindication of IK provided by Boldyrev's theory (§ 6.2) and its variants (§ 6.4): same scaling, different physics.
Below the turgid layers of EDQNM formalism, the basic physical idea (best summarised by Grappin, Müller & Verdini Reference Grappin, Müller and Verdini2016) is that residual energy is generated from the total energy by nonlinear interactions that favour magnetic-field production (the ‘dynamo effect’)Footnote 49 and removed by the ‘Alfvén effect’, which tends to equalise ![]() $\boldsymbol {u}_{\perp }$ and
$\boldsymbol {u}_{\perp }$ and ![]() $\boldsymbol {b}_{\perp }$ perturbations. A balance of these two effects leads to a prediction for the residual-energy spectrum in the form
$\boldsymbol {b}_{\perp }$ perturbations. A balance of these two effects leads to a prediction for the residual-energy spectrum in the form
where ![]() $E$ is the total-energy spectrum,
$E$ is the total-energy spectrum, ![]() $\tau _b$ is the characteristic time scale of the generation of excess magnetic energy at a given scale,
$\tau _b$ is the characteristic time scale of the generation of excess magnetic energy at a given scale, ![]() $\tau _{\mathrm {A}}$ and
$\tau _{\mathrm {A}}$ and ![]() $\tau _{\mathrm {nl}}$ are our old friends Alfvén and nonlinear times, and the exponent
$\tau _{\mathrm {nl}}$ are our old friends Alfvén and nonlinear times, and the exponent ![]() $\alpha$ depends on one's theory of how
$\alpha$ depends on one's theory of how ![]() $\tau _b$ is related to these two basic times. For example, in the IK theory,
$\tau _b$ is related to these two basic times. For example, in the IK theory, ![]() $\tau _b\sim \tau _{\mathrm {nl}}^2/\tau _{\mathrm {A}}$ (because IK turbulence is weak; cf. (4.5) and footnote 5), so
$\tau _b\sim \tau _{\mathrm {nl}}^2/\tau _{\mathrm {A}}$ (because IK turbulence is weak; cf. (4.5) and footnote 5), so ![]() $\alpha =2$. Using the IK scalings (2.5) and
$\alpha =2$. Using the IK scalings (2.5) and ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}} \sim \delta u_\lambda /v_{\mathrm {A}}$, one then gets from (10.1)
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}} \sim \delta u_\lambda /v_{\mathrm {A}}$, one then gets from (10.1)
I know of no unique or obvious way of adjusting this promising (but necessarily wrong because IK-based) result to fit a critically balanced cascade: indeed, the CB requires ![]() $\tau _{\mathrm {A}}\sim \tau _{\mathrm {nl}}$, implying
$\tau _{\mathrm {A}}\sim \tau _{\mathrm {nl}}$, implying ![]() $E_{\mathrm {res}}\sim E$, i.e., a scale-independent ratio between the residual and total energy (this was also the conclusion of Gogoberidze et al. Reference Gogoberidze, Chapman and Hnat2012, who undertook the heroic but thankless task of constructing an EDQNM theory of anisotropic, critically balanced MHD turbulence). Neither solar wind nor MHD simulations appear to agree with this (§ 10.1).
$E_{\mathrm {res}}\sim E$, i.e., a scale-independent ratio between the residual and total energy (this was also the conclusion of Gogoberidze et al. Reference Gogoberidze, Chapman and Hnat2012, who undertook the heroic but thankless task of constructing an EDQNM theory of anisotropic, critically balanced MHD turbulence). Neither solar wind nor MHD simulations appear to agree with this (§ 10.1).
Obviously, once we enter the realm of intermittent scalings of the kind described in § 6.4.1, i.e., allow the outer scale to matter, there is a whole family of possibilities admitted by the RMHD symmetry and dimensional analysis: by exactly the same argument as the one that led to (6.14) (and noting that spectrum ![]() $\sim$ amplitude
$\sim$ amplitude![]() $^2/k_{\perp }$), we must have
$^2/k_{\perp }$), we must have
where ![]() $\delta$ is some new exponent. In order to determine it, one must input some physical or mathematical insight.
$\delta$ is some new exponent. In order to determine it, one must input some physical or mathematical insight.
10.3. New theories: residual energy in weak MHD turbulence
An interesting step in this direction was made in yet another characteristically clever contribution by Boldyrev's group. They showed that even weak interactions of AW packets mathematically lead to growth of excess magnetic energy and thus of negative residual energy – Boldyrev et al. (Reference Boldyrev, Perez and Zhdankin2012) by analysing weak interaction of two model AW packets and Wang et al. (Reference Wang, Boldyrev and Perez2011) within the framework of traditional WT theory. However, all the action in their derivation was in the ![]() $k_{\parallel }=0$ modes, which hosted the excess magnetic energy generated by AW interactions – the 2D magnetic condensate whose awkward relationship with WT theory I discussed in § 4.4.
$k_{\parallel }=0$ modes, which hosted the excess magnetic energy generated by AW interactions – the 2D magnetic condensate whose awkward relationship with WT theory I discussed in § 4.4.
A version of the appropriate derivation is laid out in Appendix A.5. Quantitatively, it cannot be right because the WT approximation does not apply to the condensate, which is strongly turbulent (see Appendix A.4). Qualitatively, the outcome of the WT calculation – growth of excess magnetic energy at ![]() $k_{\parallel }=0$ – can be understood as follows. Growth of positive (negative) residual energy is the same as growth of (anti)correlation between
$k_{\parallel }=0$ – can be understood as follows. Growth of positive (negative) residual energy is the same as growth of (anti)correlation between ![]() $\boldsymbol {Z}_{\perp }^+$ and
$\boldsymbol {Z}_{\perp }^+$ and ![]() $\boldsymbol {Z}_{\perp }^-$:
$\boldsymbol {Z}_{\perp }^-$:
These correlations are created with particular ease at ![]() $k_{\parallel }=0$, where
$k_{\parallel }=0$, where ![]() $\boldsymbol {Z}_0^+$ and
$\boldsymbol {Z}_0^+$ and ![]() $\boldsymbol {Z}_0^-$ are forced by the interaction of the same pairs of AWs,
$\boldsymbol {Z}_0^-$ are forced by the interaction of the same pairs of AWs, ![]() $\boldsymbol {Z}_{k_{\parallel }}^+$ and
$\boldsymbol {Z}_{k_{\parallel }}^+$ and ![]() $\boldsymbol {Z}_{k_{\parallel }}^-$ (which themselves are allowed to be uncorrelated): this is obvious from (4.12). The result is that a magnetic condensate emerges at
$\boldsymbol {Z}_{k_{\parallel }}^-$ (which themselves are allowed to be uncorrelated): this is obvious from (4.12). The result is that a magnetic condensate emerges at ![]() $k_{\parallel }=0$, giving rise to net negative residual energy – that it should be negative is not obvious, but the WT calculation says it is (see (A38)), as, perhaps more convincingly, does a qualitative argument that I shall now explain.
$k_{\parallel }=0$, giving rise to net negative residual energy – that it should be negative is not obvious, but the WT calculation says it is (see (A38)), as, perhaps more convincingly, does a qualitative argument that I shall now explain.
10.4. New theories: residual energy in strong MHD turbulence
In the strong-turbulence regime, no quantitative calculation exists, as usual, but a reasonably compelling physical case can be made.
Emergence of negative residual energy here must be discussed in very different terms than in § 10.3. As I repeatedly stated in § 6, my preferred picture of alignment is one in which Elsasser fields dynamically shear each other into intermittent structures where they are nearly parallel to each other (Chandran et al. Reference Chandran, Schekochihin and Mallet2015). That, of course, means that they become strongly correlated: indeed, alignment between Elsasser fields is mathematically impossible without non-zero residual energy, as is obvious from the first formula in (B5) or from figure 38. That ![]() $\delta b$ should be larger than
$\delta b$ should be larger than ![]() $\delta u$ in the resulting sheet-like structures is both a selection effect and the result of dynamics.
$\delta u$ in the resulting sheet-like structures is both a selection effect and the result of dynamics.
First, the structures that have ![]() $\delta u>\delta b$ – shear layers, rather than current sheets – are prone to be destroyed by the Kelvin–Helmholtz instability and to curl up into vortices, as they do in hydrodynamic turbulence, whereas in the current sheets, the instability is happily stabilised by the magnetic field (at least before it all hits the disruption scale and current sheets become unstable as well; see § 7, Appendix D.4.2 and references therein).
$\delta u>\delta b$ – shear layers, rather than current sheets – are prone to be destroyed by the Kelvin–Helmholtz instability and to curl up into vortices, as they do in hydrodynamic turbulence, whereas in the current sheets, the instability is happily stabilised by the magnetic field (at least before it all hits the disruption scale and current sheets become unstable as well; see § 7, Appendix D.4.2 and references therein).
Secondly, there is a dynamical tendency in RMHD that favours current sheets over shear layers: the nonlinearity pushes the ‘Elsasser vorticities’ ![]() $\omega ^+ = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^+)$ and
$\omega ^+ = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^+)$ and ![]() $\omega ^- = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^-)$ in opposite directions – this is obvious from the evolution equations (A2) for these vorticities, where the nonlinear vortex-stretching terms have opposite signs for the two Elsasser fields. Thus,
$\omega ^- = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^-)$ in opposite directions – this is obvious from the evolution equations (A2) for these vorticities, where the nonlinear vortex-stretching terms have opposite signs for the two Elsasser fields. Thus, ![]() $\omega ^+$ and
$\omega ^+$ and ![]() $\omega ^-$ are ‘forced’ equally and in opposite directions at every point in space and time. The result is a negative correlation between them,
$\omega ^-$ are ‘forced’ equally and in opposite directions at every point in space and time. The result is a negative correlation between them, ![]() $\langle \omega ^+\omega ^-\rangle <0$, and thus a preference for current sheets over shear layers (see figure 23 and Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b).
$\langle \omega ^+\omega ^-\rangle <0$, and thus a preference for current sheets over shear layers (see figure 23 and Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b).

Figure 23. Joint probability distribution of Elsasser vorticities ![]() $\omega ^\pm = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^\pm )$ in an RMHD simulation by Zhdankin et al. (Reference Zhdankin, Boldyrev and Uzdensky2016b) (reprinted from Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b with the permission of AIP Publishing). The contours are elongated in the SE-NW direction, indicating
$\omega ^\pm = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^\pm )$ in an RMHD simulation by Zhdankin et al. (Reference Zhdankin, Boldyrev and Uzdensky2016b) (reprinted from Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b with the permission of AIP Publishing). The contours are elongated in the SE-NW direction, indicating ![]() $\langle \omega ^+\omega ^-\rangle <0$ and thus a preponderance of current sheets over shear layers.
$\langle \omega ^+\omega ^-\rangle <0$ and thus a preponderance of current sheets over shear layers.
Let us now imagine that this effect is strongest in the most intense structures, which in § 6.4.2 were all assumed to have the same, scale-independent amplitude. If they are current sheets with ![]() $\delta Z_{\mathrm {max}}\sim \delta b\gg \delta u$, they would, if they were alone in the world, have a spectrum of
$\delta Z_{\mathrm {max}}\sim \delta b\gg \delta u$, they would, if they were alone in the world, have a spectrum of ![]() $k_{\perp }^{-2}$ because they are just an ensemble of step functions in
$k_{\perp }^{-2}$ because they are just an ensemble of step functions in ![]() $\boldsymbol {b}_{\perp }$. The easiest way to see this is to notice that it is the spectrum of a single Heaviside step function. It is also the spectrum of many random steps: if the field flips direction randomly, with the number of flips between two points separated by a distance
$\boldsymbol {b}_{\perp }$. The easiest way to see this is to notice that it is the spectrum of a single Heaviside step function. It is also the spectrum of many random steps: if the field flips direction randomly, with the number of flips between two points separated by a distance ![]() $\lambda$ increasing
$\lambda$ increasing ![]() $\propto \lambda$, then the field increment will accumulate as a random walk:
$\propto \lambda$, then the field increment will accumulate as a random walk: ![]() $\langle \delta b_\lambda ^2\rangle \propto \lambda$, giving a
$\langle \delta b_\lambda ^2\rangle \propto \lambda$, giving a ![]() $k_{\perp }^{-2}$ spectrum.Footnote 50 In fact, there are many other fluctuations around, whose net spectrum is
$k_{\perp }^{-2}$ spectrum.Footnote 50 In fact, there are many other fluctuations around, whose net spectrum is ![]() $k_{\perp }^{-3/2}$ and in which
$k_{\perp }^{-3/2}$ and in which ![]() $\delta b\sim \delta u$. Overall, this shallower scaling would swamp
$\delta b\sim \delta u$. Overall, this shallower scaling would swamp ![]() $k_{\perp }^{-2}$. However, if the excess magnetic energy is dominated by the most intense sheets, one might imagine that the residual-energy spectrum would have a
$k_{\perp }^{-2}$. However, if the excess magnetic energy is dominated by the most intense sheets, one might imagine that the residual-energy spectrum would have a ![]() $k_{\perp }^{-2}$ scaling.
$k_{\perp }^{-2}$ scaling.
Encouragingly, recent analysis of solar-wind data by Bowen et al. (Reference Bowen, Mallet, Bonnell and Bale2018) directly established a positive correlation between the most intense, intermittent magnetic structures and residual energy. It is perhaps worth observing that the fact that a ![]() $k_{\perp }^{-2}$ spectrum consistent with the RMHD symmetry requires
$k_{\perp }^{-2}$ spectrum consistent with the RMHD symmetry requires ![]() $\delta =-1/4$ in (10.3) and, therefore, the presence of the outer scale
$\delta =-1/4$ in (10.3) and, therefore, the presence of the outer scale ![]() $L_{\parallel }$ in the expression for
$L_{\parallel }$ in the expression for ![]() $E_{\mathrm {res}}(k_{\perp })$, confirms that we must again be dealing with an intermittency effect. This said, figure 13(b) appears to be at odds with the notion of a steeper magnetic spectrum, showing smaller scaling exponents for the magnetic field than for velocity – an effect of velocity forcing? Bad statistical measurement? Sorting this out appears to be a worthwhile outstanding task.
$E_{\mathrm {res}}(k_{\perp })$, confirms that we must again be dealing with an intermittency effect. This said, figure 13(b) appears to be at odds with the notion of a steeper magnetic spectrum, showing smaller scaling exponents for the magnetic field than for velocity – an effect of velocity forcing? Bad statistical measurement? Sorting this out appears to be a worthwhile outstanding task.
Thus, admittedly, all this is less than a theory, but it is something.
10.5. Summary
Perhaps speaking of an ‘Alfvénically imbalanced regime’ of MHD turbulence is misleading. Residual energy is not an RMHD invariant, so this is not something that can be viewed as a parameter in the same way as the net Elsasser imbalance can be. It is, rather, what appears to be a feature of any (approximately balanced) MHD turbulent state (but may be a large-scale transient; see § 10.1).
This feature has so far presented itself in two seemingly distinct manifestations. The first one is the tendency for sheet-like structures in the inertial range of strong MHD turbulence to be current sheets rather than shear layers and thus to have an excess of magnetic energy – it may be possible to argue that the most extreme of these structures are responsible for a subdominant ![]() $k_{\perp }^{-2}$ spectrum of residual energy (§ 10.4). The second one is the emergence of a 2D magnetic condensate in weak MHD turbulence (§ 10.3).
$k_{\perp }^{-2}$ spectrum of residual energy (§ 10.4). The second one is the emergence of a 2D magnetic condensate in weak MHD turbulence (§ 10.3).
Are these two different phenomena? Not necessarily: in the WT context, all the residual energy is generated amongst ![]() $k_{\parallel }\approx 0$ modes, which are, in fact, strongly turbulent (see Appendices A.4 and A.5). Being strongly turbulent, this 2D condensate is strongly intermittent and appears to be dominated by sheet-like structures (Meyrand et al. Reference Meyrand, Kiyani and Galtier2015), so the physical mechanism whereby an excess of magnetic energy develops in it is likely to be the same as in strong MHD turbulence.
$k_{\parallel }\approx 0$ modes, which are, in fact, strongly turbulent (see Appendices A.4 and A.5). Being strongly turbulent, this 2D condensate is strongly intermittent and appears to be dominated by sheet-like structures (Meyrand et al. Reference Meyrand, Kiyani and Galtier2015), so the physical mechanism whereby an excess of magnetic energy develops in it is likely to be the same as in strong MHD turbulence.
11. Subviscous MHD turbulence
Let me now turn to an interesting, if somewhat boutique, regime of MHD turbulence that occurs at scales below the viscous cutoff when ![]() $\mathrm {Pm}\gg 1$. This was first studied by Cho, Lazarian & Vishniac (Reference Cho, Lazarian and Vishniac2002a), Cho et al. (Reference Cho, Lazarian and Vishniac2003) and Lazarian, Vishniac & Cho (Reference Lazarian, Vishniac and Cho2004), and recently picked up again by Xu & Lazarian (Reference Xu and Lazarian2016, Reference Xu and Lazarian2017), on the grounds that it is relevant to partially ionised interstellar medium, where viscosity is heavily dominated by the neutral atoms.Footnote 51 This is a limit in which viscous dissipation takes over from inertia in controlling the evolution of the velocity field (one might call this ‘Stokes’, or ‘Aristotelian’ dynamics; cf. Rovelli Reference Rovelli2015), while the magnetic field is still happily frozen into this viscous flow and free to have interesting MHD behaviour all the way down to the resistive scale, which, at
$\mathrm {Pm}\gg 1$. This was first studied by Cho, Lazarian & Vishniac (Reference Cho, Lazarian and Vishniac2002a), Cho et al. (Reference Cho, Lazarian and Vishniac2003) and Lazarian, Vishniac & Cho (Reference Lazarian, Vishniac and Cho2004), and recently picked up again by Xu & Lazarian (Reference Xu and Lazarian2016, Reference Xu and Lazarian2017), on the grounds that it is relevant to partially ionised interstellar medium, where viscosity is heavily dominated by the neutral atoms.Footnote 51 This is a limit in which viscous dissipation takes over from inertia in controlling the evolution of the velocity field (one might call this ‘Stokes’, or ‘Aristotelian’ dynamics; cf. Rovelli Reference Rovelli2015), while the magnetic field is still happily frozen into this viscous flow and free to have interesting MHD behaviour all the way down to the resistive scale, which, at ![]() $\mathrm {Pm}\gg 1$, is much smaller than the viscous one. The velocity perturbations below the viscous scale will be very small compared to the magnetic ones, so this is another MHD turbulent state that features an imbalance between the two fields.
$\mathrm {Pm}\gg 1$, is much smaller than the viscous one. The velocity perturbations below the viscous scale will be very small compared to the magnetic ones, so this is another MHD turbulent state that features an imbalance between the two fields.
Below, I am going to present a somewhat updated qualitative theory of the subviscous cascade – with tearing disruption and the ubiquitous Kolmogorov cutoff yet again making a cameo appearance.
11.1. Viscous cutoff
When ![]() $\mathrm {Pm}\gg 1$, there are two possibilities for the nature of turbulence at the viscous cutoff.
$\mathrm {Pm}\gg 1$, there are two possibilities for the nature of turbulence at the viscous cutoff.
The first is that ![]() $\mathrm {Pm}$ is large enough to break the condition (7.3), viz.,
$\mathrm {Pm}$ is large enough to break the condition (7.3), viz., ![]() $\mathrm {Pm}\gtrsim \mathrm {Re}^{1/9}$, so there is no tearing disruption and the (aligned) inertial-range MHD cascade encounters viscosity at the Boldyrev cutoff scale (6.24) – for
$\mathrm {Pm}\gtrsim \mathrm {Re}^{1/9}$, so there is no tearing disruption and the (aligned) inertial-range MHD cascade encounters viscosity at the Boldyrev cutoff scale (6.24) – for ![]() $\mathrm {Pm}\gg 1$, let me rename it
$\mathrm {Pm}\gg 1$, let me rename it ![]() $\lambda _{\nu }$:
$\lambda _{\nu }$:
where ![]() $\lambda _{\mathrm {CB}}$ is given by (6.16) and
$\lambda _{\mathrm {CB}}$ is given by (6.16) and ![]() $\mathrm {Re}$ by (6.23). The last two formulae follow via (6.31) (for the scale
$\mathrm {Re}$ by (6.23). The last two formulae follow via (6.31) (for the scale ![]() $\xi _{\nu }$ on which the perturbed fields vary along themselves) and (6.22) (for the parallel scale
$\xi _{\nu }$ on which the perturbed fields vary along themselves) and (6.22) (for the parallel scale ![]() $l_{\parallel \nu }$).
$l_{\parallel \nu }$).
The second (rather difficult to achieve) possibility is that ![]() $1\ll \mathrm {Pm}\ll \mathrm {Re}^{1/9}$, so (7.3) does hold and we have a tearing-mediated turbulent cascade curtailed by the Kolmogorov cutoff (7.19) – for
$1\ll \mathrm {Pm}\ll \mathrm {Re}^{1/9}$, so (7.3) does hold and we have a tearing-mediated turbulent cascade curtailed by the Kolmogorov cutoff (7.19) – for ![]() $\mathrm {Pm}\gg 1$, it is
$\mathrm {Pm}\gg 1$, it is
Either way, some finite fraction of ![]() $\varepsilon$ is thermalised at
$\varepsilon$ is thermalised at ![]() $\lambda _{\nu }$, and at
$\lambda _{\nu }$, and at ![]() $\lambda < \lambda _{\nu }$ velocity perturbations will have gradients that are smaller than the decorrelation rate at
$\lambda < \lambda _{\nu }$ velocity perturbations will have gradients that are smaller than the decorrelation rate at ![]() $\lambda _{\nu }$. This decorrelation rate is
$\lambda _{\nu }$. This decorrelation rate is
11.2. Magnetic fields at subviscous scales
In contrast to velocities, magnetic fields are immune to viscosity and so can be pushed to scales much smaller than ![]() $\lambda _{\nu }$. However, since velocity gradients are suppressed at these scales, these magnetic fields will be dominantly interacting with the viscous-scale velocities, in a scale-non-local fashion. Presumably, since the viscous-scale motions are correlated on the parallel scale
$\lambda _{\nu }$. However, since velocity gradients are suppressed at these scales, these magnetic fields will be dominantly interacting with the viscous-scale velocities, in a scale-non-local fashion. Presumably, since the viscous-scale motions are correlated on the parallel scale ![]() $l_{\parallel \nu }$, so will be these magnetic fields, i.e., there is no parallel cascade:
$l_{\parallel \nu }$, so will be these magnetic fields, i.e., there is no parallel cascade:
Numerical simulations (Cho et al. Reference Cho, Lazarian and Vishniac2002a, Reference Cho, Lazarian and Vishniac2003) confirm (11.4) and show a magnetic spectrum ![]() $\propto k^{-1}$. In the mind of any minimally erudite turbulence theorist, this cannot fail to trigger a strong temptation to consider the whole situation as a variant of Batchelor (Reference Batchelor1959) advection of a passive field: assuming a cascade of magnetic energy with cascade time
$\propto k^{-1}$. In the mind of any minimally erudite turbulence theorist, this cannot fail to trigger a strong temptation to consider the whole situation as a variant of Batchelor (Reference Batchelor1959) advection of a passive field: assuming a cascade of magnetic energy with cascade time ![]() $\tau _{\nu }$ at every scale, one gets (see Cho et al. Reference Cho, Lazarian and Vishniac2002a and figure 24a):
$\tau _{\nu }$ at every scale, one gets (see Cho et al. Reference Cho, Lazarian and Vishniac2002a and figure 24a):
where ![]() $\varepsilon _{\mathrm {m}}$ is the part of the turbulent flux that is not dissipated at the viscous cutoff (possibly about half of it, since velocity and magnetic fields have the same energies at the viscous scale and are pushed into viscous dissipation and subviscous structure, respectively, at the same rate
$\varepsilon _{\mathrm {m}}$ is the part of the turbulent flux that is not dissipated at the viscous cutoff (possibly about half of it, since velocity and magnetic fields have the same energies at the viscous scale and are pushed into viscous dissipation and subviscous structure, respectively, at the same rate ![]() $\tau _{\nu }^{-1}$). The spectrum (11.5) stretches all the way to the resistive scale, where Ohmic dissipation can rival advection:
$\tau _{\nu }^{-1}$). The spectrum (11.5) stretches all the way to the resistive scale, where Ohmic dissipation can rival advection:

Figure 24. (a) Spectra of magnetic and kinetic energy for subviscous turbulence, taken from Cho et al. (Reference Cho, Lazarian and Vishniac2003). (b) Magnetic-field strength for the filtered ![]() $k>20$ part of the field in the same simulation (from Cho et al. Reference Cho, Lazarian and Vishniac2002a; both figures © AAS, reproduced with permission). Stripy field structure is manifest.
$k>20$ part of the field in the same simulation (from Cho et al. Reference Cho, Lazarian and Vishniac2002a; both figures © AAS, reproduced with permission). Stripy field structure is manifest.
The line of reasoning leading to (11.5) should perhaps be viewed with a degree of suspicion. In a regime where magnetic fields are non-locally advected and stretched by the viscous-scale velocity field, while the latter experiences back reaction from them while constantly being dissipated by viscosity, why can one assume that magnetic energy is an independent invariant with a constant scale-to-scale flux? While this may be a plausible proposition, I do not know how to justify it beyond reasonable doubt – but I do believe the scaling (11.5) because it is bolstered by the following alternative argument of a more dynamical nature.
The situation at subviscous scales is not entirely dissimilar to a kind of dynamo (§ 13), or rather a 2D version of it in which the perturbed magnetic field ![]() $\boldsymbol {b}_{\perp }$ is randomly stretched and sheared by the viscous-scale velocity and is excused from the 2D antidynamo theorem (Zeldovich Reference Zeldovich1956) by constant resupply from the inertial range. The role of
$\boldsymbol {b}_{\perp }$ is randomly stretched and sheared by the viscous-scale velocity and is excused from the 2D antidynamo theorem (Zeldovich Reference Zeldovich1956) by constant resupply from the inertial range. The role of ![]() $\boldsymbol {B}_0$ is just to two-dimensionalise the dynamics approximately – maintaining all fields at the single parallel correlation scale
$\boldsymbol {B}_0$ is just to two-dimensionalise the dynamics approximately – maintaining all fields at the single parallel correlation scale ![]() $l_{\parallel \nu }$. The stretching and shearing of
$l_{\parallel \nu }$. The stretching and shearing of ![]() $\boldsymbol {b}_{\perp }$ leads to a folded magnetic field (figure 30) forming a stripy pattern, with multiple reversals on small scales limited from below only by
$\boldsymbol {b}_{\perp }$ leads to a folded magnetic field (figure 30) forming a stripy pattern, with multiple reversals on small scales limited from below only by ![]() $\lambda _{\eta }$ (figure 24b).Footnote 52
$\lambda _{\eta }$ (figure 24b).Footnote 52
Just like in the case of dynamo-generated fields (§ 13.3.1), our stripy fields are spatially correlated along themselves on scales ![]() $\sim \xi _{\nu }$ and so can exert coherent Lorentz forces back on the viscous-scale velocity field. These forces are tension forces consisting of two parts:
$\sim \xi _{\nu }$ and so can exert coherent Lorentz forces back on the viscous-scale velocity field. These forces are tension forces consisting of two parts:
Here let us think of ![]() $\boldsymbol {b}_{\perp }$ as just the part of
$\boldsymbol {b}_{\perp }$ as just the part of ![]() $\boldsymbol {B}$ that contains subviscous-scale variation and absorb into
$\boldsymbol {B}$ that contains subviscous-scale variation and absorb into ![]() $\boldsymbol {B}_0$ all inertial-range fields. The first term in (11.7) alternates sign on the scale
$\boldsymbol {B}_0$ all inertial-range fields. The first term in (11.7) alternates sign on the scale ![]() $\lambda _{\eta }$ (in the direction perpendicular to
$\lambda _{\eta }$ (in the direction perpendicular to ![]() $\boldsymbol {b}_{\perp }$) and so its effect on the viscous-scale motions should cancel out. In contrast, the second term is quadratic in
$\boldsymbol {b}_{\perp }$) and so its effect on the viscous-scale motions should cancel out. In contrast, the second term is quadratic in ![]() $\boldsymbol {b}_{\perp }$, and its size is
$\boldsymbol {b}_{\perp }$, and its size is ![]() $\sim b_{\perp }^2/\xi _{\nu }$. In order to be dynamically significant, it must be of the same order as the viscous and inertial forces, which are similar at the viscous scale:
$\sim b_{\perp }^2/\xi _{\nu }$. In order to be dynamically significant, it must be of the same order as the viscous and inertial forces, which are similar at the viscous scale:
On the face of it, this reproduces (11.5) (assuming ![]() $\varepsilon _{\mathrm {m}}\sim \varepsilon$). However, we need not interpret this result as specifically vindicating a Batchelor-style cascade. Instead, we could think of the reversal scale as always being
$\varepsilon _{\mathrm {m}}\sim \varepsilon$). However, we need not interpret this result as specifically vindicating a Batchelor-style cascade. Instead, we could think of the reversal scale as always being ![]() $\lambda _{\eta }$, the size of the reversing field as being
$\lambda _{\eta }$, the size of the reversing field as being ![]() $b_{\perp }\sim (\varepsilon \tau _{\nu })^{1/2}$, and interpret
$b_{\perp }\sim (\varepsilon \tau _{\nu })^{1/2}$, and interpret ![]() $\delta b_\lambda$ as the increment of a stripy field taken in two points separated by
$\delta b_\lambda$ as the increment of a stripy field taken in two points separated by ![]() $\lambda _{\eta }\ll \lambda \ll \lambda _{\nu }$. The field difference between such two points will always be either
$\lambda _{\eta }\ll \lambda \ll \lambda _{\nu }$. The field difference between such two points will always be either ![]() $\delta b_\lambda \sim 2b_{\perp }$ or zero, with equal probabilities, and so
$\delta b_\lambda \sim 2b_{\perp }$ or zero, with equal probabilities, and so ![]() $\langle \delta b_\lambda ^2\rangle \sim b_{\perp }^2\sim \varepsilon \tau _{\nu }$ (this argument is due to Yousef, Rincon & Schekochihin Reference Yousef, Rincon and Schekochihin2007, who used it to posit a
$\langle \delta b_\lambda ^2\rangle \sim b_{\perp }^2\sim \varepsilon \tau _{\nu }$ (this argument is due to Yousef, Rincon & Schekochihin Reference Yousef, Rincon and Schekochihin2007, who used it to posit a ![]() $k^{-1}$ spectrum for dynamo-generated fields at large
$k^{-1}$ spectrum for dynamo-generated fields at large ![]() $\mathrm {Pm}$, which will be visited in § 13.4.2). In other words, cascade or no cascade,
$\mathrm {Pm}$, which will be visited in § 13.4.2). In other words, cascade or no cascade, ![]() $k_{\perp }^{-1}$ can be recovered as the spectrum of sharp, repeated stripes.Footnote 53
$k_{\perp }^{-1}$ can be recovered as the spectrum of sharp, repeated stripes.Footnote 53
11.3. Velocity field at subviscous scales
Numerical simulations (Cho et al. Reference Cho, Lazarian and Vishniac2003, shown in figure 24(a)) reveal that the velocity field at subviscous scales is very small and has an approximately ![]() $k_{\perp }^{-4}$ spectrum. This can be recovered on the basis of the picture that I proposed in § 11.2, in the following way. The balance between the viscous and magnetic forces at
$k_{\perp }^{-4}$ spectrum. This can be recovered on the basis of the picture that I proposed in § 11.2, in the following way. The balance between the viscous and magnetic forces at ![]() $k_{\perp }\lambda _{\nu }\gg 1$ gives us
$k_{\perp }\lambda _{\nu }\gg 1$ gives us
where ![]() $E_u(k_{\perp })$ and
$E_u(k_{\perp })$ and ![]() $E_F(k_{\perp })$ are the spectra of the velocity and of the tension force, respectively. Let me explain why
$E_F(k_{\perp })$ are the spectra of the velocity and of the tension force, respectively. Let me explain why ![]() $E_F(k_{\perp })\sim \mathrm {const}$. If
$E_F(k_{\perp })\sim \mathrm {const}$. If ![]() $\boldsymbol {b}_{\perp }$ consists of stripes of field alternating direction on the scale
$\boldsymbol {b}_{\perp }$ consists of stripes of field alternating direction on the scale ![]() $\lambda _{\eta }$, then
$\lambda _{\eta }$, then ![]() $\boldsymbol {b}_{\perp }\cdot \boldsymbol {\nabla }\boldsymbol {b}_{\perp } \sim |\boldsymbol {b}_{\perp }|^2/\xi _{\nu }$ consists of a constant field interspersed by sharp downward spikes of width
$\boldsymbol {b}_{\perp }\cdot \boldsymbol {\nabla }\boldsymbol {b}_{\perp } \sim |\boldsymbol {b}_{\perp }|^2/\xi _{\nu }$ consists of a constant field interspersed by sharp downward spikes of width ![]() $\lambda _{\eta }$ across the field and length
$\lambda _{\eta }$ across the field and length ![]() $\xi _{\nu }$ along it. At
$\xi _{\nu }$ along it. At ![]() $k_{\perp }\lambda _{\eta }\ll 1$ and
$k_{\perp }\lambda _{\eta }\ll 1$ and ![]() $k_{\perp }\xi _{\nu }\gg 1$, these are effectively 1D delta functions, so
$k_{\perp }\xi _{\nu }\gg 1$, these are effectively 1D delta functions, so ![]() $E_F(k_{\perp })\sim \mathrm {const}$, q.e.d.Footnote 54 Note that the contribution of the first term in (11.7) to
$E_F(k_{\perp })\sim \mathrm {const}$, q.e.d.Footnote 54 Note that the contribution of the first term in (11.7) to ![]() $E_F$ should scale the same as the spectrum of
$E_F$ should scale the same as the spectrum of ![]() $\boldsymbol {b}_{\perp }$, viz.,
$\boldsymbol {b}_{\perp }$, viz., ![]() $\propto k _{\perp }^{-1}$ – or perhaps
$\propto k _{\perp }^{-1}$ – or perhaps ![]() $k_{\perp }^{-1/2}$ from the cross-term, if it does not average to zero – this should produce steeper and, therefore, subdominant contributions to
$k_{\perp }^{-1/2}$ from the cross-term, if it does not average to zero – this should produce steeper and, therefore, subdominant contributions to ![]() $E_u(k_{\perp })$.Footnote 55
$E_u(k_{\perp })$.Footnote 55
11.4. Disruption by tearing
A reader who still remembers the developments in § 7 might wonder whether these stripy fields are safe against disruption by tearing. Setting ![]() $v_{\mathrm {A}y} \sim \delta b_\lambda$ in (7.1), let us ask whether there is a disruption scale
$v_{\mathrm {A}y} \sim \delta b_\lambda$ in (7.1), let us ask whether there is a disruption scale ![]() $\lambda _{\mathrm {D,subvisc}}$ at which the local tearing rate would be larger than the stretching rate by the viscous-scale eddies:
$\lambda _{\mathrm {D,subvisc}}$ at which the local tearing rate would be larger than the stretching rate by the viscous-scale eddies:
where (11.5) was invoked for ![]() $\delta b_\lambda$. Using (11.6) to estimate the putative resistive cutoff, we get
$\delta b_\lambda$. Using (11.6) to estimate the putative resistive cutoff, we get
If we are in the parameter regime where the tearing disruption has already occurred in the inertial range (![]() $\mathrm {Pm}\lesssim \mathrm {Re}^{1/9}$) and so (11.2) holds, then
$\mathrm {Pm}\lesssim \mathrm {Re}^{1/9}$) and so (11.2) holds, then ![]() $\tau _{\nu }$ is given by the second expression in (11.3), and (11.11) implies
$\tau _{\nu }$ is given by the second expression in (11.3), and (11.11) implies
so no new disruption is possible in the subviscous range.Footnote 56
In contrast, if the inertial-range cascade was cut off in the aligned regime (![]() $\mathrm {Pm}\gtrsim \mathrm {Re}^{1/9}$), so (11.1) and the first expression in (11.3) apply, then
$\mathrm {Pm}\gtrsim \mathrm {Re}^{1/9}$), so (11.1) and the first expression in (11.3) apply, then
Modulo factors of order unity and the ludicrous smallness of the fractional power of ![]() $\mathrm {Re}$ involved, this means that if the tearing disruption did not have the chance to occur in the inertial range, it will occur in the subviscous range, and that
$\mathrm {Re}$ involved, this means that if the tearing disruption did not have the chance to occur in the inertial range, it will occur in the subviscous range, and that ![]() $\lambda _{\mathrm {D,subvisc}}$ will be the field reversal scale, not
$\lambda _{\mathrm {D,subvisc}}$ will be the field reversal scale, not ![]() $\lambda _{\eta }$. In terms of the viscous scale (11.1), which is
$\lambda _{\eta }$. In terms of the viscous scale (11.1), which is ![]() $\lambda _{\nu }\sim \lambda _{\eta }\mathrm {Pm}^{1/2}$,
$\lambda _{\nu }\sim \lambda _{\eta }\mathrm {Pm}^{1/2}$,
where I have renamed ![]() $\lambda _{\mathrm {D,subvisc}}\to \lambda _{\mathrm {D}}$, since, in this regime, this is the only disruption scale there is.
$\lambda _{\mathrm {D,subvisc}}\to \lambda _{\mathrm {D}}$, since, in this regime, this is the only disruption scale there is.
At ![]() $\lambda \lesssim \lambda _{\mathrm {D}}$, a local MHD cascade is again ignited, just like it was in § 7.2. It should not seem strange that inertial motions are again possible: viscously dominated tearing of the magnetic sheets will produce
$\lambda \lesssim \lambda _{\mathrm {D}}$, a local MHD cascade is again ignited, just like it was in § 7.2. It should not seem strange that inertial motions are again possible: viscously dominated tearing of the magnetic sheets will produce ![]() $\lambda _{\mathrm {D}}$-sized plasmoids whose turnover times are shorter than their viscous-dissipation times (I already argued this in a similar context in § 7.2.2). Indeed, similarly to (7.10), taking them to be unaligned and demanding that they pick up all the available energy flux
$\lambda _{\mathrm {D}}$-sized plasmoids whose turnover times are shorter than their viscous-dissipation times (I already argued this in a similar context in § 7.2.2). Indeed, similarly to (7.10), taking them to be unaligned and demanding that they pick up all the available energy flux ![]() $\varepsilon _{\mathrm {m}}$, one gets their amplitude
$\varepsilon _{\mathrm {m}}$, one gets their amplitude
and the associated Reynolds number for the new cascade:
This cascade is cut off, as usual, at the scale (7.19), but with this new ![]() $\mathrm {Re}$:
$\mathrm {Re}$:
the Kolmogorov scale again, obviously.
Thus, the subviscous cascade turns out to be a complicated transitional arrangement for enabling tearing disruption and restoration of the Kolmogorov cutoff (11.17). Yet again, below this cutoff, at ![]() $\lambda < \lambda _{\nu,\mathrm {new}}$, we are confronted with a purely magnetic, ‘second subviscous cascade’, but this time with the (new) viscous-scale turnover time given by the formula analogous to the second expression in (11.3), viz.,
$\lambda < \lambda _{\nu,\mathrm {new}}$, we are confronted with a purely magnetic, ‘second subviscous cascade’, but this time with the (new) viscous-scale turnover time given by the formula analogous to the second expression in (11.3), viz., ![]() $\tau _{\nu,\mathrm {new}} \sim (\varepsilon _{\mathrm {m}}/\nu )^{1/2}$. All the arguments of §§ 11.2 and 11.3 apply, but with no longer any danger of further disruption (see (11.12)).
$\tau _{\nu,\mathrm {new}} \sim (\varepsilon _{\mathrm {m}}/\nu )^{1/2}$. All the arguments of §§ 11.2 and 11.3 apply, but with no longer any danger of further disruption (see (11.12)).
A reader sceptical of the falsifiability of these arguments (given the proliferation of small fractional powers of ![]() $\mathrm {Re}$ and the piling up of twiddle algebra) might feel this is all a fiction – but it is a logical one!
$\mathrm {Re}$ and the piling up of twiddle algebra) might feel this is all a fiction – but it is a logical one!
12. Decaying MHD turbulence
Decaying MHD turbulence belongs to this part of this review because it too tends to end up in ‘imbalanced’ states dominated either by the magnetic field or by one of the Elsasser fields (and because it remains, or has done until recently, in certain important respects a ‘loose end’). On a very crude level, it is perhaps obvious that this should be so, because ideal MHD equations have two types of exact solutions for which nonlinear interactions vanish: Elsasser states (![]() $\boldsymbol {u} = \pm \boldsymbol {B}$, or
$\boldsymbol {u} = \pm \boldsymbol {B}$, or ![]() $\boldsymbol {Z}^\mp = 0$) and static force-free magnetic fields (
$\boldsymbol {Z}^\mp = 0$) and static force-free magnetic fields (![]() $\boldsymbol {B}\times \boldsymbol {J} = 0$, where
$\boldsymbol {B}\times \boldsymbol {J} = 0$, where ![]() $\boldsymbol {J} = \boldsymbol {\nabla }\times \boldsymbol {B}$). If the system finds a way towards either of these solutions, globally or locally, concentrated on scales large enough to make dissipation small, it may, subject to this small dissipation, be able to linger in those states (‘may’ because the stability of the force-free states, e.g., is not guaranteed: see discussion and references in Appendix A of Hosking, Schekochihin & Balbus Reference Hosking, Schekochihin and Balbus2020). We shall see below that both magnetically dominated scenarios and convergence to Elsasser states are possible and that recent developments point to magnetic reconnection muscling its way into this topic as well.
$\boldsymbol {J} = \boldsymbol {\nabla }\times \boldsymbol {B}$). If the system finds a way towards either of these solutions, globally or locally, concentrated on scales large enough to make dissipation small, it may, subject to this small dissipation, be able to linger in those states (‘may’ because the stability of the force-free states, e.g., is not guaranteed: see discussion and references in Appendix A of Hosking, Schekochihin & Balbus Reference Hosking, Schekochihin and Balbus2020). We shall see below that both magnetically dominated scenarios and convergence to Elsasser states are possible and that recent developments point to magnetic reconnection muscling its way into this topic as well.
The usual theoretical attitude to decaying turbulence, dating back to Kolmogorov (Reference Kolmogorov1941b,Reference Kolmogorovc), is to assume that its energy would decay slowly compared to the nonlinear interactions at small scales (simply because turnover times ![]() $\tau _{\mathrm {nl}}$ are shorter at smaller scales) and hence to expect the situation in the inertial range (below the outer scale) to be the same as in the forced case: a constant-flux energy cascade, etc. I shall discuss the numerical evidence in § 12.8, but for now let us accept this philosophy as sound. With the small scales thus taken care of, the interesting question is the large-scale behaviour: since decaying turbulence is not interfered with ‘externally’, it has the freedom to decide how fast various types of energy (kinetic, magnetic, Elsasser) decay and how the outer scale evolves. These are the ‘zeroth-order’ questions that any theory of decay must be able to answer.Footnote 57
$\tau _{\mathrm {nl}}$ are shorter at smaller scales) and hence to expect the situation in the inertial range (below the outer scale) to be the same as in the forced case: a constant-flux energy cascade, etc. I shall discuss the numerical evidence in § 12.8, but for now let us accept this philosophy as sound. With the small scales thus taken care of, the interesting question is the large-scale behaviour: since decaying turbulence is not interfered with ‘externally’, it has the freedom to decide how fast various types of energy (kinetic, magnetic, Elsasser) decay and how the outer scale evolves. These are the ‘zeroth-order’ questions that any theory of decay must be able to answer.Footnote 57
12.1. Selective decay
How to answer these questions was, like most other things in turbulence, understood already by Kolmogorov (Reference Kolmogorov1941c): one assumes that energy decays on some appropriate nonlinear time scale subject to some other invariant(s) staying constant (i.e., decaying only due to dissipation, which, at ![]() $\mathrm {Re}\to \infty$, vanishes at the outer scale); this allows one to impose enough constraints on the energy and the outer scale to determine the evolution of both. In MHD, this principle is sometimes called the ‘selective-decay hypothesis’, originating from the idea of Taylor (Reference Taylor1974) relaxation – early proponents of this view of decaying MHD turbulence were Montgomery et al. (Reference Montgomery, Turner and Vahala1978, Reference Montgomery, Turner and Vahala1979) and Matthaeus & Montgomery (Reference Matthaeus and Montgomery1980).
$\mathrm {Re}\to \infty$, vanishes at the outer scale); this allows one to impose enough constraints on the energy and the outer scale to determine the evolution of both. In MHD, this principle is sometimes called the ‘selective-decay hypothesis’, originating from the idea of Taylor (Reference Taylor1974) relaxation – early proponents of this view of decaying MHD turbulence were Montgomery et al. (Reference Montgomery, Turner and Vahala1978, Reference Montgomery, Turner and Vahala1979) and Matthaeus & Montgomery (Reference Matthaeus and Montgomery1980).
In § 12.4, I will return to what the additional invariant was for Kolmogorov, and how that can be generalised to MHD, but let me start with the most straightforward (and, historically, the earliest and most successful) application of the philosophy of selective decay – MHDturbulence in 2D.
12.1.1. Decay of 2D MHD turbulence
In 2D MHD, there are two positive-definite invariants: energy and ‘anastrophy’ ![]() $\langle A_z^2\rangle$, where
$\langle A_z^2\rangle$, where ![]() $A_z$ is the out-of-plane component of the vector potential, which in 2D behaves as a passive scalar:
$A_z$ is the out-of-plane component of the vector potential, which in 2D behaves as a passive scalar:
Like energy, it is, of course, only truly conserved when ![]() $\eta =0$, but at finite but small
$\eta =0$, but at finite but small ![]() $\eta$, it is ‘better conserved’ than energy: assuming that the latter is finite as
$\eta$, it is ‘better conserved’ than energy: assuming that the latter is finite as ![]() $\eta \to +0$,
$\eta \to +0$,
Thus, energy must decay subject to the constraint that
where ![]() $L$ is the outer scale. Assuming (faithfully to Kolmogorov Reference Kolmogorov1941b,Reference Kolmogorovc) that the (magnetic) energy decays at a rate independent of the dissipation coefficients and set instead by the outer-scale nonlinear time
$L$ is the outer scale. Assuming (faithfully to Kolmogorov Reference Kolmogorov1941b,Reference Kolmogorovc) that the (magnetic) energy decays at a rate independent of the dissipation coefficients and set instead by the outer-scale nonlinear time ![]() $\sim L/U$, one gets (Hatori Reference Hatori1984)
$\sim L/U$, one gets (Hatori Reference Hatori1984)
where ![]() $U$ has been linked to
$U$ has been linked to ![]() $B$ by assuming ideal Alfvénic dynamics (
$B$ by assuming ideal Alfvénic dynamics (![]() $U\sim B$), and
$U\sim B$), and ![]() $L$ to
$L$ to ![]() $B$ via anastrophy conservation (12.3). The scalings (12.4) were confirmed numerically by Biskamp & Welter (Reference Biskamp and Welter1989) in one of the early triumphs of high-resolution MHD simulations (earlier, lower-resolution studies were by Matthaeus & Montgomery Reference Matthaeus and Montgomery1980 and Ting, Montgomery & Matthaeus Reference Ting, Montgomery and Matthaeus1986, who explored the 2D MHD decay subject to anastrophy conservation as part of the early discussions around the ‘selective-decay hypothesis’).
$B$ via anastrophy conservation (12.3). The scalings (12.4) were confirmed numerically by Biskamp & Welter (Reference Biskamp and Welter1989) in one of the early triumphs of high-resolution MHD simulations (earlier, lower-resolution studies were by Matthaeus & Montgomery Reference Matthaeus and Montgomery1980 and Ting, Montgomery & Matthaeus Reference Ting, Montgomery and Matthaeus1986, who explored the 2D MHD decay subject to anastrophy conservation as part of the early discussions around the ‘selective-decay hypothesis’).
12.1.2. Decay of helical MHD turbulence
There is an immediate, direct generalisation of the above argument to 3D MHD without a mean field. Instead of anastrophy, the magnetic invariant in 3D MHD is helicity, ![]() $\langle H\rangle = \langle \boldsymbol {A}\cdot \boldsymbol {B}\rangle$, which again is ‘better conserved’ than energy. Indeed, assuming again that the latter decays with time in a manner independent of the dissipation coefficients, implies
$\langle H\rangle = \langle \boldsymbol {A}\cdot \boldsymbol {B}\rangle$, which again is ‘better conserved’ than energy. Indeed, assuming again that the latter decays with time in a manner independent of the dissipation coefficients, implies ![]() $\eta \langle J^2\rangle \to \mathrm {const}$ as
$\eta \langle J^2\rangle \to \mathrm {const}$ as ![]() $\eta \to +0$, whence
$\eta \to +0$, whence
Taylor (Reference Taylor1974) relaxation, which inspired the selective-decay approach to decaying MHD turbulence, was precisely the idea that MHD systems would decay to states of minimal energy subject to constant helicity.
If one adopts
as the governing constraint, the selective-decay calculation exactly analogous to (12.4) is (Hatori Reference Hatori1984; Son Reference Son1999)
again assuming ideal Alfvénic dynamics (![]() $U\sim B$).
$U\sim B$).
Here, however, what appeared a simple and compelling theory crashed against reality, or, at any rate, against what passes for reality in the world of MHD turbulence, where figments of theoretical imaginations are tested against figments of numerical computations: the scalings (12.7) badly disagreed with the latter, by Biskamp & Müller (Reference Biskamp and Müller1999, Reference Biskamp and Müller2000). Instead of ![]() $U\sim B$, these authors spotted empirically in their simulations that
$U\sim B$, these authors spotted empirically in their simulations that ![]() $U \propto B^2$ and, by modifying (12.7) accordingly, concluded
$U \propto B^2$ and, by modifying (12.7) accordingly, concluded
These scalings did indeed appear to check out numerically, both in their simulations and in some later ones (Christensson, Hindmarsh & Brandenburg Reference Christensson, Hindmarsh and Brandenburg2001; Banerjee & Jedamzik Reference Banerjee and Jedamzik2004; Frick & Stepanov Reference Frick and Stepanov2010; Berera & Linkmann Reference Berera and Linkmann2014; Brandenburg et al. Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019).Footnote 58
The status of the scalings (12.7) and (12.8) will become clear in what follows, but I want to preface what is to come by observing that the assumption ![]() $U \sim B$ underpinning (12.7) is not as intuitively obvious as it might appear to be at first glance. Formally, a system relaxing according to J. B. Taylor's principle will tend to a static state consisting of linear force-free fields. Linear force-free fields are one-scale (
$U \sim B$ underpinning (12.7) is not as intuitively obvious as it might appear to be at first glance. Formally, a system relaxing according to J. B. Taylor's principle will tend to a static state consisting of linear force-free fields. Linear force-free fields are one-scale (![]() $\boldsymbol {B}\times \boldsymbol {J} = 0$ implies
$\boldsymbol {B}\times \boldsymbol {J} = 0$ implies ![]() $\nabla ^2\boldsymbol {B} = -k^2\boldsymbol {B}$, where
$\nabla ^2\boldsymbol {B} = -k^2\boldsymbol {B}$, where ![]() $k$ is a single number that depends on the initial
$k$ is a single number that depends on the initial ![]() $\langle H\rangle$ and boundary conditions), so can hardly be thought of as a proper turbulent state, but one could nevertheless imagine MHD turbulence decaying through a sequence of magnetically dominated states featuring local patches of such fields at large scales, with flows constantly re-excited by these local patches crashing into each other (and/or going unstable). It then makes sense that the kinetic energy should be smaller than the magnetic one, and perhaps one could even hope to find magnetically dominated states in which
$\langle H\rangle$ and boundary conditions), so can hardly be thought of as a proper turbulent state, but one could nevertheless imagine MHD turbulence decaying through a sequence of magnetically dominated states featuring local patches of such fields at large scales, with flows constantly re-excited by these local patches crashing into each other (and/or going unstable). It then makes sense that the kinetic energy should be smaller than the magnetic one, and perhaps one could even hope to find magnetically dominated states in which ![]() $U/B\to 0$ with
$U/B\to 0$ with ![]() $t\to \infty$, as in (12.8).
$t\to \infty$, as in (12.8).
This argument is not a proof of anything, but it should be enough to motivate one to look carefully at the dynamical nature of the processes that underpin the decay – and, as I have emphasised repeatedly in this review, when one starts to look carefully into the nonlinear dynamics in MHD, especially in magnetically dominated configurations, sooner or later reconnection makes an entrance.
12.2. Reconnection takes over
12.2.1. Reconnection-controlled decay of 2D MHD turbulence
The argument at the end of § 12.1.2 casting doubt on the assumption ![]() $U\sim B$ in fact applies equally well to the 2D case considered in § 12.1.1, with anastrophy substituted for helicity (minimum-energy states subject to constant anastrophy are also static, linear force-free states). Except in 2D, unlike in 3D, theory (12.4) was confirmed by numerics, so why worry?
$U\sim B$ in fact applies equally well to the 2D case considered in § 12.1.1, with anastrophy substituted for helicity (minimum-energy states subject to constant anastrophy are also static, linear force-free states). Except in 2D, unlike in 3D, theory (12.4) was confirmed by numerics, so why worry?
It may well be that the only thing worse for theory than to disagree with numerics is to agree with them. It has taken 30 years since Biskamp & Welter (Reference Biskamp and Welter1989) for a clear realisation to emerge that the assumption of ideal dynamics (![]() $U\sim B$) in § 12.4 was flawed. The self-similar decaying state in which 2D MHD turbulence ends up is, in fact, quite significantly dominated by magnetic fields and, if one examines it visually, consists of magnetic islands separated by current sheets (figure 25), via which they reconnect and coalesce into bigger islands – that is how
$U\sim B$) in § 12.4 was flawed. The self-similar decaying state in which 2D MHD turbulence ends up is, in fact, quite significantly dominated by magnetic fields and, if one examines it visually, consists of magnetic islands separated by current sheets (figure 25), via which they reconnect and coalesce into bigger islands – that is how ![]() $L$ grows. While Biskamp & Welter (Reference Biskamp and Welter1989) did observe that this process was occurring in their simulations (see also Politano et al. Reference Politano, Pouquet and Sulem1989; Servidio et al. Reference Servidio, Matthaeus, Shay, Cassak and Dmitruk2009, Reference Servidio, Matthaeus, Shay, Dmitruk, Cassak and Wan2010, Reference Servidio, Dmitruk, Greco, Wan, Donato, Cassak, Shay, Carbone and Matthaeus2011a,Reference Servidio, Greco, Matthaeus, Osman and Dmitruka), it was Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019, Reference Zhou, Wu, Loureiro and Uzdensky2021) who drew the logical conclusion that the characteristic nonlinear time scale for the energy decay must then be the reconnection time:
$L$ grows. While Biskamp & Welter (Reference Biskamp and Welter1989) did observe that this process was occurring in their simulations (see also Politano et al. Reference Politano, Pouquet and Sulem1989; Servidio et al. Reference Servidio, Matthaeus, Shay, Cassak and Dmitruk2009, Reference Servidio, Matthaeus, Shay, Dmitruk, Cassak and Wan2010, Reference Servidio, Dmitruk, Greco, Wan, Donato, Cassak, Shay, Carbone and Matthaeus2011a,Reference Servidio, Greco, Matthaeus, Osman and Dmitruka), it was Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019, Reference Zhou, Wu, Loureiro and Uzdensky2021) who drew the logical conclusion that the characteristic nonlinear time scale for the energy decay must then be the reconnection time:
where ![]() $\epsilon _{\mathrm {rec}}$ is the dimensionless reconnection rate,
$\epsilon _{\mathrm {rec}}$ is the dimensionless reconnection rate, ![]() $\tilde S_L$ is the Lundquist number (adjusted for a visco-Alfvénic outflow when
$\tilde S_L$ is the Lundquist number (adjusted for a visco-Alfvénic outflow when ![]() $\mathrm {Pm}\gg 1$) and
$\mathrm {Pm}\gg 1$) and ![]() $\tilde S_{\mathrm {c}}\sim 10^4$ is its critical value above which reconnection switches from the Sweet–Parker (SP) regime (Appendix D.4.1) to the fast, plasmoid-dominated regime (Appendix D.6).Footnote 59
$\tilde S_{\mathrm {c}}\sim 10^4$ is its critical value above which reconnection switches from the Sweet–Parker (SP) regime (Appendix D.4.1) to the fast, plasmoid-dominated regime (Appendix D.6).Footnote 59

Figure 25. A snapshot of ![]() $|\boldsymbol {B}|$ from a decaying 2D MHD turbulence simulation, courtesy of D. Hosking. This run had the resolution of
$|\boldsymbol {B}|$ from a decaying 2D MHD turbulence simulation, courtesy of D. Hosking. This run had the resolution of ![]() $4608^2$ and used
$4608^2$ and used ![]() $n=4$ equal hyperviscosity and hyperresistivity (the run with
$n=4$ equal hyperviscosity and hyperresistivity (the run with ![]() $\nu _4=\eta _4=2\times 10^{-11}$ from Hosking & Schekochihin Reference Hosking and Schekochihin2021).
$\nu _4=\eta _4=2\times 10^{-11}$ from Hosking & Schekochihin Reference Hosking and Schekochihin2021).
This means that the first relation in (12.4) must, in fact, be rewritten as
Remarkably, this does not change the decay laws (12.4) because ![]() $\epsilon _{\mathrm {rec}}$ does not change with time: in the fast-reconnection regime, because
$\epsilon _{\mathrm {rec}}$ does not change with time: in the fast-reconnection regime, because ![]() $\tilde S_{\mathrm {c}}$ is a numerical constant, and in the SP regime, because
$\tilde S_{\mathrm {c}}$ is a numerical constant, and in the SP regime, because ![]() $\tilde S_L \sim \mathrm {const}$ by anastrophy conservation (12.3) (which, for pairs of reconnecting/coalescing magnetic islands of size
$\tilde S_L \sim \mathrm {const}$ by anastrophy conservation (12.3) (which, for pairs of reconnecting/coalescing magnetic islands of size ![]() $\sim L$, is just the conservation of their flux
$\sim L$, is just the conservation of their flux ![]() $\sim BL$). However, by including
$\sim BL$). However, by including ![]() $\epsilon _{\mathrm {rec}}$ into the rescaling of the time histories of
$\epsilon _{\mathrm {rec}}$ into the rescaling of the time histories of ![]() $\langle B^2\rangle$ at different
$\langle B^2\rangle$ at different ![]() $S_L$, Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019) did confirm that it was the reconnection time scale (12.9), with its
$S_L$, Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019) did confirm that it was the reconnection time scale (12.9), with its ![]() $\eta$ dependence, rather than just the Alfvén time
$\eta$ dependence, rather than just the Alfvén time ![]() $L/B$, that controlled the decay in their simulations (their
$L/B$, that controlled the decay in their simulations (their ![]() $S_L$ was small enough to stay in the SP regime). Another way to prove this is to replace the Laplacian resistivity with a hyperresistivity (dissipation operator
$S_L$ was small enough to stay in the SP regime). Another way to prove this is to replace the Laplacian resistivity with a hyperresistivity (dissipation operator ![]() $\eta _n\nabla ^n$), which, in the ‘hyper-SP’ regime, makes
$\eta _n\nabla ^n$), which, in the ‘hyper-SP’ regime, makes ![]() $\epsilon _{\mathrm {rec}}$ time-dependent and thus produces different decay laws than (12.4) – these indeed turn out to be the ones seen in such a numerical experiment (Hosking & Schekochihin Reference Hosking and Schekochihin2021, the 2D case is described in their appendix A).
$\epsilon _{\mathrm {rec}}$ time-dependent and thus produces different decay laws than (12.4) – these indeed turn out to be the ones seen in such a numerical experiment (Hosking & Schekochihin Reference Hosking and Schekochihin2021, the 2D case is described in their appendix A).
What about the kinetic energy? The turbulence that Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019) studied had none at the outset (they started with just a grid of magnetic islands), so all flows that emerged with time were due to reconnection processes. These are Alfvénic outflows (ignoring the ![]() $\mathrm {Pm}\gg 1$ case for now), so
$\mathrm {Pm}\gg 1$ case for now), so ![]() $U\sim B$, but their mean energy over the system's volume is not same as the magnetic energy because they are localised to reconnection sites, i.e., to sheets of length
$U\sim B$, but their mean energy over the system's volume is not same as the magnetic energy because they are localised to reconnection sites, i.e., to sheets of length ![]() $L$ and width
$L$ and width ![]() $\delta \sim \epsilon _{\mathrm {rec}} L$ (Hosking & Schekochihin Reference Hosking and Schekochihin2021). Therefore,
$\delta \sim \epsilon _{\mathrm {rec}} L$ (Hosking & Schekochihin Reference Hosking and Schekochihin2021). Therefore,
The turbulence is magnetically dominated because ![]() $\epsilon _{\mathrm {rec}} \ll 1$, even though the kinetic and magnetic energies’ decay laws are still the same because
$\epsilon _{\mathrm {rec}} \ll 1$, even though the kinetic and magnetic energies’ decay laws are still the same because ![]() $\epsilon _{\mathrm {rec}}\sim \mathrm {const}$.
$\epsilon _{\mathrm {rec}}\sim \mathrm {const}$.
12.2.2. Reconnection-controlled decay of helical MHD turbulence
The same arguments can be ported immediately to the 3D helical case considered in § 12.1.2. In doing so, I follow the paper by Hosking & Schekochihin (Reference Hosking and Schekochihin2021).
While the 3D dynamics might not be as vividly dominated by coalescence of magnetic structures as the 2D one is, let us nonetheless assume that the main dynamical process controlling the energy decay and transfer to larger scales is reconnection between blobs of helicity-conserving magnetic fields. To work out the consequences of this assumption, let us again replace (12.7) with (12.10), where ![]() $\epsilon _{\mathrm {rec}}$ is given by (12.9). If reconnection is fast,
$\epsilon _{\mathrm {rec}}$ is given by (12.9). If reconnection is fast, ![]() $\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ again, so the decay laws (12.7) survive, with the only amendment that kinetic energy, while decaying at the same rate as magnetic, is only a small fraction of it given by (12.11).
$\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ again, so the decay laws (12.7) survive, with the only amendment that kinetic energy, while decaying at the same rate as magnetic, is only a small fraction of it given by (12.11).
This outcome still disagrees with (12.8) and most extant numerical simulations, but it is, in fact, highly unlikely that any of these simulations are large enough to reach the fast-reconnection regime. Let us therefore work out what happens when ![]() $\epsilon _{\mathrm {rec}}$ is set by the
$\epsilon _{\mathrm {rec}}$ is set by the ![]() $\tilde S_L$-dependent SP formula in (12.9). As anastrophy is no longer conserved,
$\tilde S_L$-dependent SP formula in (12.9). As anastrophy is no longer conserved, ![]() $\tilde S_L\neq \mathrm {const}$ and (12.10) becomes
$\tilde S_L\neq \mathrm {const}$ and (12.10) becomes
Therefore, ![]() $\epsilon _{\mathrm {rec}}\propto \tilde S_L^{-1/2} \propto (BL)^{-1/2} \propto t^{-1/7}$, whence the kinetic energy's decay law is, by (12.11),
$\epsilon _{\mathrm {rec}}\propto \tilde S_L^{-1/2} \propto (BL)^{-1/2} \propto t^{-1/7}$, whence the kinetic energy's decay law is, by (12.11),
Note that, since the Lundquist number gets larger with time under this scheme (albeit quite slowly), this regime is transient, with reconnection eventually becoming fast and the scalings (12.7) and (12.11) with ![]() $\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ taking over in the long run.
$\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ taking over in the long run.
Even if we assume that simulations cannot run long enough (or to extend to large enough ![]() $L$) to reach this state, the transient scalings (12.12) and (12.13) are not particularly close to the Biskamp–Müller scalings (12.8) seen in simulations, so it would seem that these new developments bring us no confirmatory joy. In fact, things are better then they look, for two reasons.
$L$) to reach this state, the transient scalings (12.12) and (12.13) are not particularly close to the Biskamp–Müller scalings (12.8) seen in simulations, so it would seem that these new developments bring us no confirmatory joy. In fact, things are better then they look, for two reasons.
First, most numerical simulations use hyperresistivity, and the generalisation of the above scaling to the ‘hyper-SP’ reconnection does push scaling exponents of the magnetic and kinetic energy away from each other and somewhat closer to ![]() $-1/2$ and
$-1/2$ and ![]() $-1$, respectively. At any rate, the important point is that the decay laws in hyperresistive simulations that fall short of the fast-reconnection regime depend quite sensitively on the order
$-1$, respectively. At any rate, the important point is that the decay laws in hyperresistive simulations that fall short of the fast-reconnection regime depend quite sensitively on the order ![]() $n$ of the hyperresistivity and so the entire idea of reconnection-controlled decay can be tested by varying
$n$ of the hyperresistivity and so the entire idea of reconnection-controlled decay can be tested by varying ![]() $n$ – Hosking & Schekochihin (Reference Hosking and Schekochihin2021) did that and found that the decay exponents measured in such simulations agreed passably with the theoretical ones obtained in the same way as above but for different
$n$ – Hosking & Schekochihin (Reference Hosking and Schekochihin2021) did that and found that the decay exponents measured in such simulations agreed passably with the theoretical ones obtained in the same way as above but for different ![]() $n$, and disagreed fairly decisively with other theoretical schemes mooted in the literature (e.g., that of Campanelli Reference Campanelli2004, discussed in § 12.2.3).
$n$, and disagreed fairly decisively with other theoretical schemes mooted in the literature (e.g., that of Campanelli Reference Campanelli2004, discussed in § 12.2.3).
Secondly, the reconnection-controlled decay is, in fact, only justifiable if the amount of kinetic energy in the initial state is not much larger than (12.11), i.e., it is a good theory only for magnetically dominated MHD turbulence. If one starts with ![]() $\langle U^2\rangle \sim \langle B^2\rangle$, as Biskamp & Müller (Reference Biskamp and Müller1999, Reference Biskamp and Müller2000) did (or
$\langle U^2\rangle \sim \langle B^2\rangle$, as Biskamp & Müller (Reference Biskamp and Müller1999, Reference Biskamp and Müller2000) did (or ![]() $\langle U^2\rangle \gg \langle B^2\rangle$ followed by dynamo, as in Brandenburg et al. Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019), a different theory is needed to describe the initial stage of the decay (if kinetic energy still ends up decaying faster than magnetic, the system will eventually transition to the magnetically dominated regime). I shall need to introduce some further new ideas before I discuss such a theory, also proposed by Hosking & Schekochihin (Reference Hosking and Schekochihin2021), in § 12.4.3 and recover the precise scalings (12.8).
$\langle U^2\rangle \gg \langle B^2\rangle$ followed by dynamo, as in Brandenburg et al. Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019), a different theory is needed to describe the initial stage of the decay (if kinetic energy still ends up decaying faster than magnetic, the system will eventually transition to the magnetically dominated regime). I shall need to introduce some further new ideas before I discuss such a theory, also proposed by Hosking & Schekochihin (Reference Hosking and Schekochihin2021), in § 12.4.3 and recover the precise scalings (12.8).
12.2.3. Lack of rescaling symmetry for dissipation coefficients
Before I do that, let me, as a historical footnote, mention the theory of helical decay by Campanelli (Reference Campanelli2004), which can now be falsified in what appears to be a definitive way. A reader not interested in history can skip this section, as well as § 12.3, and move directly to § 12.4, where new things happen.
The theory in question is formulated in the clever language for discussing self-similar decay pioneered by Olesen (Reference Olesen1997). He observed that MHD (and, indeed, also HD) equations have the following rescaling symmetry: ![]() $\forall a$ and
$\forall a$ and ![]() $h$,
$h$,
He then posited that decaying MHD turbulence would simply go through a sequence of these transformations, with the rescaling parameter being a power of time, ![]() $a=(t/t_0)^{1/(1-h)}$, where
$a=(t/t_0)^{1/(1-h)}$, where ![]() $t_0$ is some reference (not necessarily initial) time. Then
$t_0$ is some reference (not necessarily initial) time. Then
The tricky part is, of course, to find ![]() $h$. Conservation of helicity,
$h$. Conservation of helicity, ![]() $B^2L\sim \mathrm {const}$, would require
$B^2L\sim \mathrm {const}$, would require ![]() $h=-1/2$, which gives the scalings (12.7). To get something else, Campanelli (Reference Campanelli2004) reasoned that if the magnetic field were approximately force-free, it would fall out of the momentum equation, and, the induction equation being linear, this force-free magnetic field could, therefore, be rescaled by an arbitrary constant:
$h=-1/2$, which gives the scalings (12.7). To get something else, Campanelli (Reference Campanelli2004) reasoned that if the magnetic field were approximately force-free, it would fall out of the momentum equation, and, the induction equation being linear, this force-free magnetic field could, therefore, be rescaled by an arbitrary constant: ![]() $\boldsymbol {B}\to a^{m}\boldsymbol {B}$, where
$\boldsymbol {B}\to a^{m}\boldsymbol {B}$, where ![]() $m$ did not need to be the same as
$m$ did not need to be the same as ![]() $h$. Conservation of helicity combined with the scaling of
$h$. Conservation of helicity combined with the scaling of ![]() $L$ in (12.15) then fixes
$L$ in (12.15) then fixes ![]() $m$ in terms of
$m$ in terms of ![]() $h$:
$h$:
with ![]() $h$ still undetermined;
$h$ still undetermined; ![]() $\langle U^2\rangle$ still satisfies (12.15). He then argued that
$\langle U^2\rangle$ still satisfies (12.15). He then argued that ![]() $h=-1$ because the dissipation coefficients should stay constant under the rescaling (12.14). This got him the Biskamp–Müller scalings (12.8).Footnote 60
$h=-1$ because the dissipation coefficients should stay constant under the rescaling (12.14). This got him the Biskamp–Müller scalings (12.8).Footnote 60
Campanelli's argument looks neat, but, on reflection, it is counterintuitive that everything should depend on the specific form of dissipation: indeed, if one were formally to replace viscosity and resistivity with hyperviscosity and hyperresistivity, ![]() $\eta \nabla ^2 \to \eta _n\nabla ^n$, then keeping
$\eta \nabla ^2 \to \eta _n\nabla ^n$, then keeping ![]() $\eta _n$ unchanged by the scaling (12.14) would require a different value of
$\eta _n$ unchanged by the scaling (12.14) would require a different value of ![]() $h$. Should we then expect different decay laws? This seems unlikely in the limit
$h$. Should we then expect different decay laws? This seems unlikely in the limit ![]() $\eta _n\to +0$. In any event, at finite
$\eta _n\to +0$. In any event, at finite ![]() $\eta _n$, if Campanelli is right, his theory, straightforwardly generalised, provides specific predictions for decay laws that are different for different
$\eta _n$, if Campanelli is right, his theory, straightforwardly generalised, provides specific predictions for decay laws that are different for different ![]() $n$. So does the theory of reconnection-controlled decay, in the ‘hyper-SP’ regime (§ 12.2.2) – and its prediction is different from Campanelli's. Numerical simulations with hyperdissipation are consistent with the former and rule out the latter quite convincingly (Hosking & Schekochihin Reference Hosking and Schekochihin2021).
$n$. So does the theory of reconnection-controlled decay, in the ‘hyper-SP’ regime (§ 12.2.2) – and its prediction is different from Campanelli's. Numerical simulations with hyperdissipation are consistent with the former and rule out the latter quite convincingly (Hosking & Schekochihin Reference Hosking and Schekochihin2021).
The conclusion seems to be that dissipation coefficients need not be invariant under the rescaling (12.14). This means that any decay theory that involves dissipative effects – as is the case for reconnection-controlled decay when the reconnection is not fast – need not obey Olesen's scalings (12.15) for any ![]() $h$ (and indeed the helical decay in § 12.2.2 does not).
$h$ (and indeed the helical decay in § 12.2.2 does not).
12.3. Decay of non-helical MHD turbulence: simulations and theories
What about 3D MHD turbulence with no mean field and zero helicity? Unlike anastrophy in 2D, ![]() $\langle H\rangle$ is not a sign-definite quantity and, in mirror-symmetric systems,
$\langle H\rangle$ is not a sign-definite quantity and, in mirror-symmetric systems, ![]() $\langle H\rangle =0$. This destroys the usefulness of the constant-helicity constraint (12.6) and re-opens the problem of the decay laws.Footnote 61 Physically, one might think of the helical MHD turbulence as decaying from an initial state produced by dynamo action of a helical flow (see review by Rincon Reference Rincon2019); similarly, the starting point for non-helical decay might be the saturated state of a non-helical fluctuation dynamo (§ 13).
$\langle H\rangle =0$. This destroys the usefulness of the constant-helicity constraint (12.6) and re-opens the problem of the decay laws.Footnote 61 Physically, one might think of the helical MHD turbulence as decaying from an initial state produced by dynamo action of a helical flow (see review by Rincon Reference Rincon2019); similarly, the starting point for non-helical decay might be the saturated state of a non-helical fluctuation dynamo (§ 13).
How to handle the non-helical decay has until recently remained unsettled, although quite a lot of numerical evidence about what happens in it does exist, alongside some theoretical arguments, which I will survey in a moment. I shall get to what I believe to be the right way to think about this regime in § 12.4, to which an impatient reader is welcome to turn immediately.
The conversation in the literature about non-helical decay has been heavily influenced by the fact that the 2D scalings (12.4) appear to work quite well also in 3D with zero helicity. This was reported with various degrees of certainty in a number of numerical experiments (Mac Low et al. Reference Mac Low, Klessen, Burkert and Smith1998; Biskamp & Müller Reference Biskamp and Müller1999, Reference Biskamp and Müller2000; Christensson et al. Reference Christensson, Hindmarsh and Brandenburg2001; Banerjee & Jedamzik Reference Banerjee and Jedamzik2004; Frick & Stepanov Reference Frick and Stepanov2010; Berera & Linkmann Reference Berera and Linkmann2014; Brandenburg, Kahniashvili & Tevzadze Reference Brandenburg, Kahniashvili and Tevzadze2015; Brandenburg & Kahniashvili Reference Brandenburg and Kahniashvili2017; Reppin & Banerjee Reference Reppin and Banerjee2017; Bhat et al. Reference Bhat, Zhou and Loureiro2021). The 2D analogy did not escape their authors: e.g., Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) speculated about ‘near-conservation’ of a local version of anastrophy (pace the gauge-non-invariance of ![]() $\langle A^2\rangle$). While it is true that, as observed, e.g., by Bhat et al. (Reference Bhat, Zhou and Loureiro2021),
$\langle A^2\rangle$). While it is true that, as observed, e.g., by Bhat et al. (Reference Bhat, Zhou and Loureiro2021), ![]() $\langle A^2\rangle$, while not a 3D invariant, decays slower with time than energy – indeed it does, since
$\langle A^2\rangle$, while not a 3D invariant, decays slower with time than energy – indeed it does, since ![]() $A\sim BL$,
$A\sim BL$, ![]() $B$ decays, and
$B$ decays, and ![]() $L$ grows under any sensible decay law – it is perhaps a stretch that this is the cause rather than a corollary of the latter.
$L$ grows under any sensible decay law – it is perhaps a stretch that this is the cause rather than a corollary of the latter.
Another scheme for rationalising ![]() $BL\sim \mathrm {const}$ is to argue that dynamically, the decay of the magnetic energy and the increase of the scale at which it sits are driven by mergers, via reconnection, of long flux tubes, as appears to be the case in 3D RMHD (Zhou et al. Reference Zhou, Loureiro and Uzdensky2020, see § 12.6) – Bhat et al. (Reference Bhat, Zhou and Loureiro2021) make a long and careful empirical case for such a scenario. In fact, in an earlier paper, Reppin & Banerjee (Reference Reppin and Banerjee2017) already mention (albeit gingerly and amongst other options) the possibility that ever larger magnetic structures might be generated via mergers of reconnecting flux ropes, an idea that they attribute to Müller, Malapaka & Busse (Reference Müller, Malapaka and Busse2012), who in turn credit the 2D study by Biskamp & Bremer (Reference Biskamp and Bremer1994). While it is not at all obvious (and, in fact, not true: see § 12.4.2) that flux tubes in 3D MHD without a mean field should reconnect while conserving their ‘2D poloidal flux’ (
$BL\sim \mathrm {const}$ is to argue that dynamically, the decay of the magnetic energy and the increase of the scale at which it sits are driven by mergers, via reconnection, of long flux tubes, as appears to be the case in 3D RMHD (Zhou et al. Reference Zhou, Loureiro and Uzdensky2020, see § 12.6) – Bhat et al. (Reference Bhat, Zhou and Loureiro2021) make a long and careful empirical case for such a scenario. In fact, in an earlier paper, Reppin & Banerjee (Reference Reppin and Banerjee2017) already mention (albeit gingerly and amongst other options) the possibility that ever larger magnetic structures might be generated via mergers of reconnecting flux ropes, an idea that they attribute to Müller, Malapaka & Busse (Reference Müller, Malapaka and Busse2012), who in turn credit the 2D study by Biskamp & Bremer (Reference Biskamp and Bremer1994). While it is not at all obvious (and, in fact, not true: see § 12.4.2) that flux tubes in 3D MHD without a mean field should reconnect while conserving their ‘2D poloidal flux’ (![]() $\sim BL$), it is true that the 3D non-helical decay is controlled by reconnection, just like the 2D and the 3D helical decays turned out to be in § 12.2 – the theory that I will present in § 12.4.2 will utilise this.
$\sim BL$), it is true that the 3D non-helical decay is controlled by reconnection, just like the 2D and the 3D helical decays turned out to be in § 12.2 – the theory that I will present in § 12.4.2 will utilise this.
Campanelli (Reference Campanelli2004) also has a theory of non-helical decay. This follows from his argument, already rehearsed in § 12.2.3, that the decay exponents are fixed by the requirement that the dissipation coefficients in MHD must stay constant under Olesen's rescaling symmetry (12.14), implying ![]() $h=-1$. In the non-helical case, he does not assume a force-free magnetic field, and so does not rescale it separately from (12.14). The result is the scalings (12.15), which, with
$h=-1$. In the non-helical case, he does not assume a force-free magnetic field, and so does not rescale it separately from (12.14). The result is the scalings (12.15), which, with ![]() $h=-1$, are the same as the 2D scalings (12.4). Olesen (Reference Olesen2015) commented that under this self-similarity,
$h=-1$, are the same as the 2D scalings (12.4). Olesen (Reference Olesen2015) commented that under this self-similarity, ![]() $\langle A^2\rangle = \mathrm {const}$, so anastrophy would be conserved regardless of the dimensionality of the problem (he called this ‘dimensional reduction’). Just as in the helical case, Campanelli's theory is testable numerically via its generalisation to hyperviscous and hyperresistive dissipation, and fails the numerical tests in comparison with the reconnection-controlled decay described in § 12.4.2 (Hosking & Schekochihin Reference Hosking and Schekochihin2021).
$\langle A^2\rangle = \mathrm {const}$, so anastrophy would be conserved regardless of the dimensionality of the problem (he called this ‘dimensional reduction’). Just as in the helical case, Campanelli's theory is testable numerically via its generalisation to hyperviscous and hyperresistive dissipation, and fails the numerical tests in comparison with the reconnection-controlled decay described in § 12.4.2 (Hosking & Schekochihin Reference Hosking and Schekochihin2021).
12.4. Selective decay constrained by Saffman invariants
Let me now outline a new way (or, rather, a new version of a very old way) of thinking about the non-helical MHD decay and similar problems, proposed by David Hosking (Hosking & Schekochihin Reference Hosking and Schekochihin2021).
The basic idea is that ![]() $\langle H\rangle =0$ does not mean (except in very artificial set-ups) that no part of the turbulence has any local magnetic links or twists and thus local helicity (figure 26). While helicity has to sum up to zero over the entire system, these local helical features will still impose topological constraints on the system's decay. The challenge is to express these constraints mathematically.
$\langle H\rangle =0$ does not mean (except in very artificial set-ups) that no part of the turbulence has any local magnetic links or twists and thus local helicity (figure 26). While helicity has to sum up to zero over the entire system, these local helical features will still impose topological constraints on the system's decay. The challenge is to express these constraints mathematically.

Figure 26. A cross-section of helicity density ![]() $H=\boldsymbol {A}\cdot \boldsymbol {B}$ (in Coulomb gauge,
$H=\boldsymbol {A}\cdot \boldsymbol {B}$ (in Coulomb gauge, ![]() $\boldsymbol {\nabla }\cdot \boldsymbol {A}=0$) from a decaying 3D MHD turbulence simulation (
$\boldsymbol {\nabla }\cdot \boldsymbol {A}=0$) from a decaying 3D MHD turbulence simulation (![]() $512^3$) with zero net helicity, taken from Hosking & Schekochihin (Reference Hosking and Schekochihin2022a). Red is
$512^3$) with zero net helicity, taken from Hosking & Schekochihin (Reference Hosking and Schekochihin2022a). Red is ![]() $H>0$, blue is
$H>0$, blue is ![]() $H<0$. The superimposed grey scale shows the magnitude of the current density
$H<0$. The superimposed grey scale shows the magnitude of the current density ![]() $J=|\boldsymbol {\nabla }\times \boldsymbol {B}|$, so the black patches are strong currents between, and on the edges of, local blobs of non-zero helicity – presumably, these are reconnection sites where decay occurs, at constant Hosking invariant (12.25), i.e., conserving the mean square helicity fluctuations.
$J=|\boldsymbol {\nabla }\times \boldsymbol {B}|$, so the black patches are strong currents between, and on the edges of, local blobs of non-zero helicity – presumably, these are reconnection sites where decay occurs, at constant Hosking invariant (12.25), i.e., conserving the mean square helicity fluctuations.
We shall draw inspiration from the long experience of thinking of such questions that exists in the context of (various flavours of) hydrodynamic turbulence.Footnote 62 Since Kolmogorov (Reference Kolmogorov1941c), the decay of hydrodynamic turbulence has been treated as selective decay subject to conservation of certain invariants related to the conservation of the momentum and angular momentum of the motions – but these conserved quantities, while present locally in the turbulent ‘eddies’, sum up to zero over the entire system, so cannot simply be fixed in the same way as ![]() $\langle A_z^2\rangle$ or
$\langle A_z^2\rangle$ or ![]() $\langle H\rangle$ were in §§ 12.1 and 12.2. Thus, the situation is somewhat analogous to the MHD case with
$\langle H\rangle$ were in §§ 12.1 and 12.2. Thus, the situation is somewhat analogous to the MHD case with ![]() $\langle H\rangle =0$.
$\langle H\rangle =0$.
12.4.1. Generalised Saffman invariants
Consider a conserved quantity that has a local density ![]() $\psi$ and that is not sign-definite. That
$\psi$ and that is not sign-definite. That ![]() $\langle \psi \rangle$ is conserved is expressed generically by
$\langle \psi \rangle$ is conserved is expressed generically by ![]() $\psi$ satisfying a dynamical equation of the form
$\psi$ satisfying a dynamical equation of the form
where ![]() $\boldsymbol {\Gamma }$ is the flux of
$\boldsymbol {\Gamma }$ is the flux of ![]() $\psi$. In the context of the upcoming derivations,
$\psi$. In the context of the upcoming derivations, ![]() $\psi$ might be, e.g., (a component of) the fluid velocity
$\psi$ might be, e.g., (a component of) the fluid velocity ![]() $\boldsymbol {u}$ or the helicity density
$\boldsymbol {u}$ or the helicity density ![]() $H=\boldsymbol {A}\cdot \boldsymbol {B}$. Let us now denote
$H=\boldsymbol {A}\cdot \boldsymbol {B}$. Let us now denote
where ![]() $V$ is a finite volume, and assume that
$V$ is a finite volume, and assume that ![]() $\langle \psi \rangle \equiv \lim _{V\to \infty }\overline {\psi }_V/V = 0$. The fact that the average of
$\langle \psi \rangle \equiv \lim _{V\to \infty }\overline {\psi }_V/V = 0$. The fact that the average of ![]() $\psi$ over the infinite space vanishes does not mean that its integral over a finite volume must do so, but, if we take
$\psi$ over the infinite space vanishes does not mean that its integral over a finite volume must do so, but, if we take ![]() $V\gg L^3$, where
$V\gg L^3$, where ![]() $L$ is the outer scale (correlation length) of
$L$ is the outer scale (correlation length) of ![]() $\psi$, it is reasonable to assume that the integral accumulates as a random walk:Footnote 63
$\psi$, it is reasonable to assume that the integral accumulates as a random walk:Footnote 63
 \begin{equation} \overline{\psi}_V \sim \left(\frac{V}{L^3}\right)^{1/2}\delta\psi_L L^3\quad\Rightarrow\quad \lim_{V\to\infty}\frac{\overline{\psi}_V^2}{V} \sim \delta\psi_L^2L^3, \end{equation}
\begin{equation} \overline{\psi}_V \sim \left(\frac{V}{L^3}\right)^{1/2}\delta\psi_L L^3\quad\Rightarrow\quad \lim_{V\to\infty}\frac{\overline{\psi}_V^2}{V} \sim \delta\psi_L^2L^3, \end{equation}
where ![]() $\delta \psi _L$ is the typical size of the fluctuation of
$\delta \psi _L$ is the typical size of the fluctuation of ![]() $\psi$ at its correlation scale
$\psi$ at its correlation scale ![]() $L$. This suggests that the mean square fluctuation of
$L$. This suggests that the mean square fluctuation of ![]() $\psi$ is a finite,
$\psi$ is a finite, ![]() $V$-independent quantity. It can be expressed in terms of the two-point correlation function of
$V$-independent quantity. It can be expressed in terms of the two-point correlation function of ![]() $\psi$:
$\psi$:
 \begin{equation} \lim_{V\to\infty}\frac{\overline{\psi}_V^2}{V} = \lim_{V\to\infty}\frac{1}{V}\iint_V\mathrm{d}^3\boldsymbol{r}\mathrm{d}^3\boldsymbol{r}'\,\psi(\boldsymbol{r})\psi(\boldsymbol{r}') = \int\mathrm{d}^3{\boldsymbol{l}}\left\langle\psi(\boldsymbol{r})\psi(\boldsymbol{r}+{\boldsymbol{l}})\right\rangle \equiv I_\psi. \end{equation}
\begin{equation} \lim_{V\to\infty}\frac{\overline{\psi}_V^2}{V} = \lim_{V\to\infty}\frac{1}{V}\iint_V\mathrm{d}^3\boldsymbol{r}\mathrm{d}^3\boldsymbol{r}'\,\psi(\boldsymbol{r})\psi(\boldsymbol{r}') = \int\mathrm{d}^3{\boldsymbol{l}}\left\langle\psi(\boldsymbol{r})\psi(\boldsymbol{r}+{\boldsymbol{l}})\right\rangle \equiv I_\psi. \end{equation}
The integral ![]() $I_\psi$ is finite provided correlations decay faster than
$I_\psi$ is finite provided correlations decay faster than ![]() $1/l^3$ as
$1/l^3$ as ![]() $l\to \infty$. Furthermore,
$l\to \infty$. Furthermore, ![]() $I_\psi$ is an invariant because, from (12.17),
$I_\psi$ is an invariant because, from (12.17),
The last formula holds as dissipation coefficients ![]() $\to +0$ if the dissipation terms can be argued to vanish in this limit and if all correlations decay faster than
$\to +0$ if the dissipation terms can be argued to vanish in this limit and if all correlations decay faster than ![]() $1/l^3$ (so
$1/l^3$ (so ![]() $I_\psi$ is finite and the surface integral left of the flux term vanishes).
$I_\psi$ is finite and the surface integral left of the flux term vanishes).
In a system with ![]() $\langle \psi \rangle = 0$ and
$\langle \psi \rangle = 0$ and ![]() $\psi \neq 0$ pointwise, it is subject to the conservation of
$\psi \neq 0$ pointwise, it is subject to the conservation of ![]() $I_\psi$ that one ought to assume the selective decay of energy to be happening.
$I_\psi$ that one ought to assume the selective decay of energy to be happening.
Following Davidson (Reference Davidson2013, Reference Davidson2015), I shall refer to quantities such as ![]() $I_\psi$ as (generalised) ‘Saffman invariants’. The original Saffman invariant was a measure of conservation of the linear momentum of the turbulent eddies, viz.,
$I_\psi$ as (generalised) ‘Saffman invariants’. The original Saffman invariant was a measure of conservation of the linear momentum of the turbulent eddies, viz., ![]() $\psi = \boldsymbol {u}$, and was introduced by Saffman (Reference Saffman1967), via a line of reasoning roughly analogous to the above, to constrain the decay laws for hydrodynamic turbulence with long-range correlations. His calculation, analogous to the selective-decay ones in § 12.1 (but pre-dating them considerably), is
$\psi = \boldsymbol {u}$, and was introduced by Saffman (Reference Saffman1967), via a line of reasoning roughly analogous to the above, to constrain the decay laws for hydrodynamic turbulence with long-range correlations. His calculation, analogous to the selective-decay ones in § 12.1 (but pre-dating them considerably), is
Kolmogorov (Reference Kolmogorov1941c) used (in fact, pioneered) the same method, except he assumed that ![]() $I_{\boldsymbol {u}}=0$ and conjectured that the decay of turbulence would be controlled by the Loitsyansky invariant, which is just
$I_{\boldsymbol {u}}=0$ and conjectured that the decay of turbulence would be controlled by the Loitsyansky invariant, which is just ![]() $I_\psi$ with
$I_\psi$ with ![]() $\psi = \boldsymbol {r}\times \boldsymbol {u} \equiv \boldsymbol {L}$, expressing the conservation of the angular momentum of the eddies (see Landau & Lifshitz Reference Landau and Lifshitz1987; Davidson Reference Davidson2013, Reference Davidson2015):Footnote 64
$\psi = \boldsymbol {r}\times \boldsymbol {u} \equiv \boldsymbol {L}$, expressing the conservation of the angular momentum of the eddies (see Landau & Lifshitz Reference Landau and Lifshitz1987; Davidson Reference Davidson2013, Reference Davidson2015):Footnote 64
With this constraint, one gets the famous Kolmogorov (Reference Kolmogorov1941c) laws of decay:
12.4.2. Decay of magnetically dominated non-helical MHD turbulence
Hosking & Schekochihin (Reference Hosking and Schekochihin2021) introduced a new invariant, which they called the ‘Saffman helicity invariant’ and to which I will refer as the Hosking invariant, ![]() $I_H$ (figures 27 and 26). This invariant is
$I_H$ (figures 27 and 26). This invariant is ![]() $I_\psi$ defined in (12.20) with
$I_\psi$ defined in (12.20) with ![]() $\psi = H = \boldsymbol {A}\cdot \boldsymbol {B}$, the helicity density (anticipating an erudite reader's concern, yes, they did show that it was gauge-invariant).Footnote 65 They then considered the decay of non-helical MHD turbulence subject to conservation of
$\psi = H = \boldsymbol {A}\cdot \boldsymbol {B}$, the helicity density (anticipating an erudite reader's concern, yes, they did show that it was gauge-invariant).Footnote 65 They then considered the decay of non-helical MHD turbulence subject to conservation of ![]() $I_H$,
$I_H$,
and with the assumption that the decay occurs on the reconnection time scale (12.9). When the reconnection is fast (![]() $\epsilon _{\mathrm {rec}}\sim \mathrm {const}$),
$\epsilon _{\mathrm {rec}}\sim \mathrm {const}$),
where the last formula is again (12.11) (in Olesen's language, § 12.2.3, this corresponds to ![]() $h=-5/4$). When the reconnection is slow, as in (12.12),
$h=-5/4$). When the reconnection is slow, as in (12.12),
The decay law for kinetic energy follows from (12.11) and ![]() $\epsilon _{\mathrm {rec}}\propto \tilde S_L^{-1/2}\propto (BL)^{-1/2}\propto t^{1/17}$. Note that, unlike in the case of helical decay (§ 12.2.2), the reconnection rate increases (Lundquist number decreases) with time (extremely slowly), so the system never gets out of the slow-reconnection regime. In fact, even in the case of fast reconnection (12.26), the Lundquist number decreases with time,
$\epsilon _{\mathrm {rec}}\propto \tilde S_L^{-1/2}\propto (BL)^{-1/2}\propto t^{1/17}$. Note that, unlike in the case of helical decay (§ 12.2.2), the reconnection rate increases (Lundquist number decreases) with time (extremely slowly), so the system never gets out of the slow-reconnection regime. In fact, even in the case of fast reconnection (12.26), the Lundquist number decreases with time, ![]() $\tilde S_L\propto t^{-1/9}$, so the asymptotic decay laws are ones for slow reconnection (12.27).
$\tilde S_L\propto t^{-1/9}$, so the asymptotic decay laws are ones for slow reconnection (12.27).

Figure 27. Numerical probe of the conservation of the Hosking invariant ![]() $I_H$, defined by (12.20) with
$I_H$, defined by (12.20) with ![]() $\psi = \boldsymbol {A}\cdot \boldsymbol {B}$. This plot, reproduced from Hosking & Schekochihin (Reference Hosking and Schekochihin2021), is of the mean square helicity density over a cubic volume
$\psi = \boldsymbol {A}\cdot \boldsymbol {B}$. This plot, reproduced from Hosking & Schekochihin (Reference Hosking and Schekochihin2021), is of the mean square helicity density over a cubic volume ![]() $V = (2R)^3$, at different times in a decaying, non-helical, 3D MHD turbulence simulation started in a magnetically dominated state – red-to-blue curves correspond to earlier-to-later times. The simulation box is periodic, which is why
$V = (2R)^3$, at different times in a decaying, non-helical, 3D MHD turbulence simulation started in a magnetically dominated state – red-to-blue curves correspond to earlier-to-later times. The simulation box is periodic, which is why ![]() $I_H$ is only non-zero in a range of
$I_H$ is only non-zero in a range of ![]() $R$ intermediate between the energy-containing scale
$R$ intermediate between the energy-containing scale ![]() $L$ and the box size (
$L$ and the box size (![]() $2R = 2\pi$). The inset shows the near-constancy of
$2R = 2\pi$). The inset shows the near-constancy of ![]() $I_H$ (calculated over the interval of
$I_H$ (calculated over the interval of ![]() $R$ indicated by vertical dashed lines).
$R$ indicated by vertical dashed lines).
Whilst neither (12.26) nor (12.27) are the same as the 2D scalings (12.4) that are believed to be seen in numerical simulations (§ 12.3), they are uncannily close – and almost certainly not numerically distinguishable from either each other or (12.4). Just as in the case of helical turbulence, a way to verify them numerically is to generalise (12.27) to the hyperresistive case and test the dependence of the scaling exponents on the hyperresistivity order ![]() $n$ – a test that the theory passes reasonably well (see Hosking & Schekochihin Reference Hosking and Schekochihin2021).
$n$ – a test that the theory passes reasonably well (see Hosking & Schekochihin Reference Hosking and Schekochihin2021).
As in the case of helical decay, this theory describes only magnetically dominated decay, where the kinetic energy is all due to reconnection outflows. If instead the initial state has ![]() $\langle U^2\rangle \sim \langle B^2\rangle$ (or
$\langle U^2\rangle \sim \langle B^2\rangle$ (or ![]() $\langle U^2\rangle \gg \langle B^2\rangle$ followed by dynamo), working out its decay laws requires further theoretical arrangements, described in § 12.4.3.Footnote 66
$\langle U^2\rangle \gg \langle B^2\rangle$ followed by dynamo), working out its decay laws requires further theoretical arrangements, described in § 12.4.3.Footnote 66
12.4.3. Selective decay constrained by Saffman cross-helicity invariant
The method of Saffman invariants (§ 12.4.1) is begging to be applied to another non-sign-definite conserved MHD quantity, the cross-helicity, whose density is ![]() $\boldsymbol {u}\cdot \boldsymbol {B} \equiv X$. In a balanced turbulence,
$\boldsymbol {u}\cdot \boldsymbol {B} \equiv X$. In a balanced turbulence, ![]() $\langle X\rangle = 0$, arguably a natural situation in the absence of a mean field.Footnote 67 There is then the Saffman cross-helicity invariant,
$\langle X\rangle = 0$, arguably a natural situation in the absence of a mean field.Footnote 67 There is then the Saffman cross-helicity invariant, ![]() $I_\psi$ of (12.20) with
$I_\psi$ of (12.20) with ![]() $\psi = X$ (which appears to have been first considered, for a different purpose, by Bershadskii Reference Bershadskii2019, who was inspired by the paper of Levich & Tsinober Reference Levich and Tsinober1983 already mentioned in footnote 65, and its follow-ups, e.g., Levich et al. Reference Levich, Shtilman and Tur1991 and Levich Reference Levich2009). Unlike in the case of
$\psi = X$ (which appears to have been first considered, for a different purpose, by Bershadskii Reference Bershadskii2019, who was inspired by the paper of Levich & Tsinober Reference Levich and Tsinober1983 already mentioned in footnote 65, and its follow-ups, e.g., Levich et al. Reference Levich, Shtilman and Tur1991 and Levich Reference Levich2009). Unlike in the case of ![]() $I_H$, it is not obvious (and may not be true) that
$I_H$, it is not obvious (and may not be true) that ![]() $I_X$ is conserved better than the energy because the fields involved in it (
$I_X$ is conserved better than the energy because the fields involved in it (![]() $\boldsymbol {u}$ and
$\boldsymbol {u}$ and ![]() $\boldsymbol {B}$) are the same fields as those whose mean squares make up the energy. Let us put this issue aside for further investigation and not allow it to stop us from considering what would happen if decay of MHD turbulence were constrained by the conservation of
$\boldsymbol {B}$) are the same fields as those whose mean squares make up the energy. Let us put this issue aside for further investigation and not allow it to stop us from considering what would happen if decay of MHD turbulence were constrained by the conservation of ![]() $I_X$.
$I_X$.
This is not an entirely frivolous or formalistic exercise because there is quite a lot of evidence that MHD turbulence has a tendency to break up into patches of non-zero cross-helicity (‘imbalance’) – not only in the inertial range, as I already noted in § 9.1 (see figure 17), but also in decaying, zero-mean-field turbulence, on the outer scale (e.g., Matthaeus et al. Reference Matthaeus, Pouquet, Mininni, Dmitruk and Breech2008; Servidio, Matthaeus & Dmitruk Reference Servidio, Matthaeus and Dmitruk2008). This is understandable: nonlinearity is likely to be weaker in places where the fields are closer to an Elsasser state, so decay in those places would be slower than where ![]() $Z^+\sim Z^-$, and there would be a natural tendency for the imbalanced patches to survive Darwinianly (cf. § 12.7).
$Z^+\sim Z^-$, and there would be a natural tendency for the imbalanced patches to survive Darwinianly (cf. § 12.7).
If such situations do arise, they must do so when kinetic and magnetic energies of the turbulence are (initially) not very different – precisely the case of ![]() $\langle U^2\rangle \sim \langle B^2\rangle$ that is not described by the theories of magnetically dominated decay outlined in §§ 12.2.2 and 12.4.2 and that I promised there to deal with later.
$\langle U^2\rangle \sim \langle B^2\rangle$ that is not described by the theories of magnetically dominated decay outlined in §§ 12.2.2 and 12.4.2 and that I promised there to deal with later.
Let me deal with it now, following again Hosking & Schekochihin (Reference Hosking and Schekochihin2021). Conservation of ![]() $I_X$ gives us a constraint,
$I_X$ gives us a constraint,
which in this regime should replace the estimate (12.11) of ![]() $\langle U^2\rangle$ resulting from reconnection outflows. Together with the the helicity constraint (12.6),
$\langle U^2\rangle$ resulting from reconnection outflows. Together with the the helicity constraint (12.6), ![]() $B^2L\sim \mathrm {const}$, this gives
$B^2L\sim \mathrm {const}$, this gives ![]() $U\propto B^2$ which is precisely the relationship spotted numerically by Biskamp & Müller (Reference Biskamp and Müller1999, Reference Biskamp and Müller2000). Since kinetic energy is now not small (at least initially), it is perhaps not as far-fetched as in the magnetically dominated regime to assume that the energy decays on the ideal time scale
$U\propto B^2$ which is precisely the relationship spotted numerically by Biskamp & Müller (Reference Biskamp and Müller1999, Reference Biskamp and Müller2000). Since kinetic energy is now not small (at least initially), it is perhaps not as far-fetched as in the magnetically dominated regime to assume that the energy decays on the ideal time scale ![]() $\sim L/U$. Doing so and making use of our two constraints, we get
$\sim L/U$. Doing so and making use of our two constraints, we get
These are precisely the Biskamp–Müller scalings (12.8) for helical decay. As I anticipated in § 12.2.2, since kinetic energy decays faster than magnetic, these decay laws, if correct, must be transient, eventually changing to the magnetically dominated case. This is more or less the scenario envisioned by Brandenburg et al. (Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019) based on their simulations that started with ![]() $\langle B^2\rangle \ll \langle U^2\rangle$, exhibited (helical) dynamo action that brought the magnetic field to dynamical strength, and then decayed (transiently) according to (12.29).
$\langle B^2\rangle \ll \langle U^2\rangle$, exhibited (helical) dynamo action that brought the magnetic field to dynamical strength, and then decayed (transiently) according to (12.29).
If we instead consider the non-helical case, the helicity constraint (12.6) must be replaced by the conservation of the Hosking invariant (12.25). Combined with (12.28), this gives ![]() $U\sim B^{1/5}$, i.e., magnetic field would decay faster than velocity. But that is not a sustainable proposition: by such a decay, magnetic field would be brought down below dynamical strength, whereupon it would be re-grown by dynamo action. A natural conclusion might be that magnetic field in this regime would not be able to constrain decay and instead stay just under dynamical strength and follow the velocity field, which would decay according to a purely hydrodynamic law, probably (12.24). There might be some support for this scenario in the simulations of Bhat et al. (Reference Bhat, Zhou and Loureiro2021).
$U\sim B^{1/5}$, i.e., magnetic field would decay faster than velocity. But that is not a sustainable proposition: by such a decay, magnetic field would be brought down below dynamical strength, whereupon it would be re-grown by dynamo action. A natural conclusion might be that magnetic field in this regime would not be able to constrain decay and instead stay just under dynamical strength and follow the velocity field, which would decay according to a purely hydrodynamic law, probably (12.24). There might be some support for this scenario in the simulations of Bhat et al. (Reference Bhat, Zhou and Loureiro2021).
Finally, for completeness and without much supporting evidence, but just to show the joys of the new toys that are the generalised Saffman invariants for MHD, here is what happens in 2D MHD with conserved Saffman cross-helicity – again relevant, presumably, for cases in which magnetic energy does not dominate initially. In 2D,
But the anastrophy constraint (12.3) tells us that ![]() $BL\sim \mathrm {const}$, so we must have
$BL\sim \mathrm {const}$, so we must have ![]() $U\sim \mathrm {const}$, whence
$U\sim \mathrm {const}$, whence
Thus, magnetic field will decay quite vigorously and rush to larger scales, while kinetic energy will stay constant. Just as in the case of non-helical decay in 3D, this regime cannot persist because the magnetic field will soon drop below dynamical strength – but there is no dynamo in 2D (Zeldovich Reference Zeldovich1956), so it will just decay away, exponentially in the kinematic regime, leaving 2D hydrodynamic turbulence decaying by its own, slow, devices (see Davidson Reference Davidson2013, Reference Davidson2015 for more). Qualitatively, this seems to be consistent with what Kinney et al. (Reference Kinney, Chandran, Cowley and McWilliams2000) see in their ‘2D dynamo’simulations.
12.5. Permanence of large scales vs. inverse transfer
In the introduction to § 12, I argued that the inertial-range spectrum of decaying turbulence is not an interesting new subject (I will return to this thought in § 12.8). What is interesting though is its large-scale spectrum, at ![]() $kL\ll 1$, i.e., the presence and growth, or otherwise, of long-range correlations. Consider the 1D spectrum of a statistically isotropic field
$kL\ll 1$, i.e., the presence and growth, or otherwise, of long-range correlations. Consider the 1D spectrum of a statistically isotropic field ![]() $\psi$ and Taylor-expand it in small
$\psi$ and Taylor-expand it in small ![]() $k$:
$k$:
where ![]() $I_\psi$ is the Saffman invariant (12.20) and
$I_\psi$ is the Saffman invariant (12.20) and ![]() $J_\psi = -(1/3)\int \mathrm {d}^3{\boldsymbol {l}}\,l^2 \left \langle \psi (\boldsymbol {r})\psi (\boldsymbol {r}+{\boldsymbol {l}})\right \rangle$. Obviously, the Taylor expansion can only be extended to
$J_\psi = -(1/3)\int \mathrm {d}^3{\boldsymbol {l}}\,l^2 \left \langle \psi (\boldsymbol {r})\psi (\boldsymbol {r}+{\boldsymbol {l}})\right \rangle$. Obviously, the Taylor expansion can only be extended to ![]() $k^2$ and
$k^2$ and ![]() $k^4$ if the coefficients
$k^4$ if the coefficients ![]() $I_\psi$ and
$I_\psi$ and ![]() $J_\psi$ are finite, i.e., if correlations decay faster than
$J_\psi$ are finite, i.e., if correlations decay faster than ![]() $O(l^{-3})$ and
$O(l^{-3})$ and ![]() $O(l^{-5})$, respectively.
$O(l^{-5})$, respectively.
If ![]() $I_\psi \neq 0$, then the large-scale spectrum is
$I_\psi \neq 0$, then the large-scale spectrum is ![]() $\propto k^2$ and, moreover, the energy content at low
$\propto k^2$ and, moreover, the energy content at low ![]() $k$ is frozen by the conservation of
$k$ is frozen by the conservation of ![]() $I_\psi$ – even though the outer scale
$I_\psi$ – even though the outer scale ![]() $L$ increases during decay, there is no energy transfer into larger scales, motions at those scales just take longer to decay. This feature is sometimes referred to as the ‘permanence of large scales’ (Davidson Reference Davidson2013, Reference Davidson2015).
$L$ increases during decay, there is no energy transfer into larger scales, motions at those scales just take longer to decay. This feature is sometimes referred to as the ‘permanence of large scales’ (Davidson Reference Davidson2013, Reference Davidson2015).
What, however, if ![]() $I_\psi = 0$, as it would normally be for
$I_\psi = 0$, as it would normally be for ![]() $\psi = \boldsymbol {u}$ or
$\psi = \boldsymbol {u}$ or ![]() $\psi = \boldsymbol {B}$? Then the spectrum at large scales is
$\psi = \boldsymbol {B}$? Then the spectrum at large scales is ![]() $\propto k^4$ and, if this is K41 turbulence decaying subject to the conservation of the Loitsyansky invariant (12.23),
$\propto k^4$ and, if this is K41 turbulence decaying subject to the conservation of the Loitsyansky invariant (12.23), ![]() $I_{\boldsymbol {L}}\propto J_{\boldsymbol {u}} =\mathrm {const}$, there is still permanence of large scales, although with weaker correlations. This is illustrated in figure 28(a). However,
$I_{\boldsymbol {L}}\propto J_{\boldsymbol {u}} =\mathrm {const}$, there is still permanence of large scales, although with weaker correlations. This is illustrated in figure 28(a). However, ![]() $J_\psi$ need not be an invariant for every
$J_\psi$ need not be an invariant for every ![]() $\psi$ for which
$\psi$ for which ![]() $I_\psi$ is, so, generally speaking, it will change with time. An example of that is the decay of helical MHD turbulence, the evolution of whose magnetic spectrum is shown in figure 28(c): while it still has the
$I_\psi$ is, so, generally speaking, it will change with time. An example of that is the decay of helical MHD turbulence, the evolution of whose magnetic spectrum is shown in figure 28(c): while it still has the ![]() $k^4$ long-wavelength asymptotic, the prefactor
$k^4$ long-wavelength asymptotic, the prefactor ![]() $J_{\boldsymbol {B}}$ now is manifestly not conserved, but rather grows robustly with time, meaning that magnetic energy is quite vigorously transferred to larger scales – an ‘inverse cascade’ (not necessarily local in
$J_{\boldsymbol {B}}$ now is manifestly not conserved, but rather grows robustly with time, meaning that magnetic energy is quite vigorously transferred to larger scales – an ‘inverse cascade’ (not necessarily local in ![]() $k$) associated with the conservation of magnetic helicity and its transfer to large scales, which is a well known phenomenon also in forced turbulence, often in the context of helical dynamo action (Pouquet et al. Reference Pouquet, Frisch and Leorat1976; Brandenburg Reference Brandenburg2001; Müller et al. Reference Müller, Malapaka and Busse2012; Rincon Reference Rincon2019).Footnote 68 A similar behaviour in non-helical decaying MHD turbulence has recently generated a flurry of excitement: an inverse magnetic-energy transfer was discovered there numerically by Zrake (Reference Zrake2014) and Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) (accompanied by Berera & Linkmann Reference Berera and Linkmann2014 and followed by Reppin & Banerjee Reference Reppin and Banerjee2017, Park Reference Park2017, and Bhat et al. Reference Bhat, Zhou and Loureiro2021) – figure 28(b) is from the non-helical simulation by Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) and shows healthy magnetic-energy growth at low wavenumbers.Footnote 69
$k$) associated with the conservation of magnetic helicity and its transfer to large scales, which is a well known phenomenon also in forced turbulence, often in the context of helical dynamo action (Pouquet et al. Reference Pouquet, Frisch and Leorat1976; Brandenburg Reference Brandenburg2001; Müller et al. Reference Müller, Malapaka and Busse2012; Rincon Reference Rincon2019).Footnote 68 A similar behaviour in non-helical decaying MHD turbulence has recently generated a flurry of excitement: an inverse magnetic-energy transfer was discovered there numerically by Zrake (Reference Zrake2014) and Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) (accompanied by Berera & Linkmann Reference Berera and Linkmann2014 and followed by Reppin & Banerjee Reference Reppin and Banerjee2017, Park Reference Park2017, and Bhat et al. Reference Bhat, Zhou and Loureiro2021) – figure 28(b) is from the non-helical simulation by Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) and shows healthy magnetic-energy growth at low wavenumbers.Footnote 69

Figure 28. Spectra of kinetic (blue) and magnetic (red) energies in decaying turbulence: (a) pure hydrodynamic, (b) MHD with no mean field and zero helicity, (c) MHD with no mean field and finite helicity. The time evolution is from right to left (always towards larger scales). These plots are from Brandenburg & Kahniashvili (Reference Brandenburg and Kahniashvili2017).
12.5.1. Scalings for inverse transfer of magnetic energy
Since we now do have a theory of the decay of MHD turbulence of any flavour, we can easily work out whether and how the large-scale spectrum grows: e.g.,
 \begin{align} J_{\boldsymbol{B}} \sim B^2L^5 \sim \left\{\begin{array}{@{}ll} t^{8/3}, & \text{(12.7), helical, ideal/fast-reconnecting}, B\gg U,\\ t^{16/7}, & \text{(12.12), helical, SP-reconnecting}, B\gg U,\\ t^2, & \text{(12.29), helical, ideal}, B\sim U,\\ t^{10/9}, & \text{(12.26), non-helical, ideal/fast-reconnecting}, B\gg U,\\ t^{20/17}, & \text{(12.27), non-helical, SP-reconnecting}, B\gg U,\\ t^{(5+2~m)/(1-h)}, & \$ \text{12.2.3, self-similar}. \end{array}\right. \end{align}
\begin{align} J_{\boldsymbol{B}} \sim B^2L^5 \sim \left\{\begin{array}{@{}ll} t^{8/3}, & \text{(12.7), helical, ideal/fast-reconnecting}, B\gg U,\\ t^{16/7}, & \text{(12.12), helical, SP-reconnecting}, B\gg U,\\ t^2, & \text{(12.29), helical, ideal}, B\sim U,\\ t^{10/9}, & \text{(12.26), non-helical, ideal/fast-reconnecting}, B\gg U,\\ t^{20/17}, & \text{(12.27), non-helical, SP-reconnecting}, B\gg U,\\ t^{(5+2~m)/(1-h)}, & \$ \text{12.2.3, self-similar}. \end{array}\right. \end{align}Thus, in all conceivable regimes, there is some inverse transfer of energy to large scales – not altogether surprising if the underlying dynamics involves mergers (by reconnection) of structures, rather than merely slower decay of the larger ones.
Obviously, the same logic can be applied to spectra of all other fields. E.g., Hosking & Schekochihin (Reference Hosking and Schekochihin2021) observe (and confirm numerically) that, for reconnection-dominated decay of non-helical MHD turbulence, the spectrum of the quantity ![]() $H=\boldsymbol {A}\cdot \boldsymbol {B}$ is
$H=\boldsymbol {A}\cdot \boldsymbol {B}$ is ![]() $\langle |H_{\boldsymbol {k}}|^2\rangle \propto I_H = \mathrm {const}$ at low
$\langle |H_{\boldsymbol {k}}|^2\rangle \propto I_H = \mathrm {const}$ at low ![]() $k$, recovering, in a certain sense, the principle of permanence of large scales (and providing another way to test the conservation of the Hosking invariant
$k$, recovering, in a certain sense, the principle of permanence of large scales (and providing another way to test the conservation of the Hosking invariant ![]() $I_H$).
$I_H$).
12.5.2. Self-similar spectra and inverse energy transfer
Let me show, for completeness, and for context, in view of the discussions that appear in the literature, how to obtain these results in the language of self-similar solutions introduced in § 12.2.3. Following Olesen (Reference Olesen1997), let us work out what the symmetry (12.14) implies for the spectrum of any of the fields that have it. For example, for the magnetic field, the spectrum satisfies
where I used Campanelli's more general rescaling ![]() $\boldsymbol {B}\to a^m\boldsymbol {B}$, where
$\boldsymbol {B}\to a^m\boldsymbol {B}$, where ![]() $m=-1/2$ with helicity and
$m=-1/2$ with helicity and ![]() $m=h$ without. A self-similarly evolving solution of (12.34) is
$m=h$ without. A self-similarly evolving solution of (12.34) is
where ![]() $f(x)$ is some function that needs to be integrable in an appropriate way in order for the total energy to be finite:
$f(x)$ is some function that needs to be integrable in an appropriate way in order for the total energy to be finite:
The decay laws (12.7), (12.29), and (12.26) are recovered for ![]() $(m,h)=(-1/2,-1/2)$,
$(m,h)=(-1/2,-1/2)$, ![]() $(-1/2,-1)$,
$(-1/2,-1)$, ![]() $(-5/4,-5/4)$, respectively (the SP-reconnecting cases (12.12) and (12.27) are non-self-similar).
$(-5/4,-5/4)$, respectively (the SP-reconnecting cases (12.12) and (12.27) are non-self-similar).
If ![]() $I_{\boldsymbol {B}}=0$, the magnetic-energy spectrum must be
$I_{\boldsymbol {B}}=0$, the magnetic-energy spectrum must be ![]() $\propto k^4$ at
$\propto k^4$ at ![]() $kL\ll 1$. This requires
$kL\ll 1$. This requires ![]() $f(x)\propto x^{5+2m}$, whence
$f(x)\propto x^{5+2m}$, whence
This is the same result as the last formula in (12.33). The solution (12.35) also implies that the peak of the spectrum, at ![]() $kL\sim 1$, is
$kL\sim 1$, is ![]() $E_\mathrm {max} \propto t^{(1+2m)/(1-h)}=\mathrm {const}$ for the helical case (manifestly true in figure 28c) and
$E_\mathrm {max} \propto t^{(1+2m)/(1-h)}=\mathrm {const}$ for the helical case (manifestly true in figure 28c) and ![]() $E_\mathrm {max}\propto t^{-1/2}$ for the non-helical one (figure 28b). Brandenburg & Kahniashvili (Reference Brandenburg and Kahniashvili2017) show that rescaling their time-dependent spectra in line with (12.35), or, to be precise, with the equivalent expression
$E_\mathrm {max}\propto t^{-1/2}$ for the non-helical one (figure 28b). Brandenburg & Kahniashvili (Reference Brandenburg and Kahniashvili2017) show that rescaling their time-dependent spectra in line with (12.35), or, to be precise, with the equivalent expression ![]() $E(k,t) = L(t)^{1+2m}g\bigl (kL(t)\bigr )$, where
$E(k,t) = L(t)^{1+2m}g\bigl (kL(t)\bigr )$, where ![]() $L(t)$ is measured directly at every
$L(t)$ is measured directly at every ![]() $t$, collapses them all onto a single curve, confirming self-similarity.
$t$, collapses them all onto a single curve, confirming self-similarity.
Let me observe, finally, that if the prefactor of the low-![]() $k$ asymptotic of
$k$ asymptotic of ![]() $E(k)$ changes with time, as it does in (12.37), i.e., if
$E(k)$ changes with time, as it does in (12.37), i.e., if ![]() $J_{\boldsymbol {B}}$ is not conserved, I see no reason to expect that the long-term self-similar evolution should be tied to the low-
$J_{\boldsymbol {B}}$ is not conserved, I see no reason to expect that the long-term self-similar evolution should be tied to the low-![]() $k$ scaling baked into the initial condition, as many authors, starting with Olesen (Reference Olesen1997), seem to believe. The self-similar evolution need not start at
$k$ scaling baked into the initial condition, as many authors, starting with Olesen (Reference Olesen1997), seem to believe. The self-similar evolution need not start at ![]() $t=0$, and it is perfectly possible that it is preceded by some initial non-self-similar rearrangement. There appears to be convincing numerical evidence that this is indeed what happens (e.g., Brandenburg & Kahniashvili Reference Brandenburg and Kahniashvili2017; Brandenburg et al. Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019; Hosking & Schekochihin Reference Hosking and Schekochihin2021).
$t=0$, and it is perfectly possible that it is preceded by some initial non-self-similar rearrangement. There appears to be convincing numerical evidence that this is indeed what happens (e.g., Brandenburg & Kahniashvili Reference Brandenburg and Kahniashvili2017; Brandenburg et al. Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019; Hosking & Schekochihin Reference Hosking and Schekochihin2021).
12.6. Decay of Magnetically Dominated RMHD Turbulence
Let me now finally return to MHD turbulence with a strong mean field (RMHD turbulence), which elsewhere in this review has been my primary preoccupation. Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020) initialised an RMHD simulation with an array of magnetic flux tubes parallel to the mean field and found that it behaved rather similarly to the magnetically dominated 2D MHD (§ 12.2.1), with flux tubes reconnecting (coalescing) with each other in the perpendicular plane, and the system thus decaying towards a state dominated by ever-larger-scale magnetic structures. While the ‘perpendicular anastrophy’ ![]() $\langle A_\parallel ^2\rangle$ is not formally conserved in RMHD, Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020) assumed that RMHD reconnection would nevertheless proceed in a quasi-2D fashion, preserving the poloidal ‘2D flux’:
$\langle A_\parallel ^2\rangle$ is not formally conserved in RMHD, Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020) assumed that RMHD reconnection would nevertheless proceed in a quasi-2D fashion, preserving the poloidal ‘2D flux’:
If one accepts this, the rest of the argument is exactly the same as in 2D MHD (§§ 12.1.1 and 12.2.1): replacing ![]() $B\to b _{\perp }$,
$B\to b _{\perp }$, ![]() $U\to u _{{\perp }}$, and
$U\to u _{{\perp }}$, and ![]() $L\to L _{\perp }$, one gets
$L\to L _{\perp }$, one gets
Since (12.38) implies ![]() $\tilde S_{L_{\perp }}\sim \mathrm {const}$,
$\tilde S_{L_{\perp }}\sim \mathrm {const}$, ![]() $\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ in both fast and SP reconnection regimes.
$\epsilon _{\mathrm {rec}}\sim \mathrm {const}$ in both fast and SP reconnection regimes.
A new feature is to argue that the length of the tubes along the mean field is determined by the CB condition:
Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020) check the scalings (12.39) and (12.40) in their RMHD simulations (figure 29) and declare success. It is interesting to analyse the ingredients of this success in light of the long discussion of selective decay in MHD given in the preceding sections.

Figure 29. Snapshots of vertical (![]() $z$) current density in the
$z$) current density in the ![]() $(x,y,0)$ (top row) and
$(x,y,0)$ (top row) and ![]() $(x,0,z)$ (bottom row) planes, taken at a series of subsequent times in a decaying RMHD simulation by Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020). This illustrates growth of
$(x,0,z)$ (bottom row) planes, taken at a series of subsequent times in a decaying RMHD simulation by Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020). This illustrates growth of ![]() $L_{\perp }\propto \sqrt {t}$ and
$L_{\perp }\propto \sqrt {t}$ and ![]() $L_{\parallel }\propto t$ (the scalings confirmed quantitatively in their paper) and the presence of numerous current sheets.
$L_{\parallel }\propto t$ (the scalings confirmed quantitatively in their paper) and the presence of numerous current sheets.
12.6.1. Saffman cross-helicity invariant in RMHD
The adoption of (12.38) in 3D is, of course, a bit of a sleight of hand. This is not a flux-conservation constraint: the 3D poloidal flux through the radial cross-section of the flux tube, ![]() $\sim b_{\perp }L_{\perp } L_{\parallel }$, does not stay constant under this scheme; if it did, critical balance (12.40) would then imply
$\sim b_{\perp }L_{\perp } L_{\parallel }$, does not stay constant under this scheme; if it did, critical balance (12.40) would then imply ![]() $L_{\perp }\sim \mathrm {const}$, which cannot be true.
$L_{\perp }\sim \mathrm {const}$, which cannot be true.
There is, however, another way to justify (12.38) (Hosking & Schekochihin Reference Hosking and Schekochihin2021). Since we are dealing with an RMHD system initialised with ![]() $u_{{\perp }}=0$, the cross-helicity
$u_{{\perp }}=0$, the cross-helicity ![]() $\langle X\rangle =\langle \boldsymbol {u}_{\perp }\cdot \boldsymbol {b}_{\perp }\rangle$ is zero initially and will stay small as long as
$\langle X\rangle =\langle \boldsymbol {u}_{\perp }\cdot \boldsymbol {b}_{\perp }\rangle$ is zero initially and will stay small as long as ![]() $u_{{\perp }}\ll b _{\perp }$. The logical application of the principles advocated in § 12.4 is then to posit the conservation of the Saffman cross-helicity invariant:
$u_{{\perp }}\ll b _{\perp }$. The logical application of the principles advocated in § 12.4 is then to posit the conservation of the Saffman cross-helicity invariant:
assuming ![]() $u_{{\perp }}\propto b _{\perp }$ (Alfvénic flows) and
$u_{{\perp }}\propto b _{\perp }$ (Alfvénic flows) and ![]() $L_{\parallel }\propto L_{\perp }/b_{\perp }$ (CB).
$L_{\parallel }\propto L_{\perp }/b_{\perp }$ (CB).
It is an open question whether a decaying RMHD can stay in a balanced state, ![]() $\langle X\rangle = 0$, forever, or whether a fluctuation of
$\langle X\rangle = 0$, forever, or whether a fluctuation of ![]() $\langle X\rangle$ away from zero can eventually tip it over to another decaying regime, tending to an Elsasser state (§ 12.7).
$\langle X\rangle$ away from zero can eventually tip it over to another decaying regime, tending to an Elsasser state (§ 12.7).
12.6.2. Self-similar decay in RMHD
The reader who liked Olesen's approach (§§ 12.2.3 and 12.5.2) and remembers the RMHD symmetry (3.5) has been straining at the leash to apply the former to the latter. It is, of course, the same symmetry as (12.14) if one lets ![]() $\epsilon = a^h$, except now perpendicular and parallel gradients and, therefore, distances transform differently from each other:Footnote 70
$\epsilon = a^h$, except now perpendicular and parallel gradients and, therefore, distances transform differently from each other:Footnote 70
This is just because ![]() $v_{\mathrm {A}}$ is now assumed to be an immutable constant, so
$v_{\mathrm {A}}$ is now assumed to be an immutable constant, so ![]() $r_{\parallel }$ transforms as time, rather than as distance (cf. Beresnyak Reference Beresnyak2015, and § 5.2). The resulting scalings,
$r_{\parallel }$ transforms as time, rather than as distance (cf. Beresnyak Reference Beresnyak2015, and § 5.2). The resulting scalings,
instantly reproduce (12.39) and (12.40) if ![]() $h=-1$. A useful observation perhaps is that the scaling of the parallel correlation length with time is set just by the requirement of self-similar evolution, without the need to conjecture CB explicitly, although of course the RMHD symmetry (3.5) does, in a sense, have CB hard-wired into it.
$h=-1$. A useful observation perhaps is that the scaling of the parallel correlation length with time is set just by the requirement of self-similar evolution, without the need to conjecture CB explicitly, although of course the RMHD symmetry (3.5) does, in a sense, have CB hard-wired into it.
Let me make another observation that seems to be of some (possibly marginal) interest. In the same vein as (12.34), one finds, this time for the 2D spectra:
The self-similar solution analogous to (12.35) is, therefore,
with some unknown function ![]() $F(x,y)$. The 1D perpendicular spectrum
$F(x,y)$. The 1D perpendicular spectrum ![]() $E(k_{\perp },t)$ is found by integrating (12.45) over all
$E(k_{\perp },t)$ is found by integrating (12.45) over all ![]() $k_{\parallel }$, predictably leading to the same result as (12.35) (with
$k_{\parallel }$, predictably leading to the same result as (12.35) (with ![]() $m=h$); the analog of (12.37) is
$m=h$); the analog of (12.37) is
again manifesting inverse transfer if ![]() $h=-1$. Integrating (12.45) over
$h=-1$. Integrating (12.45) over ![]() $k_{\perp }$ instead, one gets the 1D parallel spectrum:
$k_{\perp }$ instead, one gets the 1D parallel spectrum:
where ![]() $g(y)=\int _0^\infty \mathrm {d} x x^{-2-h}F(x,y)$. This result is interesting for the following reason.
$g(y)=\int _0^\infty \mathrm {d} x x^{-2-h}F(x,y)$. This result is interesting for the following reason. ![]() $E(k_{\parallel },t)$ is the spectrum of a random field reflecting its dependence on a single scalar spatial coordinate, the distance along the field. The long-wavelength,
$E(k_{\parallel },t)$ is the spectrum of a random field reflecting its dependence on a single scalar spatial coordinate, the distance along the field. The long-wavelength, ![]() $k_{\parallel} L _{\parallel } \ll 1$, asymptotic of this spectrum describes the absence of correlations at point separations
$k_{\parallel} L _{\parallel } \ll 1$, asymptotic of this spectrum describes the absence of correlations at point separations ![]() $l_{\parallel }\gg L _{\parallel }$, so it is just the spectrum of a 1D white noise (cf. Appendix C.1). Therefore,
$l_{\parallel }\gg L _{\parallel }$, so it is just the spectrum of a 1D white noise (cf. Appendix C.1). Therefore, ![]() $g(y)\to \mathrm {const}$ as
$g(y)\to \mathrm {const}$ as ![]() $y\to 0$. But (12.47) then implies that the energy content of low
$y\to 0$. But (12.47) then implies that the energy content of low ![]() $k_{\parallel }$ is frozen in time if
$k_{\parallel }$ is frozen in time if ![]() $h=-1$. This suggests that RMHD turbulence might have a ‘parallel Saffman invariant’
$h=-1$. This suggests that RMHD turbulence might have a ‘parallel Saffman invariant’ ![]() $I_{\parallel }$, so
$I_{\parallel }$, so ![]() $E(k_{\parallel },t) \propto I_{\parallel }k_{\parallel }^0 = \mathrm {const}$ at
$E(k_{\parallel },t) \propto I_{\parallel }k_{\parallel }^0 = \mathrm {const}$ at ![]() $k_{\parallel} L _{\parallel }\ll 1$ (cf. § 12.5). This invariant should have the formFootnote 71
$k_{\parallel} L _{\parallel }\ll 1$ (cf. § 12.5). This invariant should have the formFootnote 71
(instead of ![]() $\boldsymbol {b}_{\perp }$, it may involve some other linear combination of the fields
$\boldsymbol {b}_{\perp }$, it may involve some other linear combination of the fields ![]() $\boldsymbol {b}_{\perp }$ and
$\boldsymbol {b}_{\perp }$ and ![]() $\boldsymbol {u}_{\perp }$, or
$\boldsymbol {u}_{\perp }$, or ![]() $\boldsymbol {Z}_{\perp }^\pm$). If this could be shown to be the relevant conservation law for an RMHD selective decay, that would be an alternative way, to (12.41), of getting the scalings (12.39).
$\boldsymbol {Z}_{\perp }^\pm$). If this could be shown to be the relevant conservation law for an RMHD selective decay, that would be an alternative way, to (12.41), of getting the scalings (12.39).
12.7. Decay of imbalanced MHD turbulence: towards Elsasser states
Finally, let us consider RMHD (or indeed MHD) with ![]() $\langle X\rangle \neq 0$. The eventual decay of imbalanced MHD turbulence to pure Elsasser states was first mooted by Dobrowolny et al. (Reference Dobrowolny, Mangeney and Veltri1980), in response to such states being occasionally observed in the solar wind. Since
$\langle X\rangle \neq 0$. The eventual decay of imbalanced MHD turbulence to pure Elsasser states was first mooted by Dobrowolny et al. (Reference Dobrowolny, Mangeney and Veltri1980), in response to such states being occasionally observed in the solar wind. Since ![]() $\boldsymbol {Z}^+$ and
$\boldsymbol {Z}^+$ and ![]() $\boldsymbol {Z}^-$ advect each other, one can easily imagine that a fluctuation of the imbalance at the outer scale in one direction, say in favour of
$\boldsymbol {Z}^-$ advect each other, one can easily imagine that a fluctuation of the imbalance at the outer scale in one direction, say in favour of ![]() $\boldsymbol {Z}^+$, will lead to
$\boldsymbol {Z}^+$, will lead to ![]() $\boldsymbol {Z}^+$ decaying slower and
$\boldsymbol {Z}^+$ decaying slower and ![]() $\boldsymbol {Z}^-$ faster, thus increasing the imbalance further, until
$\boldsymbol {Z}^-$ faster, thus increasing the imbalance further, until ![]() $\boldsymbol {Z}^-$ disappears and
$\boldsymbol {Z}^-$ disappears and ![]() $\boldsymbol {Z}^+$ is left in splendid isolation. The crudest model of this is as follows (Maron & Goldreich Reference Maron and Goldreich2001): if
$\boldsymbol {Z}^+$ is left in splendid isolation. The crudest model of this is as follows (Maron & Goldreich Reference Maron and Goldreich2001): if ![]() $L$ is the energy-containing (outer) scale and
$L$ is the energy-containing (outer) scale and ![]() $Z^\pm$ are the two fields’ amplitudes at this scale, then
$Z^\pm$ are the two fields’ amplitudes at this scale, then
Thus, an initial imbalance in either direction will cause the (fractional) imbalance to get worse with time, until the weaker field has decayed away. In other words, cross-helicity ![]() $(Z^+)^2 - (Z^-)^2 \sim \mathrm {const}\cdot (Z^+ + Z^-)$ decays more slowly than energy, hence the increasing imbalance. The asymptotic state is an Elsasser state with
$(Z^+)^2 - (Z^-)^2 \sim \mathrm {const}\cdot (Z^+ + Z^-)$ decays more slowly than energy, hence the increasing imbalance. The asymptotic state is an Elsasser state with
Note that this simple model depends on assuming that ![]() $L$ is the same for both fields and that any alignment effects on the strength of the nonlinear interaction can be ignored, which is far from obvious and can be hard to sustain (e.g., Hossain et al. Reference Hossain, Gray, Pontius, Matthaeus and Oughton1995; Wan et al. Reference Wan, Oughton, Servidio and Matthaeus2012; Bandyopadhyay et al. Reference Bandyopadhyay, Matthaeus, Oughton and Wan2019).
$L$ is the same for both fields and that any alignment effects on the strength of the nonlinear interaction can be ignored, which is far from obvious and can be hard to sustain (e.g., Hossain et al. Reference Hossain, Gray, Pontius, Matthaeus and Oughton1995; Wan et al. Reference Wan, Oughton, Servidio and Matthaeus2012; Bandyopadhyay et al. Reference Bandyopadhyay, Matthaeus, Oughton and Wan2019).
The above scenario did, nevertheless, appear to be confirmed (very slowly in time) in the decaying RMHD simulation by Chen et al. (Reference Chen, Mallet, Yousef, Schekochihin and Horbury2011), initialised by first creating a statistically steady, forced, balanced turbulence and then switching off the forcing, so the breaking of the symmetry in favour of one of the fields arose from an initial fluctuation of the imbalance. In full-MHD simulations with a strong mean field, the same result had been found in a number of earlier papers (Oughton, Priest & Matthaeus Reference Oughton, Priest and Matthaeus1994; Maron & Goldreich Reference Maron and Goldreich2001; Cho et al. Reference Cho, Lazarian and Vishniac2002b), while in the absence of a mean field, it dates back to even earlier selective-decay literature (Montgomery et al. Reference Montgomery, Turner and Vahala1978, Reference Montgomery, Turner and Vahala1979; Matthaeus & Montgomery Reference Matthaeus and Montgomery1980; Ting et al. Reference Ting, Montgomery and Matthaeus1986; Stribling & Matthaeus Reference Stribling and Matthaeus1991; Hossain et al. Reference Hossain, Gray, Pontius, Matthaeus and Oughton1995).
Let me observe finally that, in the model (12.49), the energy fluxes
are in the same relationship with Elsasser energies as they are reported to be in forced imbalanced turbulence: see (9.1), (9.3) and § 9.4 (there is also some direct numerical support for this relationship in decaying MHD turbulence, going back to Verma et al. Reference Verma, Roberts, Goldstein, Ghosh and Stribling1996). This is perhaps another encouraging sign.
I do not have any further insights to offer about this regime, so I will stop here.
12.8. Inertial-range spectra of decaying MHD turbulence: numerical evidence
The philosophy articulated in the introduction to § 12 with regard to the inertial-range spectra does appear to be vindicated in the RMHD simulations of Chen et al. (Reference Chen, Mallet, Yousef, Schekochihin and Horbury2011), except the perpendicular spectrum was steeper than ![]() $k_{\perp }^{-3/2}$ (and closer to
$k_{\perp }^{-3/2}$ (and closer to ![]() $k_{\perp }^{-5/3}$) and the parallel one steeper than
$k_{\perp }^{-5/3}$) and the parallel one steeper than ![]() $k_{\parallel }^{-2}$ – this might actually be consistent with what one would expect for a system that moved gradually towards greater imbalance (see § 9.6).
$k_{\parallel }^{-2}$ – this might actually be consistent with what one would expect for a system that moved gradually towards greater imbalance (see § 9.6).
The evidence from the RMHD simulations by Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020) appears to point in the same direction: they report ![]() $k_{\perp }^{-3/2}$ spectra of both magnetic and kinetic energy, presumably of the same origin as those derived in § 6.
$k_{\perp }^{-3/2}$ spectra of both magnetic and kinetic energy, presumably of the same origin as those derived in § 6.
In the currently available decaying MHD simulations without a mean field, with or without helicity, there might not yet be sufficient resolution to tell what the asymptotic inertial-range spectra are (see, e.g., figure 28 and note particularly that there is no scale-by-scale equipartition between magnetic and kinetic energy at these resolutions) – or indeed whether they might be non-universal with respect to initial conditions (Lee et al. Reference Lee, Brachet, Pouquet, Mininni and Rosenberg2010), a somewhat disconcerting prospect. An oft-reported ‘non-universal’ spectrum is ![]() $k^{-2}$ (e.g., by Lee et al. Reference Lee, Brachet, Pouquet, Mininni and Rosenberg2010; Brandenburg et al. Reference Brandenburg, Kahniashvili and Tevzadze2015, Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019), which might actually be another signature of reconnection (rather than of the WT regime, as some of these authors suggest): Dallas & Alexakis (Reference Dallas and Alexakis2013a) and Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019, in 2D) interpret this spectrum geometrically as describing an ensemble of current sheets, which are step-like ‘discontinuities’ of the magnetic field (this is the same argument as I mooted for the residual energy in § 10.4; note that the spectrum of plasmoid chains would also be
$k^{-2}$ (e.g., by Lee et al. Reference Lee, Brachet, Pouquet, Mininni and Rosenberg2010; Brandenburg et al. Reference Brandenburg, Kahniashvili and Tevzadze2015, Reference Brandenburg, Kahniashvili, Mandal, Pol, Tevzadze and Vachaspati2019), which might actually be another signature of reconnection (rather than of the WT regime, as some of these authors suggest): Dallas & Alexakis (Reference Dallas and Alexakis2013a) and Zhou et al. (Reference Zhou, Bhat, Loureiro and Uzdensky2019, in 2D) interpret this spectrum geometrically as describing an ensemble of current sheets, which are step-like ‘discontinuities’ of the magnetic field (this is the same argument as I mooted for the residual energy in § 10.4; note that the spectrum of plasmoid chains would also be ![]() $k^{-2}$, as shown in Appendix D.6.2). According to Dallas & Alexakis (Reference Dallas and Alexakis2013b, Reference Dallas and Alexakis2014), however, this scaling gives way to a shallower
$k^{-2}$, as shown in Appendix D.6.2). According to Dallas & Alexakis (Reference Dallas and Alexakis2013b, Reference Dallas and Alexakis2014), however, this scaling gives way to a shallower ![]() $k^{-5/3}$ or
$k^{-5/3}$ or ![]() $k^{-3/2}$ slope at sufficiently small scales in simulations with sufficiently high resolution, as current sheets curl up and/or break up (see also Mininni, Pouquet & Montgomery Reference Mininni, Pouquet and Montgomery2006), so perhaps small-scale universality is safe after all and the current sheets are simply the effective energy-containing structures at which the ‘true’ inertial range starts (cf. § 7.4.3 and Appendix D.6.3).
$k^{-3/2}$ slope at sufficiently small scales in simulations with sufficiently high resolution, as current sheets curl up and/or break up (see also Mininni, Pouquet & Montgomery Reference Mininni, Pouquet and Montgomery2006), so perhaps small-scale universality is safe after all and the current sheets are simply the effective energy-containing structures at which the ‘true’ inertial range starts (cf. § 7.4.3 and Appendix D.6.3).
12.9. Summary
To sum up, there appear to be at least three qualitatively different regimes, or, rather, classes of regimes,Footnote 72 of decaying MHD turbulence:
(i) RMHD and MHD states with some initial imbalance tend towards enduring (i.e., decaying on the viscous/resistive time scale) pure Elsasser solutions, due to relatively slower decay of the cross-helicity compared to energy (§ 12.7).
(ii) RMHD, 2D MHD, and non-helical, zero-mean-field, 3D MHD turbulence starting in magnetically dominated and, therefore, balanced configurations, settle into a reconnection-controlled decay towards ever-larger-scale magnetic structures accompanied by flows whose kinetic energy is a time-independent fraction of the magnetic one (§§ 12.6, 12.2.1, and 12.4.2). This decay is ‘selective’, constrained by the conservation of certain invariants: anastrophy in 2D MHD (§ 12.1.1), the Hosking invariant (the Saffman helicity invariant) in 3D MHD (§ 12.4.2), and probably the Saffman cross-helicity invariant in RMHD (§ 12.6.1).
(iii) 3D MHD turbulence with no mean field but finite net helicity ends up in a decaying state dominated by an approximately force-free magnetic field. The decay is reconnection-controlled for magnetically dominated states (§ 12.2.2). It is constrained by the conservation of helicity (§ 12.1.2) and, if it starts in a state with comparable magnetic and kinetic energies, possibly also by the Saffman cross-helicity invariant (§ 12.4.3). In the latter case, the kinetic energy decays faster than magnetic, eventually pushing the system towards the magnetically dominated regime.
The different regimes are distinguished by different scaling laws for energies and energy-containing scales vs. time. In all cases, the energy-containing scale grows – and that is the result not just of larger structures decaying slower but also of some actual transfer of energy to larger scales, unlike in hydrodynamic turbulence (§ 12.5). In reconnection-controlled regimes, this transfer is achieved dynamically by coalescence of magnetic structures.
At sufficiently small scales, all these different types of decaying turbulence probably behave similarly to their forced counterparts, although it remains a challenging computational task to confirm this definitively (§ 12.8). Reconnection-controlled decaying MHD turbulence below the energy-containing scale is probably a case of (many instances of) reconnection-driven turbulence discussed in § 7.4.3.
13. MHD dynamo meets reconnection
As the field becomes more and more tangled, there will be places where the field is sharply reversed, and magnetic reconnection may set in, removing the sharpest kinks.
Kulsrud & Anderson (Reference Kulsrud and Anderson1992)
An interesting and distinct type of MHD turbulence about which I have so far said nothing except in the context of its decay (§ 12) is the case of no mean field. Starting with a steady-state, forced hydrodynamic turbulence and a dynamically weak, randomly tangled magnetic field, one observes exponential growth of the latter, a phenomenon known as small-scale dynamo (or fluctuation dynamo) – expected already by Batchelor (Reference Batchelor1950) and Biermann & Schlüter (Reference Biermann and Schlüter1951) and since confirmed numerically (Meneguzzi, Frisch & Pouquet Reference Meneguzzi, Frisch and Pouquet1981) and experimentally (Tzeferacos et al. Reference Tzeferacos, Rigby, Bott, Bell, Bingham, Casner, Cattaneo, Churazov, Emig and Fiuza2018; Bott et al. Reference Bott, Tzeferacos, Chen, Palmer, Rigby, Bell, Bingham, Birkel, Graziani and Froula2021b, Reference Bott, Chen, Tzeferacos, Palmer, Bell, Bingham, Birkel, Froula, Katz and Kunz2022). The system eventually saturates with magnetic energy comparable to kinetic, but not, it seems, necessarily equal to it scale by scale – what the final state is remains an unsolved problem, both numerically (due to lack of resolution) and theoretically (due to lack of theoreticians). Furthermore, it matters whether the turbulence possesses net helicity (injected into the flow by the forcing) and/or has a large-scale shear superimposed on it – if it does, small-scale dynamo is accompanied by a mean-field dynamo, leading to growth of a large-scale field (the large scale in question being generally larger than the outer scale of the turbulence). Saturated states of such dynamos are also poorly understood, for the same reasons.
Turbulent dynamos deserve a separate review – and they have recently received a superb one, by Rincon (Reference Rincon2019), to which I enthusiastically refer all interested public. This said, the ideas associated with the role of tearing in RMHD turbulence, reviewed in § 7, turn out to have some direct bearing on the ‘purest’ (homogeneous, non-helical, unsheared) small-scale dynamo problem. This is, therefore, a natural place for some discussion of it.
13.1. Old arguments about saturated dynamo at large  $\mathrm {Pm}$
$\mathrm {Pm}$
In regimes with ![]() $\mathrm {Pm}>1$,Footnote 73 small-scale dynamo can be understood as the process of a velocity field, restricted to scales above the viscous cutoff, randomly stretching and shearing the magnetic field, which is allowed to go to smaller scales, limited only by the Ohmic resistivity. Intuitively, it is not hard to see that embedding a tangled field line into an ‘eddy’ will lead to the field line being stretched and folded, resulting in a configuration featuring field reversals on ever smaller scales (figure 30). A combination of numerical evidence and theoretical arguments (see Schekochihin & Cowley Reference Schekochihin and Cowley2007, Rincon Reference Rincon2019, and references therein) confirms that this process does indeed lead to net amplification of magnetic energy, with that energy residing preferentially in ‘folds’ – magnetic fields that reverse direction across themselves on the resistive scale and remain approximately straight along themselves up to the scale of the velocity field. When the dynamo saturates, it does so in some poorly understood way involving these bundles of alternating fields back-reacting on the turbulent flow and arresting further amplification. Whereas in the kinematic-dynamo stage (i.e., when the field is dynamically weak), the spectrum of the magnetic energy certainly peaks at the resistive scale (Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b), what exactly happens in the saturated state is a matter of some debate. It is tempting to argue, with Biermann & Schlüter (Reference Biermann and Schlüter1951) and Kraichnan (Reference Kraichnan1965), that the system will sort itself out into a state where the magnetic energy is at the outer scale, while the smaller scales behave in exactly the same way as they would do in the presence of a strong mean field. Whether numerical evidence confirms this view is, at the resolutions achieved so far, in the eye of the beholder (Kida, Yanase & Mizushima Reference Kida, Yanase and Mizushima1991; Haugen, Brandenburg & Dobler Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004; Cho & Ryu Reference Cho and Ryu2009; Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a; Beresnyak Reference Beresnyak2012c; Teaca et al. Reference Teaca, Lalescu, Knaepen and Carati2011; Eyink et al. Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013; Porter, Jones & Ryu Reference Porter, Jones and Ryu2015; Grete et al. Reference Grete, O'Shea, Beckwith, Schmidt and Christlieb2017; Grete, O'Shea & Beckwith Reference Grete, O'Shea and Beckwith2021; McKay et al. Reference McKay, Berera and Ho2019; Bian & Aluie Reference Bian and Aluie2019; Brandenburg & Rempel Reference Brandenburg and Rempel2019; Seta et al. Reference Seta, Bushby, Shukurov and Wood2020; see figure 33b). The alternative possibility is that the magnetic energy stays at small scales – not quite as small as in the kinematic regime, but still determined by resistivity (Schekochihin et al. Reference Schekochihin, Cowley, Hammett, Maron and McWilliams2002, Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b; Maron, Cowley & McWilliams Reference Maron, Cowley and McWilliams2004). The claim is that the folded field structure persists in saturation, with the folds elongating to the outer scale (
$\mathrm {Pm}>1$,Footnote 73 small-scale dynamo can be understood as the process of a velocity field, restricted to scales above the viscous cutoff, randomly stretching and shearing the magnetic field, which is allowed to go to smaller scales, limited only by the Ohmic resistivity. Intuitively, it is not hard to see that embedding a tangled field line into an ‘eddy’ will lead to the field line being stretched and folded, resulting in a configuration featuring field reversals on ever smaller scales (figure 30). A combination of numerical evidence and theoretical arguments (see Schekochihin & Cowley Reference Schekochihin and Cowley2007, Rincon Reference Rincon2019, and references therein) confirms that this process does indeed lead to net amplification of magnetic energy, with that energy residing preferentially in ‘folds’ – magnetic fields that reverse direction across themselves on the resistive scale and remain approximately straight along themselves up to the scale of the velocity field. When the dynamo saturates, it does so in some poorly understood way involving these bundles of alternating fields back-reacting on the turbulent flow and arresting further amplification. Whereas in the kinematic-dynamo stage (i.e., when the field is dynamically weak), the spectrum of the magnetic energy certainly peaks at the resistive scale (Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b), what exactly happens in the saturated state is a matter of some debate. It is tempting to argue, with Biermann & Schlüter (Reference Biermann and Schlüter1951) and Kraichnan (Reference Kraichnan1965), that the system will sort itself out into a state where the magnetic energy is at the outer scale, while the smaller scales behave in exactly the same way as they would do in the presence of a strong mean field. Whether numerical evidence confirms this view is, at the resolutions achieved so far, in the eye of the beholder (Kida, Yanase & Mizushima Reference Kida, Yanase and Mizushima1991; Haugen, Brandenburg & Dobler Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004; Cho & Ryu Reference Cho and Ryu2009; Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a; Beresnyak Reference Beresnyak2012c; Teaca et al. Reference Teaca, Lalescu, Knaepen and Carati2011; Eyink et al. Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013; Porter, Jones & Ryu Reference Porter, Jones and Ryu2015; Grete et al. Reference Grete, O'Shea, Beckwith, Schmidt and Christlieb2017; Grete, O'Shea & Beckwith Reference Grete, O'Shea and Beckwith2021; McKay et al. Reference McKay, Berera and Ho2019; Bian & Aluie Reference Bian and Aluie2019; Brandenburg & Rempel Reference Brandenburg and Rempel2019; Seta et al. Reference Seta, Bushby, Shukurov and Wood2020; see figure 33b). The alternative possibility is that the magnetic energy stays at small scales – not quite as small as in the kinematic regime, but still determined by resistivity (Schekochihin et al. Reference Schekochihin, Cowley, Hammett, Maron and McWilliams2002, Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b; Maron, Cowley & McWilliams Reference Maron, Cowley and McWilliams2004). The claim is that the folded field structure persists in saturation, with the folds elongating to the outer scale (![]() $L$) of the turbulence and direction reversals within folds occurring on the scale
$L$) of the turbulence and direction reversals within folds occurring on the scale ![]() $\lambda _{\eta }\sim L\,\mathrm {Rm}^{-1/2}$, where the stretching rate (
$\lambda _{\eta }\sim L\,\mathrm {Rm}^{-1/2}$, where the stretching rate (![]() $\sim \delta u_L/L$) balances the Ohmic-diffusion rate (
$\sim \delta u_L/L$) balances the Ohmic-diffusion rate (![]() $\sim \eta /\lambda _{\eta }^2$).
$\sim \eta /\lambda _{\eta }^2$).

Figure 30. Stretching/shearing a field line produces direction reversals (cartoon from Schekochihin & Cowley Reference Schekochihin and Cowley2007).
Despite being associated with the latter point of view, I am not going to defend it here in its original form because of certain little known but consequential numerical developments, described in § 13.2, that occurred after that debate had its heyday. Instead, drawing on the ideas of § 7, I will propose, in §§ 13.3 and 13.4, an amended view of the saturated state of turbulent dynamo, in which reconnection and MHD turbulence will again meet and collaborate.
13.2. Numerical evidence: reconnection strikes again
The existence of turbulent dynamo was definitively established by Meneguzzi et al. (Reference Meneguzzi, Frisch and Pouquet1981) in what was then a ‘hero’ ![]() $64^3$ MHD simulation – one of those bona fide numerical discoveries that make computer simulations worthwhile. Twenty years later, when the debate about the nature of the saturated dynamo state focused on interpreting newly accessible improved numerical evidence (Kinney et al. Reference Kinney, Chandran, Cowley and McWilliams2000; Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b; Maron et al. Reference Maron, Cowley and McWilliams2004; Haugen et al. Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004), everyone was staring at not-very-conclusive magnetic spectra with some pronounced excess of the magnetic energy over kinetic at small scales, and at visualisations of magnetic fields organised in folds (especially at large
$64^3$ MHD simulation – one of those bona fide numerical discoveries that make computer simulations worthwhile. Twenty years later, when the debate about the nature of the saturated dynamo state focused on interpreting newly accessible improved numerical evidence (Kinney et al. Reference Kinney, Chandran, Cowley and McWilliams2000; Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b; Maron et al. Reference Maron, Cowley and McWilliams2004; Haugen et al. Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004), everyone was staring at not-very-conclusive magnetic spectra with some pronounced excess of the magnetic energy over kinetic at small scales, and at visualisations of magnetic fields organised in folds (especially at large ![]() $\mathrm {Pm}$). One could be a believer in universality and think of this as a non-asymptotic state that would, at infinite resolution, turn into the usual Kolmogorov-style turbulence spectrum, with magnetic energy shifting to the outer scales (Haugen et al. Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004; Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a; Beresnyak Reference Beresnyak2012c) – or one could rely on a different kind of physical intuition and argue that there was no obvious physical mechanism for unwrapping fields folded at the resistive scale (that was my view).
$\mathrm {Pm}$). One could be a believer in universality and think of this as a non-asymptotic state that would, at infinite resolution, turn into the usual Kolmogorov-style turbulence spectrum, with magnetic energy shifting to the outer scales (Haugen et al. Reference Haugen, Brandenburg and Dobler2003, Reference Haugen, Brandenburg and Dobler2004; Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a; Beresnyak Reference Beresnyak2012c) – or one could rely on a different kind of physical intuition and argue that there was no obvious physical mechanism for unwrapping fields folded at the resistive scale (that was my view).
In later, sadly unfinished, work, Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008) discovered, however, that, in simulations with moderate ![]() $\mathrm {Pm}\ge 1$ and large
$\mathrm {Pm}\ge 1$ and large ![]() $\mathrm {Re}$ (the former being the only affordable possibility compatible with the latter), magnetic folds in the nonlinear regime became current sheets, with very clear inflow–outflow patterns around the field reversals (figure 31). One might say that this should have been obvious from the start, although perhaps less so in the case of
$\mathrm {Re}$ (the former being the only affordable possibility compatible with the latter), magnetic folds in the nonlinear regime became current sheets, with very clear inflow–outflow patterns around the field reversals (figure 31). One might say that this should have been obvious from the start, although perhaps less so in the case of ![]() $\mathrm {Pm}\gg 1$ (see § 13.3.3). We also found that the folds became corrugated, and plasmoid-like structures (probably flux ropes) formed, with (perhaps) approximately circularised cross-sections. Larger simulations by Beresnyak (Reference Beresnyak2012b), also unpublished (except for some bits in Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and Beresnyak Reference Beresnyak2012c), revealed the same feature, with the numerical box now teaming with small plasmoid-like structures and rippled folds (figure 32), a result confirmed at even higher resolutions by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022). Thus, while the folds could not perhaps be literally unwrapped, they did turn out to be prone to breaking up and seeding populations of smaller structures.Footnote 74
$\mathrm {Pm}\gg 1$ (see § 13.3.3). We also found that the folds became corrugated, and plasmoid-like structures (probably flux ropes) formed, with (perhaps) approximately circularised cross-sections. Larger simulations by Beresnyak (Reference Beresnyak2012b), also unpublished (except for some bits in Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and Beresnyak Reference Beresnyak2012c), revealed the same feature, with the numerical box now teaming with small plasmoid-like structures and rippled folds (figure 32), a result confirmed at even higher resolutions by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022). Thus, while the folds could not perhaps be literally unwrapped, they did turn out to be prone to breaking up and seeding populations of smaller structures.Footnote 74

Figure 31. From unpublished work by Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008): a ![]() $512^3$ incompressible-MHD simulation of saturated fluctuation dynamo,
$512^3$ incompressible-MHD simulation of saturated fluctuation dynamo, ![]() $\mathrm {Pm}=1$,
$\mathrm {Pm}=1$, ![]() $\mathrm {Re}=1360$ (defined
$\mathrm {Re}=1360$ (defined ![]() $=u_{\mathrm {rms}}/\nu k_0$, where
$=u_{\mathrm {rms}}/\nu k_0$, where ![]() $k_0=2\pi$ is the forcing wavenumber, corresponding to the box size; this is the same numerical set up as in Iskakov et al. Reference Iskakov, Schekochihin, Cowley, McWilliams and Proctor2007 and Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007). These are 2D cuts from instantaneous snapshots of absolute values of (a) velocity, (b) magnetic field. Panel (c) is a cut out from these snapshots, zooming in on the horizontal fold just down towards the left from the centre of the snapshot. Stream lines are in black and field lines are in red. A reconnecting-sheet structure, with field reversal, inflows and outflows is manifest. Very pretty 3D visualisations of this kind of reconnecting structure extracted from an MHD turbulence simulation can be found in Lalescu et al. (Reference Lalescu, Shi, Eyink, Drivas, Vishniac and Lazarian2015).
$k_0=2\pi$ is the forcing wavenumber, corresponding to the box size; this is the same numerical set up as in Iskakov et al. Reference Iskakov, Schekochihin, Cowley, McWilliams and Proctor2007 and Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007). These are 2D cuts from instantaneous snapshots of absolute values of (a) velocity, (b) magnetic field. Panel (c) is a cut out from these snapshots, zooming in on the horizontal fold just down towards the left from the centre of the snapshot. Stream lines are in black and field lines are in red. A reconnecting-sheet structure, with field reversal, inflows and outflows is manifest. Very pretty 3D visualisations of this kind of reconnecting structure extracted from an MHD turbulence simulation can be found in Lalescu et al. (Reference Lalescu, Shi, Eyink, Drivas, Vishniac and Lazarian2015).

Figure 32. From unpublished work by Beresnyak (Reference Beresnyak2012b) (reproduced with the kind permission of the author): snapshot of the absolute value of magnetic field in a ![]() $\mathrm {Pm}=10$ and
$\mathrm {Pm}=10$ and ![]() $\mathrm {Re}\approx 500$ simulation at
$\mathrm {Re}\approx 500$ simulation at ![]() $1024^3$ (the same numerical set up as in Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and Beresnyak Reference Beresnyak2012c; note that Beresnyak defines his
$1024^3$ (the same numerical set up as in Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and Beresnyak Reference Beresnyak2012c; note that Beresnyak defines his ![]() $\mathrm {Re}$ in terms of the ‘true’ integral scale of the flow calculated from its spectrum). Plasmoids/fold corrugations galore. In his other simulations within this sequence, there are even more plasmoid-like-looking features at
$\mathrm {Re}$ in terms of the ‘true’ integral scale of the flow calculated from its spectrum). Plasmoids/fold corrugations galore. In his other simulations within this sequence, there are even more plasmoid-like-looking features at ![]() $\mathrm {Pm}=1$ and
$\mathrm {Pm}=1$ and ![]() $\mathrm {Re}\approx 6000$, with some sign of them breaking up into even smaller structures (cf. § 13.3.3). In contrast, they start disappearing at
$\mathrm {Re}\approx 6000$, with some sign of them breaking up into even smaller structures (cf. § 13.3.3). In contrast, they start disappearing at ![]() $\mathrm {Pm}=10^2$ and
$\mathrm {Pm}=10^2$ and ![]() $\mathrm {Re}\approx 80$ and are gone completely in the ‘Stokes’regime
$\mathrm {Re}\approx 80$ and are gone completely in the ‘Stokes’regime ![]() $\mathrm {Pm}=10^4$ and
$\mathrm {Pm}=10^4$ and ![]() $\mathrm {Re}\approx 2$. Very similar results have been found by Galishnikova, Kunz & Schekochihin (Reference Galishnikova, Kunz and Schekochihin2022).
$\mathrm {Re}\approx 2$. Very similar results have been found by Galishnikova, Kunz & Schekochihin (Reference Galishnikova, Kunz and Schekochihin2022).
There is little definitive analysis of all this available in print. There is, however, an intriguing finding by Brandenburg (Reference Brandenburg2014), who analysed his own simulations and those of Sahoo et al. (Reference Sahoo, Perlekar and Pandit2011) and discovered that the ratio of energy dissipated resistively to that dissipated viscously decreased at larger ![]() $\mathrm {Pm}$ (Beresnyak Reference Beresnyak2012b also had this result, and Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022 confirm it; McKay et al. Reference McKay, Berera and Ho2019, however, raise a degree of doubt as to whether it will survive at larger
$\mathrm {Pm}$ (Beresnyak Reference Beresnyak2012b also had this result, and Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022 confirm it; McKay et al. Reference McKay, Berera and Ho2019, however, raise a degree of doubt as to whether it will survive at larger ![]() $\mathrm {Rm}$). One might plausibly argue that something like this could happen if kinetic energy, first converted into magnetic one as fields were amplified and folded by large-scale turbulent flows, were then to be recovered from magnetic energy at smaller scales as fluid motions were generated by reconnection and instabilities (presumably, tearing instabilities) in the folds. Brandenburg & Rempel (Reference Brandenburg and Rempel2019), while they do not engage with the notion of reconnecting folds, do confirm explicitly that, in larger-
$\mathrm {Rm}$). One might plausibly argue that something like this could happen if kinetic energy, first converted into magnetic one as fields were amplified and folded by large-scale turbulent flows, were then to be recovered from magnetic energy at smaller scales as fluid motions were generated by reconnection and instabilities (presumably, tearing instabilities) in the folds. Brandenburg & Rempel (Reference Brandenburg and Rempel2019), while they do not engage with the notion of reconnecting folds, do confirm explicitly that, in larger-![]() $\mathrm {Pm}$ simulations, there is increasing net transfer of magnetic to kinetic energy at small scales, with kinetic energy's viscous thermalisation increasingly dominating the overall dissipation rate (this was checked, and checked out, in the simulations by Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
$\mathrm {Pm}$ simulations, there is increasing net transfer of magnetic to kinetic energy at small scales, with kinetic energy's viscous thermalisation increasingly dominating the overall dissipation rate (this was checked, and checked out, in the simulations by Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
A signature of this behaviour is discernible if one examines the magnetic- and, especially, kinetic-energy spectra in saturated dynamo simulations at relatively high resolutions (![]() $512^3$ and up), without attempting to see what one might want to see, e.g., scale-by-scale equipartition or
$512^3$ and up), without attempting to see what one might want to see, e.g., scale-by-scale equipartition or ![]() $k^{-5/3}$. Figure 33(a), taken from the unpublished simulations by Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008), shows that the kinetic-energy spectrum steepens at large scales compared to the hydrodynamic case (the empirical slope is
$k^{-5/3}$. Figure 33(a), taken from the unpublished simulations by Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008), shows that the kinetic-energy spectrum steepens at large scales compared to the hydrodynamic case (the empirical slope is ![]() $k^{-7/3}$; see Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b and St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020), but picks up around the same wavenumber where the magnetic-energy spectrum has its peak and becomes shallower than Kolmogorov – Grete et al. (Reference Grete, O'Shea and Beckwith2021) find
$k^{-7/3}$; see Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b and St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020), but picks up around the same wavenumber where the magnetic-energy spectrum has its peak and becomes shallower than Kolmogorov – Grete et al. (Reference Grete, O'Shea and Beckwith2021) find ![]() $k^{-4/3}$ to be a good fit, both in their simulations and, in retrospect, in many previous ones (figure 33b). By analysing energy transfers, they attribute this to significant transfer of energy from magnetic to kinetic, by means of the tension force – this is analogous to the result of Brandenburg & Rempel (Reference Brandenburg and Rempel2019) and again entirely consistent with (although does not amount to a definitive confirmation of) the idea that reconnection in the folds drives small-scale motions, which come to dominate the kinetic-energy spectrum at those scales. This is perhaps reinforced by their (Grete et al. Reference Grete, O'Shea and Beckwith2021) observation (in disagreement with Bian & Aluie Reference Bian and Aluie2019) that the energy cascade at small scales is almost entirely controlled by magnetic forces, rather than by hydrodynamic advection. The paper by Grete et al. (Reference Grete, O'Shea and Beckwith2021) appears to be the first one for well over a decade that, having measured different kinetic- and magnetic-energy spectra, dares to consider the possibility that this might mean something physical, rather than merely insufficient resolution for expected asymptotic recovery of
$k^{-4/3}$ to be a good fit, both in their simulations and, in retrospect, in many previous ones (figure 33b). By analysing energy transfers, they attribute this to significant transfer of energy from magnetic to kinetic, by means of the tension force – this is analogous to the result of Brandenburg & Rempel (Reference Brandenburg and Rempel2019) and again entirely consistent with (although does not amount to a definitive confirmation of) the idea that reconnection in the folds drives small-scale motions, which come to dominate the kinetic-energy spectrum at those scales. This is perhaps reinforced by their (Grete et al. Reference Grete, O'Shea and Beckwith2021) observation (in disagreement with Bian & Aluie Reference Bian and Aluie2019) that the energy cascade at small scales is almost entirely controlled by magnetic forces, rather than by hydrodynamic advection. The paper by Grete et al. (Reference Grete, O'Shea and Beckwith2021) appears to be the first one for well over a decade that, having measured different kinetic- and magnetic-energy spectra, dares to consider the possibility that this might mean something physical, rather than merely insufficient resolution for expected asymptotic recovery of ![]() $k^{-5/3}$ or
$k^{-5/3}$ or ![]() $k^{-3/2}$.
$k^{-3/2}$.

Figure 33. (a) Spectra of saturated MHD dynamo: kinetic-energy (red, compensated by ![]() $k^{5/3}$) and magnetic-energy (blue) spectra from a series of incompressible-MHD simulations with
$k^{5/3}$) and magnetic-energy (blue) spectra from a series of incompressible-MHD simulations with ![]() $\mathrm {Pm}=10$ and increasing
$\mathrm {Pm}=10$ and increasing ![]() $\mathrm {Re}$ by Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008). The numerical set up is the same as in Schekochihin et al. (Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007); the resolution is
$\mathrm {Re}$ by Iskakov & Schekochihin (Reference Iskakov and Schekochihin2008). The numerical set up is the same as in Schekochihin et al. (Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007); the resolution is ![]() $512^3$ (so the highest-
$512^3$ (so the highest-![]() $\mathrm {Re}$ run may be numerically suspect). (b) A summary plot from Grete et al. (Reference Grete, O'Shea and Beckwith2021) (© AAS, reproduced with permission) of their and several other numerical studies, viz., from top to bottom, Bian & Aluie (Reference Bian and Aluie2019); Grete et al. (Reference Grete, O'Shea and Beckwith2021); Porter et al. (Reference Porter, Jones and Ryu2015); Eyink et al. (Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013); Haugen et al. (Reference Haugen, Brandenburg and Dobler2004). The kinetic-energy (solid lines) and magnetic-energy (dot-dashed lines) spectra are all compensated by
$\mathrm {Re}$ run may be numerically suspect). (b) A summary plot from Grete et al. (Reference Grete, O'Shea and Beckwith2021) (© AAS, reproduced with permission) of their and several other numerical studies, viz., from top to bottom, Bian & Aluie (Reference Bian and Aluie2019); Grete et al. (Reference Grete, O'Shea and Beckwith2021); Porter et al. (Reference Porter, Jones and Ryu2015); Eyink et al. (Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013); Haugen et al. (Reference Haugen, Brandenburg and Dobler2004). The kinetic-energy (solid lines) and magnetic-energy (dot-dashed lines) spectra are all compensated by ![]() $k^{4/3}$ to highlight the shallow kinetic-energy spectrum at small scales. Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022) report very similar spectra and find also the magnetic spectrum steepening at even smaller scales, perhaps vindicating the prediction of § 13.3.3.
$k^{4/3}$ to highlight the shallow kinetic-energy spectrum at small scales. Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022) report very similar spectra and find also the magnetic spectrum steepening at even smaller scales, perhaps vindicating the prediction of § 13.3.3.
Thus, reconnection appears to have caught up with dynamo, just as it did with Alfvénic turbulence in § 7, the general principle at work in both cases being that while large-scale motions push magnetic fields into small-scale, direction-reversing configurations, resistive effects invariably manage to break those up (provided ![]() $\mathrm {Re}\gg 1$; see below).
$\mathrm {Re}\gg 1$; see below).
13.3. Towards a new theory of reconnection-limited dynamo
13.3.1. Kinematic dynamo and onset of tearing
Consider first a weak, tangled magnetic field being stretched by fluid motions whose scale is ![]() $\ell$ (why I call it
$\ell$ (why I call it ![]() $\ell$ rather than
$\ell$ rather than ![]() $\lambda$ is about to become obvious). Let us imagine that these fluid motions are part of vanilla Kolmogorov turbulence, described, inevitably, by (2.2):
$\lambda$ is about to become obvious). Let us imagine that these fluid motions are part of vanilla Kolmogorov turbulence, described, inevitably, by (2.2):
Balancing the associated stretching rate with the Ohmic-diffusion rate gives one the resistive scale:
 \begin{align} \tau_{\mathrm{nl}}^{{-}1} \sim \frac{\delta u_\ell}{\ell} \sim \frac{\varepsilon^{1/3}}{\ell^{2/3}} \sim\tau_{\eta}^{{-}1}\sim\frac{\eta}{\lambda_{\eta}^2}\quad\Rightarrow\quad \lambda_{\eta}(\ell)\sim(\eta\tau_{\mathrm{nl}})^{1/2} \sim \ell\, \mathrm{Rm}_\ell^{{-}1/2},\quad \mathrm{Rm}_\ell = \frac{\delta u_\ell \ell}{\eta}. \end{align}
\begin{align} \tau_{\mathrm{nl}}^{{-}1} \sim \frac{\delta u_\ell}{\ell} \sim \frac{\varepsilon^{1/3}}{\ell^{2/3}} \sim\tau_{\eta}^{{-}1}\sim\frac{\eta}{\lambda_{\eta}^2}\quad\Rightarrow\quad \lambda_{\eta}(\ell)\sim(\eta\tau_{\mathrm{nl}})^{1/2} \sim \ell\, \mathrm{Rm}_\ell^{{-}1/2},\quad \mathrm{Rm}_\ell = \frac{\delta u_\ell \ell}{\eta}. \end{align}
The scale ![]() $\lambda _{\eta }(\ell )$ is the reversal scale of the magnetic field generated by the dynamo of the eddies of size
$\lambda _{\eta }(\ell )$ is the reversal scale of the magnetic field generated by the dynamo of the eddies of size ![]() $\ell$; this field's typical coherence scale along itself will be
$\ell$; this field's typical coherence scale along itself will be ![]() $\ell$.
$\ell$.
Imagine now a general configuration in which magnetic field ![]() $B_\lambda$ (as usual, in velocity units) reverses direction on some scale
$B_\lambda$ (as usual, in velocity units) reverses direction on some scale ![]() $\lambda$, not necessarily equal to
$\lambda$, not necessarily equal to ![]() $\lambda _{\eta }$. It will be subject to tearing at the rate (7.1), but with
$\lambda _{\eta }$. It will be subject to tearing at the rate (7.1), but with ![]() $v_{\mathrm {A}y}$ replaced by
$v_{\mathrm {A}y}$ replaced by ![]() $B_\lambda$:
$B_\lambda$:
When ![]() $B_\lambda$ is infinitesimally small, as it would be in the kinematic stage of the dynamo, the tearing rate is small,
$B_\lambda$ is infinitesimally small, as it would be in the kinematic stage of the dynamo, the tearing rate is small, ![]() $\gamma \ll \tau _{\eta }^{-1}$. It will become comparable to the resistive-diffusion rate at
$\gamma \ll \tau _{\eta }^{-1}$. It will become comparable to the resistive-diffusion rate at ![]() $\lambda =\lambda _{\eta }$ when the fields reversing at this scale grow to be at least
$\lambda =\lambda _{\eta }$ when the fields reversing at this scale grow to be at least
Here ![]() $\widetilde {\mathrm {Re}}_\ell$ is equal to the usual Reynolds number
$\widetilde {\mathrm {Re}}_\ell$ is equal to the usual Reynolds number ![]() $\mathrm {Re}_\ell$ when
$\mathrm {Re}_\ell$ when ![]() $\mathrm {Pm}\gtrsim 1$ and to the magnetic Reynolds number
$\mathrm {Pm}\gtrsim 1$ and to the magnetic Reynolds number ![]() $\mathrm {Rm}_\ell$ when
$\mathrm {Rm}_\ell$ when ![]() $\mathrm {Pm}\ll 1$ (cf. (6.24)). In the former case, since the stretching rate
$\mathrm {Pm}\ll 1$ (cf. (6.24)). In the former case, since the stretching rate ![]() $\tau _{\mathrm {nl}}^{-1}$ at the viscous scale
$\tau _{\mathrm {nl}}^{-1}$ at the viscous scale ![]() $\ell \sim \ell _{\nu } = \varepsilon ^{-1/4}\nu ^{3/4}$ is the largest, it is the viscous-scale eddies that will play the dominant role in amplifying an infinitesimally small magnetic field, but the dynamo will go nonlinear as soon as the field's energy becomes comparable to the energy of the viscous-scale motions,
$\ell \sim \ell _{\nu } = \varepsilon ^{-1/4}\nu ^{3/4}$ is the largest, it is the viscous-scale eddies that will play the dominant role in amplifying an infinitesimally small magnetic field, but the dynamo will go nonlinear as soon as the field's energy becomes comparable to the energy of the viscous-scale motions, ![]() $B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$. Since, by definition of
$B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$. Since, by definition of ![]() $\ell _{\nu }$,
$\ell _{\nu }$, ![]() $\mathrm {Re}_{\ell _{\nu }}\sim 1$, the estimate (13.4) also turns into
$\mathrm {Re}_{\ell _{\nu }}\sim 1$, the estimate (13.4) also turns into ![]() $B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$, i.e., tearing in the folds will start outpacing Ohmic diffusion at exactly the same moment as the nonlinearity kicks in (this is perhaps obvious because tearing needs Lorentz force: see Appendix D.1). Thus, a nonlinear dynamo is also a reconnecting dynamo, which I shall call ‘tearing-limited’.
$B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$, i.e., tearing in the folds will start outpacing Ohmic diffusion at exactly the same moment as the nonlinearity kicks in (this is perhaps obvious because tearing needs Lorentz force: see Appendix D.1). Thus, a nonlinear dynamo is also a reconnecting dynamo, which I shall call ‘tearing-limited’.
In the limit of ![]() $\mathrm {Pm}\ll 1$, the fastest eddies capable of field amplification are at the resistive scale,
$\mathrm {Pm}\ll 1$, the fastest eddies capable of field amplification are at the resistive scale, ![]() $\ell \sim \lambda _{\eta }$ (e.g., Boldyrev & Cattaneo Reference Boldyrev and Cattaneo2004). Since
$\ell \sim \lambda _{\eta }$ (e.g., Boldyrev & Cattaneo Reference Boldyrev and Cattaneo2004). Since ![]() $\widetilde {\mathrm {Re}}_{\lambda _{\eta }}\sim \mathrm {Rm}_{\lambda _{\eta }}\sim 1$, the estimate (13.4) becomes
$\widetilde {\mathrm {Re}}_{\lambda _{\eta }}\sim \mathrm {Rm}_{\lambda _{\eta }}\sim 1$, the estimate (13.4) becomes ![]() $B_{\lambda _{\eta }}\sim \delta u_{\lambda _{\eta }}$, so it again tells us that the nonlinearity and tearing become important at the same time. Admittedly, there is no longer a scale separation between
$B_{\lambda _{\eta }}\sim \delta u_{\lambda _{\eta }}$, so it again tells us that the nonlinearity and tearing become important at the same time. Admittedly, there is no longer a scale separation between ![]() $\ell$ and
$\ell$ and ![]() $\lambda _{\eta }$ in this situation, so the magnetic field is not, strictly speaking, ‘folded’ (this is quite obvious from the snapshots of growing fields in Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007), although one might still speculate that tearing is possible across generic
$\lambda _{\eta }$ in this situation, so the magnetic field is not, strictly speaking, ‘folded’ (this is quite obvious from the snapshots of growing fields in Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007), although one might still speculate that tearing is possible across generic ![]() $X$-point configurations. I shall keep my discussion general, but it might be easier for a doubtful reader just to think of large
$X$-point configurations. I shall keep my discussion general, but it might be easier for a doubtful reader just to think of large ![]() $\mathrm {Pm}$ in all cases.
$\mathrm {Pm}$ in all cases.
13.3.2. Self-similar dynamo
It has been argued by Schekochihin et al. (Reference Schekochihin, Cowley, Hammett, Maron and McWilliams2002, Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b) and Maron et al. (Reference Maron, Cowley and McWilliams2004) (with later variants by Beresnyak Reference Beresnyak2012c and Xu & Lazarian Reference Xu and Lazarian2016) and numerically confirmed in a conclusive fashion by Cho et al. (Reference Cho, Vishniac, Beresnyak, Lazarian and Ryu2009) and Beresnyak (Reference Beresnyak2012c) that, once the dynamo goes nonlinear, the field continues to be amplified, but by ever larger-scale motions that are, at a given time, just as energetic as the field is at that time.Footnote 75 That is, the scale ![]() $\ell (t)$ of the motions amplifying the field at time
$\ell (t)$ of the motions amplifying the field at time ![]() $t$ is set by the condition
$t$ is set by the condition
This leads, neatly, to a self-similar amplification regime:
After a few outer-scale eddy-turnover times, ![]() $t\sim L/\delta u_L$, the field's energy becomes comparable to that of the flow,
$t\sim L/\delta u_L$, the field's energy becomes comparable to that of the flow, ![]() $B_\lambda \sim \delta u_L$, and the dynamo saturates. At any time during the self-similar growth, the cascade below
$B_\lambda \sim \delta u_L$, and the dynamo saturates. At any time during the self-similar growth, the cascade below ![]() $\ell$ presumably looks just like the cascade in the saturated state, whereas above
$\ell$ presumably looks just like the cascade in the saturated state, whereas above ![]() $\ell$, the turbulence is still hydrodynamic.
$\ell$, the turbulence is still hydrodynamic.
13.3.3. Tearing-limited dynamo: universality regained
Schekochihin et al. (Reference Schekochihin, Cowley, Hammett, Maron and McWilliams2002, Reference Schekochihin, Cowley, Taylor, Maron and McWilliams2004b) calculated the field-reversal scale ![]() $\lambda$ in the self-similar and saturated dynamo regimes by balancing
$\lambda$ in the self-similar and saturated dynamo regimes by balancing ![]() $\delta u_\ell /\ell$ with the Ohmic-dissipation rate
$\delta u_\ell /\ell$ with the Ohmic-dissipation rate ![]() $\eta /\lambda ^2$. I now know, thanks to the argument in § 13.3.1 (obvious in retrospect!), that the folds generated by this process will in fact tear faster than they diffuse. So let me therefore balance the tearing rate (13.3) with
$\eta /\lambda ^2$. I now know, thanks to the argument in § 13.3.1 (obvious in retrospect!), that the folds generated by this process will in fact tear faster than they diffuse. So let me therefore balance the tearing rate (13.3) with ![]() $\delta u_\ell /\ell$ and obtain a scale familiar from the ‘ideal-tearing’condition (D57) (Pucci & Velli Reference Pucci and Velli2014; Tenerani et al. Reference Tenerani, Rappazzo, Velli and Pucci2015a):
$\delta u_\ell /\ell$ and obtain a scale familiar from the ‘ideal-tearing’condition (D57) (Pucci & Velli Reference Pucci and Velli2014; Tenerani et al. Reference Tenerani, Rappazzo, Velli and Pucci2015a):
In order for tearing to supersede Ohmic diffusion, we must haveFootnote 76
where ![]() $\lambda _{\eta }(\ell )$ was taken from (13.2). Note that
$\lambda _{\eta }(\ell )$ was taken from (13.2). Note that ![]() $\lambda \ll \ell$ always, except, for low
$\lambda \ll \ell$ always, except, for low ![]() $\mathrm {Pm}$, at the start of the self-similar regime, when
$\mathrm {Pm}$, at the start of the self-similar regime, when ![]() $\mathrm {Rm}_\ell \sim 1$ (this seems to suggest that even a low-
$\mathrm {Rm}_\ell \sim 1$ (this seems to suggest that even a low-![]() $\mathrm {Pm}$ dynamo may form reconnecting folds in the nonlinear regime).
$\mathrm {Pm}$ dynamo may form reconnecting folds in the nonlinear regime).
Let us imagine for now that that the self-similar evolution (13.6) has run its course and the dynamo has saturated in a state where the only motions that are responsible for the (re)generation of the folds are on the outer scale, viz., ![]() $\ell \sim L$, while the motions below this scale no longer affect the magnetic field (I will experiment with relaxing this assumption in § 13.4.1). The reversal scale of the folds is then set by (13.7) with
$\ell \sim L$, while the motions below this scale no longer affect the magnetic field (I will experiment with relaxing this assumption in § 13.4.1). The reversal scale of the folds is then set by (13.7) with ![]() $\ell \sim L$. I shall call this scale
$\ell \sim L$. I shall call this scale
Consider a fold of length ![]() $L$ and width
$L$ and width ![]() $\lambda _{\mathrm {R}}$. Its tearing will produce islands whose number can be inferred from (7.4):
$\lambda _{\mathrm {R}}$. Its tearing will produce islands whose number can be inferred from (7.4):
Just as I did at the end of § 7.1, I can argue here that these islands will grow, perhaps circularise, and turn into plasmoids (flux ropes) of transverse size ![]() $\lambda _{\mathrm {R}}$, while their mother fold is destroyed. Similarly to § 7.2, I can entertain the possibility that they are the outer-scale structures of a new turbulent cascade, seeded by the tearing of the fold at the scale
$\lambda _{\mathrm {R}}$, while their mother fold is destroyed. Similarly to § 7.2, I can entertain the possibility that they are the outer-scale structures of a new turbulent cascade, seeded by the tearing of the fold at the scale ![]() $\lambda _{\mathrm {R}}$. At scales below
$\lambda _{\mathrm {R}}$. At scales below ![]() $\lambda _{\mathrm {R}}$, this new cascade is of the usual RMHD kind considered in §§ 5–7 – the mean field now is
$\lambda _{\mathrm {R}}$, this new cascade is of the usual RMHD kind considered in §§ 5–7 – the mean field now is ![]() $B_{\lambda _{\mathrm {R}}}$, assuming that fields that make up the folds are unlikely to be exactly anti-parallel and so there is some component of the folded field, generally of the same order as its reversing component, pointing in the direction perpendicular both to the latter and to the direction of reversal.Footnote 77
$B_{\lambda _{\mathrm {R}}}$, assuming that fields that make up the folds are unlikely to be exactly anti-parallel and so there is some component of the folded field, generally of the same order as its reversing component, pointing in the direction perpendicular both to the latter and to the direction of reversal.Footnote 77
Let the flux rope have a circulation velocity ![]() $\delta u_{\lambda _{\mathrm {R}}}$ and a perturbed field
$\delta u_{\lambda _{\mathrm {R}}}$ and a perturbed field ![]() $\delta b_{\lambda _{\mathrm {R}}}\sim \delta u_{\lambda _{\mathrm {R}}}$. One can estimate these quantities by the same logic as led to (7.10): if this new cascade is to carry (a finite fraction of) the same energy flux as produced the fold,Footnote 78 then
$\delta b_{\lambda _{\mathrm {R}}}\sim \delta u_{\lambda _{\mathrm {R}}}$. One can estimate these quantities by the same logic as led to (7.10): if this new cascade is to carry (a finite fraction of) the same energy flux as produced the fold,Footnote 78 then
 \begin{equation} \frac{\delta u_{\lambda_{\mathrm{R}}}^3}{\lambda_{\mathrm{R}}}\sim\varepsilon\quad\Rightarrow\quad \delta u_{\lambda_{\mathrm{R}}}\sim(\varepsilon\lambda_{\mathrm{R}})^{1/3}\sim\delta u_L\left(\frac{\lambda_{\mathrm{R}}}{L}\right)^{1/3} \sim \delta u_L\mathrm{Rm}_L^{{-}1/9}(1+\mathrm{Pm})^{{-}1/18}\sim\delta b_{\lambda_{\mathrm{R}}}. \end{equation}
\begin{equation} \frac{\delta u_{\lambda_{\mathrm{R}}}^3}{\lambda_{\mathrm{R}}}\sim\varepsilon\quad\Rightarrow\quad \delta u_{\lambda_{\mathrm{R}}}\sim(\varepsilon\lambda_{\mathrm{R}})^{1/3}\sim\delta u_L\left(\frac{\lambda_{\mathrm{R}}}{L}\right)^{1/3} \sim \delta u_L\mathrm{Rm}_L^{{-}1/9}(1+\mathrm{Pm})^{{-}1/18}\sim\delta b_{\lambda_{\mathrm{R}}}. \end{equation}
Finally, the length of the flux rope (its ‘parallel’ scale) is set by critical balance: the scale over which coherence can be maintained by propagating information at the Alfvén speed ![]() $\sim B_{\lambda _{\mathrm {R}}}\sim \delta u_L$ is
$\sim B_{\lambda _{\mathrm {R}}}\sim \delta u_L$ is
Thus, we have got ourselves a critically balanced RMHD-type cascade, with ![]() $\delta u_L\sim B_{\lambda _{\mathrm {R}}}$ being the Alfvén speed,
$\delta u_L\sim B_{\lambda _{\mathrm {R}}}$ being the Alfvén speed, ![]() $\lambda _{\mathrm {R}}$ given by (13.9) playing the role of
$\lambda _{\mathrm {R}}$ given by (13.9) playing the role of ![]() $\lambda _{\mathrm {CB}}$,
$\lambda _{\mathrm {CB}}$, ![]() $l_{\parallel }$ given by (13.12) in the role of the parallel outer scale
$l_{\parallel }$ given by (13.12) in the role of the parallel outer scale ![]() $L_{\parallel }$, and the outer-scale amplitude
$L_{\parallel }$, and the outer-scale amplitude ![]() $\delta u_{\lambda _{\mathrm {R}}}$ given by (13.11). The RMHD ordering parameter for this cascade is, therefore,
$\delta u_{\lambda _{\mathrm {R}}}$ given by (13.11). The RMHD ordering parameter for this cascade is, therefore,
not terribly small in any real-world situation, but perfectly legitimate in principle. The Reynolds number of this cascade will generally large:
The latter inequality might not always be satisfied when ![]() $\mathrm {Pm}\gg 1$, but again is a perfectly legitimate limit. When it is not satisfied, the motions produced by the tearing of the folds will be quickly dissipated by viscosity and thus cannot seed a proper cascade; one option then is to invoke § 13.4.1 to deal with the sub-
$\mathrm {Pm}\gg 1$, but again is a perfectly legitimate limit. When it is not satisfied, the motions produced by the tearing of the folds will be quickly dissipated by viscosity and thus cannot seed a proper cascade; one option then is to invoke § 13.4.1 to deal with the sub-![]() $\lambda _{\mathrm {R}}$ structure.
$\lambda _{\mathrm {R}}$ structure.
The RMHD cascade seeded by the production of flux ropes in tearing folds, as just described, will do what an RMHD cascade does: push energy to smaller scales, become aligned and be eventually disrupted by tearing. The arguments of § 7.2 apply: a succession of mini-cascades will be seeded, etc., as per figure 14. One expects a ![]() $k^{-3/2}$ spectrum (6.25) down to the disruption scale set by (7.2), viz.,
$k^{-3/2}$ spectrum (6.25) down to the disruption scale set by (7.2), viz.,
Below this scale, the mini-cascades will have a ![]() $k^{-11/5}$ spectral envelope described by (7.15) (figure 34). The final resistive cut-off is then determined by (7.19):
$k^{-11/5}$ spectral envelope described by (7.15) (figure 34). The final resistive cut-off is then determined by (7.19):
the Kolmogorov scale again – reassuringly, some things in the world never change.

Figure 34. Spectrum of isotropic MHD turbulence, which is the saturated state of small-scale dynamo. The universal cascade below the fold-reversal scale ![]() $\lambda _{\mathrm {R}}$ (see (13.9)) is described in § 13.3.3. Various options for the spectrum at
$\lambda _{\mathrm {R}}$ (see (13.9)) is described in § 13.3.3. Various options for the spectrum at ![]() $k\lambda _{\mathrm {R}} < 1$ are discussed in § 13.4; in particular, the
$k\lambda _{\mathrm {R}} < 1$ are discussed in § 13.4; in particular, the ![]() $k^{-1}$ magnetic spectrum is (13.18). The velocity spectrum may well be steeper than
$k^{-1}$ magnetic spectrum is (13.18). The velocity spectrum may well be steeper than ![]() $k^{-5/3}$ at the largest scales and then shallower at smaller ones (see § 13.2), before steepening again at
$k^{-5/3}$ at the largest scales and then shallower at smaller ones (see § 13.2), before steepening again at ![]() $k\lambda _{\mathrm {R}}\gtrsim 1$. The disruption (
$k\lambda _{\mathrm {R}}\gtrsim 1$. The disruption (![]() $\lambda _{\mathrm {D}}$) and dissipation (
$\lambda _{\mathrm {D}}$) and dissipation (![]() $\lambda _{\eta }$) scales are given by (13.15) and (13.16), respectively. Whether the
$\lambda _{\eta }$) scales are given by (13.15) and (13.16), respectively. Whether the ![]() $k^{-11/5}$ spectrum starts at
$k^{-11/5}$ spectrum starts at ![]() $\lambda _{\mathrm {R}}$ (§ 13.4.1) or at
$\lambda _{\mathrm {R}}$ (§ 13.4.1) or at ![]() $\lambda _{\mathrm {D}}$ (§ 13.3.3) is not obvious because how the spectra at scales below and above
$\lambda _{\mathrm {D}}$ (§ 13.3.3) is not obvious because how the spectra at scales below and above ![]() $\lambda _{\mathrm {R}}$ are connected remains an open question.
$\lambda _{\mathrm {R}}$ are connected remains an open question.
Thus, turbulence in the saturated state of small-scale dynamo is, at scales below ![]() $\lambda _{\mathrm {R}}$, likely to be similar to the tearing-mediated turbulence of § 7.2, the only difference being that the direction of the ‘local mean field’ will be fluctuating strongly and all the statistics will be isotropic overall (although certainly not isotropic with respect to this fluctuating local mean field, as indeed spotted by Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and, most recently, studied by St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020; in a decaying, no-mean-field set up, this was shown already by Milano et al. Reference Milano, Matthaeus, Dmitruk and Montgomery2001). An aesthetically pleasing conclusion from all this is that universality is regained at small scales: even without the crutch of the mean field, MHD turbulence manages to turn itself into RMHD (Alfvénic) turbulence, at least in local patches where it is seeded by the tearing of the folds.
$\lambda _{\mathrm {R}}$, likely to be similar to the tearing-mediated turbulence of § 7.2, the only difference being that the direction of the ‘local mean field’ will be fluctuating strongly and all the statistics will be isotropic overall (although certainly not isotropic with respect to this fluctuating local mean field, as indeed spotted by Beresnyak & Lazarian Reference Beresnyak and Lazarian2009a and, most recently, studied by St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020; in a decaying, no-mean-field set up, this was shown already by Milano et al. Reference Milano, Matthaeus, Dmitruk and Montgomery2001). An aesthetically pleasing conclusion from all this is that universality is regained at small scales: even without the crutch of the mean field, MHD turbulence manages to turn itself into RMHD (Alfvénic) turbulence, at least in local patches where it is seeded by the tearing of the folds.
13.3.4. Outstanding question
Just as I did in § 7.3, let me ask first whether this is a falsifiable theory. The numerical evidence that I described in § 13.2 was what encouraged me to bring reconnection into the dynamo game. However, it is clear that the state-of-the-art numerical spectra shown in figure 33 describe a still non-asymptotic situation, at best only just starting to capture reconnection in the folds. Is there hope to do better any time soon? Surprisingly, the answer is ‘yes’. Just like in the case of the tearing-mediated cascade with a mean field (§ 7.3), numerical verification that, in the earlier version of this review, I referred to as a distant hope, is becoming reality before the final version goes into print. The just-published dynamo simulations by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022), which aimed specially to assess the fold-tearing scenario proposed above and pushed the numerical resolution upwards another notch (to ![]() $2240^3$), appear to offer some tentative evidence for the disruption of folds by tearing, the
$2240^3$), appear to offer some tentative evidence for the disruption of folds by tearing, the ![]() $\lambda _{\mathrm {R}}\propto \mathrm {Rm}^{1/3}$ scaling of the field-reversal scale,Footnote 79 and a steeper-than-Kolmogorov spectrum below that scale (
$\lambda _{\mathrm {R}}\propto \mathrm {Rm}^{1/3}$ scaling of the field-reversal scale,Footnote 79 and a steeper-than-Kolmogorov spectrum below that scale (![]() $\propto k^{-2}$ or thereabouts). Thus, I present this scenario now with greater confidence than I originally thought I could. The theory appears to be on the brink of being testable by state-of-the-art numerics.
$\propto k^{-2}$ or thereabouts). Thus, I present this scenario now with greater confidence than I originally thought I could. The theory appears to be on the brink of being testable by state-of-the-art numerics.
The key open question, however, both theoretically and numerically, is still what has been the central question of small-scale-dynamo theory since the founding papers of Batchelor (Reference Batchelor1950) and Biermann & Schlüter (Reference Biermann and Schlüter1951) and preoccupied the authors of the long string of papers cited in § 13.1: will magnetic energy get stuck at small scales (in my current scheme, at the reversal scale ![]() $\lambda _{\mathrm {R}}$ given by (13.9)) or will it make its way to the outer scale
$\lambda _{\mathrm {R}}$ given by (13.9)) or will it make its way to the outer scale ![]() $L$ via some further step in the nonlinear evolution? The latter would be everyone's preferred outcome: astrophysicists could then have their cosmic magnetic fields at the scales where they are observed to be,Footnote 80 while theoretical physicists could stop worrying about no-mean-field vs. strong-mean-field MHD upsetting their universalist dream by exhibiting different kinds of turbulence – the fluctuating field at
$L$ via some further step in the nonlinear evolution? The latter would be everyone's preferred outcome: astrophysicists could then have their cosmic magnetic fields at the scales where they are observed to be,Footnote 80 while theoretical physicists could stop worrying about no-mean-field vs. strong-mean-field MHD upsetting their universalist dream by exhibiting different kinds of turbulence – the fluctuating field at ![]() $L$ would just be the effective mean field in the inertial range, as Kraichnan (Reference Kraichnan1965) prophesied.
$L$ would just be the effective mean field in the inertial range, as Kraichnan (Reference Kraichnan1965) prophesied.
As I already said in §§ 13.1 and 13.2, a definitive demonstration of an inverse cascade in forced, isotropic MHD turbulence has remained elusive, leaving space for disagreement over how to interpret insufficiently asymptotic simulations; the highest-resolution-to-date study by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022) does not nail the issue completely but would be much easier to interpret if a compelling argument in favour of an ![]() $\mathrm {Rm}$-independent magnetic-energy-containing scale were available after all. I do not currently have such an argument – but I am able to offer, in § 13.4, a small catalogue of speculations, mine and other people's, about some relevant physical processes – note that they are not necessarily all mutually exclusive and may coexist in a saturated dynamo state, perhaps in different spatial patches or at different times.
$\mathrm {Rm}$-independent magnetic-energy-containing scale were available after all. I do not currently have such an argument – but I am able to offer, in § 13.4, a small catalogue of speculations, mine and other people's, about some relevant physical processes – note that they are not necessarily all mutually exclusive and may coexist in a saturated dynamo state, perhaps in different spatial patches or at different times.
13.4. Saturation scenarios
Wenn es aber Wirklichkeitssinn gibt, und niemand wird bezweifeln, daß er seine Daseinsberechtigung hat, dann muß es auch etwas geben, das man Möglichkeitssinn nennen kann. Wer ihn besitzt, sagt beispielweise nicht: Hier ist dies oder das geschehet, wird geschehen, muß geschehen; sondern er erfindet: Hier könnte, sollte oder müßte geschehen; und wenn man ihm von irgend etwas erklärt, daß es so sei, wie es sei, dann denkt er: Nun, es könnte wahrscheinlich auch anders sein. So ließe sich der Möglichkeitssinn geradezu als die Fähigkeit definieren, alles, was ebensogut sein könnte, zu denken und das, was ist, nicht wichtiger zu nehmen als das, was nicht ist. Man sieht, daß die Folgen solcher schöpferischen Anlage bemerkenswert sein können…
Robert Musil, Der Mann ohne Eigenshaften Footnote 81
To start with, let me imagine for the time being that the state with field reversals at the scale ![]() $\lambda _{\mathrm {R}}$ conjectured in § 13.3.3 is the saturated state and explore what happens between
$\lambda _{\mathrm {R}}$ conjectured in § 13.3.3 is the saturated state and explore what happens between ![]() $\lambda _{\mathrm {R}}$ and the outer scale
$\lambda _{\mathrm {R}}$ and the outer scale ![]() $L$. I shall discuss this in §§ 13.4.1–13.4.3 before moving on to schemes for bringing magnetic energy to the outer scale in §§ 13.4.4–13.4.8. A reader wary of speculations (who has, nevertheless, inexplicably, got this far) may wish just to read §§ 13.4.2 and 13.4.4 and move on to § 14.
$L$. I shall discuss this in §§ 13.4.1–13.4.3 before moving on to schemes for bringing magnetic energy to the outer scale in §§ 13.4.4–13.4.8. A reader wary of speculations (who has, nevertheless, inexplicably, got this far) may wish just to read §§ 13.4.2 and 13.4.4 and move on to § 14.
13.4.1. Multiscale folds?
In § 13.3.3, I assumed that in the saturated state, the only motions capable of stretching magnetic fields into folds were the outer-scale motions and that, consequently, all folds had length ![]() $L$ and reversal scale
$L$ and reversal scale ![]() $\lambda _{\mathrm {R}}=\lambda (L)$ (see (13.7) and (13.9)). Let me now relax this assumption and inquire what would happen if motions across some scale range
$\lambda _{\mathrm {R}}=\lambda (L)$ (see (13.7) and (13.9)). Let me now relax this assumption and inquire what would happen if motions across some scale range ![]() $\ell < L$ produced different, independent folds. As I argued in § 13.3.2, the fields produced by any given motion cannot be stronger than this motion, so let us take a bold leap and guess that, for
$\ell < L$ produced different, independent folds. As I argued in § 13.3.2, the fields produced by any given motion cannot be stronger than this motion, so let us take a bold leap and guess that, for ![]() $\lambda < \lambda _{\mathrm {R}}$,
$\lambda < \lambda _{\mathrm {R}}$,
where ![]() $\ell (\lambda )$ is obtained by inverting the
$\ell (\lambda )$ is obtained by inverting the ![]() $\lambda (\ell )$ dependence (13.7). In a triumph of dimensional inevitability, this is just the same as the scaling (7.14), leading to the familiar
$\lambda (\ell )$ dependence (13.7). In a triumph of dimensional inevitability, this is just the same as the scaling (7.14), leading to the familiar ![]() $k^{-11/5}$ spectrum (7.15) (cf. the relationship between the
$k^{-11/5}$ spectrum (7.15) (cf. the relationship between the ![]() $\xi$ and
$\xi$ and ![]() $\lambda$ scalings in § 7.2.3). One can now again fantasise about these folds breaking up into flux ropes as described by § 13.3.3, seeding mini-cascades similar to those produced by the successive disruptions of the RMHD cascade – those too have a
$\lambda$ scalings in § 7.2.3). One can now again fantasise about these folds breaking up into flux ropes as described by § 13.3.3, seeding mini-cascades similar to those produced by the successive disruptions of the RMHD cascade – those too have a ![]() $k^{-11/5}$ upper envelope, so perhaps this scaling emerges as an inevitable outcome at small scales of pretty much any scenario that involves resistivity.
$k^{-11/5}$ upper envelope, so perhaps this scaling emerges as an inevitable outcome at small scales of pretty much any scenario that involves resistivity.
Note that in the scheme leading to (13.17), interactions between velocities and magnetic fields are non-local in scale: velocities at scale ![]() $\ell$ interact with magnetic fields at scale
$\ell$ interact with magnetic fields at scale ![]() $\lambda (\ell )\ll \ell$, and vice versa.Footnote 82 This non-locality is more extreme than, e.g., Beresnyak (Reference Beresnyak2012c) would have it, because
$\lambda (\ell )\ll \ell$, and vice versa.Footnote 82 This non-locality is more extreme than, e.g., Beresnyak (Reference Beresnyak2012c) would have it, because ![]() $\lambda /\ell$ in (13.7) scales with
$\lambda /\ell$ in (13.7) scales with ![]() $\mathrm {Rm}_\ell$ and
$\mathrm {Rm}_\ell$ and ![]() $\mathrm {Pm}$, which are asymptotically large numbers (although it scales with quite modest fractional powers of them). Below
$\mathrm {Pm}$, which are asymptotically large numbers (although it scales with quite modest fractional powers of them). Below ![]() $\lambda _{\mathrm {R}}$, however, this non-locality should get swamped by the local RMHD cascade proposed in § 13.3.3.
$\lambda _{\mathrm {R}}$, however, this non-locality should get swamped by the local RMHD cascade proposed in § 13.3.3.
13.4.2. Spectra above the reversal scale
In the absence, as yet, of any mechanism for magnetic structures at scales longer than ![]() $\lambda _{\mathrm {R}}$ to emerge dynamically, the magnetic spectrum at scales above
$\lambda _{\mathrm {R}}$ to emerge dynamically, the magnetic spectrum at scales above ![]() $\lambda _{\mathrm {R}}$ should reflect the volume-filling properties of folds and the distribution of their reversal scales (all of this is certain to be highly intermittent). The simplest, perhaps too simple, guess is that it will be (Yousef et al. Reference Yousef, Rincon and Schekochihin2007),
$\lambda _{\mathrm {R}}$ should reflect the volume-filling properties of folds and the distribution of their reversal scales (all of this is certain to be highly intermittent). The simplest, perhaps too simple, guess is that it will be (Yousef et al. Reference Yousef, Rincon and Schekochihin2007),
which follows if one assumes that magnetic increments across any point separation ![]() $>\lambda _{\mathrm {R}}$ will tend to have, in an averaged sense, the same value, roughly equal to the rms field
$>\lambda _{\mathrm {R}}$ will tend to have, in an averaged sense, the same value, roughly equal to the rms field ![]() $B_{\lambda _{\mathrm {R}}}$, i.e., that there is the same magnetic energy at every scale
$B_{\lambda _{\mathrm {R}}}$, i.e., that there is the same magnetic energy at every scale ![]() $\in [\lambda _{\mathrm {R}},L]$ (cf. § 11.2). The spectrum (13.18) has the unique property that, while its peak would sit at some
$\in [\lambda _{\mathrm {R}},L]$ (cf. § 11.2). The spectrum (13.18) has the unique property that, while its peak would sit at some ![]() $k_\mathrm {peak}\sim L^{-1}$, its energy is weakly (logarithmically) dominated by its small-scale cutoff, viz.,
$k_\mathrm {peak}\sim L^{-1}$, its energy is weakly (logarithmically) dominated by its small-scale cutoff, viz., ![]() $k\sim \lambda _{\mathrm {R}}^{-1}$. Remarkably, this appears to be consistent, or at least not inconsistent, with what was found by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022), who see the spectral peak at a wavenumber (approximately) independent of
$k\sim \lambda _{\mathrm {R}}^{-1}$. Remarkably, this appears to be consistent, or at least not inconsistent, with what was found by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022), who see the spectral peak at a wavenumber (approximately) independent of ![]() $\mathrm {Rm}$, but a fairly flat profile of
$\mathrm {Rm}$, but a fairly flat profile of ![]() $k E_B(k)$ up to
$k E_B(k)$ up to ![]() $k\sim \lambda _{\mathrm {R}}^{-1}$.
$k\sim \lambda _{\mathrm {R}}^{-1}$.
There is still the question of what the velocity field does in the interval ![]() $[\lambda _{\mathrm {R}},L]$. In (13.17), I blithely assumed that it continued to obey the Kolmogorov scaling (13.1). If this were true, that would connect nicely onto the flux-rope amplitude (13.11) (and hence onward to the universal tearing-mediated cascade). Admittedly, however, the justification for a Kolmogorov scaling in that case is difficult as (13.17) implies energy exchanges with the folds at smaller scales and thus undermines the assumption of a constant flux through the scale range between
$[\lambda _{\mathrm {R}},L]$. In (13.17), I blithely assumed that it continued to obey the Kolmogorov scaling (13.1). If this were true, that would connect nicely onto the flux-rope amplitude (13.11) (and hence onward to the universal tearing-mediated cascade). Admittedly, however, the justification for a Kolmogorov scaling in that case is difficult as (13.17) implies energy exchanges with the folds at smaller scales and thus undermines the assumption of a constant flux through the scale range between ![]() $L$ and
$L$ and ![]() $\lambda _{\mathrm {R}}$. If the energy flux
$\lambda _{\mathrm {R}}$. If the energy flux ![]() $\varepsilon$ were depleted in favour of the folds at each scale
$\varepsilon$ were depleted in favour of the folds at each scale ![]() $\ell$ on the same typical time scale
$\ell$ on the same typical time scale ![]() $\ell /\delta u_\ell$ as the cascade of
$\ell /\delta u_\ell$ as the cascade of ![]() $\delta u_\ell$ proceeded, then the velocity field would have a steeper-than-Kolmogorov spectrum. The most elementary way to see this is to model the evolution of the spectrum
$\delta u_\ell$ proceeded, then the velocity field would have a steeper-than-Kolmogorov spectrum. The most elementary way to see this is to model the evolution of the spectrum ![]() $E_k$ as (cf. Batchelor Reference Batchelor1953)
$E_k$ as (cf. Batchelor Reference Batchelor1953)
where ![]() $\varepsilon _k$ is the energy flux through wavenumber
$\varepsilon _k$ is the energy flux through wavenumber ![]() $k$,
$k$, ![]() $\tau _k$ is the cascade time (
$\tau _k$ is the cascade time (![]() $\sim \tau _{\mathrm {nl}}$), and
$\sim \tau _{\mathrm {nl}}$), and ![]() $\gamma _k$ is the rate of transfer of the kinetic energy into the folds. Letting
$\gamma _k$ is the rate of transfer of the kinetic energy into the folds. Letting ![]() $\gamma _k\tau _k = \sigma = \mathrm {const}$ and seeking a steady-state solution gives
$\gamma _k\tau _k = \sigma = \mathrm {const}$ and seeking a steady-state solution gives
Note that, via a calculation analogous to (13.17), this would lead to a steeper-than-![]() $k^{-11/5}$ spectrum of folds, meaning that folds with reversal scales smaller than
$k^{-11/5}$ spectrum of folds, meaning that folds with reversal scales smaller than ![]() $\lambda _{\mathrm {R}}$ would get swamped by the tearing-mediated cascade originating from the longest,
$\lambda _{\mathrm {R}}$ would get swamped by the tearing-mediated cascade originating from the longest, ![]() $\lambda _{\mathrm {R}}$-scale folds, and we would be back to the scenario described in § 13.3.3.
$\lambda _{\mathrm {R}}$-scale folds, and we would be back to the scenario described in § 13.3.3.
Cho et al. (Reference Cho, Vishniac, Beresnyak, Lazarian and Ryu2009) and Beresnyak (Reference Beresnyak2012c) report that the fraction of the energy flux transferred into magnetic fields during the self-similar regime described in § 13.3.2 is numerically quite small – between 0.04 and 0.07. This suggests that any steepening of the velocity spectrum compared to the Kolmogorov scaling should, in theory, be very slight. The numerical evidence on velocity spectra was reviewed at the end of § 13.2 (see figure 33) – there is some steepening at low ![]() $k$ and shallowing at higher
$k$ and shallowing at higher ![]() $k$, as magnetic fields’ back reaction kicks in, effectively making
$k$, as magnetic fields’ back reaction kicks in, effectively making ![]() $\gamma _k<0$ in (13.19), but none of the extant simulations is likely to have reached asymptotically large
$\gamma _k<0$ in (13.19), but none of the extant simulations is likely to have reached asymptotically large ![]() $\mathrm {Re}$ or
$\mathrm {Re}$ or ![]() $\mathrm {Rm}$.
$\mathrm {Rm}$.
13.4.3. Magnetoelastic turbulence?
Let us now explore what happens in the scale interval ![]() $[\lambda _{\mathrm {R}},L]$ if we abandon (13.17) and return to the scenario in which the velocity field at the outer scale
$[\lambda _{\mathrm {R}},L]$ if we abandon (13.17) and return to the scenario in which the velocity field at the outer scale ![]() $L$ constantly passes a certain fraction of the injected power
$L$ constantly passes a certain fraction of the injected power ![]() $\varepsilon$ to the folds with reversals at
$\varepsilon$ to the folds with reversals at ![]() $\lambda _{\mathrm {R}}$ and hence into the tearing-mediated cascade, while the rest of the injected power goes into some motions on scales
$\lambda _{\mathrm {R}}$ and hence into the tearing-mediated cascade, while the rest of the injected power goes into some motions on scales ![]() $[\lambda _{\mathrm {R}},L]$ that do not exchange energy with that cascade, i.e., do not stretch the field or cause it to develop sub-
$[\lambda _{\mathrm {R}},L]$ that do not exchange energy with that cascade, i.e., do not stretch the field or cause it to develop sub-![]() $\lambda _{\mathrm {R}}$ structure. What kind of motions can these be?
$\lambda _{\mathrm {R}}$ structure. What kind of motions can these be?
In search of the answer to this question, I wish to revisit the old idea (Moffatt Reference Moffatt1986; Gruzinov & Diamond Reference Gruzinov and Diamond1996; Chandran Reference Chandran1997; Schekochihin et al. Reference Schekochihin, Cowley, Hammett, Maron and McWilliams2002; Maron et al. Reference Maron, Cowley and McWilliams2004) that a tangled mess of small-scale magnetic fields provides an elastic background through which larger-scale Alfvén waves can propagate isotropically. The relevant calculation is straightforward. Consider the equations of incompressible MHD without a mean field:
where the equation for pressure ![]() $p$ is
$p$ is ![]() $\partial _i u_i = 0$ and
$\partial _i u_i = 0$ and ![]() $M_{ij}=B_i B_j$ is the Maxwell stress tensor (the magnetic field is in velocity units). We can recast the induction equation (13.22) in terms of
$M_{ij}=B_i B_j$ is the Maxwell stress tensor (the magnetic field is in velocity units). We can recast the induction equation (13.22) in terms of ![]() $M_{ij}$ and forget about
$M_{ij}$ and forget about ![]() $B_i$:
$B_i$:
The information about magnetic fields’ reversals is now hidden away and only their ability to exert Lorentz force, quadratic in ![]() $B_i$, is retained. Let us expand the flows and the Maxwell stresses around a time- and space-averaged state:
$B_i$, is retained. Let us expand the flows and the Maxwell stresses around a time- and space-averaged state:
Linearising (13.21) and (13.23) around this ‘equilibrium’ filled with tangled fields, we get isotropically propagating Alfvén waves whose dispersion relation and eigenvector areFootnote 83
where ![]() $\xi _i$ is the displacement (
$\xi _i$ is the displacement (![]() $\partial _t\xi _i = u_i$). These can be dubbed magnetoelastic waves to highlight the formal mathematical (Ogilvie & Proctor Reference Ogilvie and Proctor2003) and obvious physical analogy between a magnetised plasma and certain types of polymeric fluids. Admittedly, this analogy between magnetic field lines and polymer strands moving with the fluid and elastically back-reacting on it becomes precarious if one looks beyond the ideal description: there is no such thing as ‘antiparallel’ polymers strands, and so there is no reconnection. It is not obvious whether fast reconnection of field lines can foil their ability to make plasma an elastic medium: do tangled fields spring back when pushed at or just reconnect quickly to accommodate the push? Here, I shall imagine that they do spring back and explore the consequences.
$\partial _t\xi _i = u_i$). These can be dubbed magnetoelastic waves to highlight the formal mathematical (Ogilvie & Proctor Reference Ogilvie and Proctor2003) and obvious physical analogy between a magnetised plasma and certain types of polymeric fluids. Admittedly, this analogy between magnetic field lines and polymer strands moving with the fluid and elastically back-reacting on it becomes precarious if one looks beyond the ideal description: there is no such thing as ‘antiparallel’ polymers strands, and so there is no reconnection. It is not obvious whether fast reconnection of field lines can foil their ability to make plasma an elastic medium: do tangled fields spring back when pushed at or just reconnect quickly to accommodate the push? Here, I shall imagine that they do spring back and explore the consequences.
One of the consequences appears to be a surprising return of the IK turbulence (§ 2.2), which I have so far thoroughly dismissed – perhaps an indication that a clever idea, however wrong, never goes to waste. The reason that the IK scheme was wrong in the presence of a strong mean magnetic field was that Alfvén waves could not be legitimately expected to run around isotropically at small scales. Well, according to (13.25), the magnetoelastic waves do run around isotropically, and so the IK theory is back in business. While Kraichnan's dimensional argument leading to (2.5) may or may not be compelling, the version of the IK theory outlined in footnote 5 is perhaps sensible. Indeed, whereas at the outer scale ![]() $L$, the nonlinear time
$L$, the nonlinear time ![]() $\tau _{\mathrm {nl}}\sim L/\delta u_L$ and the Alfvén time
$\tau _{\mathrm {nl}}\sim L/\delta u_L$ and the Alfvén time ![]() $\tau _{\mathrm {A}}\sim L/v_{\mathrm {A}}$ are certainly comparable (because
$\tau _{\mathrm {A}}\sim L/v_{\mathrm {A}}$ are certainly comparable (because ![]() $\langle B^2\rangle \sim \delta u_L^2$ for saturated dynamo), the former will shorten less quickly than the latter at smaller scales (
$\langle B^2\rangle \sim \delta u_L^2$ for saturated dynamo), the former will shorten less quickly than the latter at smaller scales (![]() $\tau _{\mathrm {A}}\propto \ell$, while
$\tau _{\mathrm {A}}\propto \ell$, while ![]() $\tau _{\mathrm {nl}}\propto$ a fractional power of
$\tau _{\mathrm {nl}}\propto$ a fractional power of ![]() $\ell$). Thus, at scales
$\ell$). Thus, at scales ![]() $\ell \ll L$, the magnetoelastic turbulence might be expected to be weak. The cascade time is then worked out from the random-walk argument (4.5), and the spectrum follows from the constancy of flux:
$\ell \ll L$, the magnetoelastic turbulence might be expected to be weak. The cascade time is then worked out from the random-walk argument (4.5), and the spectrum follows from the constancy of flux:
Presumably, this cascade terminates when it hits ![]() $k\sim \lambda _{\mathrm {R}}^{-1}$, where the scale separation between the magnetoelastic waves and the magnetic fields associated with the tearing-mediated cascade of § 13.3.3 breaks down.
$k\sim \lambda _{\mathrm {R}}^{-1}$, where the scale separation between the magnetoelastic waves and the magnetic fields associated with the tearing-mediated cascade of § 13.3.3 breaks down.
There is some numerical evidence in favour of an isotropic ![]() $k^{-3/2}$ spectrum of perturbations with a sound-like isotropic dispersion relation
$k^{-3/2}$ spectrum of perturbations with a sound-like isotropic dispersion relation ![]() $\omega \propto k$ – the MHD fast (magnetoacoustic) waves: see Cho & Lazarian (Reference Cho and Lazarian2002, Reference Cho and Lazarian2003), who were inspired by the same scaling derived for weak turbulence of sound waves by Zakharov & Sagdeev (Reference Zakharov and Sagdeev1970); a later study by Kowal & Lazarian (Reference Kowal and Lazarian2010) appears to be less certain about the scaling exponent.
$\omega \propto k$ – the MHD fast (magnetoacoustic) waves: see Cho & Lazarian (Reference Cho and Lazarian2002, Reference Cho and Lazarian2003), who were inspired by the same scaling derived for weak turbulence of sound waves by Zakharov & Sagdeev (Reference Zakharov and Sagdeev1970); a later study by Kowal & Lazarian (Reference Kowal and Lazarian2010) appears to be less certain about the scaling exponent.
One might have thought that some evidence as to how much of a fiction, or otherwise, the spectrum (13.26) were in an elastic medium, could be found in simulations of polymer-laden turbulence. Surprisingly, the state of the art in this area features much smaller resolutions than in MHD. The most recent relevant numerical papers appear to be Valente, da Silva & Pinho (Reference Valente, da Silva and Pinho2016) and Fathali & Khoei (Reference Fathali and Khoei2019) (see references therein for the paper trail). They report significant energy transfer in the inertial range from the motions of the solvent fluid to the elastic polymer admixture and back (quite a lot of it non-local in ![]() $k$, from large-scale flows to small-scale polymer structure, perhaps analogous to dynamo); they also see spectral exponents in the
$k$, from large-scale flows to small-scale polymer structure, perhaps analogous to dynamo); they also see spectral exponents in the ![]() $[-5/3,-3/2]$ range, at modest resolutions. They do not appear to be aware of, or interested in, the possibility of elastic waves.Footnote 84
$[-5/3,-3/2]$ range, at modest resolutions. They do not appear to be aware of, or interested in, the possibility of elastic waves.Footnote 84
Since polymer-laden turbulence has the advantage of being (relatively) easy to set up and measure in the laboratory, numerical simulations are not the only evidence available – there is a lively history of real experiments, amongst which some recent ones appear to have been important breakthroughs. Thus, Varshney & Steinberg (Reference Varshney and Steinberg2019) have, for the first time, it seems, managed to excite and measure elastic waves experimentally. Zhang et al. (Reference Zhang, Bodenschatz, Xu and Xi2021) report detailed measurements of scalings in the inertial range that imply an energy flux, increasing with ![]() $k$, from the fluid motions into the elastic energy of the polymers, and kinetic-energy spectra that have a well-developed power law,
$k$, from the fluid motions into the elastic energy of the polymers, and kinetic-energy spectra that have a well-developed power law, ![]() $k^{-2.38}$, which is steeper than what numerics show but shallower than what theoreticians (cited in footnote 84) predict. An open field for further theorising then, with not much more known definitively than in MHD.
$k^{-2.38}$, which is steeper than what numerics show but shallower than what theoreticians (cited in footnote 84) predict. An open field for further theorising then, with not much more known definitively than in MHD.
In MHD turbulence, even whether the magnetoelastic cascade (13.26), or indeed the magnetoelastic waves, exist at all remains an open question. Hosking et al. (Reference Hosking, Schekochihin and Balbus2020) have shown numerically that magnetoelastic waves do exist in certain tangled, force-free magnetic configurations, and are well described by (13.25) (modulo some further nuance that can mean that ![]() $v_{\mathrm {A}}$ is somewhat reduced for tangled fields that are spatially intermittent). What is still unknown is whether they can propagate against the background of a saturated dynamo state or are quickly damped by small-scale motions and thus rendered irrelevant.
$v_{\mathrm {A}}$ is somewhat reduced for tangled fields that are spatially intermittent). What is still unknown is whether they can propagate against the background of a saturated dynamo state or are quickly damped by small-scale motions and thus rendered irrelevant.
13.4.4. Fast-reconnection-limited dynamo?
The narrative arc that in § 7.4.2 led me to examine, with Loureiro & Boldyrev (Reference Loureiro and Boldyrev2020), the possibility of a competition between tearing and fast plasmoid reconnection (reviewed in Appendix D.6), naturally invites doing the same for dynamo. For magnetic folds with reversals at scale ![]() $\lambda$ driven by motions at scale
$\lambda$ driven by motions at scale ![]() $\ell$ and energetically comparable to those motions, let us compare the fast-reconnection time given by a formula analogous to (7.25) with the characteristic time of the flow:
$\ell$ and energetically comparable to those motions, let us compare the fast-reconnection time given by a formula analogous to (7.25) with the characteristic time of the flow:
where ![]() $\epsilon _{\mathrm {rec}}\sim \tilde S_{\mathrm {c}}^{-1/2}(1+\mathrm {Pm})^{-1/2}$ and
$\epsilon _{\mathrm {rec}}\sim \tilde S_{\mathrm {c}}^{-1/2}(1+\mathrm {Pm})^{-1/2}$ and ![]() $\tilde S_{\mathrm {c}}\sim 10^4$ (see Appendix D.4.2). In order for any possible effect of the onset of fast reconnection to matter,
$\tilde S_{\mathrm {c}}\sim 10^4$ (see Appendix D.4.2). In order for any possible effect of the onset of fast reconnection to matter, ![]() $\lambda _{\mathrm {rec}}$ must be larger than the reversal scale (13.7) set by tearing, which is achieved provided
$\lambda _{\mathrm {rec}}$ must be larger than the reversal scale (13.7) set by tearing, which is achieved provided
a tough ask, but not as bad as (7.26). Note that this condition, if satisfied, amply guarantees that the fast-reconnection regime actually is reached, i.e., that the slow-reconnection rate (see Appendix D.4.1) is slower than fast one, or
What would happen if (13.28) were achieved? In principle, this means that a fast-reconnecting plasmoid chain could be formed out of such a fold, seeding a reconnection-driven cascade (§ 7.4.3). In order for this to happen, the folds, rather than being destroyed by tearing and/or swept away by the flow, would have to stick around long enough for the plasmoids (flux ropes) in them to gobble up more flux and grow to bigger sizes than the reversal scale set by tearing (![]() $\lambda _{\mathrm {R}}$ for
$\lambda _{\mathrm {R}}$ for ![]() $\ell = L$), eventually to
$\ell = L$), eventually to ![]() $\lambda _{\mathrm {rec}}$. If this were to be the fate of most folds,
$\lambda _{\mathrm {rec}}$. If this were to be the fate of most folds, ![]() $\lambda _{\mathrm {rec}}$ would be the effective reversal scale, independent of
$\lambda _{\mathrm {rec}}$ would be the effective reversal scale, independent of ![]() $\mathrm {Rm}$, as ideally desired.
$\mathrm {Rm}$, as ideally desired.
I shall not go as far as claiming that this outcome accords with simulations – both because they do not usually have ![]() $\mathrm {Rm}$ anywhere close to (13.28) and because (13.27) with
$\mathrm {Rm}$ anywhere close to (13.28) and because (13.27) with ![]() $\ell = L$ would imply a magnetic-energy-containing scale
$\ell = L$ would imply a magnetic-energy-containing scale ![]() $\sim 10^2$ shorter than the outer scale, which is rather too short. However, if
$\sim 10^2$ shorter than the outer scale, which is rather too short. However, if ![]() $\epsilon _{\mathrm {rec}}$ were closer to
$\epsilon _{\mathrm {rec}}$ were closer to ![]() $10^{-1}$ than to
$10^{-1}$ than to ![]() $10^{-2}$ in a turbulent environment characteristic of the saturated dynamo, both the condition (13.28) and the prediction (13.27) would start looking much more realistic (alternatively, another method of increasing the field's scale is needed; see, e.g., § 13.4.8). Thus, a promising scenario, but still very much to be confirmed – and it is far from clear that it can be confirmed at the numerical resolutions likely to be available any time soon (cf. Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
$10^{-2}$ in a turbulent environment characteristic of the saturated dynamo, both the condition (13.28) and the prediction (13.27) would start looking much more realistic (alternatively, another method of increasing the field's scale is needed; see, e.g., § 13.4.8). Thus, a promising scenario, but still very much to be confirmed – and it is far from clear that it can be confirmed at the numerical resolutions likely to be available any time soon (cf. Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
It cannot have escaped the reader that an even more attractive possibility would be ![]() $\epsilon _{\mathrm {rec}} \sim 1$, which would absolve us from any constraints on
$\epsilon _{\mathrm {rec}} \sim 1$, which would absolve us from any constraints on ![]() $\mathrm {Rm}$ and set
$\mathrm {Rm}$ and set ![]() $\lambda _{\mathrm {rec}}\sim L$ in the saturated state. That possibility is effectively the one associated with the other type of fast reconnection – stochastic reconnection (Appendix D.7) – and is the currently trending dynamo-evolution scenario examined in § 13.4.5.
$\lambda _{\mathrm {rec}}\sim L$ in the saturated state. That possibility is effectively the one associated with the other type of fast reconnection – stochastic reconnection (Appendix D.7) – and is the currently trending dynamo-evolution scenario examined in § 13.4.5.
13.4.5. Xu & Lazarian (Reference Xu and Lazarian2016)
Xu & Lazarian (Reference Xu and Lazarian2016) propose that, in a ![]() $\mathrm {Pm}\gg 1$ system, once the magnetic energy has grown to be comparable to the energy of the viscous-scale eddies (
$\mathrm {Pm}\gg 1$ system, once the magnetic energy has grown to be comparable to the energy of the viscous-scale eddies (![]() $B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$; cf. § 13.3.1), its spectrum will embark on a rearrangement exercise in which its spectral peak moves from the resistive to the viscous scale while the overall magnetic energy stays constant (I will discuss this proposition in a moment). Once it reaches the viscous scale, a self-similar secular regime follows, of the kind described in § 13.3.2,Footnote 85 except the scale of
$B_{\lambda _{\eta }}\sim \delta u_{\ell _{\nu }}$; cf. § 13.3.1), its spectrum will embark on a rearrangement exercise in which its spectral peak moves from the resistive to the viscous scale while the overall magnetic energy stays constant (I will discuss this proposition in a moment). Once it reaches the viscous scale, a self-similar secular regime follows, of the kind described in § 13.3.2,Footnote 85 except the scale of ![]() $B_\lambda$ is now the same as the scale of the motions that are performing the dynamo action,
$B_\lambda$ is now the same as the scale of the motions that are performing the dynamo action, ![]() $\lambda \sim \ell (t)$, whereas below that scale, a GS95-type turbulent cascade forms, with
$\lambda \sim \ell (t)$, whereas below that scale, a GS95-type turbulent cascade forms, with ![]() $B_{\ell (t)}$ playing the role of the mean field (this is also the view of Beresnyak Reference Beresnyak2012c). As time advances,
$B_{\ell (t)}$ playing the role of the mean field (this is also the view of Beresnyak Reference Beresnyak2012c). As time advances, ![]() $\ell (t)\to L$, and the dynamo saturates with scale-by-scale equipartitioned
$\ell (t)\to L$, and the dynamo saturates with scale-by-scale equipartitioned ![]() $k^{-5/3}$ magnetic and velocity spectra, just like everyone since Biermann & Schlüter (Reference Biermann and Schlüter1951) has always wanted it to do.
$k^{-5/3}$ magnetic and velocity spectra, just like everyone since Biermann & Schlüter (Reference Biermann and Schlüter1951) has always wanted it to do.
In the narrative of Xu & Lazarian (Reference Xu and Lazarian2016), this pleasing outcome depends on the assumption, unproven, but not in principle impossible, that fast stochastic reconnection (reviewed in Appendix D.7) will always provide just enough turbulent magnetic diffusivity to prevent the dynamo-generated field from organising into folds with reversals at scales much below ![]() $\ell (t)$. I cannot rule this out definitively without a clear dynamical picture of the turbulence in the presence of dynamically significant dynamo-generated fields.Footnote 86
$\ell (t)$. I cannot rule this out definitively without a clear dynamical picture of the turbulence in the presence of dynamically significant dynamo-generated fields.Footnote 86
If this assumption proves true, the Xu & Lazarian (Reference Xu and Lazarian2016) scenario for the ![]() $\mathrm {Pm}\gg 1$ case still needs the earlier transitional stage to move the magnetic energy (i.e., the field-reversal scale) from the resistive to the viscous scale. They justify this by arguing that, since the spectrum of the magnetic field is
$\mathrm {Pm}\gg 1$ case still needs the earlier transitional stage to move the magnetic energy (i.e., the field-reversal scale) from the resistive to the viscous scale. They justify this by arguing that, since the spectrum of the magnetic field is ![]() $\propto k^{3/2}$ at
$\propto k^{3/2}$ at ![]() $k\ll \lambda _{\eta }^{-1}$ (Kazantsev Reference Kazantsev1968; Kulsrud & Anderson Reference Kulsrud and Anderson1992), the magnetic modes with
$k\ll \lambda _{\eta }^{-1}$ (Kazantsev Reference Kazantsev1968; Kulsrud & Anderson Reference Kulsrud and Anderson1992), the magnetic modes with ![]() $k\ll \lambda _{\eta }^{-1}$ can continue being amplified by the viscous-scale motions after those with
$k\ll \lambda _{\eta }^{-1}$ can continue being amplified by the viscous-scale motions after those with ![]() $k\sim \lambda _{\eta }^{-1}$ have reached energetic equipartition with those motions – if the overall magnetic energy is assumed to stay constant, this then leads to a gradual ‘overturning’ of the spectrum and shifts its peak towards the viscous scale.
$k\sim \lambda _{\eta }^{-1}$ have reached energetic equipartition with those motions – if the overall magnetic energy is assumed to stay constant, this then leads to a gradual ‘overturning’ of the spectrum and shifts its peak towards the viscous scale.
I do not think this argument is entirely satisfactory, for two reasons. First, the ![]() $k^{3/2}$ spectrum is a Fourier-space representation of the growing, folding field – it seems dubious to me to disaggregate it into individual Fourier modes and view each of them as an independent entity that back-reacts on the velocity field or is amplified by the latter separately from all others (amplification of the field is always accompanied by a change in its scale and the
$k^{3/2}$ spectrum is a Fourier-space representation of the growing, folding field – it seems dubious to me to disaggregate it into individual Fourier modes and view each of them as an independent entity that back-reacts on the velocity field or is amplified by the latter separately from all others (amplification of the field is always accompanied by a change in its scale and the ![]() $k^{3/2}$ spectrum is the resulting mean distribution of the magnetic energy amongst wavenumbers during its growth; see, e.g., lecture notes by Schekochihin Reference Schekochihin2022). Secondly, I do not see why the energy should stay constant, putting the self-similar stage on hold until magnetic fields and motions are at the same scale, rather than proceeding to grow in the way described in § 13.3.2. It seems to me that if the Xu & Lazarian (Reference Xu and Lazarian2016) scheme were correct, we should see their spectral rearrangement at constant energy already in numerical simulations with
$k^{3/2}$ spectrum is the resulting mean distribution of the magnetic energy amongst wavenumbers during its growth; see, e.g., lecture notes by Schekochihin Reference Schekochihin2022). Secondly, I do not see why the energy should stay constant, putting the self-similar stage on hold until magnetic fields and motions are at the same scale, rather than proceeding to grow in the way described in § 13.3.2. It seems to me that if the Xu & Lazarian (Reference Xu and Lazarian2016) scheme were correct, we should see their spectral rearrangement at constant energy already in numerical simulations with ![]() $\mathrm {Pm}\gg 1$ and
$\mathrm {Pm}\gg 1$ and ![]() $\mathrm {Re}\sim 1$, which is the only glimpse of truly scale-separated large-
$\mathrm {Re}\sim 1$, which is the only glimpse of truly scale-separated large-![]() $\mathrm {Pm}$ dynamics that we currently have. In the event, we do see the spectrum in the nonlinear stage of such simulations become shallower than
$\mathrm {Pm}$ dynamics that we currently have. In the event, we do see the spectrum in the nonlinear stage of such simulations become shallower than ![]() $k^{3/2}$ and shift a little towards larger scales – but not all the way to the flow's scale, with the peak still, it seems, at the resistive scale (this behaviour appears to be accounted for adequately by assuming that the magnetic folds locally anisotropise the viscous-scale flow and thus stymie its ability to amplify them: see Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Hammett, Maron and McWilliams2004a,Reference Schekochihin, Cowley, Taylor, Maron and McWilliamsb and St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020).
$k^{3/2}$ and shift a little towards larger scales – but not all the way to the flow's scale, with the peak still, it seems, at the resistive scale (this behaviour appears to be accounted for adequately by assuming that the magnetic folds locally anisotropise the viscous-scale flow and thus stymie its ability to amplify them: see Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Hammett, Maron and McWilliams2004a,Reference Schekochihin, Cowley, Taylor, Maron and McWilliamsb and St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020).
Thus, in my view, the Xu & Lazarian (Reference Xu and Lazarian2016) scenario, while attractive if true, remains at least as much of a speculation as anything that my exasperated reader will find elsewhere in this section.
13.4.6. Subramanian (Reference Subramanian1999)
While I am not proposing to review here the entire history of dynamo saturation schemes, it is useful to describe a model proposed a long time ago by Subramanian (Reference Subramanian1999), which, in a certain general sense, anticipated the Xu & Lazarian (Reference Xu and Lazarian2016) scenario and many other similar schemes, including § 13.4.4.
Subramanian (Reference Subramanian1999) conjectured that the effect of nonlinear back-reaction of magnetic fields on the flow would be to increase the effective magnetic diffusivity of the turbulent medium:
where ![]() $\tau$ is some adjustable constant with dimensions of time. This would go on until the effective magnetic Reynolds number reached the critical value at which the small-scale dynamo was at its threshold – known to be
$\tau$ is some adjustable constant with dimensions of time. This would go on until the effective magnetic Reynolds number reached the critical value at which the small-scale dynamo was at its threshold – known to be ![]() $\mathrm {Rm}_{\mathrm {c}}\sim 10^{1\dots 2}$ (see, e.g., Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007). He then proposed that the saturated state of the dynamo would simply be the marginal state of a kinematic dynamo with
$\mathrm {Rm}_{\mathrm {c}}\sim 10^{1\dots 2}$ (see, e.g., Schekochihin et al. Reference Schekochihin, Iskakov, Cowley, McWilliams, Proctor and Yousef2007). He then proposed that the saturated state of the dynamo would simply be the marginal state of a kinematic dynamo with ![]() $\mathrm {Rm}_\mathrm {eff}=\mathrm {Rm}_{\mathrm {c}}$, and with magnetic energy therefore sitting at the scale
$\mathrm {Rm}_\mathrm {eff}=\mathrm {Rm}_{\mathrm {c}}$, and with magnetic energy therefore sitting at the scale
This is not far from where, quantitatively, numerical simulations have been placing the peak of the magnetic-energy spectrum in finite-resolution boxes for the last two decades (see references in §§ 13.1 and 13.2). Indeed, Subramanian's prescription was operationalised by Schober et al. (Reference Schober, Schleicher, Federrath, Bovino and Klessen2015) to produce a fully fledged modelling tool for unfailingly successful comparisons with simulation outputs for specific values of ![]() $\mathrm {Re}$ and
$\mathrm {Re}$ and ![]() $\mathrm {Rm}$, as well as of the Mach number.
$\mathrm {Rm}$, as well as of the Mach number.
The key conceptual point is that under this scheme, there is no dependence of ![]() $\lambda _B$ on
$\lambda _B$ on ![]() $\mathrm {Rm}$. A slight wrinkle is that the magnetic energy in the saturated state is
$\mathrm {Rm}$. A slight wrinkle is that the magnetic energy in the saturated state is
if one makes the most obvious choice ![]() $\tau \sim L/\delta u_L$, i.e.,
$\tau \sim L/\delta u_L$, i.e., ![]() $\langle B^2\rangle$ is uncomfortably smaller than the kinetic energy (although not outrageously inconsistent with numerical evidence). Since
$\langle B^2\rangle$ is uncomfortably smaller than the kinetic energy (although not outrageously inconsistent with numerical evidence). Since ![]() $\mathrm {Rm}_{\mathrm {c}}$ is, however, merely a constant, this can be fudged by judicious modelling choices or, following Subramanian (Reference Subramanian1999), by arguing that if the magnetic energy is concentrated in flux ropes of radius
$\mathrm {Rm}_{\mathrm {c}}$ is, however, merely a constant, this can be fudged by judicious modelling choices or, following Subramanian (Reference Subramanian1999), by arguing that if the magnetic energy is concentrated in flux ropes of radius ![]() $\lambda _B$ and length
$\lambda _B$ and length ![]() $L$, then
$L$, then ![]() $\langle B^2\rangle \sim B^2(\lambda _B/L)^2 \sim B^2\mathrm {Rm}_{\mathrm {c}}^{-1}$, so the actual magnetic fields are, in fact, locally as strong as the flow.
$\langle B^2\rangle \sim B^2(\lambda _B/L)^2 \sim B^2\mathrm {Rm}_{\mathrm {c}}^{-1}$, so the actual magnetic fields are, in fact, locally as strong as the flow.
This approach does not address any rearrangements that might be caused by the Alfvénic dynamics, nor does it produce a specific mechanism for the enhancement of magnetic diffusivity, but it does capture, conceptually, a broad general class of physically plausible models, to which both § 13.4.4 and Xu & Lazarian (Reference Xu and Lazarian2016) also belong (stochastic reconnection is, of course, turbulent magnetic diffusivity by another name). To explain the enhancement of ![]() $\eta$, Brandenburg & Subramanian (Reference Brandenburg and Subramanian2005) introduce an effective ‘nonlinear drift’
$\eta$, Brandenburg & Subramanian (Reference Brandenburg and Subramanian2005) introduce an effective ‘nonlinear drift’ ![]() $\propto \boldsymbol {J}\times \boldsymbol {B}$, justified by analogy with ambipolar effects (a version of that was also explored by Subramanian Reference Subramanian2003, where he complicated his model somewhat to produce turbulent magnetic hyperdiffusion). Interestingly, an even earlier paper by Kulsrud & Anderson (Reference Kulsrud and Anderson1992) already contained calculations of nonlinear dynamo both for a model with ambipolar damping, featuring an effective magnetic diffusivity of the form (13.30), and with magnetic reconnection (see their § 4.2). In the latter case, instead of an effective magnetic diffusivity, they have a damping rate of the form
$\propto \boldsymbol {J}\times \boldsymbol {B}$, justified by analogy with ambipolar effects (a version of that was also explored by Subramanian Reference Subramanian2003, where he complicated his model somewhat to produce turbulent magnetic hyperdiffusion). Interestingly, an even earlier paper by Kulsrud & Anderson (Reference Kulsrud and Anderson1992) already contained calculations of nonlinear dynamo both for a model with ambipolar damping, featuring an effective magnetic diffusivity of the form (13.30), and with magnetic reconnection (see their § 4.2). In the latter case, instead of an effective magnetic diffusivity, they have a damping rate of the form ![]() $-\epsilon _{\mathrm {rec}}\langle B^2\rangle ^{1/2} k$ modelling the removal of magnetic structure by fast reconnection with dimensionless rate
$-\epsilon _{\mathrm {rec}}\langle B^2\rangle ^{1/2} k$ modelling the removal of magnetic structure by fast reconnection with dimensionless rate ![]() $\epsilon _{\mathrm {rec}}$ (this is just
$\epsilon _{\mathrm {rec}}$ (this is just ![]() $\tau _{\mathrm {rec}}^{-1}$ of § 13.4.4), but the outcome is similar. Their calculation of the self-similar dynamo regime with reconnection appears to be functionally equivalent to that of Xu & Lazarian (Reference Xu and Lazarian2016), and produces the same result.
$\tau _{\mathrm {rec}}^{-1}$ of § 13.4.4), but the outcome is similar. Their calculation of the self-similar dynamo regime with reconnection appears to be functionally equivalent to that of Xu & Lazarian (Reference Xu and Lazarian2016), and produces the same result.
To put all this in context, the other, competing general class of models includes schemes in which dynamically strong magnetic fields locally change the nature of the flow to stop it from amplifying them further (e.g., Cattaneo, Hughes & Kim Reference Cattaneo, Hughes and Kim1996; Zienicke, Politano & Pouquet Reference Zienicke, Politano and Pouquet1998; Kim Reference Kim1999; Schekochihin et al. Reference Schekochihin, Cowley, Taylor, Hammett, Maron and McWilliams2004a,Reference Schekochihin, Cowley, Taylor, Maron and McWilliamsb; Cattaneo & Tobias Reference Cattaneo and Tobias2009; Baggaley et al. Reference Baggaley, Shukurov, Barenghi and Subramanian2010; Rempel et al. Reference Rempel, Chian, Brandenburg, Muñoz and Shadden2013; Seta et al. Reference Seta, Bushby, Shukurov and Wood2020; St-Onge et al. Reference St-Onge, Kunz, Squire and Schekochihin2020). It is if one wants to make a theory of this type work that one is required to produce a dynamical mechanism for transferring magnetic energy from small scales to large.
13.4.7. Inverse magnetic-energy transfer via sporadic decay?
In pursuit of such mechanisms, an interesting recent development came from simulations of decaying MHD turbulence without a mean field: as I already mentioned in § 12.5, Zrake (Reference Zrake2014) and Brandenburg et al. (Reference Brandenburg, Kahniashvili and Tevzadze2015) discovered numerically that such a turbulence, even without net helicity, could support a certain amount of inverse transfer of magnetic energy from small to large scales (as expected theoretically: see § 12.5).Footnote 87 In § 12.4.2, I argued, following Zhou et al. (Reference Zhou, Loureiro and Uzdensky2020), Bhat et al. (Reference Bhat, Zhou and Loureiro2021), and Hosking & Schekochihin (Reference Hosking and Schekochihin2021), that the dynamical mechanism by which large-scale magnetic fields are generated in decaying, non-helical MHD turbulence starting from a magnetically dominated state is the merger of reconnecting flux ropes. Let me explore what would happen if the same mechanism were to apply locally to the magnetic structures at the reversal scale ![]() $\lambda _{\mathrm {R}}$, which are flux ropes (plasmoids), released from disintegrating folds.
$\lambda _{\mathrm {R}}$, which are flux ropes (plasmoids), released from disintegrating folds.
Imagine that, instead of being continuously forced everywhere, our saturated dynamo were to be left alone for a period of time (and/or in a region of space) – this could be due to the natural spatiotemporal intermittency of the system or to a method of forcing leading to sporadic energy-injection events with quiescent periods of decaying turbulence in between (e.g., in galaxy clusters: Roh et al. Reference Roh, Ryu, Kang, Ha and Jang2019). With the arrival of each quiescent period, mergers between the flux ropes should push magnetic energy to larger scales.
The salient bit of theory that is needed to assess this effect is that the magnetic-energy-containing scale will grow with time as a power law during the decay of the turbulence: thus, if the field starts at scale ![]() $\lambda _{\mathrm {R}}$, its scale after a period of decay will be
$\lambda _{\mathrm {R}}$, its scale after a period of decay will be
where ![]() $0<\alpha <1$ in all conceivable circumstances (
$0<\alpha <1$ in all conceivable circumstances (![]() $\alpha =4/9$ for fast-reconnection- controlled decay; see § 12.4.2),
$\alpha =4/9$ for fast-reconnection- controlled decay; see § 12.4.2), ![]() $\tau _{\mathrm {rec}}$ is the characteristic reconnection time (12.9) at the beginning of the decay, with the initial energy-containing scale
$\tau _{\mathrm {rec}}$ is the characteristic reconnection time (12.9) at the beginning of the decay, with the initial energy-containing scale ![]() $\lambda _{\mathrm {R}}$ given by (13.9) and
$\lambda _{\mathrm {R}}$ given by (13.9) and ![]() $B_{\lambda _{\mathrm {R}}}\sim \delta u_L$ (same as the outer-scale velocity field). Suppose the decay is allowed to proceed for about one outer-scale turnover time
$B_{\lambda _{\mathrm {R}}}\sim \delta u_L$ (same as the outer-scale velocity field). Suppose the decay is allowed to proceed for about one outer-scale turnover time ![]() $L/\delta u_L$. The magnetic field's scale after that will be
$L/\delta u_L$. The magnetic field's scale after that will be
If reconnection is fast (Appendix D.6), or stochastic (Appendix D.7), ![]() $\lambda _B$ will have a weaker
$\lambda _B$ will have a weaker ![]() $\mathrm {Rm}_L$ scaling than
$\mathrm {Rm}_L$ scaling than ![]() $\lambda _{\mathrm {R}}$, but it is not a triumph of inverse transfer. Another way to reach the same tepid conclusion is by asking how long it would take to get the magnetic field to the outer scale,
$\lambda _{\mathrm {R}}$, but it is not a triumph of inverse transfer. Another way to reach the same tepid conclusion is by asking how long it would take to get the magnetic field to the outer scale, ![]() $\lambda _B\sim L$. The answer is
$\lambda _B\sim L$. The answer is
 \begin{equation} t \sim \tau_{\mathrm{rec}}\left(\frac{L}{\lambda_{\mathrm{R}}}\right)^{1/\alpha} \sim \frac{L}{\delta u_L}\,\epsilon_{\mathrm{rec}}^{{-}1}\left(\frac{L}{\lambda_{\mathrm{R}}}\right)^{(1-\alpha)/\alpha} \gg \frac{L}{\delta u_L}, \end{equation}
\begin{equation} t \sim \tau_{\mathrm{rec}}\left(\frac{L}{\lambda_{\mathrm{R}}}\right)^{1/\alpha} \sim \frac{L}{\delta u_L}\,\epsilon_{\mathrm{rec}}^{{-}1}\left(\frac{L}{\lambda_{\mathrm{R}}}\right)^{(1-\alpha)/\alpha} \gg \frac{L}{\delta u_L}, \end{equation}quite a long time, as expected, i.e., the forcing would have to be very sporadic to achieve this.
Obviously, all this does not amount to much more than an initial ‘back-of-the-envelope’ assessment, and a more sophisticated treatment might still yield a more pleasing outcome.
13.4.8. Local shear dynamo?
Let me complete my catalogue of speculations regarding the structure of the saturated dynamo state by invoking another piece of dynamo physics that, despite being of potentially fundamental and ubiquitous nature, emerged relatively late in the game. A combination of small-scale turbulence and a large-scale shear generically leads to the emergence of large-scale magnetic field, even when the turbulence has no net helicity – an effect known as the ‘shear dynamo’. This was mooted theoretically in several early mean-field-dynamo schemes and then confirmed numerically by Yousef et al. (Reference Yousef, Heinemann, Schekochihin, Kleeorin, Rogachevskii, Iskakov, Cowley and McWilliams2008b,Reference Yousef, Heinemann, Rincon, Schekochihin, Kleeorin, Rogachevskii, Cowley and McWilliamsc) (see references therein for the precursor theories, numerics and counter-arguments). This result turned out to be due to a form of ‘stochastic ![]() $\alpha$ effect’ (Heinemann, McWilliams & Schekochihin Reference Heinemann, McWilliams and Schekochihin2011; Jingade, Singh & Sridhar Reference Jingade, Singh and Sridhar2018), depending, therefore, on fluctuating helicity in the flow. Interestingly, the shear dynamo turned out to work also when the small-scale turbulence was magnetic, i.e., by the combination of a large-scale shear and the saturated state of small-scale dynamo (Yousef et al. Reference Yousef, Heinemann, Rincon, Schekochihin, Kleeorin, Rogachevskii, Cowley and McWilliams2008a). Squire & Bhattacharjee (Reference Squire and Bhattacharjee2015, Reference Squire and Bhattacharjee2016) made sense of that by discovering semi-analytically the ‘magnetic shear-current effect’ and showing that small-scale magnetic fields were actively helpful in enabling the shear dynamo.
$\alpha$ effect’ (Heinemann, McWilliams & Schekochihin Reference Heinemann, McWilliams and Schekochihin2011; Jingade, Singh & Sridhar Reference Jingade, Singh and Sridhar2018), depending, therefore, on fluctuating helicity in the flow. Interestingly, the shear dynamo turned out to work also when the small-scale turbulence was magnetic, i.e., by the combination of a large-scale shear and the saturated state of small-scale dynamo (Yousef et al. Reference Yousef, Heinemann, Rincon, Schekochihin, Kleeorin, Rogachevskii, Cowley and McWilliams2008a). Squire & Bhattacharjee (Reference Squire and Bhattacharjee2015, Reference Squire and Bhattacharjee2016) made sense of that by discovering semi-analytically the ‘magnetic shear-current effect’ and showing that small-scale magnetic fields were actively helpful in enabling the shear dynamo.
The outcome of § 13.3.3 was a situation in which the outer-scale (![]() $L$) field-stretching motions (plus possibly some sort of kinetic-energy cascade to smaller scales) coexisted with MHD turbulence produced by the break up of the folds, with an effective outer scale
$L$) field-stretching motions (plus possibly some sort of kinetic-energy cascade to smaller scales) coexisted with MHD turbulence produced by the break up of the folds, with an effective outer scale ![]() $\lambda _{\mathrm {R}}\ll L$ (in §§ 13.4.4, 13.4.6, and § 13.4.7, this scale was increased, but remained smaller than
$\lambda _{\mathrm {R}}\ll L$ (in §§ 13.4.4, 13.4.6, and § 13.4.7, this scale was increased, but remained smaller than ![]() $L$). It seems to be an attractive speculation that the combination of this turbulence with the local shears associated with the ‘hydrodynamic’ scales
$L$). It seems to be an attractive speculation that the combination of this turbulence with the local shears associated with the ‘hydrodynamic’ scales ![]() $>\lambda _{\mathrm {R}}$ might act as a local shear dynamo and create ‘local mean fields’ on scales
$>\lambda _{\mathrm {R}}$ might act as a local shear dynamo and create ‘local mean fields’ on scales ![]() $>\lambda _{\mathrm {R}}$. It would be interesting to investigate whether such a mechanism exists and, if it does, whether it can push the magnetic-energy-containing scale closer to
$>\lambda _{\mathrm {R}}$. It would be interesting to investigate whether such a mechanism exists and, if it does, whether it can push the magnetic-energy-containing scale closer to ![]() $L$.
$L$.
To conclude, there are plenty of potential theories – far too many, so no convincing one theory yet. Hero numerics reaching for asymptoticity, and intelligently analysed, might help pare down this field and finally give our understanding of the saturated MHD dynamo a modicum of completeness to match what has been achieved for MHD turbulence with a mean field.
14. The Frontier: kinetic turbulence
We can measure the globula of matter and the distances between them, but Space plasm itself is incomputable.
Vladimir Nabokov, Ada, or Ardor
14.1. Sundry microphysics at low collisionality
I ended the first part of this review with a proclamation in § 8.1 that the story of MHD turbulence looked reasonably complete (before spending five chapters on the loose ends!). Since the main reason for this triumphalism was that MHD cascade finally made sense at the dissipation scales – and the key role in making it make sense belonged to reconnection, a dissipative phenomenon – it is an inevitable complication that microphysics of dissipation may matter. The viscoresistive MHD description adopted here does apply to some natural plasmas, e.g., stellar convective zones or colder parts of accretion discs. These are mostly low-![]() $\mathrm {Pm}$ environments. Whereas I have made an effort to keep all results general and applicable to the high-
$\mathrm {Pm}$ environments. Whereas I have made an effort to keep all results general and applicable to the high-![]() $\mathrm {Pm}$ limit, it is, in fact, quite hard to find naturally occurring high-
$\mathrm {Pm}$ limit, it is, in fact, quite hard to find naturally occurring high-![]() $\mathrm {Pm}$ plasmas for which the standard viscoresistive MHD equations are a good model: this would require the particles’ collision rate to be larger than their Larmor frequency, which rarely happens at high temperatures and low densities needed to achieve high
$\mathrm {Pm}$ plasmas for which the standard viscoresistive MHD equations are a good model: this would require the particles’ collision rate to be larger than their Larmor frequency, which rarely happens at high temperatures and low densities needed to achieve high ![]() $\mathrm {Pm}$ (one exception, quite popular these days, is plasmas created in laser experiments: see, e.g., Bott et al. Reference Bott, Tzeferacos, Chen, Palmer, Rigby, Bell, Bingham, Birkel, Graziani and Froula2021b, Reference Bott, Chen, Tzeferacos, Palmer, Bell, Bingham, Birkel, Froula, Katz and Kunz2022). In fact, most of the interesting (and observed) plasmas in this hot, rarefied category are either ‘dilute’ (an apt term coined by Balbus Reference Balbus2004 to describe plasmas where turbulence is on scales larger than the mean free path, but the Larmor motion is on smaller scales that it – a good example is galaxy clusters; see, e.g., Melville, Schekochihin & Kunz Reference Melville, Schekochihin and Kunz2016 and references therein) or downright collisionless (i.e., everything happens on scales smaller than the mean free path; the most obvious example is the solar wind: see the mega-review by Bruno & Carbone Reference Bruno and Carbone2013 or a human-sized one by Chen Reference Chen2016). In either case, between the ‘ideal-MHD scales’ and the resistive scale, there is a number of other scales at which the physics changes. These changes are of two distinct kinds.
$\mathrm {Pm}$ (one exception, quite popular these days, is plasmas created in laser experiments: see, e.g., Bott et al. Reference Bott, Tzeferacos, Chen, Palmer, Rigby, Bell, Bingham, Birkel, Graziani and Froula2021b, Reference Bott, Chen, Tzeferacos, Palmer, Bell, Bingham, Birkel, Froula, Katz and Kunz2022). In fact, most of the interesting (and observed) plasmas in this hot, rarefied category are either ‘dilute’ (an apt term coined by Balbus Reference Balbus2004 to describe plasmas where turbulence is on scales larger than the mean free path, but the Larmor motion is on smaller scales that it – a good example is galaxy clusters; see, e.g., Melville, Schekochihin & Kunz Reference Melville, Schekochihin and Kunz2016 and references therein) or downright collisionless (i.e., everything happens on scales smaller than the mean free path; the most obvious example is the solar wind: see the mega-review by Bruno & Carbone Reference Bruno and Carbone2013 or a human-sized one by Chen Reference Chen2016). In either case, between the ‘ideal-MHD scales’ and the resistive scale, there is a number of other scales at which the physics changes. These changes are of two distinct kinds.
The first is the appearance of dispersion in the wave physics: Alfvén waves become kinetic Alfvén waves (KAWs), with a different linear response and, therefore, a different variety of critically balanced cascade (Cho & Lazarian Reference Cho and Lazarian2004; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Reference Schekochihin, Kawazura and Barnes2019; Boldyrev & Perez Reference Boldyrev and Perez2012; Boldyrev et al. Reference Boldyrev, Horaites, Xia and Perez2013; Chen & Boldyrev Reference Chen and Boldyrev2017; Passot et al. Reference Passot, Sulem and Tassi2017; Milanese et al. Reference Milanese, Loureiro, Daschner and Boldyrev2020). The culprits here are the ion inertial scale (at which the Hall effect comes in), the ion sound scale (at which the electron-pressure-gradient force becomes important in Ohm's law), and the ion Larmor scale (at which the finite size of ion Larmor orbits starts playing a role). Which of these matters most depends on plasma beta and on the ratio of the ion and electron temperatures, but they all are essentially ion-electron decoupling effects and lead to more or less similar kinds of turbulence, at least in what concerns the KAW cascade. Note that the subviscous regime (§ 11) is, of course, irrelevant for such plasmas – except possibly, in a somewhat exotic way, at high beta (Kawazura et al. Reference Kawazura, Barnes and Schekochihin2019).
The second important modification of MHD is that reconnection in a collisionless plasma need not be done by resistivity, but can be due to other physics that breaks flux conservation, viz., electron inertia, electron finite Larmor radius (FLR) and, more generally, other kinetic features of the electron pressure tensor. Tearing modes are different in such plasmas, with a double ion-electron layer structure and a variety of scalings in a variety of parameter regimes.Footnote 88 Since tearing is important for rounding off the MHD cascade, all these effects must be considered and appropriate modifications worked out for the theory of tearing-mediated turbulence described in § 7 – some of this has been done by Mallet et al. (Reference Mallet, Schekochihin and Chandran2017a) and by Loureiro & Boldyrev (Reference Loureiro and Boldyrev2017a). It is going to be interesting to find out whether, where, and when any of this matters or if perhaps the aligned MHD cascade just segues directly into the KAW cascade (see, however, a discussion in a moment as to what that means). Since there are some mysteries still outstanding with regard to the scale at which the spectrum of solar-wind turbulence has a spectral break between the inertial range and the ‘kinetic’ (KAW) range (Chen et al. Reference Chen, Leung, Boldyrev, Maruca and Bale2014a; Boldyrev et al. Reference Boldyrev, Chen, Xia and Zhdankin2015), perhaps something interesting can be done here (e.g., is the break set by the onset of reconnection, rather than by the Larmor scale? – see Vech et al. Reference Vech, Mallet, Klein and Kasper2018).
Furthermore, KAW turbulence in the kinetic range and its relationship with reconnection is a topic that is rapidly becoming very popular with both numerical modellers (e.g., TenBarge & Howes Reference TenBarge and Howes2013; Bañón Navarro et al. Reference Bañón Navarro, Teaca, Told, Grošelj, Crandall and Jenko2016; Cerri & Califano Reference Cerri and Califano2017; Franci et al. Reference Franci, Cerri, Califano, Landi, Papini, Verdini, Matteini, Jenko and Hellinger2017, Reference Franci, Landi, Verdini, Matteini and Hellinger2018) and observational space physicists (e.g., Greco et al. Reference Greco, Perri, Servidio, Yordanova and Veltri2016). There is a promise of interesting physics – interesting both conceptually and because it is eminently measurable in space. In the context of the prominent role that was given in § 7 to the break up of MHD sheets in setting up the tail end of the MHD cascade, I want to highlight an intriguing suggestion (implicitly) contained in the paper by Cerri & Califano (Reference Cerri and Califano2017) and further fleshed out by Franci et al. (Reference Franci, Cerri, Califano, Landi, Papini, Verdini, Matteini, Jenko and Hellinger2017). They look (numerically) at the formation of current sheets in kinetic turbulence and the disruption of these sheets by tearing (plasmoid) instabilities – and discover that it is precisely these processes that appear to seed the sub-Larmor-scale cascade with a steep (steeper than in the inertial range) energy spectrum usually associated with KAW turbulence. One might wonder then if such a KAW cascade is an entirely distinct phenomenon from a collisionless version of tearing-mediated turbulence. If we allow ourselves to get excited about this question, we might speculate that it rhymes nicely with the idea on which Boldyrev & Perez (Reference Boldyrev and Perez2012) relied to advocate a steeper (![]() $-8/3$) slope of KAW turbulence than the
$-8/3$) slope of KAW turbulence than the ![]() $-7/3$ implied by the standard CB-based theory (Cho & Lazarian Reference Cho and Lazarian2004; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009). They argued that the energetically dominant perturbations at each scale were concentrated in 2D structures, thus making turbulence non-volume-filling (and perhaps monofractal; cf. Kiyani et al. Reference Kiyani, Chapman, Khotyaintsev, Dunlop and Sahraoui2009 and Chen et al. Reference Chen, Sorriso-Valvo, Šafránková and Němeček2014b). While Boldyrev & Perez (Reference Boldyrev and Perez2012) did not appear to think of these 2D structures as reconnecting sheets, an interpretation of them as such does not seem a priori unreasonable. So perhaps this is what happens in collisionless turbulence: sheet-like structures form in the usual (MHD) way, get disrupted by collisionless tearing and/or related instabilities and seed sub-Larmor turbulence,Footnote 89 which stays mostly concentrated in those sheets or their remnants, with an effectively 2D filling fraction. Another possibility – or a version of this scheme – is to abandon the old KAW cascade altogether and declare sub-Larmor turbulence to be entirely controlled by (collisionless) tearing in a similar way to the tearing-mediated cascade of § 7.2, with spectral slopes between
$-7/3$ implied by the standard CB-based theory (Cho & Lazarian Reference Cho and Lazarian2004; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009). They argued that the energetically dominant perturbations at each scale were concentrated in 2D structures, thus making turbulence non-volume-filling (and perhaps monofractal; cf. Kiyani et al. Reference Kiyani, Chapman, Khotyaintsev, Dunlop and Sahraoui2009 and Chen et al. Reference Chen, Sorriso-Valvo, Šafránková and Němeček2014b). While Boldyrev & Perez (Reference Boldyrev and Perez2012) did not appear to think of these 2D structures as reconnecting sheets, an interpretation of them as such does not seem a priori unreasonable. So perhaps this is what happens in collisionless turbulence: sheet-like structures form in the usual (MHD) way, get disrupted by collisionless tearing and/or related instabilities and seed sub-Larmor turbulence,Footnote 89 which stays mostly concentrated in those sheets or their remnants, with an effectively 2D filling fraction. Another possibility – or a version of this scheme – is to abandon the old KAW cascade altogether and declare sub-Larmor turbulence to be entirely controlled by (collisionless) tearing in a similar way to the tearing-mediated cascade of § 7.2, with spectral slopes between ![]() $-3$ and
$-3$ and ![]() $-8/3$, still consistent with observations and simulations (Loureiro & Boldyrev Reference Loureiro and Boldyrev2017a; Boldyrev & Loureiro Reference Boldyrev and Loureiro2019).
$-8/3$, still consistent with observations and simulations (Loureiro & Boldyrev Reference Loureiro and Boldyrev2017a; Boldyrev & Loureiro Reference Boldyrev and Loureiro2019).
What I have said about kinetic physics so far might not sound like a true conceptual leap: basically, at small scales, we have different linear physics and a zoo of possibilities, depending on parameter regimes; one could work productively on porting some of the basic ideas developed in the preceding sections to these situations.Footnote 90 There are, however, ways in which kinetic physics does bring in something altogether new. Four examples of that, chosen in a very biased way, are given in what follows.
14.2. Cascade fragility
FLR effects do not just change how linear waves propagate at sub-Larmor scales. They also change the nature of the second conserved quantity (the first being energy) possessed by the plasma: (R)MHD cross-helicity (imbalance) becomes magnetic helicity in the transition from the inertial to the sub-Larmor scale range. The trouble is that the KAW helicity is a quantity that naturally wants to cascade inversely, from small scales to large (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Cho Reference Cho2011; Kim & Cho Reference Kim and Cho2015; Cho & Kim Reference Cho and Kim2016; Miloshevich et al. Reference Miloshevich, Laveder, Passot and Sulem2021). In low-beta plasmas, there is no dissipation at the ion Larmor scale (Schekochihin et al. Reference Schekochihin, Kawazura and Barnes2019), so an imbalanced cascade arriving from the inertial range would get thoroughly ‘confused’ by the sudden need to reverse the direction of the helicity cascade. The result, it turns out, is a mighty blow back from the small scales to large and a failure to achieve a constant-flux steady state (see figure 35, taken from Meyrand et al. Reference Meyrand, Squire, Schekochihin and Dorland2021; some evidence of strange behaviour of energy fluxes in imbalanced solar-wind turbulence does appear to exist: see, e.g., Smith et al. Reference Smith, Stawarz, Vasquez, Forman and MacBride2009). The imbalanced cascades are fragile.

Figure 35. Spectra of Elsasser field for (c) heavily imbalanced RMHD turbulence (![]() $\varepsilon ^+/\varepsilon ^-\approx 16$), (a) similarly imbalanced turbulence in an RMHD-like model of a low-beta collisionless plasma with FLR effects, and (b) balanced turbulence in the same model. Purple-to-yellow colour scale shows time evolution of the spectra. The spectrum of the stronger Elsasser field in (a) does not reach a steady state, with spectral break moving to larger scales. Measurement of energy fluxes in these simulations shows that (a) is not a constant-flux solution, whereas (b) is. These results are from Meyrand et al. (Reference Meyrand, Squire, Schekochihin and Dorland2021).
$\varepsilon ^+/\varepsilon ^-\approx 16$), (a) similarly imbalanced turbulence in an RMHD-like model of a low-beta collisionless plasma with FLR effects, and (b) balanced turbulence in the same model. Purple-to-yellow colour scale shows time evolution of the spectra. The spectrum of the stronger Elsasser field in (a) does not reach a steady state, with spectral break moving to larger scales. Measurement of energy fluxes in these simulations shows that (a) is not a constant-flux solution, whereas (b) is. These results are from Meyrand et al. (Reference Meyrand, Squire, Schekochihin and Dorland2021).
What this means for the real plasmas that these models aspire to describe (low beta, high Elsasser imbalance – typical solar-wind conditions close to the Sun, currently sampled with gusto by the Parker Solar Probe; see, e.g., Chen et al. Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020) is that the fluid approximation is broken – not just in the sense of requiring FLR bolt-ons, but in the sense that the system cannot accommodate a steady-state turbulent cascade while still respecting the ![]() $k_{\parallel }\ll k _{\perp }$ and low-frequency (viz.,
$k_{\parallel }\ll k _{\perp }$ and low-frequency (viz., ![]() $\omega \ll$ the ion cyclotron frequency) ordering associated with the gyrokinetic approximation and its various fluid-like reductions (Howes et al. Reference Howes, Cowley, Dorland, Hammett, Quataert and Schekochihin2006; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Reference Schekochihin, Kawazura and Barnes2019). A fully kinetic system deals with this problem by building up energy in the stronger Elsasser field and, therefore, shortening the nonlinear cascade times
$\omega \ll$ the ion cyclotron frequency) ordering associated with the gyrokinetic approximation and its various fluid-like reductions (Howes et al. Reference Howes, Cowley, Dorland, Hammett, Quataert and Schekochihin2006; Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Reference Schekochihin, Kawazura and Barnes2019). A fully kinetic system deals with this problem by building up energy in the stronger Elsasser field and, therefore, shortening the nonlinear cascade times ![]() $\tau _{\mathrm {nl}}$, until critical balance pushes
$\tau _{\mathrm {nl}}$, until critical balance pushes ![]() $k_{\parallel }$ high enough to break the gyrokinetic approximation and open up a new dissipation channel via the ion-cyclotron resonance – a happy reconciliation, it seems, between ample observational evidence both for ion-cyclotron heating and for low-frequency cascades in the solar wind (Squire et al. Reference Squire, Meyrand, Kunz, Arzamasskiy, Schekochihin and Quataert2022).
$k_{\parallel }$ high enough to break the gyrokinetic approximation and open up a new dissipation channel via the ion-cyclotron resonance – a happy reconciliation, it seems, between ample observational evidence both for ion-cyclotron heating and for low-frequency cascades in the solar wind (Squire et al. Reference Squire, Meyrand, Kunz, Arzamasskiy, Schekochihin and Quataert2022).
This is a relatively rare example of plasma microphysics seriously upsetting system-scale macrophysics (another possible set of such examples is flagged in § 14.5). Sadly, this renders much of § 9 almost completely irrelevant for such plasmas – unless an effective way can be found of modifying the dissipation-scale pinning scheme (§ 9.6.4) to account for the kinetic dissipation mechanism described above.
14.3. Phase-space turbulence
What is turbulence? Some energy is injected into some part of the phase space of a nonlinear system (in fluid systems, that simply means position or wavenumber space), which is, generally speaking, not the part of the phase space where it can be efficiently thermalised. So turbulence is a process whereby this energy finds its way from where it is injected to where it can be dissipated, and its means of doing this is nonlinear coupling, usually from large to small scales (I am now putting to one side the upsetting example of the failure of this process discussed in § 14.2). What kind of coupling is possible and at what rate the energy can be transferred from scale to scale then determines such things as energy spectra in a stationary state with a constant flux of energy.
The same principle applies to kinetic turbulence, but now the phase space is 6D rather than 3D: the particle distribution depends on positions and velocities, and energy transfer can be from large to small scales (or vice versa) in all six coordinates. The transfer of (free) energy to small scales in velocity space, leading ultimately to activation of collisions, however small the collision rate, is known as ‘phase mixing’. It is not always a nonlinear phenomenon: the simplest (although not necessarily very simple) phase-mixing process is the linear Landau (Reference Landau1946) damping. In a magnetised plasma, this is the parallel (to ![]() $\boldsymbol {B}_0$) phase mixing, whereas the perpendicular phase mixing is nonlinear and has to do with particles on Larmor orbits experiencing different electromagnetic fields depending on the radius of the orbit (the Larmor radius is a kinetic variable, being proportional to
$\boldsymbol {B}_0$) phase mixing, whereas the perpendicular phase mixing is nonlinear and has to do with particles on Larmor orbits experiencing different electromagnetic fields depending on the radius of the orbit (the Larmor radius is a kinetic variable, being proportional to ![]() $v_{\perp }$). The latter phenomenon leads to an interesting phase-space ‘entropy cascade’ (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Plunk, Quataert and Tatsuno2008, Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Tatsuno et al. Reference Tatsuno, Dorland, Schekochihin, Plunk, Barnes, Cowley and Howes2009; Plunk et al. Reference Plunk, Cowley, Schekochihin and Tatsuno2010; Cerri, Kunz & Califano Reference Cerri, Kunz and Califano2018; Eyink Reference Eyink2018; Kawazura et al. Reference Kawazura, Barnes and Schekochihin2019; cf. Pezzi et al. Reference Pezzi, Servidio, Perrone, Valentini, Sorriso-Valvo, Greco, Matthaeus and Veltri2018), which is one of the more exotic phenomena that await a curious researcher at sub-Larmor scales. Its importance in the grand scheme of things is that it channels turbulent energy into ion heat, while the KAW cascade heats electrons – the question of which dissipation channel is the more important one, and when, being both fundamental and ‘applied’(in the astrophysical sense of the word – e.g., to accretion flows: see Quataert & Gruzinov Reference Quataert and Gruzinov1999, Event Horizon Telescope Collaboration 2019). Understanding how energy is transferred between scales in phase space requires thinking somewhat outside the standard turbulence paradigm and so perhaps counts as conceptual novelty. Not much of it has been done so far and it is worth doing more.
$v_{\perp }$). The latter phenomenon leads to an interesting phase-space ‘entropy cascade’ (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Plunk, Quataert and Tatsuno2008, Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Tatsuno et al. Reference Tatsuno, Dorland, Schekochihin, Plunk, Barnes, Cowley and Howes2009; Plunk et al. Reference Plunk, Cowley, Schekochihin and Tatsuno2010; Cerri, Kunz & Califano Reference Cerri, Kunz and Califano2018; Eyink Reference Eyink2018; Kawazura et al. Reference Kawazura, Barnes and Schekochihin2019; cf. Pezzi et al. Reference Pezzi, Servidio, Perrone, Valentini, Sorriso-Valvo, Greco, Matthaeus and Veltri2018), which is one of the more exotic phenomena that await a curious researcher at sub-Larmor scales. Its importance in the grand scheme of things is that it channels turbulent energy into ion heat, while the KAW cascade heats electrons – the question of which dissipation channel is the more important one, and when, being both fundamental and ‘applied’(in the astrophysical sense of the word – e.g., to accretion flows: see Quataert & Gruzinov Reference Quataert and Gruzinov1999, Event Horizon Telescope Collaboration 2019). Understanding how energy is transferred between scales in phase space requires thinking somewhat outside the standard turbulence paradigm and so perhaps counts as conceptual novelty. Not much of it has been done so far and it is worth doing more.
Returning to parallel phase mixing, this too turns out to be interesting in a nonlinear setting, even though it is a linear phenomenon itself. First theoretical (Schekochihin et al. Reference Schekochihin, Parker, Highcock, Dellar, Dorland and Hammett2016; Adkins & Schekochihin Reference Adkins and Schekochihin2018) and numerical (Parker et al. Reference Parker, Highcock, Schekochihin and Dellar2016; Meyrand et al. Reference Meyrand, Kanekar, Dorland and Schekochihin2019) analyses suggest that, in a turbulent system, parallel phase mixing is effectively suppressed by the stochastic plasma echo, perhaps rendering kinetic systems that are notionally subject to Landau damping effectively fluid, at least in terms of their energy-flow budgets. In the context of inertial-range MHD turbulence, this is relevant to the compressive (‘slow-mode’) perturbations, which, in a collisionless plasma, are energetically decoupled from, and nonlinearly slaved to, the Alfvénic ones, while the latter are still governed by RMHD (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015). Linearly, these compressive perturbations must be damped – but nonlinearly they are not (Meyrand et al. Reference Meyrand, Kanekar, Dorland and Schekochihin2019), thus accounting for them exhibiting a healthy power-law spectrum and other fluid features in the solar wind (Chen Reference Chen2016; Verscharen, Chen & Wicks Reference Verscharen, Chen and Wicks2017). In this vein, one might also ask whether the Landau damping of KAWs at sub-Larmor scales is always efficient or even present at all – and if it is, as TenBarge & Howes (Reference TenBarge and Howes2013), Bañón Navarro et al. (Reference Bañón Navarro, Teaca, Told, Grošelj, Crandall and Jenko2016), Kobayashi et al. (Reference Kobayashi, Sahraoui, Passot, Laveder, Sulem, Huang, Henri and Smets2017), and Chen, Klein & Howes (Reference Chen, Klein and Howes2019) all say, then what is different at these scales. Given that Loureiro, Schekochihin & Zocco (Reference Loureiro, Schekochihin and Zocco2013b) see a characteristic signature of phase mixing in collisionless reconnection, reconnection might yet again turn out to be the key player, as indeed it has been conjectured to be at these scales (see § 14.1).
The broader question is whether there is generally Landau damping in turbulent systems and whether, therefore, to put it crudely, ‘all turbulence is fluid’. While it might be a little disappointing if it is, the way and the sense in which this seems to be achieved are surprising and pleasingly non-trivial – and possibly soon to be amenable to direct measurement if the first MMS results on velocity-space (Hermite) spectra in the Earth's magnetosheath (Servidio et al. Reference Servidio, Chasapis, Matthaeus, Perrone, Valentini, Parashar, Veltri, Gershman, Russell and Giles2017) are a good indication of the possibilities that are opening up.
14.4. Statistical thermodynamics of collisionless plasma
At quite uncertain times and places,
The atoms left their heavenly path,
And by fortuitous embraces,
Engendered all that being hath.
And though they seem to cling together,
And form “associations” here,
Yet, soon or late, they burst their tether,
And through the depths of space career.
J. C. Maxwell, Molecular Evolution
Phase-space turbulence is interesting not just in its own right, but also as a means to answering an even broader, and trickier, question, or class of questions, which concerns the equilibria underlying the fluid-like (or kinetic) dynamics of plasma turbulence. The meaning of the word ‘thermalised’, which I used to describe the fate of turbulent energy at the beginning of § 14.3, is really only fully clear if the underlying equilibrium is Maxwellian – i.e., if significant departures from thermal equilibrium occur, and, therefore, a kinetic description is required, only for the turbulent fluctuations. This is certainly not the case for many natural plasmas, where the overall distribution functions both of ions and of electrons, can be order-unity non-Maxwellian – the prime and most accessible example of that being the solar wind (e.g., Marsch Reference Marsch2006, Reference Marsch2018; Martinović et al. Reference Martinović, Klein, Kasper, Case, Korreck, Larson, Livi, Stevens, Whittlesey and Chandran2020). A number of questions then arise, all of them both unsolved and extremely fundamental on a level that should appeal to any physicist:
(i) Do universal (i.e., not sensitive in detail to initial conditions) collisionless equilibria exist?
(ii) If they do, is it possible to find them by constructing a statistical thermodynamics of collisionless plasma based on such staples as the maximum-entropy principle? And if so, what is the correct definition of entropy for a collisionless plasma (cf. Fowler Reference Fowler1968; Eyink Reference Eyink2018; Matthaeus et al. Reference Matthaeus, Yang, Wan, Parashar, Bandyopadhyay, Chasapis, Pezzi and Valentini2020; Zhdankin Reference Zhdankin2022a; Chavanis Reference Chavanis2021; Ewart et al. Reference Ewart, Brown, Adkins and Schekochihin2022)?
(iii) Is it then possible to have a theory of plasma relaxation to these equilibria in terms of some ‘collisionless collision integrals’? What is the relaxation rate (i.e., the ‘effective collision rate’)? It is at this step that the structure of phase-space turbulence, which was flagged as interesting in its own right in § 14.3, comes in as an essential ingredient: in the same way as the ‘fluid’ theory of turbulent transport in, e.g., a gyrokinetic plasma, requires knowledge of the statistical properties of turbulence, which determine turbulent fluxes of conserved ‘fluid’ quantities (e.g., Abel et al. Reference Abel, Plunk, Wang, Barnes, Cowley, Dorland and Schekochihin2013), the kinetic theory of collisionless relaxation requires one to know the phase-space correlation function of the perturbed distribution function, which determines the turbulent flux of phase density (Kadomtsev & Pogutse Reference Kadomtsev and Pogutse1970; Chavanis Reference Chavanis2021; Ewart et al. Reference Ewart, Brown, Adkins and Schekochihin2022).
(iv) And if all, or some, of the above is accomplished, how can one then describe the passage of energy from turbulent fluctuations into the equilibrium distribution (‘thermalisation’)Footnote 91 in terms of some generalised free-energy cascade (one example of how one might approach this question can be gleaned from a comparison between Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, where a Maxwellian equilibrium is assumed, and Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015, Reference Kunz, Abel, Klein and Schekochihin2018, where the equilibrium is allowed to be pressure-anisotropic).
These questions are not new – there was some very interesting activity around them half a century ago (e.g., Fowler Reference Fowler1968; Kadomtsev & Pogutse Reference Kadomtsev and Pogutse1970; Dupree Reference Dupree1972), which produced a flurry of follow-ups, but the subject has since gone into abeyance, due, in large part, to the impossibility at the time of numerical or observational testing of any of the theories. This is now changing: computers are big enough to handle the vastness of the 6D phase space, and the heliosphere is teeming with spacecraft full of eager and discerning instruments. This is a exciting research frontier if there has ever been one. Here is not the place to review this topic in any further detail – my own take on it, as pedagogical as I can manage, alongside a more complete set of references, can be found in Schekochihin (Reference Schekochihin2022).
14.5. Macro- and microphysical consequences of pressure anisotropy
Another line of inquiry pregnant with conceptual novelty concerns the effect of self-generated pressure anisotropy on MHD dynamics. Pressure anisotropies are generated in response to any motion in a magnetised collisionless or weakly collisional plasma as long as this motion leads to a change in the strength of the magnetic field. The conservation of the magnetic moment (![]() $\propto v_{\perp }^2/B\propto$ the angular momentum of Larmor-gyrating particles) then causes positive (if the field grows) or negative (if it decreases) pressure anisotropy to arise (see, e.g., Schekochihin et al. Reference Schekochihin, Cowley, Rincon and Rosin2010). This is usually quite small, but it becomes relevant at high beta, when even small anisotropies (of order
$\propto v_{\perp }^2/B\propto$ the angular momentum of Larmor-gyrating particles) then causes positive (if the field grows) or negative (if it decreases) pressure anisotropy to arise (see, e.g., Schekochihin et al. Reference Schekochihin, Cowley, Rincon and Rosin2010). This is usually quite small, but it becomes relevant at high beta, when even small anisotropies (of order ![]() $1/\beta$) can have a dramatic effect, in two ways. Dynamically, pressure anisotropy supplies additional stress, which, when the anisotropy is negative (
$1/\beta$) can have a dramatic effect, in two ways. Dynamically, pressure anisotropy supplies additional stress, which, when the anisotropy is negative (![]() $p_{\perp }< p_{\parallel }$), can cancel Maxwell's stress and thus remove magnetic tension – the simplest way to think of this is in terms of the Alfvén speed being modified so:
$p_{\perp }< p_{\parallel }$), can cancel Maxwell's stress and thus remove magnetic tension – the simplest way to think of this is in terms of the Alfvén speed being modified so:
Kinetically, pressure anisotropy is a source of free energy and will trigger fast, small-scale instabilities, most notably mirror and firehose (see Kunz, Schekochihin & Stone Reference Kunz, Schekochihin and Stone2014 and references therein). The firehose corresponds to the Alfvén speed (14.1) turning imaginary, i.e., it is an instability caused by negative tension; the mirror is not quite as simple to explain, but is fundamentally a result of effective magnetic pressure going negative by means of some subtle resonant-particle dynamics (see Southwood & Kivelson Reference Southwood and Kivelson1993, Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015 and references therein). These instabilities in turn can regulate the anisotropy by scattering particles or by subtler, more devious means (see Melville et al. Reference Melville, Schekochihin and Kunz2016 and references therein).
Investigating of the dynamics of a simple finite-amplitude Alfvén wave in a collisionless, high-beta plasma, Squire et al. (Reference Squire, Quataert and Schekochihin2016, Reference Squire, Schekochihin and Quataert2017b,Reference Squire, Kunz, Quataert and Schekochihinc) showed that both of these effects did occur and altered the wave's behaviour drastically: it first slows down to a near halt due to the removal of magnetic tension, transferring much of its kinetic energy into heat and then, having spawned a colony of particle-scattering Larmor-scale perturbations, dissipates as if it were propagating in a plasma with a large Braginskii (Reference Braginskii1965) parallel viscosity. Sound waves in a collisionless plasma get similarly infested by firehoses and mirrors, except the resulting effective collisionality helps them propagate in a medium that they thus render more fluid and, therefore, incapable of Landau damping (a different mechanism than discussed in § 14.3, but a similar outcome; see Kunz et al. Reference Kunz, Squire, Schekochihin and Quataert2020).
These effects occur provided the amplitude of the waves is above a certain limit that scales with plasma beta: this is because pressure anisotropy must be large enough to compete with tension in (14.1) and the amount of anisotropy that can be generated is of the order of the field-strength perturbation. For an Alfvén wave, the latter is quadratic in the wave's amplitude:
In formal terms, this means that in high-beta collisionless plasmas, the small-amplitude and high-beta limits do not commute. The conventional picture of Alfvénic turbulence simply obeying RMHD equations, even in a collisionless plasma (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009; Kunz et al. Reference Kunz, Schekochihin, Chen, Abel and Cowley2015), may then have to be seriously revised for such environments as galaxy clusters, for example, where ![]() $\beta \sim 10^2$ (Schekochihin & Cowley Reference Schekochihin and Cowley2006) (a first step in this direction has been taken by Squire et al. Reference Squire, Schekochihin, Quataert and Kunz2019, who found that MHD turbulence with Braginskii viscosity, while looking in many respects similar to the usual Alfvénic turbulence, nevertheless manages to minimise changes in the magnetic-field strength to a much greater extent – a property they dubbed ‘magneto-immutability’; cf. Tenerani & Velli Reference Tenerani and Velli2020a, who do not like the term, but explore useful dynamical scenarios for achieving just such a state).Footnote 92
$\beta \sim 10^2$ (Schekochihin & Cowley Reference Schekochihin and Cowley2006) (a first step in this direction has been taken by Squire et al. Reference Squire, Schekochihin, Quataert and Kunz2019, who found that MHD turbulence with Braginskii viscosity, while looking in many respects similar to the usual Alfvénic turbulence, nevertheless manages to minimise changes in the magnetic-field strength to a much greater extent – a property they dubbed ‘magneto-immutability’; cf. Tenerani & Velli Reference Tenerani and Velli2020a, who do not like the term, but explore useful dynamical scenarios for achieving just such a state).Footnote 92
In situations where the inequality (14.2) is not satisfied, but the pressure anisotropy is a feature of the equilibrium, it seems we can live with the current theory as long as ![]() $\beta$ is not too large. This is the case for most instances of the solar wind, where negative pressure anisotropy is driven by expansion away from the Sun (so
$\beta$ is not too large. This is the case for most instances of the solar wind, where negative pressure anisotropy is driven by expansion away from the Sun (so ![]() $B$ decreases with heliocentric distance) and
$B$ decreases with heliocentric distance) and ![]() $\beta$ ranges from
$\beta$ ranges from ![]() $\ll 1$ closer to the Sun to
$\ll 1$ closer to the Sun to ![]() $\gtrsim 1$ in our own neighbourhood. A recent investigation by Bott et al. (Reference Bott, Arzamasskiy, Kunz, Quataert and Squire2021a) of Alfvénic turbulence in an expanding box (mimicking the solar wind) at
$\gtrsim 1$ in our own neighbourhood. A recent investigation by Bott et al. (Reference Bott, Arzamasskiy, Kunz, Quataert and Squire2021a) of Alfvénic turbulence in an expanding box (mimicking the solar wind) at ![]() $\beta \approx 2-4$ found that a kinetic version of the firehose instability, which, at these
$\beta \approx 2-4$ found that a kinetic version of the firehose instability, which, at these ![]() $\beta$ (unlike at
$\beta$ (unlike at ![]() $\beta \gg 1$), kicks in at a (negative) value of
$\beta \gg 1$), kicks in at a (negative) value of ![]() $p_{\perp }-p_{\parallel }$ order-unity short of zeroing out the magnetic tension, generated a sea of microscale magnetic perturbations that, by scattering particles, adjusted the effective collisionality of the plasma to keep it exactly marginal to that kinetic firehose; the critically balanced, Alfvénic cascade survived unscathed, albeit with the Alfvén speed modified according to (14.1). The (potentially) more interesting things that happen at
$p_{\perp }-p_{\parallel }$ order-unity short of zeroing out the magnetic tension, generated a sea of microscale magnetic perturbations that, by scattering particles, adjusted the effective collisionality of the plasma to keep it exactly marginal to that kinetic firehose; the critically balanced, Alfvénic cascade survived unscathed, albeit with the Alfvén speed modified according to (14.1). The (potentially) more interesting things that happen at ![]() $\beta \gg 1$ are costlier to tackle numerically, but, it seems, soon will be.
$\beta \gg 1$ are costlier to tackle numerically, but, it seems, soon will be.
Existing understanding of another basic high-beta MHD process, the small-scale dynamo, which I discussed at length in § 13, is also potentially endangered by ubiquitous pressure anisotropies – but has survived the first contact with direct numerical experimentation, which required extra-large, ‘hero’ kinetic simulations (Rincon et al. Reference Rincon, Califano, Schekochihin and Valentini2016; Kunz et al. Reference Kunz, Stone and Quataert2016; St-Onge & Kunz Reference St-Onge and Kunz2018).Footnote 93 So far it appears that in this problem as well, changing magnetic fields render plasma more collisional in some effective sense and so large-![]() $\mathrm {Pm}$ dynamo remains a relevant paradigm. The same conclusion was reached by St-Onge et al. (Reference St-Onge, Kunz, Squire and Schekochihin2020), who simulated dynamo action and saturation in MHD with Braginskii stress (the collisional limit of pressure-anisotropic dynamics).
$\mathrm {Pm}$ dynamo remains a relevant paradigm. The same conclusion was reached by St-Onge et al. (Reference St-Onge, Kunz, Squire and Schekochihin2020), who simulated dynamo action and saturation in MHD with Braginskii stress (the collisional limit of pressure-anisotropic dynamics).
This line of investigation may be particularly rich in surprises because pressure- anisotropy stress undermines much of our basic intuition for ideal-MHD dynamics, not just modifies microscale plasma physics. This said, it is not entirely inconceivable that, at the end of the day (or of the decade), in some grossly coarse-grained sense, turbulent plasmas will just turn out to supply their own effective collisionality even where Coulomb collisions are rare – and so astrophysicists, with their focus on large-scale motions, need not be too worried about the validity of fluid models beyond requiring a few easily implementable tweaks. I hope life is not quite so boring, although, as a theoretical physicist and, therefore, a believer in universality, I should perhaps expect to be pleased by such an outcome.
15. Conclusion
Let us stop here. The story of MHD turbulence is a fascinating one – both the story of what happens physically and the story of how it has been understood. It is remarkable how long it takes to figure out simple things, obvious in retrospect. It is even more remarkable (and reassuring) that we get there after all, in finite time. This story now looks reasonably complete, at least in broad-brush outline (§ 8.1) and modulo numerous caveats and many interesting loose ends (§§ 9–13). Is this an illusion? Is it all wrong again? We shall know soon enough, but in the meanwhile, the siren call of kinetic physics is too strong to resist and the unexplored terrain seems vast and fertile (§ 14). Is everything different there? Or will it all, in the end, turn out to be the same, with nature proving itself a universalist bore and contriving to supply effective collisions where nominally there are few? Is turbulence always basically fluid or do subtle delights await us in phase space? Even if we are in danger of being disappointed by the answers to these questions, getting there is proving to be a journey of amusing twists and turns.
Acknowledgements
For a topic as broad as this, it is difficult to list all the people from whom I have learned what I know (or think I know) of this subject. The most important such influence has been Steve Cowley. The views expressed in the first part of this paper (§§ 5–7) were informed largely by my collaboration with Alfred Mallet and Ben Chandran and by conversations with Andrey Beresnyak (even if he is likely to disagree with my conclusions), Nuno Loureiro and Dmitri Uzdensky. I have learned most of what I know of reconnection from Nuno and Dmitri and of the solar wind from Chris Chen, Tim Horbury, and Rob Wicks. I owe the first epigraph of this paper to the erudition of Richard McCabe and the second (as well as the epigraph of § 7) to that of Matt Kunz. The sections on weak turbulence (§ 4, Appendix A and especially Appendix A.4) would have been different had Thomas Foster not convinced me to make a modicum of peace with the traditional theory. Ben Chandran, Nuno Loureiro, and Andrey Beresnyak have also helped me think coherently of imbalanced turbulence – without necessarily endorsing the outcome (§ 9). David Hosking demolished comprehensively my first version of § 12 (on decaying MHD turbulence) and forced a complete rewrite – in the process, he wrote what I consider to be one of the more beautiful papers that I have had the good fortune of being involved with (Hosking & Schekochihin Reference Hosking and Schekochihin2021). The contents of § 13 were inspired by discussions with Andrey Beresnyak, Alisa Galishnikova, François Rincon, and Matt Kunz, re-examining my views on small-scale dynamo that had been formed in the early 2000s. The views expressed in § 14 are in large measure a result of my recent and current excursions to the kinetic frontier in the company of Toby Adkins, Lev Arzamasskiy, Michael Barnes, Archie Bott, Andrew Brown, Bill Dorland, Robbie Ewart, Yohei Kawazura, Matt Kunz, Romain Meyrand, Michael Nastac, Eliot Quataert, and Jono Squire. Besides the colleagues and friends mentioned above, conversations with Allen Boozer, Axel Brandenburg, Mike Brown, Peter Davidson, Daniele Del Sarto, Greg Eyink, Henrik Latter, Alex Lazarian, Sergey Nazarenko, Maurizio Ottaviani, Felix Parra, Marco Velli, Mahendra Verma, and Muni Zhou have helped me work out what to say, and how to say it, in various bits of this review. I would also like to thank the authors whose figures appear in the text for giving me permission to reproduce their art. I hasten to add that none of those mentioned here bear any responsibility for my many enduring confusions and biases. If, nevertheless, this paper occasionally manages to make sense to its readers, its six referees deserve some significant share of credit for that – they all provided reviews both spirited and helpful, ranging from spotting multitudinous typos to calling out preposterous stylistic bloopers to identifying important logical pitfalls, and even, in one instance, offering detailed suggestions as to the most appropriate wine selection to go with each section.
Visits to a number of pleasant places have helped bring this work to completion. I am delighted to acknowledge the hospitality of the Wolfgang Pauli Institute, Vienna, where, in meetings held annually for 12 years (2007-19), many key interactions took place and ideas were hatched. This paper started as an ‘opinion piece’ written for the 1st JPP Frontiers of Plasma Physics Conference at the Abbazia di Spineto in 2017, when the news of tearing-mediated turbulence was very fresh. My extended stay in 2018 at the Niels Bohr International Academy, Copenhagen, where some non-trivial i's were dotted and t's crossed, was supported by the Simons Foundation (via Martin Pessah, to whom I am grateful for offering me NBIA's hospitality). Another place whose hospitality, in 2019, proved germane to making progress was the Kavli Institute of Theoretical Physics, Santa Barbara, during its programme on ‘Multiscale Phenomena in Plasma Astrophysics’ led by Anatoly Spitkovsky. The manuscript was finally finished during the first COVID-19 lockdown (in 2020) and revised during the second (2021), but I offer no thanks to the virus.
Editor William Dorland thanks the referees for their advice in evaluating this article.
Funding
This work was supported in part by grants from STFC (ST/N000919/1 and ST/W000903/1) and EPSRC (EP/M022331/1 and EP/R034737/1).
Declaration of interests
The author reports no conflict of interest.
APPENDICES
Appendix A. Splendours and miseries of WT theory
A.1. RMHD in scalar form
It is convenient to rewrite the RMHD equations (3.1) in terms of two scalar fields, so-called Elsasser potentials ![]() $\zeta ^\pm$, which are the stream functions for the 2D-solenoidal fields
$\zeta ^\pm$, which are the stream functions for the 2D-solenoidal fields ![]() $\boldsymbol {Z}_{\perp }^\pm$ (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009), viz.,
$\boldsymbol {Z}_{\perp }^\pm$ (Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009), viz.,
where ![]() $\hat {\boldsymbol {z}} = \boldsymbol {B}_0/B_0$. Then
$\hat {\boldsymbol {z}} = \boldsymbol {B}_0/B_0$. Then ![]() $\zeta ^\pm$ satisfy, as shown by taking the curl of (3.1) and using (A1),
$\zeta ^\pm$ satisfy, as shown by taking the curl of (3.1) and using (A1),
where ![]() $\omega ^\pm = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^\pm ) = \nabla _{\perp }^2\zeta ^\pm$ are Elsasser vorticities, all dissipative terms have been dropped, and
$\omega ^\pm = \hat {\boldsymbol {z}}\cdot (\boldsymbol {\nabla }_{\perp }\times \boldsymbol {Z}_{\perp }^\pm ) = \nabla _{\perp }^2\zeta ^\pm$ are Elsasser vorticities, all dissipative terms have been dropped, and
Note that I have written (A2) in a slightly different (but equivalent) form than in Schekochihin et al. (Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009). The present version emphasises that the two physical influences of the nonlinearity on the Elsasser vorticities are advection by the other Elsasser field ![]() $\boldsymbol {Z}_{\perp }^\mp$ (the first term on the right-hand side) and ‘vortex stretching’ (the second term) (cf. Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b) – this is a useful way to write these equations for, e.g., the argument in § 10.4 about the build-up of negative correlations between
$\boldsymbol {Z}_{\perp }^\mp$ (the first term on the right-hand side) and ‘vortex stretching’ (the second term) (cf. Zhdankin et al. Reference Zhdankin, Boldyrev and Uzdensky2016b) – this is a useful way to write these equations for, e.g., the argument in § 10.4 about the build-up of negative correlations between ![]() $\omega ^+$ and
$\omega ^+$ and ![]() $\omega ^-$ (residual energy).
$\omega ^-$ (residual energy).
In Fourier space, (A2) has a nicely generic form
with the coupling coefficients
where ![]() $\phi$ is the angle between
$\phi$ is the angle between ![]() ${\boldsymbol {k}}_{\perp }$ and
${\boldsymbol {k}}_{\perp }$ and ![]() ${\boldsymbol {q}}_{\perp }$.
${\boldsymbol {q}}_{\perp }$.
A.2. Classic WT calculation
Our objective is to derive an evolution equation for the spectra ![]() $C^\pm _{\boldsymbol {k}} = \left \langle |\zeta ^\pm _{\boldsymbol {k}}|^2\right \rangle$. Multiplying (A4) by
$C^\pm _{\boldsymbol {k}} = \left \langle |\zeta ^\pm _{\boldsymbol {k}}|^2\right \rangle$. Multiplying (A4) by ![]() $\zeta ^{\pm *}_{\boldsymbol {k}}$ and adding to the resulting equation its complex conjugate, we get
$\zeta ^{\pm *}_{\boldsymbol {k}}$ and adding to the resulting equation its complex conjugate, we get
Similarly, the evolution equation for the triple correlator appearing in the right-hand side of (A6) is
 \begin{align} \partial_t \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \mp i2p_{{\parallel}}v_{\mathrm{A}} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle =& \sum_{{\boldsymbol{k}}'{\boldsymbol{k}}''} \left[ M_{{\boldsymbol{p}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{p}},{\boldsymbol{k}}'+{\boldsymbol{k}}''} \left\langle\zeta^\pm_{{\boldsymbol{k}}'}\zeta^\mp_{{\boldsymbol{k}}''}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \right. \nonumber\\ &\quad\left. +\, M_{{\boldsymbol{q}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{q}},{\boldsymbol{k}}'+{\boldsymbol{k}}''}\right. \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\mp_{{\boldsymbol{k}}'}\zeta^\pm_{{\boldsymbol{k}}''}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \nonumber\\ &\quad\left. +\, M_{{\boldsymbol{k}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{k}},{\boldsymbol{k}}'+{\boldsymbol{k}}''} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{{\boldsymbol{k}}'}\zeta^{{\pm}*}_{{\boldsymbol{k}}''}\right\rangle\right] \equiv A_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}}, \end{align}
\begin{align} \partial_t \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \mp i2p_{{\parallel}}v_{\mathrm{A}} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle =& \sum_{{\boldsymbol{k}}'{\boldsymbol{k}}''} \left[ M_{{\boldsymbol{p}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{p}},{\boldsymbol{k}}'+{\boldsymbol{k}}''} \left\langle\zeta^\pm_{{\boldsymbol{k}}'}\zeta^\mp_{{\boldsymbol{k}}''}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \right. \nonumber\\ &\quad\left. +\, M_{{\boldsymbol{q}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{q}},{\boldsymbol{k}}'+{\boldsymbol{k}}''}\right. \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\mp_{{\boldsymbol{k}}'}\zeta^\pm_{{\boldsymbol{k}}''}\zeta^{{\pm}*}_{\boldsymbol{k}}\right\rangle \nonumber\\ &\quad\left. +\, M_{{\boldsymbol{k}}{\boldsymbol{k}}'{\boldsymbol{k}}''}\delta_{{\boldsymbol{k}},{\boldsymbol{k}}'+{\boldsymbol{k}}''} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{{\boldsymbol{k}}'}\zeta^{{\pm}*}_{{\boldsymbol{k}}''}\right\rangle\right] \equiv A_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}}, \end{align}
where, in working out the linear term, it has been opportune to take account of ![]() $k_{\parallel } = p_{\parallel } + q_{\parallel }$. To lowest order in the WT expansion, with
$k_{\parallel } = p_{\parallel } + q_{\parallel }$. To lowest order in the WT expansion, with ![]() $A_{{\boldsymbol {k}}{\boldsymbol {p}}{\boldsymbol {q}}}$ approximated as constant in time, the solution to this equation is
$A_{{\boldsymbol {k}}{\boldsymbol {p}}{\boldsymbol {q}}}$ approximated as constant in time, the solution to this equation is
This is the moment when it turns out that every interaction must involve the ![]() $p_{\parallel }=0$ mode, for which the WT approximation is, in fact, broken.
$p_{\parallel }=0$ mode, for which the WT approximation is, in fact, broken.
Pressing on regardless, let us adopt the random-phase approximation, as always in WT (Zakharov et al. Reference Zakharov, L'vov and Falkovich1992; Nazarenko Reference Nazarenko2011). Namely, to lowest order in the WT expansion, any wave field is only correlated with itself at the same ![]() ${\boldsymbol {k}}$, all odd correlators vanish (which is why I had to iterate from (A6) to (A7)), and all even correlators are split into products of quadratic ones, viz.,
${\boldsymbol {k}}$, all odd correlators vanish (which is why I had to iterate from (A6) to (A7)), and all even correlators are split into products of quadratic ones, viz.,
Therefore, noticing that ![]() $M_{{\boldsymbol {q}},-{\boldsymbol {p}},{\boldsymbol {k}}}=-M_{{\boldsymbol {k}}{\boldsymbol {p}}{\boldsymbol {q}}}k_{\perp }^2/q_{\perp }^2$, we get
$M_{{\boldsymbol {q}},-{\boldsymbol {p}},{\boldsymbol {k}}}=-M_{{\boldsymbol {k}}{\boldsymbol {p}}{\boldsymbol {q}}}k_{\perp }^2/q_{\perp }^2$, we get
Combining (A14) with (A8) and putting the latter back into (A6), we arrive at the classic WT equation derived by Galtier et al. (Reference Galtier, Nazarenko, Newell and Pouquet2000):
 \begin{equation} \partial_t N^\pm_{\boldsymbol{k}} = \frac{\pi}{v_{\mathrm{A}}}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}} \frac{k_{{\perp}}^2 M^2_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}}}{p_{{\perp}}^2q_{{\perp}}^2}\,\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}}\, \delta(p_{{\parallel}})\, N^\mp_{\boldsymbol{p}}\left(N^\pm_{\boldsymbol{q}} - N^\pm_{\boldsymbol{k}}\right), \end{equation}
\begin{equation} \partial_t N^\pm_{\boldsymbol{k}} = \frac{\pi}{v_{\mathrm{A}}}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}} \frac{k_{{\perp}}^2 M^2_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}}}{p_{{\perp}}^2q_{{\perp}}^2}\,\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}}\, \delta(p_{{\parallel}})\, N^\mp_{\boldsymbol{p}}\left(N^\pm_{\boldsymbol{q}} - N^\pm_{\boldsymbol{k}}\right), \end{equation}
where ![]() $N^\pm _{\boldsymbol {k}} = k_{\perp }^2 C^\pm _{\boldsymbol {k}} = \left \langle |\boldsymbol {Z}^\pm _{\perp {\boldsymbol {k}}}|^2\right \rangle$.
$N^\pm _{\boldsymbol {k}} = k_{\perp }^2 C^\pm _{\boldsymbol {k}} = \left \langle |\boldsymbol {Z}^\pm _{\perp {\boldsymbol {k}}}|^2\right \rangle$.
A.3. Solution of WT equation
The wavenumber sum in (A15) is turned into an integral in the usual fashion: taking account of the restriction ![]() ${\boldsymbol {k}} = {\boldsymbol {p}}+{\boldsymbol {q}}$ and of the fact that the integrand is even in
${\boldsymbol {k}} = {\boldsymbol {p}}+{\boldsymbol {q}}$ and of the fact that the integrand is even in ![]() $\phi$,
$\phi$,
 \begin{equation} \sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\left(\dots\right) = 2\,\frac{V}{(2\pi)^3}\int_{-\infty}^{+\infty}\mathrm{d}q_{{\parallel}} \int_0^\infty\mathrm{d}q_{{\perp}}q_{{\perp}}\int_0^\pi\mathrm{d}\phi \left(\dots\right), \end{equation}
\begin{equation} \sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\left(\dots\right) = 2\,\frac{V}{(2\pi)^3}\int_{-\infty}^{+\infty}\mathrm{d}q_{{\parallel}} \int_0^\infty\mathrm{d}q_{{\perp}}q_{{\perp}}\int_0^\pi\mathrm{d}\phi \left(\dots\right), \end{equation}
where ![]() $V = L_{\perp }^2L_{\parallel }$ is the volume of the box. The angle integral can be recast as an integral with respect to
$V = L_{\perp }^2L_{\parallel }$ is the volume of the box. The angle integral can be recast as an integral with respect to ![]() $p_{\perp }$:
$p_{\perp }$:
 \begin{equation} p_{{\perp}}^2 = k_{{\perp}}^2 + q_{{\perp}}^2 - 2k_{{\perp}}q_{{\perp}}\cos\phi\quad\Rightarrow\quad \int_0^\pi\mathrm{d}\phi\sin\phi\left(\dots\right) = \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\frac{\mathrm{d}p_{{\perp}}p_{{\perp}}}{k_{{\perp}}q_{{\perp}}}\left(\dots\right). \end{equation}
\begin{equation} p_{{\perp}}^2 = k_{{\perp}}^2 + q_{{\perp}}^2 - 2k_{{\perp}}q_{{\perp}}\cos\phi\quad\Rightarrow\quad \int_0^\pi\mathrm{d}\phi\sin\phi\left(\dots\right) = \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\frac{\mathrm{d}p_{{\perp}}p_{{\perp}}}{k_{{\perp}}q_{{\perp}}}\left(\dots\right). \end{equation}
Finally, defining the 2D spectra ![]() $E_{\mathrm {2D}}^\pm (k_{\perp },k_{\parallel }) = k_{\perp } N^\pm _{\boldsymbol {k}} V/(2\pi )^2$, we get
$E_{\mathrm {2D}}^\pm (k_{\perp },k_{\parallel }) = k_{\perp } N^\pm _{\boldsymbol {k}} V/(2\pi )^2$, we get
 \begin{align} \partial_t E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}}) = &\ \frac{1}{v_{\mathrm{A}}}\int_0^\infty\mathrm{d}q_{{\perp}} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}} \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}}\sin\phi\cos^2\phi \nonumber\\ &\ \times \frac{E_{\mathrm{2D}}^\mp(p_{{\perp}},0)}{p_{{\perp}}} \left[\frac{E_{\mathrm{2D}}^\pm(q_{{\perp}},k_{{\parallel}})}{q_{{\perp}}} - \frac{E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}})}{k_{{\perp}}}\right], \end{align}
\begin{align} \partial_t E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}}) = &\ \frac{1}{v_{\mathrm{A}}}\int_0^\infty\mathrm{d}q_{{\perp}} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}} \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}}\sin\phi\cos^2\phi \nonumber\\ &\ \times \frac{E_{\mathrm{2D}}^\mp(p_{{\perp}},0)}{p_{{\perp}}} \left[\frac{E_{\mathrm{2D}}^\pm(q_{{\perp}},k_{{\parallel}})}{q_{{\perp}}} - \frac{E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}})}{k_{{\perp}}}\right], \end{align}
where ![]() $\cos \phi = (k_{\perp }^2 + q_{\perp }^2 - p_{\perp }^2)/2k_{\perp }q_{\perp }$ and
$\cos \phi = (k_{\perp }^2 + q_{\perp }^2 - p_{\perp }^2)/2k_{\perp }q_{\perp }$ and ![]() $\sin \phi = (1 - \cos ^2\phi )^{1/2}$.
$\sin \phi = (1 - \cos ^2\phi )^{1/2}$.
Let us now, as anticipated in (4.9), assume
substitute these into the right-hand side of (A18) and non-dimensionalise the integral by changing the integration variables to ![]() $x = q_{\perp }/k_{\perp }$ and
$x = q_{\perp }/k_{\perp }$ and ![]() $y = p_{\perp }/k_{\perp }$:
$y = p_{\perp }/k_{\perp }$:
 \begin{align} I(\mu,\mu_0) &= \int_0^\infty\mathrm{d} x\int_{|1-x|}^{1+x}\mathrm{d} y\, y^{{-}2 + \mu_0} x^2 \left(x^{\mu - 1} - 1\right) \sin\phi\cos^2\phi, \end{align}
\begin{align} I(\mu,\mu_0) &= \int_0^\infty\mathrm{d} x\int_{|1-x|}^{1+x}\mathrm{d} y\, y^{{-}2 + \mu_0} x^2 \left(x^{\mu - 1} - 1\right) \sin\phi\cos^2\phi, \end{align}
where ![]() $\cos \phi = (1+x^2-y^2)/2x$. The energy flux formally introduced in (A20) is
$\cos \phi = (1+x^2-y^2)/2x$. The energy flux formally introduced in (A20) is
 \begin{equation} \varPi^\pm(k_{{\perp}},k_{{\parallel}}) ={-}\frac{f^\mp(0)f^\pm(k_{{\parallel}})}{v_{\mathrm{A}}} \frac{I(\mu^\pm,\mu_0^\mp)}{\mu^\pm+ \mu_0^\mp+ 4}\,k_{{\perp}}^{\mu^\pm + \mu_0^\mp + 4}. \end{equation}
\begin{equation} \varPi^\pm(k_{{\perp}},k_{{\parallel}}) ={-}\frac{f^\mp(0)f^\pm(k_{{\parallel}})}{v_{\mathrm{A}}} \frac{I(\mu^\pm,\mu_0^\mp)}{\mu^\pm+ \mu_0^\mp+ 4}\,k_{{\perp}}^{\mu^\pm + \mu_0^\mp + 4}. \end{equation}
It is assumed here that the flux in ![]() $(k_{\perp },k_{\parallel })$ space is in the
$(k_{\perp },k_{\parallel })$ space is in the ![]() $k_{\perp }$ direction only (no parallel cascade in WT). In order for (A22) to be independent of
$k_{\perp }$ direction only (no parallel cascade in WT). In order for (A22) to be independent of ![]() $k_{\perp }$, it must be the case thatFootnote 94
$k_{\perp }$, it must be the case thatFootnote 94
but then, in order for the expression in (A22) to have a finite value, it must also be the case that ![]() $I(\mu,\mu _0) \to 0$ when
$I(\mu,\mu _0) \to 0$ when ![]() $\mu + \mu _0 + 4 \to 0$. That this is indeed the case is shown by changing the integration variables to
$\mu + \mu _0 + 4 \to 0$. That this is indeed the case is shown by changing the integration variables to ![]() $\xi = 1/x$,
$\xi = 1/x$, ![]() $\eta = y/x$, a change that leaves the domain of integration in (A21) the same (a Zakharov transformation; see Zakharov et al. Reference Zakharov, L'vov and Falkovich1992). In these new variables,
$\eta = y/x$, a change that leaves the domain of integration in (A21) the same (a Zakharov transformation; see Zakharov et al. Reference Zakharov, L'vov and Falkovich1992). In these new variables,
 \begin{equation} I(\mu,\mu_0) ={-}\int_0^\infty\mathrm{d}\xi\int_{|1-\xi|}^{1+\xi}\mathrm{d}\eta\, \eta^{{-}2 + \mu_0} \xi^{-\mu-\mu_0-2} \left(\xi^{\mu - 1} - 1\right) \sin\phi\cos^2\phi, \end{equation}
\begin{equation} I(\mu,\mu_0) ={-}\int_0^\infty\mathrm{d}\xi\int_{|1-\xi|}^{1+\xi}\mathrm{d}\eta\, \eta^{{-}2 + \mu_0} \xi^{-\mu-\mu_0-2} \left(\xi^{\mu - 1} - 1\right) \sin\phi\cos^2\phi, \end{equation}
where ![]() $\cos \phi = (1+\xi ^2-\eta ^2)/2\xi$. When
$\cos \phi = (1+\xi ^2-\eta ^2)/2\xi$. When ![]() $\mu + \mu _0 = -4$, this is exactly the same integral as (A21), except with a minus sign, so
$\mu + \mu _0 = -4$, this is exactly the same integral as (A21), except with a minus sign, so ![]() $I = - I = 0$, q.e.d.
$I = - I = 0$, q.e.d.
The problem with this otherwise respectable-looking calculation is that ![]() $E_{\mathrm {2D}}^\mp (p_{\perp },0)$, which plays a key role in (A18), is the spectrum of zero-frequency,
$E_{\mathrm {2D}}^\mp (p_{\perp },0)$, which plays a key role in (A18), is the spectrum of zero-frequency, ![]() $p_{\parallel }=0$ modes, for which the WT approximation cannot be used, so
$p_{\parallel }=0$ modes, for which the WT approximation cannot be used, so ![]() $\mu _0^\mp$ is certainly not determinable within WT, the random-phase approximation should not have been used for these modes (and has been explicitly shown not to hold for them by Meyrand et al. Reference Meyrand, Kiyani and Galtier2015), and so it is at the very least doubtful that (A18) can be used for the determination of
$\mu _0^\mp$ is certainly not determinable within WT, the random-phase approximation should not have been used for these modes (and has been explicitly shown not to hold for them by Meyrand et al. Reference Meyrand, Kiyani and Galtier2015), and so it is at the very least doubtful that (A18) can be used for the determination of ![]() $\mu ^\pm$, the scaling exponents for the waves, either. For the moment, let me put aside the latter doubt and act on the assumption that if I can figure out
$\mu ^\pm$, the scaling exponents for the waves, either. For the moment, let me put aside the latter doubt and act on the assumption that if I can figure out ![]() $\mu _0^\mp$ in some way,
$\mu _0^\mp$ in some way, ![]() $\mu ^\pm$ will follow by (A23).
$\mu ^\pm$ will follow by (A23).
A.4. Case of broad-band forcing: spectral continuity
The argument that is about to be presented here is heuristic and routed in the ideas about the treatment of strong turbulence described in §§ 2.3 and 5 – it turns out that, to understand weak turbulence, one must understand strong turbulence first. I will, therefore, not attempt to deal with imbalanced WT – because, even though I did, in § 9.6, attempt to construct a coherent picture of strong imbalanced turbulence, it is too tentative and too fiddly to be inserted into what follows, which will be tentative and fiddly in its own right. Thus, the ‘![]() $\pm$’ tags are now dropped everywhere.
$\pm$’ tags are now dropped everywhere.
In reality, the delta function ![]() $\delta (p_{\parallel })$ in (A8) has a width equal to the characteristic broadening of the frequency resonance due to nonlinear interactions,
$\delta (p_{\parallel })$ in (A8) has a width equal to the characteristic broadening of the frequency resonance due to nonlinear interactions, ![]() $\varDelta k _{\parallel } \sim \tau _{\mathrm {nl}}^{-1}/v_{\mathrm {A}}$, to wit,
$\varDelta k _{\parallel } \sim \tau _{\mathrm {nl}}^{-1}/v_{\mathrm {A}}$, to wit,
 \begin{equation} \delta(p_{{\parallel}}) =\frac{\varDelta k_{{\parallel}}}{\pi}\frac{1}{p_{{\parallel}}^2 + \varDelta k_{{\parallel}}^2} \end{equation}
\begin{equation} \delta(p_{{\parallel}}) =\frac{\varDelta k_{{\parallel}}}{\pi}\frac{1}{p_{{\parallel}}^2 + \varDelta k_{{\parallel}}^2} \end{equation}
(in the WT approximation, ![]() $\varDelta k _{\parallel } \to 0$). At
$\varDelta k _{\parallel } \to 0$). At ![]() $p_{\parallel } \lesssim \varDelta k _{\parallel }$, the ‘
$p_{\parallel } \lesssim \varDelta k _{\parallel }$, the ‘![]() $p_{\parallel }=0$’ condensate resides, whose turbulence is strong (figure 36). Let us work out the structure of this turbulence.
$p_{\parallel }=0$’ condensate resides, whose turbulence is strong (figure 36). Let us work out the structure of this turbulence.

Figure 36. Cartoon of the 2D spectrum of broad-band-forced weak turbulence. Schematic contour lines of ![]() $E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ are the brown dotted lines. Red arrows are energy fluxes:
$E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ are the brown dotted lines. Red arrows are energy fluxes: ![]() $\varPi (k_{\perp },k_{\parallel })\sim \varepsilon L _{\parallel }$ arriving from the forcing wavenumbers to the ‘2D condensate’ at each
$\varPi (k_{\perp },k_{\parallel })\sim \varepsilon L _{\parallel }$ arriving from the forcing wavenumbers to the ‘2D condensate’ at each ![]() $k_{\parallel }$,
$k_{\parallel }$, ![]() $\varepsilon L _{\parallel }\varDelta k _{\parallel }$ flowing through the condensate (see (A26)), and
$\varepsilon L _{\parallel }\varDelta k _{\parallel }$ flowing through the condensate (see (A26)), and ![]() $\varepsilon = \mathrm {const}$ after the transition to critically balanced cascade (cf. figure 40a).
$\varepsilon = \mathrm {const}$ after the transition to critically balanced cascade (cf. figure 40a).
Let us assume that our WT is forced in a broad band of parallel wavenumbers ![]() $k_{\parallel } \in (0,2\pi /L_{\parallel })$ (obviously, the parallel size of ‘the box’ must be
$k_{\parallel } \in (0,2\pi /L_{\parallel })$ (obviously, the parallel size of ‘the box’ must be ![]() $\gg L _{\parallel }$). This can happen, e.g., if the forcing is completely random with parallel coherence length
$\gg L _{\parallel }$). This can happen, e.g., if the forcing is completely random with parallel coherence length ![]() $L_{\parallel }$, in which case its
$L_{\parallel }$, in which case its ![]() $k_{\parallel }$ spectrum at
$k_{\parallel }$ spectrum at ![]() $k_{\parallel } < 2\pi /L_{\parallel }$ is flat (a white noise). Thus, the same amount of energy is injected into each
$k_{\parallel } < 2\pi /L_{\parallel }$ is flat (a white noise). Thus, the same amount of energy is injected into each ![]() $k_{\parallel }$, this energy is cascaded weakly in
$k_{\parallel }$, this energy is cascaded weakly in ![]() $k_{\perp }$ (by the still-to-be-worked-out condensate) without change in
$k_{\perp }$ (by the still-to-be-worked-out condensate) without change in ![]() $k_{\parallel }$ until it arrives at the CB scale associated with this
$k_{\parallel }$ until it arrives at the CB scale associated with this ![]() $k_{\parallel }$, i.e., at the
$k_{\parallel }$, i.e., at the ![]() $k_{\perp }$ for which
$k_{\perp }$ for which ![]() $\varDelta k _{\parallel }(k_{\perp }) \sim k_{\parallel }$ (equivalently,
$\varDelta k _{\parallel }(k_{\perp }) \sim k_{\parallel }$ (equivalently, ![]() $\tau _{\mathrm {nl}}^{-1}\sim k_{\parallel} v _{\mathrm {A}}$), where it joins the condensate. Therefore, the flux of energy into, and via, the condensate is not scale-independent: at any given
$\tau _{\mathrm {nl}}^{-1}\sim k_{\parallel} v _{\mathrm {A}}$), where it joins the condensate. Therefore, the flux of energy into, and via, the condensate is not scale-independent: at any given ![]() $k_{\perp }$, it is
$k_{\perp }$, it is
where I have assumed that ![]() $\varPi (k_{\perp },k_{\parallel })\sim \varepsilon L _{\parallel }$ is a constant in both of its arguments (constant in
$\varPi (k_{\perp },k_{\parallel })\sim \varepsilon L _{\parallel }$ is a constant in both of its arguments (constant in ![]() $k_{\perp }$ because the WT cascade is a constant-flux one and constant in
$k_{\perp }$ because the WT cascade is a constant-flux one and constant in ![]() $k_{\parallel }$ because the amount of energy injection is the same at every
$k_{\parallel }$ because the amount of energy injection is the same at every ![]() $k_{\parallel }$). Then, for the condensate at scale
$k_{\parallel }$). Then, for the condensate at scale ![]() $\lambda \sim k_{\perp }^{-1}$,
$\lambda \sim k_{\perp }^{-1}$,
 \begin{equation} \frac{\delta Z_\lambda^2}{\tau_{\mathrm{nl}}} \sim \varepsilon L_{{\parallel}}\varDelta k_{{\parallel}}(k_{{\perp}}) \sim \frac{\varepsilon L_{{\parallel}}}{\tau_{\mathrm{nl}}v_{\mathrm{A}}} \quad\Rightarrow\quad \delta Z_\lambda \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2} \quad\Rightarrow\quad E_0(k_{{\perp}}) \sim \frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\,k_{{\perp}}^{{-}1}, \end{equation}
\begin{equation} \frac{\delta Z_\lambda^2}{\tau_{\mathrm{nl}}} \sim \varepsilon L_{{\parallel}}\varDelta k_{{\parallel}}(k_{{\perp}}) \sim \frac{\varepsilon L_{{\parallel}}}{\tau_{\mathrm{nl}}v_{\mathrm{A}}} \quad\Rightarrow\quad \delta Z_\lambda \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2} \quad\Rightarrow\quad E_0(k_{{\perp}}) \sim \frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\,k_{{\perp}}^{{-}1}, \end{equation}
where ![]() $E_0(k_{\perp })$ is the condensate's 1D spectrum.
$E_0(k_{\perp })$ is the condensate's 1D spectrum.
This 1D spectrum is the 2D spectrum ![]() $E_0(k_{\perp },k_{\parallel })$ integrated over all parallel wavenumbers belonging to the condensate, viz.,
$E_0(k_{\perp },k_{\parallel })$ integrated over all parallel wavenumbers belonging to the condensate, viz.,
The last step is valid on the assumption that ![]() $E_0(k_{\perp },k_{\parallel })$ is, in fact, independent of
$E_0(k_{\perp },k_{\parallel })$ is, in fact, independent of ![]() $k_{\parallel }$, because by the usual CB assumption, there cannot be any correlations at parallel scales
$k_{\parallel }$, because by the usual CB assumption, there cannot be any correlations at parallel scales ![]() $k_{\parallel }^{-1}>v_{\mathrm {A}}\tau _{\mathrm {nl}}\sim \varDelta k _{\parallel }^{-1}(k_{\perp })$ and so the corresponding
$k_{\parallel }^{-1}>v_{\mathrm {A}}\tau _{\mathrm {nl}}\sim \varDelta k _{\parallel }^{-1}(k_{\perp })$ and so the corresponding ![]() $k_{\parallel }$ spectrum is that of a white noise (cf. Appendix C.1).
$k_{\parallel }$ spectrum is that of a white noise (cf. Appendix C.1).
The cascade time for the condensate (which advects itself) is
 \begin{equation} \tau_{\mathrm{nl}}^{{-}1} \sim \frac{\delta Z_\lambda}{\lambda} \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\lambda^{{-}1} \quad\Rightarrow\quad\varDelta k_{{\parallel}}(k_{{\perp}}) \sim \frac{\left(\varepsilon L_{{\parallel}}\right)^{1/2}}{v_{\mathrm{A}}^{3/2}}\,k_{{\perp}}. \end{equation}
\begin{equation} \tau_{\mathrm{nl}}^{{-}1} \sim \frac{\delta Z_\lambda}{\lambda} \sim \left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\lambda^{{-}1} \quad\Rightarrow\quad\varDelta k_{{\parallel}}(k_{{\perp}}) \sim \frac{\left(\varepsilon L_{{\parallel}}\right)^{1/2}}{v_{\mathrm{A}}^{3/2}}\,k_{{\perp}}. \end{equation}I am assuming that there is no dynamic alignment (§ 6) for the condensate because the condensate is effectively forced at every scale by the WT cascade – this is not a proof, but a conjecture, adopted for its simplicity and plausibility. Finally, (A29), via (A28) and (A27), leads to
Thus, ![]() $\mu _0= -2$ for reasons that have little to do with weak interactions, and, therefore, by (A23),
$\mu _0= -2$ for reasons that have little to do with weak interactions, and, therefore, by (A23), ![]() $\mu = -2$ as well (in Appendix C.5, the same results are rederived in a slightly different way, which may or may not shed more light).
$\mu = -2$ as well (in Appendix C.5, the same results are rederived in a slightly different way, which may or may not shed more light).
Thus, there is, in fact, no difference between the WT spectrum at ![]() $k_{\parallel }>\varDelta k _{\parallel }(k_{\perp })$ and the condensate's spectrum at
$k_{\parallel }>\varDelta k _{\parallel }(k_{\perp })$ and the condensate's spectrum at ![]() $k_{\parallel } < \varDelta k _{\parallel }(k_{\perp })$, even though the nature of turbulence in these two regions is quite different. The above construction can thus be viewed as a physical argument in support of spectral continuity. It does not make the derivation of the WT equation in Appendix A.2 formally correct but it does perhaps lend it some credibility.
$k_{\parallel } < \varDelta k _{\parallel }(k_{\perp })$, even though the nature of turbulence in these two regions is quite different. The above construction can thus be viewed as a physical argument in support of spectral continuity. It does not make the derivation of the WT equation in Appendix A.2 formally correct but it does perhaps lend it some credibility.
A.5. Residual energy in WT
If one takes this appearance of WT credibility seriously, there is another result that can be ‘derived’ within it. The random-phase approximation for Alfvén waves implied the absence of correlations between the counterpropagating Elsasser fields, (A10). What if we relax this assumption – and only this assumption! – while still splitting fourth-order correlators into second-order ones? Namely, let us set
where ![]() $R_{\boldsymbol {k}}^{-*} = R_{\boldsymbol {k}}^+\equiv R_{\boldsymbol {k}}$, and work out the WT evolution equation for
$R_{\boldsymbol {k}}^{-*} = R_{\boldsymbol {k}}^+\equiv R_{\boldsymbol {k}}$, and work out the WT evolution equation for ![]() $R_{\boldsymbol {k}}$. This is interesting, inter alia, because
$R_{\boldsymbol {k}}$. This is interesting, inter alia, because ![]() $\mathrm {Re} R_{\boldsymbol {k}}$ is the 3D residual-energy spectrum and so the derivation I am about to present (which is a version of what Wang et al. Reference Wang, Boldyrev and Perez2011 did) has a claim to providing theoretical backing to the presence of negative residual energy both in observed and in numerically simulated MHD turbulence (see § 10).
$\mathrm {Re} R_{\boldsymbol {k}}$ is the 3D residual-energy spectrum and so the derivation I am about to present (which is a version of what Wang et al. Reference Wang, Boldyrev and Perez2011 did) has a claim to providing theoretical backing to the presence of negative residual energy both in observed and in numerically simulated MHD turbulence (see § 10).
From the field equation (A4), straightforwardly,
Following the same protocol as in Appendix A.2, let us write the evolution equation for the third-order correlators in (A32) in terms of fourth-order correlators and then split the latter into second-order ones, but now allowing non-zero correlations between different Elsasser fields according to (A31):
 \begin{align} \partial_t\left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{\boldsymbol{k}}\right\rangle & \mp i2q_{{\parallel}}v_{\mathrm{A}} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{\boldsymbol{k}}\right\rangle = \delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}}\frac{\hat{\boldsymbol{z}}\cdot\left({\boldsymbol{k}}_{{\perp}}\times{\boldsymbol{q}}_{{\perp}}\right)}{k_{{\perp}}^2p_{{\perp}}^2q_{{\perp}}^2} \left[{\boldsymbol{k}}_{{\perp}}\cdot{\boldsymbol{p}}_{{\perp}} N^\pm_{\boldsymbol{q}}\left(N^\mp_{\boldsymbol{k}} - N^\mp_{\boldsymbol{p}}\right)\right.\nonumber\\ &\quad+ \left.{\boldsymbol{k}}_{{\perp}}\cdot{\boldsymbol{q}}_{{\perp}}\left(R^\mp_{\boldsymbol{p}} R^\pm_{\boldsymbol{q}} - R^\pm_{\boldsymbol{k}} N^\mp_{\boldsymbol{p}}\right) + {\boldsymbol{p}}_{{\perp}}\cdot{\boldsymbol{q}}_{{\perp}}\left(R^\pm_{\boldsymbol{k}} R^\pm_{\boldsymbol{q}} - R^\mp_{\boldsymbol{p}} N^\mp_{\boldsymbol{k}}\right)\right]. \end{align}
\begin{align} \partial_t\left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{\boldsymbol{k}}\right\rangle & \mp i2q_{{\parallel}}v_{\mathrm{A}} \left\langle\zeta^\mp_{\boldsymbol{p}}\zeta^\pm_{\boldsymbol{q}}\zeta^{{\mp}*}_{\boldsymbol{k}}\right\rangle = \delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}}\frac{\hat{\boldsymbol{z}}\cdot\left({\boldsymbol{k}}_{{\perp}}\times{\boldsymbol{q}}_{{\perp}}\right)}{k_{{\perp}}^2p_{{\perp}}^2q_{{\perp}}^2} \left[{\boldsymbol{k}}_{{\perp}}\cdot{\boldsymbol{p}}_{{\perp}} N^\pm_{\boldsymbol{q}}\left(N^\mp_{\boldsymbol{k}} - N^\mp_{\boldsymbol{p}}\right)\right.\nonumber\\ &\quad+ \left.{\boldsymbol{k}}_{{\perp}}\cdot{\boldsymbol{q}}_{{\perp}}\left(R^\mp_{\boldsymbol{p}} R^\pm_{\boldsymbol{q}} - R^\pm_{\boldsymbol{k}} N^\mp_{\boldsymbol{p}}\right) + {\boldsymbol{p}}_{{\perp}}\cdot{\boldsymbol{q}}_{{\perp}}\left(R^\pm_{\boldsymbol{k}} R^\pm_{\boldsymbol{q}} - R^\mp_{\boldsymbol{p}} N^\mp_{\boldsymbol{k}}\right)\right]. \end{align}
The presence of the first term is proof that ![]() $R_{\boldsymbol {k}}=0$ is, generally speaking, not a sustainable solution. However, since growth of correlations between counterpropagating Elsasser fields contradicts the random-phase approximation and thus undermines WT, perhaps we could hope (falsely, as I will show shortly) that
$R_{\boldsymbol {k}}=0$ is, generally speaking, not a sustainable solution. However, since growth of correlations between counterpropagating Elsasser fields contradicts the random-phase approximation and thus undermines WT, perhaps we could hope (falsely, as I will show shortly) that ![]() $R_{\boldsymbol {k}}$ might be small and so the terms containing
$R_{\boldsymbol {k}}$ might be small and so the terms containing ![]() $R_{\boldsymbol {k}}$ in (A33) could be neglected for the time being. Then the solution of (A33) is
$R_{\boldsymbol {k}}$ in (A33) could be neglected for the time being. Then the solution of (A33) is
Substituting this into (A32), solving that in turn, and denoting
we find
 \begin{align} \mathrm{Re} R_{\boldsymbol{k}} &= \mathrm{Re}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}} \frac{1}{i2q_{{\parallel}}v_{\mathrm{A}}}\left(\frac{1-e^{i2k_{\parallel} v_{\mathrm{A}} t}}{i2k_{\parallel} v_{\mathrm{A}}} - e^{i2q_{{\parallel}}v_{\mathrm{A}} t}\frac{1-e^{i2p_{{\parallel}}v_{\mathrm{A}} t}}{i2p_{{\parallel}}v_{\mathrm{A}}}\right) B_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}},\nonumber\\ &\to \frac{\pi^2}{4v_{\mathrm{A}}^2}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}} \delta(p_{{\parallel}})\delta(q_{{\parallel}}) B_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}} \quad\mathrm{as}\quad t\to \infty. \end{align}
\begin{align} \mathrm{Re} R_{\boldsymbol{k}} &= \mathrm{Re}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}} \frac{1}{i2q_{{\parallel}}v_{\mathrm{A}}}\left(\frac{1-e^{i2k_{\parallel} v_{\mathrm{A}} t}}{i2k_{\parallel} v_{\mathrm{A}}} - e^{i2q_{{\parallel}}v_{\mathrm{A}} t}\frac{1-e^{i2p_{{\parallel}}v_{\mathrm{A}} t}}{i2p_{{\parallel}}v_{\mathrm{A}}}\right) B_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}},\nonumber\\ &\to \frac{\pi^2}{4v_{\mathrm{A}}^2}\sum_{{\boldsymbol{p}}{\boldsymbol{q}}}\delta_{{\boldsymbol{k}},{\boldsymbol{p}}+{\boldsymbol{q}}} \delta(p_{{\parallel}})\delta(q_{{\parallel}}) B_{{\boldsymbol{k}}{\boldsymbol{p}}{\boldsymbol{q}}} \quad\mathrm{as}\quad t\to \infty. \end{align}The 2D spectrum of residual energy is, therefore,
 \begin{align} & E_{\mathrm{res,2D}}(k_{{\perp}},k_{{\parallel}}) = \frac{Vk_{{\perp}}\mathrm{Re} R_{\boldsymbol{k}}}{(2\pi)^2} ={-} \frac{\pi \delta(k_{{\parallel}})}{4v_{\mathrm{A}}^2}\int_0^\infty\mathrm{d}q_{{\perp}} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}}\, \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}}\,\sin\phi\cos^2\phi \nonumber\\ &\times\left\{\frac{E^+(q_{{\perp}},0)}{q_{{\perp}}}\left[\frac{E^-(k_{{\perp}},0)}{k_{{\perp}}} - \frac{E^-(p_{{\perp}},0)}{p_{{\perp}}}\right] + \frac{E^-(q_{{\perp}},0)}{q_{{\perp}}}\left[\frac{E^+(k_{{\perp}},0)}{k_{{\perp}}} - \frac{E^+(p_{{\perp}},0)}{p_{{\perp}}}\right]\right\}, \end{align}
\begin{align} & E_{\mathrm{res,2D}}(k_{{\perp}},k_{{\parallel}}) = \frac{Vk_{{\perp}}\mathrm{Re} R_{\boldsymbol{k}}}{(2\pi)^2} ={-} \frac{\pi \delta(k_{{\parallel}})}{4v_{\mathrm{A}}^2}\int_0^\infty\mathrm{d}q_{{\perp}} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}}\, \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}}\,\sin\phi\cos^2\phi \nonumber\\ &\times\left\{\frac{E^+(q_{{\perp}},0)}{q_{{\perp}}}\left[\frac{E^-(k_{{\perp}},0)}{k_{{\perp}}} - \frac{E^-(p_{{\perp}},0)}{p_{{\perp}}}\right] + \frac{E^-(q_{{\perp}},0)}{q_{{\perp}}}\left[\frac{E^+(k_{{\perp}},0)}{k_{{\perp}}} - \frac{E^+(p_{{\perp}},0)}{p_{{\perp}}}\right]\right\}, \end{align}where the wavenumber integrals have been manipulated in exactly the same way as they were in Appendix A.2, in the lead-up to (A18). Again assuming the power-law solutions (A19), we get
One establishes that the prefactor is negative (i.e., ![]() $\mathrm {const} > 0$) by computing the wavenumber integral directly (Wang et al. Reference Wang, Boldyrev and Perez2011).
$\mathrm {const} > 0$) by computing the wavenumber integral directly (Wang et al. Reference Wang, Boldyrev and Perez2011).
What does this result tell us? Primarily, it tells us that the WT calculation that has led to it is formally invalid and can, at best, be interpreted as a qualitative indication of what is going on. All the action has turned out to be concentrated in the ![]() $k_{\parallel }=0$ condensate, while for Alfvén waves with
$k_{\parallel }=0$ condensate, while for Alfvén waves with ![]() $k_{\parallel }\neq 0$, there is no residual energy. That we were going to end up with
$k_{\parallel }\neq 0$, there is no residual energy. That we were going to end up with ![]() $\delta (k_{\parallel })$ was, in fact, already obvious from the presence of the oscillatory term in (A32). Nevertheless, without a claim to mathematical rigour, one can, as I did in Appendix A.4, interpret the delta function in (A38) as having a width
$\delta (k_{\parallel })$ was, in fact, already obvious from the presence of the oscillatory term in (A32). Nevertheless, without a claim to mathematical rigour, one can, as I did in Appendix A.4, interpret the delta function in (A38) as having a width ![]() $\varDelta k _{\parallel } \sim \tau _{\mathrm {nl}}^{-1}/v_{\mathrm {A}}$, where
$\varDelta k _{\parallel } \sim \tau _{\mathrm {nl}}^{-1}/v_{\mathrm {A}}$, where ![]() $\tau _{\mathrm {nl}}\propto k _{\perp }^{-1}$ is the cascade time for the condensate, worked out in (A29). Taking
$\tau _{\mathrm {nl}}\propto k _{\perp }^{-1}$ is the cascade time for the condensate, worked out in (A29). Taking ![]() $\mu _0^+ + \mu _0^- = -4$ and
$\mu _0^+ + \mu _0^- = -4$ and ![]() $\delta (k_{\parallel })\sim \varDelta k _{\parallel }^{-1} \propto k_{\perp }^{-1}$ in (A38) gets us
$\delta (k_{\parallel })\sim \varDelta k _{\parallel }^{-1} \propto k_{\perp }^{-1}$ in (A38) gets us
whereas the 1D spectrum can be calculated either by integrating out the delta function in (A38) or by integrating its broadened version in (A39) over its width ![]() $\varDelta k _{\parallel }~\propto ~k_{\perp }$:
$\varDelta k _{\parallel }~\propto ~k_{\perp }$:
This is the result of Wang et al. (Reference Wang, Boldyrev and Perez2011), who, however, go to slightly greater lengths in setting up a quasi-quantitative calculation in which they introduce by hand a nonlinear relaxation rate ![]() $\tau _{\mathrm {nl}}^{-1}\propto k _{\perp }$ into (A32) and thus get their
$\tau _{\mathrm {nl}}^{-1}\propto k _{\perp }$ into (A32) and thus get their ![]() $\delta (k_{\parallel })$ to acquire the Lorentzian shape (A25). They attribute this relaxation to the
$\delta (k_{\parallel })$ to acquire the Lorentzian shape (A25). They attribute this relaxation to the ![]() $R_{\boldsymbol {k}}$-dependent terms in (A33), which is qualitatively correct, but quantitatively just as invalid as is generally the application of the WT approximation (i.e., correlator splitting) to the strongly turbulent condensate.
$R_{\boldsymbol {k}}$-dependent terms in (A33), which is qualitatively correct, but quantitatively just as invalid as is generally the application of the WT approximation (i.e., correlator splitting) to the strongly turbulent condensate.
Note that (A40) is, in fact, the same result as (A27) – by comparing (A19) with (A30), or just by dimensional analysis, it is easy to confirm that ![]() $f^\pm (0)\sim (\varepsilon L _{\parallel }v_{\mathrm {A}})^{1/2}$, so the dimensional prefactors match. Thus, all we have learned from the above calculation is that the condensate has residual energy and that the amount of the latter is comparable, at every scale, to the amount of energy in the condensate. One might argue that the added value of the WT calculation was in confirming that this residual energy was negative – although the negativity of the prefactor in (A38) is a quantitative result, not a qualitative one (one just has to calculate the appropriate integral and discover it to be negative, as Wang et al. Reference Wang, Boldyrev and Perez2011 did), and so, in principle, cannot be guaranteed to hold for the true, strongly turbulent condensate. I find the qualitative argument for the development of negative vorticity correlation
$f^\pm (0)\sim (\varepsilon L _{\parallel }v_{\mathrm {A}})^{1/2}$, so the dimensional prefactors match. Thus, all we have learned from the above calculation is that the condensate has residual energy and that the amount of the latter is comparable, at every scale, to the amount of energy in the condensate. One might argue that the added value of the WT calculation was in confirming that this residual energy was negative – although the negativity of the prefactor in (A38) is a quantitative result, not a qualitative one (one just has to calculate the appropriate integral and discover it to be negative, as Wang et al. Reference Wang, Boldyrev and Perez2011 did), and so, in principle, cannot be guaranteed to hold for the true, strongly turbulent condensate. I find the qualitative argument for the development of negative vorticity correlation ![]() $\langle \omega ^+\omega ^-\rangle <0$ explained in § 10.4 more compelling. The WT calculation above basically just confirms that growth of residual energy is a strong-turbulence effect.
$\langle \omega ^+\omega ^-\rangle <0$ explained in § 10.4 more compelling. The WT calculation above basically just confirms that growth of residual energy is a strong-turbulence effect.
The qualitative considerations presented above are given some credence by the numerical simulations of WT reported by Wang et al. (Reference Wang, Boldyrev and Perez2011): their residual energy does indeed have a ![]() $k_{\perp }^{-1}$ spectrum and concentrates in a wedge of wavenumber space
$k_{\perp }^{-1}$ spectrum and concentrates in a wedge of wavenumber space ![]() $k_{\parallel } \lesssim \varDelta k _{\parallel }\propto k_{\perp }$, quite convincingly (figure 37).
$k_{\parallel } \lesssim \varDelta k _{\parallel }\propto k_{\perp }$, quite convincingly (figure 37).

Figure 37. (a) The 2D spectrum, ![]() $E_{\mathrm {res,2D}}(k_{\perp },k_{\parallel })/E_{\mathrm {res,2D}}(k_{\perp },0)$ and (b) the 1D,
$E_{\mathrm {res,2D}}(k_{\perp },k_{\parallel })/E_{\mathrm {res,2D}}(k_{\perp },0)$ and (b) the 1D, ![]() $k_{\perp }^{-1}$- compensated spectrum of residual energy from WT simulations by Wang et al. (Reference Wang, Boldyrev and Perez2011) (
$k_{\perp }^{-1}$- compensated spectrum of residual energy from WT simulations by Wang et al. (Reference Wang, Boldyrev and Perez2011) (![]() $512^3$, broad-band forced at
$512^3$, broad-band forced at ![]() $k_{\parallel } = 1,\ldots,16$ and
$k_{\parallel } = 1,\ldots,16$ and ![]() $k_{\perp }=1,2$; © AAS, reproduced with permission).
$k_{\perp }=1,2$; © AAS, reproduced with permission).
A.6. Imbalanced WT
As I acknowledged in § 4.3, I do not know how to construct a good theory of imbalanced WT. If imbalanced WT, like the balanced one, spawns a 2D condensate that is predominantly magnetic, that may be a helpful insight, as the presence of significant residual energy would impose geometric constraints (Appendix B.1) on the ‘![]() $+$’ and ‘
$+$’ and ‘![]() $-$’ components of the condensate. Boldyrev & Perez (Reference Boldyrev and Perez2009) do find a magnetic condensate in an imbalanced simulation, but they only have results for order-unity imbalance. They also point out that if the cross-correlations (A31) are retained in the derivation of the WT equation (A15) for
$-$’ components of the condensate. Boldyrev & Perez (Reference Boldyrev and Perez2009) do find a magnetic condensate in an imbalanced simulation, but they only have results for order-unity imbalance. They also point out that if the cross-correlations (A31) are retained in the derivation of the WT equation (A15) for ![]() $N_{\boldsymbol {k}}$, this makes the evolution equation (A18) for
$N_{\boldsymbol {k}}$, this makes the evolution equation (A18) for ![]() $E_{\mathrm {2D}}^\pm (k_{\perp },0)$ acquire terms under the integral containing
$E_{\mathrm {2D}}^\pm (k_{\perp },0)$ acquire terms under the integral containing ![]() $E_{\mathrm {2D}}^\pm (k_{\perp },0)E_{\mathrm {res,2D}}(p_{\perp },0) + E_{\mathrm {2D}}^\pm (p_{\perp },0)E_{\mathrm {res,2D}}(k_{\perp },0)$. Steady-state solutions then turn out to be possible only if
$E_{\mathrm {2D}}^\pm (k_{\perp },0)E_{\mathrm {res,2D}}(p_{\perp },0) + E_{\mathrm {2D}}^\pm (p_{\perp },0)E_{\mathrm {res,2D}}(k_{\perp },0)$. Steady-state solutions then turn out to be possible only if
i.e., the degeneracy of the ![]() $\mu _0^+ + \mu _0^- = -4$ solution is lifted and all scalings are fixed. Perhaps this points us in the right direction, despite the fact that the WT equation for
$\mu _0^+ + \mu _0^- = -4$ solution is lifted and all scalings are fixed. Perhaps this points us in the right direction, despite the fact that the WT equation for ![]() $E_{\mathrm {2D}}^\pm (k_{\perp },0)$, whose derivation requires correlator splitting etc., is not, in fact, quantitatively valid for the condensate.
$E_{\mathrm {2D}}^\pm (k_{\perp },0)$, whose derivation requires correlator splitting etc., is not, in fact, quantitatively valid for the condensate.
In their mildly imbalanced WT simulation, Boldyrev & Perez (Reference Boldyrev and Perez2009) find that ![]() $E^+(k_{\perp })$ and
$E^+(k_{\perp })$ and ![]() $E^-(k_{\perp })$ have, respectively, a steeper and a shallower slope than
$E^-(k_{\perp })$ have, respectively, a steeper and a shallower slope than ![]() $k_{\perp }^{-2}$, but the spectra appear to be pinned (equal) at the dissipation scale and thus get closer to each other with increased resolution. Thus, if one wants a theory that describes finite-resolution simulations, some scheme like the one I proposed in § 9.6 would need to be invented for the WT regime, generalising Appendix A.4 to the imbalanced case.
$k_{\perp }^{-2}$, but the spectra appear to be pinned (equal) at the dissipation scale and thus get closer to each other with increased resolution. Thus, if one wants a theory that describes finite-resolution simulations, some scheme like the one I proposed in § 9.6 would need to be invented for the WT regime, generalising Appendix A.4 to the imbalanced case.
Appendix B. Alignment, imbalance, and reduction of nonlinearity
These topics have cropped up repeatedly (e.g., in §§ 6.1 and 9.1). This appendix is an attempt to treat them carefully.
B.1. Geometry and types of alignment
Let us consider the formal relationship between imbalance, residual energy, and (the two kinds of) alignment. The first salient fact is purely geometric (figure 38): the two alignment angles (defined for a particular pair of field increments)
 \begin{equation} \sin\theta = \frac{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}\times\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|}{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}| |\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|},\quad \sin\theta^{ub} = \frac{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}\times\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|}{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}| |\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|}, \end{equation}
\begin{equation} \sin\theta = \frac{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}\times\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|}{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}| |\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|},\quad \sin\theta^{ub} = \frac{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}\times\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|}{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}| |\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|}, \end{equation}and the Elsasser and Alfvén ratios
 \begin{equation} R_{\mathrm{E}} = \frac{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}|^2}{|\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|^2},\quad R_{\mathrm{A}} = \frac{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}|^2}{|\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|^2} \end{equation}
\begin{equation} R_{\mathrm{E}} = \frac{|\delta\boldsymbol{Z}^+_{\boldsymbol{\lambda}}|^2}{|\delta\boldsymbol{Z}^-_{\boldsymbol{\lambda}}|^2},\quad R_{\mathrm{A}} = \frac{|\delta\boldsymbol{u}_{\boldsymbol{\lambda}}|^2}{|\delta\boldsymbol{b}_{\boldsymbol{\lambda}}|^2} \end{equation}are related by the following equations
so only two of these four quantities are independent. Equivalently, in terms of the normalised local cross-helicity and residual energy, defined by
respectively, the alignment angles are (Wicks et al. Reference Wicks, Mallet, Horbury, Chen, Schekochihin and Mitchell2013a)Footnote 95
This means that alignment between the velocity and magnetic field is not formally the same thing as alignment between the Elsasser fields, and it is a non-trivial decision which of these one believes to matter for the determination of ![]() $\tau _{\mathrm {nl}}^\pm$.
$\tau _{\mathrm {nl}}^\pm$.

Figure 38. Geometry of velocity, magnetic and Elsasser fields (![]() $\boldsymbol {B}_0$ is perpendicular to the page). All four fields are aligned: the angles
$\boldsymbol {B}_0$ is perpendicular to the page). All four fields are aligned: the angles ![]() $\theta$,
$\theta$, ![]() $\theta ^{ub}$,
$\theta ^{ub}$, ![]() $\theta ^\pm$ are all small (although they do not have to be). Also shown are the axes along which the
$\theta ^\pm$ are all small (although they do not have to be). Also shown are the axes along which the ![]() $\lambda$ and
$\lambda$ and ![]() $\xi$ scales in (6.4) are meant to be calculated (along and across
$\xi$ scales in (6.4) are meant to be calculated (along and across ![]() $\boldsymbol {Z}_{\perp }^+$, respectively). The angle between these axes is
$\boldsymbol {Z}_{\perp }^+$, respectively). The angle between these axes is ![]() $\phi = \pi /2 - \theta$ and so
$\phi = \pi /2 - \theta$ and so ![]() $\cos \phi = \sin \theta$.
$\cos \phi = \sin \theta$.
Before taking a side on this question in Appendix B.2, let me consider a strongly imbalanced situation, where ![]() $R_{\mathrm {E}}\gg 1$, i.e., the cross-helicity is large (
$R_{\mathrm {E}}\gg 1$, i.e., the cross-helicity is large (![]() $\sigma _{\mathrm {c}}\approx 1$) – in view of the discussion in § 9.1, this is probably an asymptotic case best related to the generic situation, at least locally. In this limit, (B3) gives us
$\sigma _{\mathrm {c}}\approx 1$) – in view of the discussion in § 9.1, this is probably an asymptotic case best related to the generic situation, at least locally. In this limit, (B3) gives us
Mallet & Schekochihin (Reference Mallet and Schekochihin2011) found, unsurprisingly, that these relations were extremely well satisfied in their RMHD simulations (the first relation even in balanced ones – see figure 39). Thus, (local) imbalance implies that ![]() $\boldsymbol {u}_{\perp }$ and
$\boldsymbol {u}_{\perp }$ and ![]() $\boldsymbol {b}_{\perp }$ are both closely aligned and have nearly the same amplitude (this is geometrically obvious from figure 38), but whether or not the Elsasser fields are aligned is up to the turbulence to decide. It does seem to decide to align them (see § 9.2, item (v)), hence the way in which I drew the field increments in figure 38.
$\boldsymbol {b}_{\perp }$ are both closely aligned and have nearly the same amplitude (this is geometrically obvious from figure 38), but whether or not the Elsasser fields are aligned is up to the turbulence to decide. It does seem to decide to align them (see § 9.2, item (v)), hence the way in which I drew the field increments in figure 38.

Figure 39. A direct test of the relations (B6) in globally balanced (![]() $\varepsilon ^+/\varepsilon ^-=1$, panels (a,c)) and imbalanced (
$\varepsilon ^+/\varepsilon ^-=1$, panels (a,c)) and imbalanced (![]() $\varepsilon ^+/\varepsilon ^-=10$, panels (b,d)) RMHD simulations by Mallet & Schekochihin (Reference Mallet and Schekochihin2011) (these are the same unpublished simulations as tabulated in figure 19). The plots show histograms of
$\varepsilon ^+/\varepsilon ^-=10$, panels (b,d)) RMHD simulations by Mallet & Schekochihin (Reference Mallet and Schekochihin2011) (these are the same unpublished simulations as tabulated in figure 19). The plots show histograms of ![]() $4\sin ^2\theta /R_{\mathrm {E}}\sin ^2\theta ^{ub}$ (a,b) and
$4\sin ^2\theta /R_{\mathrm {E}}\sin ^2\theta ^{ub}$ (a,b) and ![]() $(1-R_{\mathrm {A}})^2R_{\mathrm {E}}/16\cos ^2\theta$ (c,d) vs. perpendicular point separation
$(1-R_{\mathrm {A}})^2R_{\mathrm {E}}/16\cos ^2\theta$ (c,d) vs. perpendicular point separation ![]() $\lambda$. Note that the first relation in (B6) is reasonably well satisfied even in the globally balanced simulation.
$\lambda$. Note that the first relation in (B6) is reasonably well satisfied even in the globally balanced simulation.
If ![]() $R_{\mathrm {E}}$ (equivalently,
$R_{\mathrm {E}}$ (equivalently, ![]() $\sigma _{\mathrm {c}}$) is independent of scale in the inertial range, as reported for the solar wind by Podesta & Borovsky (Reference Podesta and Borovsky2010) and Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020), then the first relation in (B6) implies that
$\sigma _{\mathrm {c}}$) is independent of scale in the inertial range, as reported for the solar wind by Podesta & Borovsky (Reference Podesta and Borovsky2010) and Chen et al. (Reference Chen, Bale, Bonnell, Borovikov, Bowen, Burgess, Case, Chandran, Dudok de Wit and Goetz2020), then the first relation in (B6) implies that ![]() $\theta ^{ub}$ and
$\theta ^{ub}$ and ![]() $\theta$ should have the same scaling. In numerical simulations, they appear to do so, approximately, in balanced turbulence (Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016), which, of course, is patch-wise imbalanced (§ 9.1), but not in the strongly imbalanced cases studied by Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) and Mallet & Schekochihin (Reference Mallet and Schekochihin2011) (see § 9.2). Accordingly, Mallet & Schekochihin (Reference Mallet and Schekochihin2011) found the dependence of
$\theta$ should have the same scaling. In numerical simulations, they appear to do so, approximately, in balanced turbulence (Mallet et al. Reference Mallet, Schekochihin, Chandran, Chen, Horbury, Wicks and Greenan2016), which, of course, is patch-wise imbalanced (§ 9.1), but not in the strongly imbalanced cases studied by Beresnyak & Lazarian (Reference Beresnyak and Lazarian2009b) and Mallet & Schekochihin (Reference Mallet and Schekochihin2011) (see § 9.2). Accordingly, Mallet & Schekochihin (Reference Mallet and Schekochihin2011) found the dependence of ![]() $\theta ^{ub}$ on
$\theta ^{ub}$ on ![]() $\lambda$ getting shallower with increased imbalance, as
$\lambda$ getting shallower with increased imbalance, as ![]() $R_{\mathrm {E}}$ vs.
$R_{\mathrm {E}}$ vs. ![]() $\lambda$ got steeper (see figure 19) and
$\lambda$ got steeper (see figure 19) and ![]() $\theta$ stayed approximately the same. Alas, those simulations are in all probability not in the asymptotic regime, so a follow-up study at higher resolutions would be very welcome.
$\theta$ stayed approximately the same. Alas, those simulations are in all probability not in the asymptotic regime, so a follow-up study at higher resolutions would be very welcome.
B.2. Alignment and reduction of nonlinearity
The nonlinear term in RMHD (3.1) can be expressed so:
 \begin{align} \boldsymbol{Z}_{{\perp}}^+ \cdot \boldsymbol{\nabla}_{{\perp}}\boldsymbol{Z}_{{\perp}}^- &= \boldsymbol{Z}_{{\perp}}^- \cdot \boldsymbol{\nabla}_{{\perp}}\boldsymbol{Z}_{{\perp}}^+ + \boldsymbol{\nabla}_{{\perp}}\times\left(\boldsymbol{Z}_{{\perp}}^- \times \boldsymbol{Z}_{{\perp}}^+\right) \nonumber\\ &= \boldsymbol{\nabla}_{{\perp}}\cdot\left(\boldsymbol{u}_{{\perp}}\boldsymbol{u}_{{\perp}} - \boldsymbol{b}_{{\perp}}\boldsymbol{b}_{{\perp}}\right) + \boldsymbol{\nabla}_{{\perp}}\times\left(\boldsymbol{u}_{{\perp}}\times\boldsymbol{b}_{{\perp}}\right). \end{align}
\begin{align} \boldsymbol{Z}_{{\perp}}^+ \cdot \boldsymbol{\nabla}_{{\perp}}\boldsymbol{Z}_{{\perp}}^- &= \boldsymbol{Z}_{{\perp}}^- \cdot \boldsymbol{\nabla}_{{\perp}}\boldsymbol{Z}_{{\perp}}^+ + \boldsymbol{\nabla}_{{\perp}}\times\left(\boldsymbol{Z}_{{\perp}}^- \times \boldsymbol{Z}_{{\perp}}^+\right) \nonumber\\ &= \boldsymbol{\nabla}_{{\perp}}\cdot\left(\boldsymbol{u}_{{\perp}}\boldsymbol{u}_{{\perp}} - \boldsymbol{b}_{{\perp}}\boldsymbol{b}_{{\perp}}\right) + \boldsymbol{\nabla}_{{\perp}}\times\left(\boldsymbol{u}_{{\perp}}\times\boldsymbol{b}_{{\perp}}\right). \end{align}The first of these identities already appeared as (6.2). As I already explained in § 6.1, it says, schematically,
which makes the connection between Elsasser-field alignment (![]() $\sin \theta \ll 1$) and reduction of nonlinearity (
$\sin \theta \ll 1$) and reduction of nonlinearity (![]() $\xi ^\pm \gg \lambda$) a consistent choice (although not a mathematically inevitable one). This conclusion is valid regardless of the degree of imbalance.
$\xi ^\pm \gg \lambda$) a consistent choice (although not a mathematically inevitable one). This conclusion is valid regardless of the degree of imbalance.
Consider now the second identity in (B7). Without imbalance, i.e., if ![]() $\delta Z_\lambda ^+\sim \delta Z_\lambda ^-\sim \delta u_\lambda \sim \delta b_\lambda$, it implies, analogously to (B8),
$\delta Z_\lambda ^+\sim \delta Z_\lambda ^-\sim \delta u_\lambda \sim \delta b_\lambda$, it implies, analogously to (B8),
where ![]() $\xi ^u$ and
$\xi ^u$ and ![]() $\xi ^b$ are the characteristic scales of variation of
$\xi ^b$ are the characteristic scales of variation of ![]() $\boldsymbol {u}_{\perp }$ and
$\boldsymbol {u}_{\perp }$ and ![]() $\boldsymbol {b}_{\perp }$, respectively, along themselves. Again it is a consistent choice to posit that
$\boldsymbol {b}_{\perp }$, respectively, along themselves. Again it is a consistent choice to posit that ![]() $\xi ^u\sim \xi ^b\sim \xi ^\pm$ and
$\xi ^u\sim \xi ^b\sim \xi ^\pm$ and ![]() $\lambda /\xi \sim \sin \theta ^{ub}\ll 1$. In contrast, if (locally or globally)
$\lambda /\xi \sim \sin \theta ^{ub}\ll 1$. In contrast, if (locally or globally) ![]() $\sqrt {R_{\mathrm {E}}}=\delta Z_\lambda ^+/\delta Z_\lambda ^- \gg 1$, we have
$\sqrt {R_{\mathrm {E}}}=\delta Z_\lambda ^+/\delta Z_\lambda ^- \gg 1$, we have
 \begin{equation} \frac{\left(\delta Z_\lambda^+\right)^2}{\xi^-\sqrt{R_{\mathrm{E}}}} \sim \frac{\delta u_\lambda^2(1-R_{\mathrm{A}})}{\xi^{u,b}} + \frac{\delta u_\lambda\delta b_\lambda\sin\theta^{ub}}{\lambda} \sim \left(\frac{\cos\theta}{\xi^{u,b}} + \frac{\sin\theta}{\lambda}\right) \frac{\left(\delta Z_\lambda^+\right)^2}{\sqrt{R_{\mathrm{E}}}}, \end{equation}
\begin{equation} \frac{\left(\delta Z_\lambda^+\right)^2}{\xi^-\sqrt{R_{\mathrm{E}}}} \sim \frac{\delta u_\lambda^2(1-R_{\mathrm{A}})}{\xi^{u,b}} + \frac{\delta u_\lambda\delta b_\lambda\sin\theta^{ub}}{\lambda} \sim \left(\frac{\cos\theta}{\xi^{u,b}} + \frac{\sin\theta}{\lambda}\right) \frac{\left(\delta Z_\lambda^+\right)^2}{\sqrt{R_{\mathrm{E}}}}, \end{equation}
where at the last step, I used both of the geometric relations (B6) and ![]() $\delta u_\lambda \sim \delta b_\lambda \sim \delta Z_\lambda ^+$. Again, the connection between reduced nonlinearity and alignment is consistent, but it is the alignment of Elsasser fields that matters (if
$\delta u_\lambda \sim \delta b_\lambda \sim \delta Z_\lambda ^+$. Again, the connection between reduced nonlinearity and alignment is consistent, but it is the alignment of Elsasser fields that matters (if ![]() $\sin \theta \sim 1$,
$\sin \theta \sim 1$, ![]() $\xi ^-\sim \lambda$, so no reduction). The alignment between
$\xi ^-\sim \lambda$, so no reduction). The alignment between ![]() $\boldsymbol {u}_{\perp }$ and
$\boldsymbol {u}_{\perp }$ and ![]() $\boldsymbol {b}_{\perp }$ is geometrically inevitable, but does not by itself imply anything about a reduction of nonlinearity.
$\boldsymbol {b}_{\perp }$ is geometrically inevitable, but does not by itself imply anything about a reduction of nonlinearity.
This is all perhaps too obvious to have needed spelling out. To summarise, in balanced turbulence (or rather in balanced regions within turbulence), which type of alignment one prefers to think about, or measure, does not appear to make a qualitative difference, whereas in the presence of imbalance, Elsasser alignment is a natural choice. Since both globally balanced and imbalanced turbulence is likely to be imbalanced locally (see § 9.1), and indeed since it is in locally imbalanced patches that most of the ‘action’ is likely to be, I have made Elsasser alignment synonymous with ‘alignment’ everywhere in this review.
As I stated repeatedly in the main text, the supporting physical (dynamical) argument is to think of alignment as a result of mutual shearing of Elsasser fields, following Chandran et al. (Reference Chandran, Schekochihin and Mallet2015). Such a shearing will lead to simultaneous reduction of ![]() $\lambda /\xi$ and
$\lambda /\xi$ and ![]() $\sin \theta$. This approach is circumstantially supported by the ‘refined critical balance’ discovered by Mallet et al. (Reference Mallet, Schekochihin and Chandran2015) – the remarkable self-similarity shown by the ratio
$\sin \theta$. This approach is circumstantially supported by the ‘refined critical balance’ discovered by Mallet et al. (Reference Mallet, Schekochihin and Chandran2015) – the remarkable self-similarity shown by the ratio ![]() $\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}^\pm$, with
$\tau _{\mathrm {A}}/\tau _{\mathrm {nl}}^\pm$, with ![]() $\tau _{\mathrm {nl}}^\pm$ defined by (6.4), using the angle between the Elsasser fields (see figure 6). Arguably, this says that it is this
$\tau _{\mathrm {nl}}^\pm$ defined by (6.4), using the angle between the Elsasser fields (see figure 6). Arguably, this says that it is this ![]() $\tau _{\mathrm {nl}}^\pm$ that
$\tau _{\mathrm {nl}}^\pm$ that ![]() $\tau _{\mathrm {A}}$ (and, therefore,
$\tau _{\mathrm {A}}$ (and, therefore, ![]() $l_{\parallel }^\pm$) ‘knows’about, so it is this
$l_{\parallel }^\pm$) ‘knows’about, so it is this ![]() $\tau _{\mathrm {nl}}^\pm$ that should be viewed as the decorrelation (cascade) time of the turbulent structures. The same paper spotted a pronounced anticorrelation, at a given
$\tau _{\mathrm {nl}}^\pm$ that should be viewed as the decorrelation (cascade) time of the turbulent structures. The same paper spotted a pronounced anticorrelation, at a given ![]() $\lambda$, between the Elsasser alignment angle
$\lambda$, between the Elsasser alignment angle ![]() $\theta$ and the magnitudes of the Elsasser-field increments – as noted in § 6.3, this is again consistent with the shearing origin of alignment.
$\theta$ and the magnitudes of the Elsasser-field increments – as noted in § 6.3, this is again consistent with the shearing origin of alignment.
Another useful way to look at the relationship between alignment and nonlinearity is afforded by casting RMHD in the form (A2), in terms of Elsasser potentials and vorticities. Alignment between ![]() $\boldsymbol {Z}_{\perp }^+$ and
$\boldsymbol {Z}_{\perp }^+$ and ![]() $\boldsymbol {Z}_{\perp }^-$ is equivalent to alignment between
$\boldsymbol {Z}_{\perp }^-$ is equivalent to alignment between ![]() $\boldsymbol {\nabla }_{\perp }\zeta ^+$ and
$\boldsymbol {\nabla }_{\perp }\zeta ^+$ and ![]() $\boldsymbol {\nabla }_{\perp }\zeta ^-$, so small
$\boldsymbol {\nabla }_{\perp }\zeta ^-$, so small ![]() $\sin \theta$ will imply smallness of the second term on the right-hand side of (A2). The first term,
$\sin \theta$ will imply smallness of the second term on the right-hand side of (A2). The first term, ![]() $\left \{\zeta ^\mp,\omega ^\pm \right \}$, is small if
$\left \{\zeta ^\mp,\omega ^\pm \right \}$, is small if ![]() $\boldsymbol {\nabla }_{\perp }\zeta ^\mp$ and
$\boldsymbol {\nabla }_{\perp }\zeta ^\mp$ and ![]() $\boldsymbol {\nabla }_{\perp }\omega ^\pm$ are approximately aligned, i.e., if contours of constant
$\boldsymbol {\nabla }_{\perp }\omega ^\pm$ are approximately aligned, i.e., if contours of constant ![]() $\zeta ^\mp$ and
$\zeta ^\mp$ and ![]() $\omega ^\pm$ approximately coincide. The latter condition is indeed satisfied for perturbations that resemble tubes or sheets – and thus for perturbations that are likely to emerge from mutual shearing of Elsasser fields (this observation is due to Bowen et al. Reference Bowen, Badman, Bale, Dudok de Wit, Horbury, Klein, Larson, Mallet, Matteini and McManus2021).
$\omega ^\pm$ approximately coincide. The latter condition is indeed satisfied for perturbations that resemble tubes or sheets – and thus for perturbations that are likely to emerge from mutual shearing of Elsasser fields (this observation is due to Bowen et al. Reference Bowen, Badman, Bale, Dudok de Wit, Horbury, Klein, Larson, Mallet, Matteini and McManus2021).
Finally, let me reiterate that Elsasser alignment is not formally obliged to be co-located with locally imbalanced regions, even if observations by Wicks et al. (Reference Wicks, Roberts, Mallet, Schekochihin, Horbury and Chen2013b) discussed in § 9.1 suggest that it might be, and even though the reduction of nonlinearity in the aligned MHD cascade has originally been argued to be connected to local enhancements of cross-helicity (see footnote 16 and references therein). In numerical simulations, it remains to be checked whether such an intrinsic connection does exist.
Appendix C. 2D spectra of RMHD turbulence
As we trade in ![]() $k_{\perp }$ (or
$k_{\perp }$ (or ![]() $\lambda$) and
$\lambda$) and ![]() $k_{\parallel }$ (or
$k_{\parallel }$ (or ![]() $l_{\parallel }$) scalings, it is only natural that we might wish to have 2D spectra of RMHD turbulence,
$l_{\parallel }$) scalings, it is only natural that we might wish to have 2D spectra of RMHD turbulence, ![]() $E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$. It is quite easy to work them out, given the information we already have about the
$E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$. It is quite easy to work them out, given the information we already have about the ![]() $\lambda$ and
$\lambda$ and ![]() $l_{\parallel }$ scalings of the Elsasser increments.
$l_{\parallel }$ scalings of the Elsasser increments.
Since, as I explained in § 5.3, the physically meaningful parallel correlations are along the local mean field, we should think of our Elsasser fields ![]() $\boldsymbol {Z}_{\perp }^\pm$ as being mapped on a grid of values of
$\boldsymbol {Z}_{\perp }^\pm$ as being mapped on a grid of values of ![]() $(\boldsymbol {r}_{\perp },r_{\parallel })$, where
$(\boldsymbol {r}_{\perp },r_{\parallel })$, where ![]() $r_{\parallel }$ is the distance measured along the exact field line (what matters here is not that the parallel distances are slightly longer than their projection on the
$r_{\parallel }$ is the distance measured along the exact field line (what matters here is not that the parallel distances are slightly longer than their projection on the ![]() $z$ axis – the difference is small in the RMHD ordering – but that we probe correlations along the exact field line rather than slipping off it; see figure 8). The Fourier transform of
$z$ axis – the difference is small in the RMHD ordering – but that we probe correlations along the exact field line rather than slipping off it; see figure 8). The Fourier transform of ![]() $\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },r_{\parallel })$ is a function of
$\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },r_{\parallel })$ is a function of ![]() $k_{\perp }$ and
$k_{\perp }$ and ![]() $k_{\parallel }$,
$k_{\parallel }$, ![]() $\boldsymbol {Z}_{\perp }^\pm ({\boldsymbol {k}}_{\perp },k_{\parallel })$, and the 2D spectrum is defined to be
$\boldsymbol {Z}_{\perp }^\pm ({\boldsymbol {k}}_{\perp },k_{\parallel })$, and the 2D spectrum is defined to be
Let us start with the premise that ![]() $E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ will be a product of power laws in both of its arguments and that the scaling exponents of these power laws will be different depending on where we are in the
$E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ will be a product of power laws in both of its arguments and that the scaling exponents of these power laws will be different depending on where we are in the ![]() $(k_{\perp },k_{\parallel })$ space vis-à-vis the line of critical balance, which is also a power-law relation, between
$(k_{\perp },k_{\parallel })$ space vis-à-vis the line of critical balance, which is also a power-law relation, between ![]() $k_{\perp }$ and
$k_{\perp }$ and ![]() $k_{\parallel }$:
$k_{\parallel }$:
We shall treat the wavenumbers as dimensionless, ![]() $k_{\parallel} L _{\parallel }\to k _{\parallel }$,
$k_{\parallel} L _{\parallel }\to k _{\parallel }$, ![]() $k_{\perp }\lambda _{\mathrm {CB}}\to k _{\perp }$. According to (6.22),
$k_{\perp }\lambda _{\mathrm {CB}}\to k _{\perp }$. According to (6.22),
Thus, we shall look for the 2D spectrum in the form
 \begin{equation} E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \left\{\begin{array}{@{}ll} k_{{\parallel}}^{-\alpha}k_{{\perp}}^\beta, & k_{{\parallel}} \gtrsim k_{{\perp}}^\sigma,\\\\ k_{{\parallel}}^{\delta}k_{{\perp}}^{-\gamma}, & k_{{\parallel}} \lesssim k_{{\perp}}^\sigma. \end{array}\right. \end{equation}
\begin{equation} E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \left\{\begin{array}{@{}ll} k_{{\parallel}}^{-\alpha}k_{{\perp}}^\beta, & k_{{\parallel}} \gtrsim k_{{\perp}}^\sigma,\\\\ k_{{\parallel}}^{\delta}k_{{\perp}}^{-\gamma}, & k_{{\parallel}} \lesssim k_{{\perp}}^\sigma. \end{array}\right. \end{equation}
The four exponents ![]() $\alpha$,
$\alpha$, ![]() $\beta$,
$\beta$, ![]() $\gamma$, and
$\gamma$, and ![]() $\delta$ can be determined by the following arguments, broadly analogous to those proposed by Schekochihin et al. (Reference Schekochihin, Parker, Highcock, Dellar, Dorland and Hammett2016) for drift-kinetic turbulence except for the calculation of
$\delta$ can be determined by the following arguments, broadly analogous to those proposed by Schekochihin et al. (Reference Schekochihin, Parker, Highcock, Dellar, Dorland and Hammett2016) for drift-kinetic turbulence except for the calculation of ![]() $\beta$ (and hence of
$\beta$ (and hence of ![]() $\alpha$), which will be significantly modified here in light of some new theoretical developments (Appendix C.3).
$\alpha$), which will be significantly modified here in light of some new theoretical developments (Appendix C.3).
C.1. Determining  $\delta$: long parallel wavelengths
$\delta$: long parallel wavelengths
At long parallel wavelengths, ![]() $k_{\parallel }\ll k _{\perp }^\sigma$, the
$k_{\parallel }\ll k _{\perp }^\sigma$, the ![]() $k_{\parallel }$ spectrum measures correlation between points along the field line that are separated by longer distances than an Alfvén wave can travel in one nonlinear time (
$k_{\parallel }$ spectrum measures correlation between points along the field line that are separated by longer distances than an Alfvén wave can travel in one nonlinear time (![]() $\tau _{\mathrm {A}}\gg \tau _{\mathrm {nl}}$) and, consequently, are causally disconnected (§ 5.1). Therefore, their parallel correlation function is that of a 1D white noise and the corresponding spectrum is flat:
$\tau _{\mathrm {A}}\gg \tau _{\mathrm {nl}}$) and, consequently, are causally disconnected (§ 5.1). Therefore, their parallel correlation function is that of a 1D white noise and the corresponding spectrum is flat:
It may be worth belabouring this point: the flat ![]() $k_{\parallel }$ spectrum at
$k_{\parallel }$ spectrum at ![]() $k_{\parallel }\lesssim k _{\perp }^\sigma$ (figure 40b) is the Fourier-space signature of CB turbulence, not an indication of the presence of quasi-2D motions or of failure of local-in-scale interactions (as, e.g., Meyrand et al. Reference Meyrand, Galtier and Kiyani2016 appear to imply). This highlights the fact that the wavenumbers where energy is present are not quite the same thing as the correlation scales of the turbulent field, and so one should not expect that CB requires a spectrum peaked at
$k_{\parallel }\lesssim k _{\perp }^\sigma$ (figure 40b) is the Fourier-space signature of CB turbulence, not an indication of the presence of quasi-2D motions or of failure of local-in-scale interactions (as, e.g., Meyrand et al. Reference Meyrand, Galtier and Kiyani2016 appear to imply). This highlights the fact that the wavenumbers where energy is present are not quite the same thing as the correlation scales of the turbulent field, and so one should not expect that CB requires a spectrum peaked at ![]() $k_{\parallel }\sim k _{\perp }^\sigma$ (a fallacy that has made it into a number of published texts, rigorous peer review notwithstanding). The same argument applies to frequency spectra, should one want to plot them: there must be a flat spectrum at
$k_{\parallel }\sim k _{\perp }^\sigma$ (a fallacy that has made it into a number of published texts, rigorous peer review notwithstanding). The same argument applies to frequency spectra, should one want to plot them: there must be a flat spectrum at ![]() $\omega \lesssim \tau _{\mathrm {nl}}^{-1}$ because instances separated by times longer than
$\omega \lesssim \tau _{\mathrm {nl}}^{-1}$ because instances separated by times longer than ![]() $\tau _{\mathrm {nl}}$ are uncorrelated and will, therefore, have white-noise statistics.
$\tau _{\mathrm {nl}}$ are uncorrelated and will, therefore, have white-noise statistics.
C.2. Determining  $\gamma$: short perpendicular wavelengths
$\gamma$: short perpendicular wavelengths
Let us calculate the 1D ![]() $k_{\perp }$ spectrum: if we assume (and promise to check later) that
$k_{\perp }$ spectrum: if we assume (and promise to check later) that ![]() $\alpha >1$, then the
$\alpha >1$, then the ![]() $k_{\parallel }$ integral over
$k_{\parallel }$ integral over ![]() $E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ is dominated by the region
$E_{\mathrm {2D}}(k_{\perp },k_{\parallel })$ is dominated by the region ![]() $k_{\parallel }\lesssim k _{\perp }^\sigma$ and the 1D spectrum is mostly determined by the CB scales
$k_{\parallel }\lesssim k _{\perp }^\sigma$ and the 1D spectrum is mostly determined by the CB scales ![]() $k_{\parallel }\sim k _{\perp }^\sigma$ (as is indeed argued in the GS95 theory and its descendants reviewed in the main text):
$k_{\parallel }\sim k _{\perp }^\sigma$ (as is indeed argued in the GS95 theory and its descendants reviewed in the main text):
Then the amplitude of an Elsasser field at scale ![]() $\lambda =k_{\perp }^{-1}$ is
$\lambda =k_{\perp }^{-1}$ is
assuming ![]() $\gamma -\sigma > 1$. On the other hand, the usual Kolmogorov constant-flux condition coupled with the CB conjecture gives us
$\gamma -\sigma > 1$. On the other hand, the usual Kolmogorov constant-flux condition coupled with the CB conjecture gives us
Comparing this with (C7), we get
The 1D spectral exponent in (C6) is then ![]() $-\gamma +\sigma =-3/2$, as it should be (see (6.22)).
$-\gamma +\sigma =-3/2$, as it should be (see (6.22)).
C.3. Determining  $\beta$: long perpendicular wavelengths
$\beta$: long perpendicular wavelengths
This turns out to be a somewhat subtle issue, connected to an interesting recent development in turbulence theory.
C.3.1. Kinematics
Consider first the following naïve, purely kinematic calculation. Let us write the desired spectrum (C1) as
 \begin{align} \langle|\boldsymbol{Z}_{{\perp}}^\pm({\boldsymbol{k}}_{{\perp}},k_{{\parallel}})|^2\rangle &= \int\frac{\mathrm{d}^2\boldsymbol{r}_{{\perp}}}{(2\pi)^2}\,e^{{-}i{\boldsymbol{k}}_{{\perp}}\cdot\boldsymbol{r}_{{\perp}}} \langle\boldsymbol{Z}_{{\perp}}^\pm(\boldsymbol{r}_{{\perp}},k_{{\parallel}})\cdot\boldsymbol{Z}_{{\perp}}^{{\pm} *}(0,k_{{\parallel}})\rangle \nonumber\\ &= \frac{1}{2\pi}\int_0^\infty\mathrm{d}r_{{\perp}}r_{{\perp}} J_0(k_{{\perp}}r_{{\perp}}) C^\pm(r_{{\perp}},k_{{\parallel}}), \end{align}
\begin{align} \langle|\boldsymbol{Z}_{{\perp}}^\pm({\boldsymbol{k}}_{{\perp}},k_{{\parallel}})|^2\rangle &= \int\frac{\mathrm{d}^2\boldsymbol{r}_{{\perp}}}{(2\pi)^2}\,e^{{-}i{\boldsymbol{k}}_{{\perp}}\cdot\boldsymbol{r}_{{\perp}}} \langle\boldsymbol{Z}_{{\perp}}^\pm(\boldsymbol{r}_{{\perp}},k_{{\parallel}})\cdot\boldsymbol{Z}_{{\perp}}^{{\pm} *}(0,k_{{\parallel}})\rangle \nonumber\\ &= \frac{1}{2\pi}\int_0^\infty\mathrm{d}r_{{\perp}}r_{{\perp}} J_0(k_{{\perp}}r_{{\perp}}) C^\pm(r_{{\perp}},k_{{\parallel}}), \end{align}
where ![]() $C^\pm (r_{\perp },k_{\parallel })$ is the two-point correlation function of
$C^\pm (r_{\perp },k_{\parallel })$ is the two-point correlation function of ![]() $\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })$. It is only a function of the point separation
$\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })$. It is only a function of the point separation ![]() $r_{\perp }$ because of statistical homogeneity and isotropy in the perpendicular plane. For any given
$r_{\perp }$ because of statistical homogeneity and isotropy in the perpendicular plane. For any given ![]() $k_{\parallel }$, it might seem reasonable, by the CB conjecture, to estimate the correlation length of the field to be
$k_{\parallel }$, it might seem reasonable, by the CB conjecture, to estimate the correlation length of the field to be ![]() $\lambda \sim k_{\parallel }^{-1/\sigma }$ and assume therefore that the integral in (C10) is effectively restricted by
$\lambda \sim k_{\parallel }^{-1/\sigma }$ and assume therefore that the integral in (C10) is effectively restricted by ![]() $C^\pm (r_{\perp },k_{\parallel })$ to
$C^\pm (r_{\perp },k_{\parallel })$ to ![]() $r_{\perp }\lesssim \lambda$. If we now let
$r_{\perp }\lesssim \lambda$. If we now let ![]() $k_{\perp }\lambda \ll 1$ (equivalently,
$k_{\perp }\lambda \ll 1$ (equivalently, ![]() $k_{\perp }^\sigma \ll k _{\parallel }$), then the Bessel function can be expanded in small argument:
$k_{\perp }^\sigma \ll k _{\parallel }$), then the Bessel function can be expanded in small argument: ![]() $J_0(k_{\perp }r_{\perp }) = 1 - k_{\perp }^2r_{\perp }^2/4 + \dots$. The spectrum (C1) is then
$J_0(k_{\perp }r_{\perp }) = 1 - k_{\perp }^2r_{\perp }^2/4 + \dots$. The spectrum (C1) is then
The first of these coefficients, ![]() $C_0 = \int \mathrm {d}^2\boldsymbol {r}_{\perp }\langle \boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })\cdot \boldsymbol {Z}_{\perp }^{\pm *}(0,k_{\parallel })\rangle$, vanishes if
$C_0 = \int \mathrm {d}^2\boldsymbol {r}_{\perp }\langle \boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })\cdot \boldsymbol {Z}_{\perp }^{\pm *}(0,k_{\parallel })\rangle$, vanishes if ![]() $\int \mathrm {d}^2\boldsymbol {r}_{\perp }\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })=0$, which seems to be a reasonable assumption for a solenoidal field (see (A1)) in a box. This leaves us with the series (C11) for
$\int \mathrm {d}^2\boldsymbol {r}_{\perp }\boldsymbol {Z}_{\perp }^\pm (\boldsymbol {r}_{\perp },k_{\parallel })=0$, which seems to be a reasonable assumption for a solenoidal field (see (A1)) in a box. This leaves us with the series (C11) for ![]() $E_{\mathrm {2D}}$ starting at the second term and so
$E_{\mathrm {2D}}$ starting at the second term and so ![]() $E_{\mathrm {2D}}\propto k_{\perp }^3$ to lowest order. Thus,
$E_{\mathrm {2D}}\propto k_{\perp }^3$ to lowest order. Thus,
I have added a question mark to this statement because it is, in fact, not as straightforwardly obvious as the kinematic argument suggests.
C.3.2. Thermodynamics
What if one applies to the long perpendicular scales the same logic as I did to long parallel scales in Appendix C.1? If the fields are decorrelated at scales longer than the CB scale, i.e., at ![]() $k_{\perp }\ll k_{\parallel }^{1/\sigma }$, assuming a 2D white-noise spectrum (at fixed
$k_{\perp }\ll k_{\parallel }^{1/\sigma }$, assuming a 2D white-noise spectrum (at fixed ![]() $k_{\parallel }$) would imply that
$k_{\parallel }$) would imply that
This is just a thermal equilibrium spectrum, with energy equipartitioned amongst all available ![]() ${\boldsymbol {k}}_{\perp }$'s. The tendency for a thermal spectrum to emerge at large scales has indeed been recently noticed in 3D forced hydrodynamic turbulence (where the corresponding spectrum is
${\boldsymbol {k}}_{\perp }$'s. The tendency for a thermal spectrum to emerge at large scales has indeed been recently noticed in 3D forced hydrodynamic turbulence (where the corresponding spectrum is ![]() $\propto k^2$; see Alexakis & Brachet Reference Alexakis and Brachet2019 and references therein).
$\propto k^2$; see Alexakis & Brachet Reference Alexakis and Brachet2019 and references therein).
C.3.3. Dynamics
How then does one reconcile the thermodynamical result (C14) with the kinematic one (C13)? Let me give here the RMHD analog of the argument proposed by Hosking & Schekochihin (Reference Hosking and Schekochihin2022b) for hydrodynamic turbulence. The effect of the energy-containing scales (in our case, of the CB scales ![]() $k_{\parallel }\sim k _{\perp }^\sigma$) on the longer perpendicular scales is twofold: modes with larger
$k_{\parallel }\sim k _{\perp }^\sigma$) on the longer perpendicular scales is twofold: modes with larger ![]() ${\boldsymbol {k}}_{\perp }$'s couple (‘beat’) to feed those with smaller
${\boldsymbol {k}}_{\perp }$'s couple (‘beat’) to feed those with smaller ![]() ${\boldsymbol {k}}_{\perp }$'s, thus creating long-scale perturbations; and those long-scale perturbations are mixed to even longer scales by the turbulent diffusion arising from the energy-containing-scale fields. It turns out that the balance between these two effects produces the thermal spectrum (C14), while pushing the kinematic asymptotic (C13) to ever smaller
${\boldsymbol {k}}_{\perp }$'s, thus creating long-scale perturbations; and those long-scale perturbations are mixed to even longer scales by the turbulent diffusion arising from the energy-containing-scale fields. It turns out that the balance between these two effects produces the thermal spectrum (C14), while pushing the kinematic asymptotic (C13) to ever smaller ![]() $k_{\perp }$'s as time goes on.
$k_{\perp }$'s as time goes on.
Thanks to Appendix A, I already have the analytical tools to demonstrate this (semi)quantitatively. Since we are dealing with modes for which ![]() $\tau _{\mathrm {nl}}^{-1}\ll k_{\parallel} v _{\mathrm {A}}$, it is not unreasonable to apply the WT approximation to their description (with the usual disclaimer about its formal breakdown wherever it involves CB perturbations). Let us therefore consider (A18), but now at wavenumbers
$\tau _{\mathrm {nl}}^{-1}\ll k_{\parallel} v _{\mathrm {A}}$, it is not unreasonable to apply the WT approximation to their description (with the usual disclaimer about its formal breakdown wherever it involves CB perturbations). Let us therefore consider (A18), but now at wavenumbers ![]() $k_{\perp }$ that are much smaller than the wavenumbers at which
$k_{\perp }$ that are much smaller than the wavenumbers at which ![]() $E_{\mathrm {2D}}^\pm (q_{\perp },k_{\parallel })$ or
$E_{\mathrm {2D}}^\pm (q_{\perp },k_{\parallel })$ or ![]() $E_{\mathrm {2D}}^\pm (q_{\perp },0)$ contain most of their energy. Then, in (A18), the integral over
$E_{\mathrm {2D}}^\pm (q_{\perp },0)$ contain most of their energy. Then, in (A18), the integral over ![]() $q_{\perp }$ can be assumed to be dominated by
$q_{\perp }$ can be assumed to be dominated by ![]() $q_{\perp }\gg k_{\perp }$, and the integral over
$q_{\perp }\gg k_{\perp }$, and the integral over ![]() $p_{\perp }=q_{\perp } + \kappa$ is, therefore,
$p_{\perp }=q_{\perp } + \kappa$ is, therefore,
 \begin{align} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}} \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}^2}\sin\phi\cos^2\phi\,E_{\mathrm{2D}}^\mp(p_{{\perp}},0) &\approx E_{\mathrm{2D}}^\mp(q_{{\perp}},0) \int_{{-}k_{{\perp}}}^{{+}k_{{\perp}}}\mathrm{d}\kappa\,\kappa^2\sqrt{1-\frac{\kappa^2}{k_{{\perp}}^2}} \nonumber\\ & = \frac{\pi}{8}k_{{\perp}}^3 E_{\mathrm{2D}}^\mp(q_{{\perp}},0). \end{align}
\begin{align} \int_{|k_{{\perp}}-q_{{\perp}}|}^{k_{{\perp}}+q_{{\perp}}}\mathrm{d}p_{{\perp}} \frac{k_{{\perp}}^2q_{{\perp}}^2}{p_{{\perp}}^2}\sin\phi\cos^2\phi\,E_{\mathrm{2D}}^\mp(p_{{\perp}},0) &\approx E_{\mathrm{2D}}^\mp(q_{{\perp}},0) \int_{{-}k_{{\perp}}}^{{+}k_{{\perp}}}\mathrm{d}\kappa\,\kappa^2\sqrt{1-\frac{\kappa^2}{k_{{\perp}}^2}} \nonumber\\ & = \frac{\pi}{8}k_{{\perp}}^3 E_{\mathrm{2D}}^\mp(q_{{\perp}},0). \end{align}This turns the WT equation (A18) into
The left-hand side of (C16) features the usual turbulent diffusion with diffusivity
due entirely to the ![]() $q_{\parallel }=0$ modes – but these, in the context, are just the CB modes with
$q_{\parallel }=0$ modes – but these, in the context, are just the CB modes with ![]() $q_{\parallel }\sim q _{\perp }^\sigma$ (cf. Appendix A.4). The right-hand side of (C16) contains the effect of energy-containing-scale modes coupling to feed the long-scale spectrum:
$q_{\parallel }\sim q _{\perp }^\sigma$ (cf. Appendix A.4). The right-hand side of (C16) contains the effect of energy-containing-scale modes coupling to feed the long-scale spectrum:
The fact that this enters in (C16) with a prefactor of ![]() $k_{\perp }^3$ is a reflection of the fields being solenoidal and thus requiring the kinematic asymptotic (C13) at
$k_{\perp }^3$ is a reflection of the fields being solenoidal and thus requiring the kinematic asymptotic (C13) at ![]() $k_{\perp }\to 0$.
$k_{\perp }\to 0$.
The solution of (C16) (starting from zero initial condition) is
 \begin{align} E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}}) = k_{{\perp}}\left(1 - e^{{-}D^\pm k_{{\perp}}^2 t}\right) \frac{F^\pm(k_{{\parallel}})}{D^\pm} \to\left\{\begin{array}{ll} k_{{\perp}}^3 tF^\pm(k_{{\parallel}}), & k_{{\perp}} \ll (D^\pm t)^{{-}1/2},\\\\ k_{{\perp}} F^\pm(k_{{\parallel}})/D^\pm, & k_{{\perp}} \gg (D^\pm t)^{{-}1/2},\\ \end{array}\right. \end{align}
\begin{align} E_{\mathrm{2D}}^\pm(k_{{\perp}},k_{{\parallel}}) = k_{{\perp}}\left(1 - e^{{-}D^\pm k_{{\perp}}^2 t}\right) \frac{F^\pm(k_{{\parallel}})}{D^\pm} \to\left\{\begin{array}{ll} k_{{\perp}}^3 tF^\pm(k_{{\parallel}}), & k_{{\perp}} \ll (D^\pm t)^{{-}1/2},\\\\ k_{{\perp}} F^\pm(k_{{\parallel}})/D^\pm, & k_{{\perp}} \gg (D^\pm t)^{{-}1/2},\\ \end{array}\right. \end{align}
the latter asymptotic being the steady-state solution. Thus, the kinematic asymptotic (C13) does exist, but is constantly pushed to larger scales as time goes on. Formally this means that the correlation scale ![]() $\lambda$ above which the correlation function
$\lambda$ above which the correlation function ![]() $C^\pm (r_{\perp },r_{\parallel })$ in (C10) decays sufficiently fast with
$C^\pm (r_{\perp },r_{\parallel })$ in (C10) decays sufficiently fast with ![]() $r_{\perp }$ to enable the expansion (C11), is not the CB scale
$r_{\perp }$ to enable the expansion (C11), is not the CB scale ![]() $\lambda \sim k_{\parallel }^{-1/\sigma }$ as naïvely assumed in Appendix C.3.1, but rather
$\lambda \sim k_{\parallel }^{-1/\sigma }$ as naïvely assumed in Appendix C.3.1, but rather ![]() $\lambda \sim (D^\pm t)^{1/2}$. Since I am interested in asymptotically long times, I will adopt the thermal spectrum (C14) in what follows.
$\lambda \sim (D^\pm t)^{1/2}$. Since I am interested in asymptotically long times, I will adopt the thermal spectrum (C14) in what follows.
C.4. Determining  $\alpha$: short parallel wavelengths
$\alpha$: short parallel wavelengths
Finally, ![]() $\alpha$ is determined simply by the requirement that the 2D spectra match along the CB line: substituting
$\alpha$ is determined simply by the requirement that the 2D spectra match along the CB line: substituting ![]() $k_{\parallel }\sim k _{\perp }^\sigma$ into (C4) and equating powers of
$k_{\parallel }\sim k _{\perp }^\sigma$ into (C4) and equating powers of ![]() $k_{\perp }$, we get
$k_{\perp }$, we get
This somewhat ridiculous exponentFootnote 96 suggests that there is very little energy indeed in wave-like perturbations with ![]() $\tau _{\mathrm {A}}\ll \tau _{\mathrm {nl}}$.
$\tau _{\mathrm {A}}\ll \tau _{\mathrm {nl}}$.
Note that the consistency of what I have done above can be checked by calculating the 1D ![]() $k_{\parallel }$ spectrum:
$k_{\parallel }$ spectrum:
 \begin{equation} E(k_{{\parallel}}) = \int\mathrm{d}k_{{\perp}}E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \int_0^{k_{{\parallel}}^{1/\sigma}}\mathrm{d}k_{{\perp}} k_{{\parallel}}^{-\alpha}k_{{\perp}}^\beta + \int_{k_{{\parallel}}^{1/\sigma}}^\infty\mathrm{d}k_{{\perp}} k_{{\parallel}}^\delta k_{{\perp}}^{-\gamma} \sim k_{{\parallel}}^{-\zeta}, \end{equation}
\begin{equation} E(k_{{\parallel}}) = \int\mathrm{d}k_{{\perp}}E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \int_0^{k_{{\parallel}}^{1/\sigma}}\mathrm{d}k_{{\perp}} k_{{\parallel}}^{-\alpha}k_{{\perp}}^\beta + \int_{k_{{\parallel}}^{1/\sigma}}^\infty\mathrm{d}k_{{\perp}} k_{{\parallel}}^\delta k_{{\perp}}^{-\gamma} \sim k_{{\parallel}}^{-\zeta}, \end{equation}where
as it should be (see § 5.2).
To summarise, the 2D spectrum (C4) of critically balanced Alfvénic turbulence is
 \begin{align} E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \left\{\begin{array}{@{}ll} k_{{\parallel}}^{{-}6}k_{{\perp}}^1, & k_{{\parallel}} \gtrsim k_{{\perp}}^{1/2},\\ k_{{\parallel}}^0k_{{\perp}}^{{-}2}, & k_{{\parallel}} \lesssim k_{{\perp}}^{1/2}, \end{array}\right. \end{align}
\begin{align} E_{\mathrm{2D}}(k_{{\perp}},k_{{\parallel}}) \sim \left\{\begin{array}{@{}ll} k_{{\parallel}}^{{-}6}k_{{\perp}}^1, & k_{{\parallel}} \gtrsim k_{{\perp}}^{1/2},\\ k_{{\parallel}}^0k_{{\perp}}^{{-}2}, & k_{{\parallel}} \lesssim k_{{\perp}}^{1/2}, \end{array}\right. \end{align}
leading to 1D spectra ![]() $E(k_{\perp })\sim k_{\perp }^{-3/2}$ and
$E(k_{\perp })\sim k_{\perp }^{-3/2}$ and ![]() $E(k_{\parallel })\sim k _{\parallel }^{-2}$. The spectra (C23) are sketched in figure 40.
$E(k_{\parallel })\sim k _{\parallel }^{-2}$. The spectra (C23) are sketched in figure 40.
I leave it as an exercise for the reader to show that if the same scheme is applied to the tearing-mediated turbulence described in § 7.2 (starting with (7.22) for ![]() $\sigma$), the exponents in (C4) are
$\sigma$), the exponents in (C4) are
and ![]() $\zeta =2$, unchanged from (C22) (as it should be, according to § 7.2.4).
$\zeta =2$, unchanged from (C22) (as it should be, according to § 7.2.4).
C.5. 2D spectrum of WT
The 2D spectrum of broad-band-forced WT determined in Appendix A.4 can easily be obtained by arguments analogous to the above:
for the same reason as in Appendix C.1,
similarly to Appendix C.2, but now employing the non-constant-flux argument (A27),
by the WT condition (A23) with ![]() $\mu _0 = -\gamma$ and
$\mu _0 = -\gamma$ and ![]() $\mu = \beta$ instead of the calculation of Appendix C.3 (which would only apply above the perpendicular scale of the forcing), and, finally, matching the spectra as in Appendix C.4, we get
$\mu = \beta$ instead of the calculation of Appendix C.3 (which would only apply above the perpendicular scale of the forcing), and, finally, matching the spectra as in Appendix C.4, we get
Here we can either set ![]() $\sigma = 1$ by assuming a non-aligned cascade of the condensate, as in (A29), and hence get
$\sigma = 1$ by assuming a non-aligned cascade of the condensate, as in (A29), and hence get ![]() $\alpha = 0$, or set
$\alpha = 0$, or set ![]() $\alpha = 0$ by assuming no parallel cascade and equal forcing at all
$\alpha = 0$ by assuming no parallel cascade and equal forcing at all ![]() $k_{\parallel }$ in the WT regime, in which case the matching condition (C28) requires
$k_{\parallel }$ in the WT regime, in which case the matching condition (C28) requires ![]() $\sigma =1$ (and so I would have had to contend with discontinuous spectra if, in Appendix A.4, I had allowed the condensate to have alignment). Thus,
$\sigma =1$ (and so I would have had to contend with discontinuous spectra if, in Appendix A.4, I had allowed the condensate to have alignment). Thus,
Note that the 2D spectrum in the strongly nonlinear region ![]() $k_{\parallel } \lesssim k_{\perp }^\sigma$ does not actually change at the transition from the WT to the CB turbulence (although the CB boundary does) – spectral continuity vindicated.
$k_{\parallel } \lesssim k_{\perp }^\sigma$ does not actually change at the transition from the WT to the CB turbulence (although the CB boundary does) – spectral continuity vindicated.
Appendix D. A reconnection primer
Since it is now clear that reconnection phenomena play an essential role in MHD turbulence, it is useful to provide a series of shortcuts to the key results. I will not do any precise calculations of the kind that make the theory of resistive MHD instabilities such a mathematically accomplished subject (what better example on which to teach an undergraduate class to solve ODEs with boundary layers than the many incarnations of the tearing mode!), but will instead go for ‘quick and dirty’ ways of getting at the right scalings. Readers yearning for more exactitude will find it, e.g., in a recent treatment by Boldyrev & Loureiro (Reference Boldyrev and Loureiro2018); those who prefer to get their instruction from the original source should start with the foundational papers by Furth et al. (Reference Furth, Killeen and Rosenbluth1963) and Coppi et al. (Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976).
When dealing with resistive MHD instabilities, it is convenient to write the RMHD equations in their original form (Strauss Reference Strauss1976), in terms of the stream (flux) functions for the velocity and magnetic fields:
Since the Elsasser potentials introduced in (A1) are just ![]() $\zeta ^\pm = \varPhi \pm \varPsi$, one can recover the equations for
$\zeta ^\pm = \varPhi \pm \varPsi$, one can recover the equations for ![]() $\varPhi$ and
$\varPhi$ and ![]() $\varPsi$ from (A2), or, indeed, use (D1) and derive them directly from the momentum and induction equations of MHD (see Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Oughton et al. Reference Oughton, Matthaeus and Dmitruk2017, and references therein):
$\varPsi$ from (A2), or, indeed, use (D1) and derive them directly from the momentum and induction equations of MHD (see Schekochihin et al. Reference Schekochihin, Cowley, Dorland, Hammett, Howes, Quataert and Tatsuno2009, Oughton et al. Reference Oughton, Matthaeus and Dmitruk2017, and references therein):
where the difference between the Ohmic diffusivity ![]() $\eta$ and viscosity
$\eta$ and viscosity ![]() $\nu$ has been restored.
$\nu$ has been restored.
D.1. Tearing instability
Let us ignore the parallel derivatives in (D2–D3) and consider small perturbations of a simple static equilibrium in which the in-plane magnetic field points in the ![]() $y$ direction and reverses direction at
$y$ direction and reverses direction at ![]() $x=0$:
$x=0$:
where ![]() $b_0(x)=\varPsi _0'(x)$ is an odd function describing the reversing field profile and
$b_0(x)=\varPsi _0'(x)$ is an odd function describing the reversing field profile and ![]() $\gamma$ is the rate at which perturbations of this profile will grow (if they are interesting). Now linearise the RMHD equations (D2–D3) and Fourier-transform them in the
$\gamma$ is the rate at which perturbations of this profile will grow (if they are interesting). Now linearise the RMHD equations (D2–D3) and Fourier-transform them in the ![]() $y$ direction:
$y$ direction:
When ![]() $\eta$ is small, this system has a boundary layer around
$\eta$ is small, this system has a boundary layer around ![]() $x=0$, of width
$x=0$, of width ![]() $\delta _{\mathrm {in}}$, outside which the solution is an ideal-MHD one and inside which resistivity is important and reconnection occurs.
$\delta _{\mathrm {in}}$, outside which the solution is an ideal-MHD one and inside which resistivity is important and reconnection occurs.
D.1.1. Outer solution
If we assume that the outer-region solution has scale ![]() $\lambda$ and
$\lambda$ and
where ![]() $v_{\mathrm {A}y} \equiv \lambda b_0'(0)$, then the outer solution satisfies
$v_{\mathrm {A}y} \equiv \lambda b_0'(0)$, then the outer solution satisfies
The first of these equations is the (perturbed) magnetic-force balance (inertial terms are neglected), the second describes the ideal-MHD advection of the equilibrium magnetic field by the perturbed flow.
Since ![]() $\psi$ is even and the magnetic field
$\psi$ is even and the magnetic field ![]() $b_y = \partial _x\psi$ must reverse direction at
$b_y = \partial _x\psi$ must reverse direction at ![]() $x=0$,
$x=0$, ![]() $\psi$ has a discontinuous derivative (figure 41). This corresponds to a singular current that is developed by the ideal-MHD solution as it approaches the boundary layer – with the singularity resolved inside the layer by resistivity. The solutions outside and inside the layer are matched to each other by equating the discontinuity in the former to the total change in
$\psi$ has a discontinuous derivative (figure 41). This corresponds to a singular current that is developed by the ideal-MHD solution as it approaches the boundary layer – with the singularity resolved inside the layer by resistivity. The solutions outside and inside the layer are matched to each other by equating the discontinuity in the former to the total change in ![]() $\partial _x\psi$ calculated from the latter:
$\partial _x\psi$ calculated from the latter:
 \begin{equation} \varDelta' \equiv \frac{\left[\partial_x\psi_\mathrm{out}\right]_{{-}0}^{{+}0}}{\psi_\mathrm{out}(0)} = \frac{2}{\delta_{\mathrm{in}}}\int_0^\infty\mathrm{d} X\,\frac{\partial_X^2\psi_\mathrm{in}(X)}{\psi_\mathrm{in}(0)}, \end{equation}
\begin{equation} \varDelta' \equiv \frac{\left[\partial_x\psi_\mathrm{out}\right]_{{-}0}^{{+}0}}{\psi_\mathrm{out}(0)} = \frac{2}{\delta_{\mathrm{in}}}\int_0^\infty\mathrm{d} X\,\frac{\partial_X^2\psi_\mathrm{in}(X)}{\psi_\mathrm{in}(0)}, \end{equation}
where ![]() $\psi _\mathrm {out}(x)=\psi (x)$ is the outer solution,
$\psi _\mathrm {out}(x)=\psi (x)$ is the outer solution, ![]() $\psi _\mathrm {in}(X)=\psi (X\delta _{\mathrm {in}})$ is the inner one, and
$\psi _\mathrm {in}(X)=\psi (X\delta _{\mathrm {in}})$ is the inner one, and ![]() $X = x/\delta _{\mathrm {in}}$ is the ‘inner’ variable, rescaled to the current layer's width
$X = x/\delta _{\mathrm {in}}$ is the ‘inner’ variable, rescaled to the current layer's width ![]() $\delta _{\mathrm {in}}$.
$\delta _{\mathrm {in}}$.

Figure 41. The outer solution for a tearing mode in a large-aspect-ratio sheet (adapted from Loureiro et al. Reference Loureiro, Schekochihin and Cowley2007). ![]() $\varDelta '$ measures the discontinuity of
$\varDelta '$ measures the discontinuity of ![]() $\partial _x\psi$ at
$\partial _x\psi$ at ![]() $x=0$ (see (D9)).
$x=0$ (see (D9)).
To find ![]() $\varDelta '$ from the outer solution, one must solve (D8) for some particular form of
$\varDelta '$ from the outer solution, one must solve (D8) for some particular form of ![]() $b_0(x)$. For our purposes, all that is needed is the asymptotic behaviour of
$b_0(x)$. For our purposes, all that is needed is the asymptotic behaviour of ![]() $\varDelta '$ in the limit of
$\varDelta '$ in the limit of ![]() $k_y\lambda \ll 1$, where
$k_y\lambda \ll 1$, where ![]() $\lambda$ is the characteristic scale of
$\lambda$ is the characteristic scale of ![]() $b_0(x)$. While in general this asymptotic depends on the functional form of
$b_0(x)$. While in general this asymptotic depends on the functional form of ![]() $b_0(x)$, it is
$b_0(x)$, it is
if one can assume that ![]() $b_0(x)$ varies faster at
$b_0(x)$ varies faster at ![]() $|x|\lesssim \lambda$, in the region where it reverses direction, than at
$|x|\lesssim \lambda$, in the region where it reverses direction, than at ![]() $|x|\gg \lambda$, where it might be approximately flat (see Appendix D.1.2). An example of such a situation is the exactly solvable and ubiquitously useful Harris (Reference Harris1962) sheet
$|x|\gg \lambda$, where it might be approximately flat (see Appendix D.1.2). An example of such a situation is the exactly solvable and ubiquitously useful Harris (Reference Harris1962) sheet ![]() $b_0(x) = v_{\mathrm {A}y} \tanh (x/\lambda )$. This situation might be particularly relevant because in ideal MHD, field-reversing configurations of the kind that we need to support a tearing mode tend to be collapsing sheets, with
$b_0(x) = v_{\mathrm {A}y} \tanh (x/\lambda )$. This situation might be particularly relevant because in ideal MHD, field-reversing configurations of the kind that we need to support a tearing mode tend to be collapsing sheets, with ![]() $\lambda$ shrinking dynamically compared to the characteristic scales in the
$\lambda$ shrinking dynamically compared to the characteristic scales in the ![]() $y$ direction or indeed in the
$y$ direction or indeed in the ![]() $x$ direction away from the field-reversal region (see further discussion in Appendix D.5).
$x$ direction away from the field-reversal region (see further discussion in Appendix D.5).
D.1.2. Scaling of  $\varDelta '$
$\varDelta '$
A reader who is happy to accept (D10) can now skip to Appendix D.1.3. For those who would like to see a more detailed derivation leading to (D10), let me put forward the following argument, which is adapted from Loureiro et al. (Reference Loureiro, Schekochihin and Cowley2007); Loureiro, Schekochihin & Uzdensky (Reference Loureiro, Schekochihin and Uzdensky2013a).
Consider first ![]() $|x|\sim \lambda$. Since
$|x|\sim \lambda$. Since ![]() $b_0''/b_0 \sim 1/\lambda ^2 \gg k_y^2$, we may neglect the
$b_0''/b_0 \sim 1/\lambda ^2 \gg k_y^2$, we may neglect the ![]() $k_y^2$ term in (D8) and seek a solution in the form
$k_y^2$ term in (D8) and seek a solution in the form ![]() $\psi = b_0(x)\chi (x)$. This allows us to integrate the equation directly, with the result
$\psi = b_0(x)\chi (x)$. This allows us to integrate the equation directly, with the result
where ![]() $\pm$ refer to solutions at positive and negative
$\pm$ refer to solutions at positive and negative ![]() $x$, respectively,
$x$, respectively, ![]() $C^\pm _{1,2}$ are integration constants and
$C^\pm _{1,2}$ are integration constants and ![]() $x_0\sim \lambda$ is some integration limit, whose precise value does not matter (any difference that it makes can be absorbed into
$x_0\sim \lambda$ is some integration limit, whose precise value does not matter (any difference that it makes can be absorbed into ![]() $C_1^\pm$). Since
$C_1^\pm$). Since ![]() $b_0(x)$ is an odd function,
$b_0(x)$ is an odd function,
Taking ![]() $x\to 0$ in (D11), we can, therefore, fix the constant
$x\to 0$ in (D11), we can, therefore, fix the constant ![]() $C_2^\pm$ via
$C_2^\pm$ via
Now formally taking ![]() $x\to \pm \infty$ and assuming that
$x\to \pm \infty$ and assuming that ![]() $b_0(x)\to \pm v _{\mathrm {A}y,\infty }=\mathrm {const}$ in these limits, we find that the solution (D11) asymptotes to
$b_0(x)\to \pm v _{\mathrm {A}y,\infty }=\mathrm {const}$ in these limits, we find that the solution (D11) asymptotes to
Let us return to (D8) and consider ![]() $|x|\gg \lambda$. At such distances,
$|x|\gg \lambda$. At such distances, ![]() $b_0''/b_0\to 0$ by assumption, so we must now solve (D8) neglecting the
$b_0''/b_0\to 0$ by assumption, so we must now solve (D8) neglecting the ![]() $b_0''/b_0$ terms while retaining
$b_0''/b_0$ terms while retaining ![]() $k_y^2$, and then match the resulting ‘outer–outer’ solution to the large-
$k_y^2$, and then match the resulting ‘outer–outer’ solution to the large-![]() $|x|$ asymptotic (D14) of the ‘outer–inner’ solution (D11). The solution that vanishes at infinity is
$|x|$ asymptotic (D14) of the ‘outer–inner’ solution (D11). The solution that vanishes at infinity is
and its ![]() $k_y x\ll 1$ asymptotic is
$k_y x\ll 1$ asymptotic is
Demanding that this match (D14), we get
Finally, returning to (D11), which, with (D13) and (D17), has become
 \begin{equation} \psi = b_0(x)\frac{v_{\mathrm{A}y}\psi(0)}{\lambda}\left[{\pm} \frac{1}{k_yv_{\mathrm{A}y,\infty}^2} - \int_{{\pm} x_0}^x\frac{\mathrm{d} x'}{b_0^2(x')}\right], \end{equation}
\begin{equation} \psi = b_0(x)\frac{v_{\mathrm{A}y}\psi(0)}{\lambda}\left[{\pm} \frac{1}{k_yv_{\mathrm{A}y,\infty}^2} - \int_{{\pm} x_0}^x\frac{\mathrm{d} x'}{b_0^2(x')}\right], \end{equation}
and using (D12), we obtain, for ![]() $k_y\lambda \ll 1$,
$k_y\lambda \ll 1$,
Pending detailed insight into the functional form of the aligned fluctuations in MHD turbulence, I am going to treat this scaling of ![]() $\varDelta '$ with
$\varDelta '$ with ![]() $k_y$ and
$k_y$ and ![]() $\lambda$ as generic. A more general scaling
$\lambda$ as generic. A more general scaling
corresponds, for ![]() $n>1$, to
$n>1$, to ![]() $b_0(x)$ decaying to zero at large
$b_0(x)$ decaying to zero at large ![]() $x$ on the same scale as it reverses direction around
$x$ on the same scale as it reverses direction around ![]() $x=0$: e.g., one gets
$x=0$: e.g., one gets ![]() $n=2$ for
$n=2$ for ![]() $b_0(x)=v_{\mathrm {A}y}\tanh (x/\lambda )/\cosh ^2(x/\lambda )$ (Porcelli et al. Reference Porcelli, Borgogno, Califano, Grasso, Ottaviani and Pegoraro2002) or for a simple sinusoidal profile (Ottaviani & Porcelli Reference Ottaviani and Porcelli1993; Boldyrev & Loureiro Reference Boldyrev and Loureiro2018). There is some space for discussion as to whether
$b_0(x)=v_{\mathrm {A}y}\tanh (x/\lambda )/\cosh ^2(x/\lambda )$ (Porcelli et al. Reference Porcelli, Borgogno, Califano, Grasso, Ottaviani and Pegoraro2002) or for a simple sinusoidal profile (Ottaviani & Porcelli Reference Ottaviani and Porcelli1993; Boldyrev & Loureiro Reference Boldyrev and Loureiro2018). There is some space for discussion as to whether ![]() $n=1$ or
$n=1$ or ![]() $n=2$ is the best model for what happens in a typical MHD-turbulent structure (cf. Walker et al. Reference Walker, Boldyrev and Loureiro2018). Generalising all the scalings derived throughout this review to arbitrary
$n=2$ is the best model for what happens in a typical MHD-turbulent structure (cf. Walker et al. Reference Walker, Boldyrev and Loureiro2018). Generalising all the scalings derived throughout this review to arbitrary ![]() $n$ is a tedious but straightforward exercise (Pucci et al. Reference Pucci, Velli, Tenerani and Del Sarto2018; Singh et al. Reference Singh, Pucci, Tenerani, Shibata, Hillier and Velli2019; Boldyrev & Loureiro Reference Boldyrev and Loureiro2020), which I have opted to forgo, to avoid bulky
$n$ is a tedious but straightforward exercise (Pucci et al. Reference Pucci, Velli, Tenerani and Del Sarto2018; Singh et al. Reference Singh, Pucci, Tenerani, Shibata, Hillier and Velli2019; Boldyrev & Loureiro Reference Boldyrev and Loureiro2020), which I have opted to forgo, to avoid bulky ![]() $n$-dependent exponents everywhere. A meticulous reader who wishes to do this exercise will find the tearing-mode scalings for arbitrary
$n$-dependent exponents everywhere. A meticulous reader who wishes to do this exercise will find the tearing-mode scalings for arbitrary ![]() $n$ in Appendix D.1.5 and take it from there. For the tearing-mediated RMHD cascade (§ 7), the resulting turbulence scalings were worked out by Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017, Reference Boldyrev and Loureiro2020); for the tearing-mediated dynamo (§ 13.3), by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022).
$n$ in Appendix D.1.5 and take it from there. For the tearing-mediated RMHD cascade (§ 7), the resulting turbulence scalings were worked out by Boldyrev & Loureiro (Reference Boldyrev and Loureiro2017, Reference Boldyrev and Loureiro2020); for the tearing-mediated dynamo (§ 13.3), by Galishnikova et al. (Reference Galishnikova, Kunz and Schekochihin2022).
D.1.3. Inner solution
In the inner region, whose width is ![]() $\delta _{\mathrm {in}}$, we can approximate the equilibrium magnetic field's profile by (D12). Since
$\delta _{\mathrm {in}}$, we can approximate the equilibrium magnetic field's profile by (D12). Since ![]() $k_y\ll \partial _x\sim \delta _{\mathrm {in}}^{-1}$, the equations (D5) and (D6) for the tearing perturbation become
$k_y\ll \partial _x\sim \delta _{\mathrm {in}}^{-1}$, the equations (D5) and (D6) for the tearing perturbation become
The first of these is the balance of inertia, viscous and Lorentz forces, the second is Ohm's law in resistive MHD. Combining them, we get
This immediately tells us what the width of the boundary layer is:
 \begin{align} \frac{\nu}{\gamma\delta_{\mathrm{in}}^2}\ll1\quad&\Rightarrow\quad \left(\frac{\gamma\lambda}{k_yv_{\mathrm{A}y}}\right)^2\frac{\eta}{\gamma\delta_{\mathrm{in}}^4}\sim 1 \quad\Rightarrow\quad\frac{\delta_{\mathrm{in}}}{\lambda}\sim\left(\frac{\gamma\tau_{\mathrm{A}y}^2}{\tau_{\eta}}\right)^{1/4}\frac{1}{(k_y\lambda)^{1/2}}, \end{align}
\begin{align} \frac{\nu}{\gamma\delta_{\mathrm{in}}^2}\ll1\quad&\Rightarrow\quad \left(\frac{\gamma\lambda}{k_yv_{\mathrm{A}y}}\right)^2\frac{\eta}{\gamma\delta_{\mathrm{in}}^4}\sim 1 \quad\Rightarrow\quad\frac{\delta_{\mathrm{in}}}{\lambda}\sim\left(\frac{\gamma\tau_{\mathrm{A}y}^2}{\tau_{\eta}}\right)^{1/4}\frac{1}{(k_y\lambda)^{1/2}}, \end{align} \begin{align} \frac{\nu}{\gamma\delta_{\mathrm{in}}^2}\gg1 \quad&\Rightarrow\quad \left(\frac{\gamma\lambda}{k_yv_{\mathrm{A}y}}\right)^2\frac{\eta\nu}{\gamma^2\delta_{\mathrm{in}}^6}\sim 1 \quad\Rightarrow\quad \frac{\delta_{\mathrm{in}}}{\lambda}\sim\left(\frac{\tau_{\mathrm{A}y}^2}{\tau_{\eta}\tau_{\nu}}\right)^{1/6}\frac{1}{(k_y\lambda)^{1/3}}. \end{align}
\begin{align} \frac{\nu}{\gamma\delta_{\mathrm{in}}^2}\gg1 \quad&\Rightarrow\quad \left(\frac{\gamma\lambda}{k_yv_{\mathrm{A}y}}\right)^2\frac{\eta\nu}{\gamma^2\delta_{\mathrm{in}}^6}\sim 1 \quad\Rightarrow\quad \frac{\delta_{\mathrm{in}}}{\lambda}\sim\left(\frac{\tau_{\mathrm{A}y}^2}{\tau_{\eta}\tau_{\nu}}\right)^{1/6}\frac{1}{(k_y\lambda)^{1/3}}. \end{align}The latter regime, in which viscosity is large, is a slightly less popular version of the tearing mode, but it can be treated together with the classic limit (D24) at little extra cost.
Let us now rescale ![]() $x=X\delta _{\mathrm {in}}$ in (D23). Then
$x=X\delta _{\mathrm {in}}$ in (D23). Then ![]() $\psi _\mathrm {in}(X) \equiv \psi (X\delta _{\mathrm {in}})$ satisfies
$\psi _\mathrm {in}(X) \equiv \psi (X\delta _{\mathrm {in}})$ satisfies
In both cases, the inner solution depends on a single dimensionless parameter ![]() $\varLambda$ (the eigenvalue). In view of (D24–D25), this parameter is, in both cases, just the ratio of the growth rate of the mode to the rate of resistive diffusion across a layer of width
$\varLambda$ (the eigenvalue). In view of (D24–D25), this parameter is, in both cases, just the ratio of the growth rate of the mode to the rate of resistive diffusion across a layer of width ![]() $\delta _{\mathrm {in}}$, with the appropriate scaling of
$\delta _{\mathrm {in}}$, with the appropriate scaling of ![]() $\delta _{\mathrm {in}}$:
$\delta _{\mathrm {in}}$:
 \begin{equation} \varLambda \sim \frac{\gamma\delta_{\mathrm{in}}^2}{\eta} \sim \left\{\begin{array}{@{}ll} \displaystyle\dfrac{\gamma^{3/2}\tau_{\eta}^{1/2}\tau_{\mathrm{A}y}}{k_y\lambda} \sim\dfrac{(\gamma\tau_{\mathrm{A}y})^{3/2}}{k_y\lambda}\,S_\lambda^{1/2}, & \displaystyle\dfrac{\nu}{\gamma\delta_{\mathrm{in}}^2}\sim\dfrac{\mathrm{Pm}}{\varLambda}\ll1,\\\\ \displaystyle\dfrac{\gamma\tau_{\eta}^{2/3}\tau_{\nu}^{{-}1/3}\tau_{\mathrm{A}y}^{2/3}}{(k_y\lambda)^{2/3}} \sim \left[\dfrac{(\gamma\tau_{\mathrm{A}y})^{3/2}}{k_y\lambda}\,(S_\lambda\mathrm{Pm})^{1/2}\right]^{2/3}, & \displaystyle\dfrac{\nu}{\gamma\delta_{\mathrm{in}}^2}\sim\dfrac{\mathrm{Pm}}{\varLambda}\gg1, \end{array}\right. \end{equation}
\begin{equation} \varLambda \sim \frac{\gamma\delta_{\mathrm{in}}^2}{\eta} \sim \left\{\begin{array}{@{}ll} \displaystyle\dfrac{\gamma^{3/2}\tau_{\eta}^{1/2}\tau_{\mathrm{A}y}}{k_y\lambda} \sim\dfrac{(\gamma\tau_{\mathrm{A}y})^{3/2}}{k_y\lambda}\,S_\lambda^{1/2}, & \displaystyle\dfrac{\nu}{\gamma\delta_{\mathrm{in}}^2}\sim\dfrac{\mathrm{Pm}}{\varLambda}\ll1,\\\\ \displaystyle\dfrac{\gamma\tau_{\eta}^{2/3}\tau_{\nu}^{{-}1/3}\tau_{\mathrm{A}y}^{2/3}}{(k_y\lambda)^{2/3}} \sim \left[\dfrac{(\gamma\tau_{\mathrm{A}y})^{3/2}}{k_y\lambda}\,(S_\lambda\mathrm{Pm})^{1/2}\right]^{2/3}, & \displaystyle\dfrac{\nu}{\gamma\delta_{\mathrm{in}}^2}\sim\dfrac{\mathrm{Pm}}{\varLambda}\gg1, \end{array}\right. \end{equation}
where the Lundquist number (associated with scale ![]() $\lambda$) and the magnetic Prandtl number are defined as follows:
$\lambda$) and the magnetic Prandtl number are defined as follows:
D.1.4. Peak growth rate and wavenumber
Whatever the specific form of the solution of (D26) (Coppi et al. Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976; Boldyrev & Loureiro Reference Boldyrev and Loureiro2018) or (D27), ![]() $\varDelta '$ calculated from it according to (D9) (and non-dimensionalised) must be a function only of
$\varDelta '$ calculated from it according to (D9) (and non-dimensionalised) must be a function only of ![]() $\varLambda$:
$\varLambda$:
Equating this to the value (D10) calculated from the outer solution, we arrive at an equation for ![]() $\varLambda$:
$\varLambda$:
 \begin{equation} f(\varLambda) \sim \frac{\delta_{\mathrm{in}}}{k_y\lambda^2} \sim \left\{\begin{array}{@{}ll} \displaystyle\dfrac{\gamma^{1/4}\tau_{\mathrm{A}y}^{1/2}\tau_{\eta}^{{-}1/4}}{(k_y\lambda)^{3/2}} \sim \varLambda^{1/6}\left(k_y\lambda\, S_\lambda^{1/4}\right)^{{-}4/3}, & \mathrm{Pm}\ll\varLambda,\\\\ \displaystyle \dfrac{\tau_{\mathrm{A}y}^{1/3}(\tau_{\eta}\tau_{\nu})^{{-}1/6}}{(k_y\lambda)^{4/3}} \sim\left(k_y\lambda\, S_\lambda^{1/4}\mathrm{Pm}^{{-}1/8}\right)^{{-}4/3}, & \mathrm{Pm}\gg\varLambda. \end{array}\right. \end{equation}
\begin{equation} f(\varLambda) \sim \frac{\delta_{\mathrm{in}}}{k_y\lambda^2} \sim \left\{\begin{array}{@{}ll} \displaystyle\dfrac{\gamma^{1/4}\tau_{\mathrm{A}y}^{1/2}\tau_{\eta}^{{-}1/4}}{(k_y\lambda)^{3/2}} \sim \varLambda^{1/6}\left(k_y\lambda\, S_\lambda^{1/4}\right)^{{-}4/3}, & \mathrm{Pm}\ll\varLambda,\\\\ \displaystyle \dfrac{\tau_{\mathrm{A}y}^{1/3}(\tau_{\eta}\tau_{\nu})^{{-}1/6}}{(k_y\lambda)^{4/3}} \sim\left(k_y\lambda\, S_\lambda^{1/4}\mathrm{Pm}^{{-}1/8}\right)^{{-}4/3}, & \mathrm{Pm}\gg\varLambda. \end{array}\right. \end{equation}
Since the function ![]() $f(\varLambda )$ does not depend on any parameters apart from
$f(\varLambda )$ does not depend on any parameters apart from ![]() $\varLambda$, one might intuit that the maximum growth of the tearing mode should occur at
$\varLambda$, one might intuit that the maximum growth of the tearing mode should occur at ![]() $\varLambda \sim 1$, when
$\varLambda \sim 1$, when ![]() $f(\varLambda )\sim 1$ (I shall confirm this momentarily). Using these estimates in (D31) and (D28), we find
$f(\varLambda )\sim 1$ (I shall confirm this momentarily). Using these estimates in (D31) and (D28), we find
where ![]() $\mathrm {Pm}$ only matters if it is large. Note that if
$\mathrm {Pm}$ only matters if it is large. Note that if ![]() $S_\lambda \gg (1+\mathrm {Pm})^{1/2}$, the assumption
$S_\lambda \gg (1+\mathrm {Pm})^{1/2}$, the assumption ![]() $k_y\lambda \ll 1$ is confirmed. These are the maximum growth rate and the corresponding wavenumber of the tearing mode.Footnote 97 Note that, for this solution, since
$k_y\lambda \ll 1$ is confirmed. These are the maximum growth rate and the corresponding wavenumber of the tearing mode.Footnote 97 Note that, for this solution, since ![]() $f(\varLambda )\sim 1$, (D31) gives us
$f(\varLambda )\sim 1$, (D31) gives us
If setting ![]() $\varLambda \sim 1$,
$\varLambda \sim 1$, ![]() $f(\varLambda )\sim 1$ did not feel inevitable to the reader, perhaps the following considerations will help. Let us consider two physically meaningful limits that do not satisfy these assumptions.
$f(\varLambda )\sim 1$ did not feel inevitable to the reader, perhaps the following considerations will help. Let us consider two physically meaningful limits that do not satisfy these assumptions.
First, let us ask what happens if ![]() $\varLambda \ll 1$. This means that the mode grows slowly compared to the Ohmic diffusion rate in the current layer,
$\varLambda \ll 1$. This means that the mode grows slowly compared to the Ohmic diffusion rate in the current layer, ![]() $\gamma \ll \eta /\delta _{\mathrm {in}}^2$, a situation that corresponds, in a sense that is to be quantified in a moment, to small
$\gamma \ll \eta /\delta _{\mathrm {in}}^2$, a situation that corresponds, in a sense that is to be quantified in a moment, to small ![]() $\varDelta '$. In this limit,
$\varDelta '$. In this limit, ![]() $f(\varLambda )\sim \varLambda$ to lowest order in the Taylor expansion (I have not shown this rigorously, but hopefully it is plausible to the reader; for a derivation, see, e.g., Taylor & Newton Reference Taylor and Newton2015 or Boldyrev & Loureiro Reference Boldyrev and Loureiro2018). Putting this into (D31) and using (D28) to unpack
$f(\varLambda )\sim \varLambda$ to lowest order in the Taylor expansion (I have not shown this rigorously, but hopefully it is plausible to the reader; for a derivation, see, e.g., Taylor & Newton Reference Taylor and Newton2015 or Boldyrev & Loureiro Reference Boldyrev and Loureiro2018). Putting this into (D31) and using (D28) to unpack ![]() $\varLambda$, we find
$\varLambda$, we find
 \begin{equation} \gamma\tau_{\mathrm{A}y} \sim\left\{\begin{array}{@{}ll} \displaystyle S_\lambda^{{-}3/5}(k_y\lambda)^{{-}2/5}, & k_y\lambda \ll S_\lambda^{{-}1/4}\mathrm{Pm}^{{-}5/8},\\\\ \displaystyle S_\lambda^{{-}2/3}\mathrm{Pm}^{{-}1/6}(k_y\lambda)^{{-}2/3}, & k_y\lambda \gg S_\lambda^{{-}1/4}\mathrm{Pm}^{{-}5/8}. \end{array}\right. \end{equation}
\begin{equation} \gamma\tau_{\mathrm{A}y} \sim\left\{\begin{array}{@{}ll} \displaystyle S_\lambda^{{-}3/5}(k_y\lambda)^{{-}2/5}, & k_y\lambda \ll S_\lambda^{{-}1/4}\mathrm{Pm}^{{-}5/8},\\\\ \displaystyle S_\lambda^{{-}2/3}\mathrm{Pm}^{{-}1/6}(k_y\lambda)^{{-}2/3}, & k_y\lambda \gg S_\lambda^{{-}1/4}\mathrm{Pm}^{{-}5/8}. \end{array}\right. \end{equation}
This is the famous FKR solution (Furth et al. Reference Furth, Killeen and Rosenbluth1963; see also Porcelli Reference Porcelli1987 for the large-![]() $\mathrm {Pm}$ case). Since, to get it,
$\mathrm {Pm}$ case). Since, to get it, ![]() $\varLambda \ll 1$ was assumed, substituting (D34) into (D28) tells us that the approximation is valid at wavenumbers exceeding the wavenumber (D32) of peak growth,
$\varLambda \ll 1$ was assumed, substituting (D34) into (D28) tells us that the approximation is valid at wavenumbers exceeding the wavenumber (D32) of peak growth, ![]() $k_y\gg k_*$. Note that this imposes an upper bound on
$k_y\gg k_*$. Note that this imposes an upper bound on ![]() $\varDelta '$:
$\varDelta '$:
This is sometimes (perhaps misleadingly) called the ‘small-![]() $\varDelta '$’ (or weakly driven) limit.
$\varDelta '$’ (or weakly driven) limit.
Let us now consider the limit opposite to (D35), i.e., when ![]() $\varDelta '$ is very large and
$\varDelta '$ is very large and ![]() $k_y \ll k_*$. In (D31), this corresponds to
$k_y \ll k_*$. In (D31), this corresponds to ![]() $f(\varLambda )\to \infty$ and we argue that this limit must be reached for some value
$f(\varLambda )\to \infty$ and we argue that this limit must be reached for some value ![]() $\varLambda \sim 1$ (it is not physically reasonable to expect that
$\varLambda \sim 1$ (it is not physically reasonable to expect that ![]() $\varLambda \gg 1$, i.e., that the growth rate of the mode can be much larger than the Ohmic diffusion rate in the current layer; this reasoning is confirmed by the exact solution – see Coppi et al. Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976). This implies, with the aid of (D28),
$\varLambda \gg 1$, i.e., that the growth rate of the mode can be much larger than the Ohmic diffusion rate in the current layer; this reasoning is confirmed by the exact solution – see Coppi et al. Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976). This implies, with the aid of (D28),
This long-wavelength (‘infinite-![]() $\varDelta '$’, or strongly driven) limit of the tearing mode was first derived by Coppi et al. (Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976) (and by Porcelli Reference Porcelli1987 for the large-
$\varDelta '$’, or strongly driven) limit of the tearing mode was first derived by Coppi et al. (Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976) (and by Porcelli Reference Porcelli1987 for the large-![]() $\mathrm {Pm}$ case).
$\mathrm {Pm}$ case).
We see that the small-![]() $k_y$ asymptotic (D36) is an ascending and the large-
$k_y$ asymptotic (D36) is an ascending and the large-![]() $k_y$ one (D34) a descending function of
$k_y$ one (D34) a descending function of ![]() $k_y$ (figure 42). The wavenumber
$k_y$ (figure 42). The wavenumber ![]() $k_*$ of peak growth lies in between, where these two asymptotics meet, which is quite obviously the solution (D32).
$k_*$ of peak growth lies in between, where these two asymptotics meet, which is quite obviously the solution (D32).

Figure 42. Tearing growth rate ![]() $\gamma$ vs.
$\gamma$ vs. ![]() $k_y$: the Coppi et al. (Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976) solution (D36) for
$k_y$: the Coppi et al. (Reference Coppi, Galvão, Pellat, Rosenbluth and Rutherford1976) solution (D36) for ![]() $k_y\ll k_*$, where
$k_y\ll k_*$, where ![]() $k_*$ is given in (D32), and the FKR solution (D34) at
$k_*$ is given in (D32), and the FKR solution (D34) at ![]() $k_y\gg k_*$. The viscous version of the latter takes over at
$k_y\gg k_*$. The viscous version of the latter takes over at ![]() $k_y\gg k_\mathrm {visc}$, where
$k_y\gg k_\mathrm {visc}$, where ![]() $k_\mathrm {visc}\lambda \sim S_\lambda ^{-1/4}\mathrm {Pm}^{-5/8}$. This cartoon is for
$k_\mathrm {visc}\lambda \sim S_\lambda ^{-1/4}\mathrm {Pm}^{-5/8}$. This cartoon is for ![]() $\mathrm {Pm}\ll 1$; if
$\mathrm {Pm}\ll 1$; if ![]() $\mathrm {Pm}\gg 1$, the viscous-FKR scaling starts at
$\mathrm {Pm}\gg 1$, the viscous-FKR scaling starts at ![]() $k_*$.
$k_*$.
The applicability of this solution is subject to an important caveat. The Harris-like equilibrium that was used to obtain it is a 1D configuration, implicitly assumed to extend as far in the ![]() $y$ direction as the mode requires to develop. In reality, any sheet-like configuration forming as a result of (ideal) MHD dynamics will have a length, as well as width:
$y$ direction as the mode requires to develop. In reality, any sheet-like configuration forming as a result of (ideal) MHD dynamics will have a length, as well as width: ![]() $\xi \gg \lambda$, but still finite. The finiteness of
$\xi \gg \lambda$, but still finite. The finiteness of ![]() $\xi$ will limit the wavenumbers of the tearing perturbations that can be accommodated. The fastest-growing mode (D32) will only fit into the sheet if
$\xi$ will limit the wavenumbers of the tearing perturbations that can be accommodated. The fastest-growing mode (D32) will only fit into the sheet if
If this condition fails to be satisfied, i.e., if the aspect ratio of the sheet is too small, the fastest-growing mode will be the FKR mode (D34) with the smallest possible allowed wavenumber ![]() $k_y\xi \sim 1$. Thus, low-aspect-ratio sheets will develop tearing perturbations comprising just one or two islands, whereas the high-aspect-ratio ones will spawn whole chains of them, consisting of
$k_y\xi \sim 1$. Thus, low-aspect-ratio sheets will develop tearing perturbations comprising just one or two islands, whereas the high-aspect-ratio ones will spawn whole chains of them, consisting of ![]() $N \sim k_*\xi$ islands.
$N \sim k_*\xi$ islands.
D.1.5. Case of arbitrary scaling of  $\varDelta '$
$\varDelta '$
As promised at the end of Appendix D.1.2, here is the generalisation of the main tearing-mode scalings to the case of ![]() $\varDelta '$ scaling according to (D20). For
$\varDelta '$ scaling according to (D20). For ![]() $\varLambda \sim 1$, (D28), which is independent of
$\varLambda \sim 1$, (D28), which is independent of ![]() $n$, implies
$n$, implies
Using (D20) in (D30) and setting ![]() $f(\varLambda )\sim 1$ gets us, instead of (D33),
$f(\varLambda )\sim 1$ gets us, instead of (D33),
Combining this with (D38) leads to the generalised version of (D32):
Using these scalings instead of (D32) in (7.4) and (7.1) introduces ![]() $n$ dependence everywhere in §§ 7, 11.4, and 13.3, but does not appear to change anything qualitatively (Boldyrev & Loureiro Reference Boldyrev and Loureiro2017, Reference Boldyrev and Loureiro2020; Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
$n$ dependence everywhere in §§ 7, 11.4, and 13.3, but does not appear to change anything qualitatively (Boldyrev & Loureiro Reference Boldyrev and Loureiro2017, Reference Boldyrev and Loureiro2020; Galishnikova et al. Reference Galishnikova, Kunz and Schekochihin2022).
D.2. Onset of nonlinearity
The tearing mode normally enters a nonlinear regime when the width ![]() $w$ of its islands becomes comparable to
$w$ of its islands becomes comparable to ![]() $\delta _{\mathrm {in}}$. The islands then grow secularly (Rutherford Reference Rutherford1973) until
$\delta _{\mathrm {in}}$. The islands then grow secularly (Rutherford Reference Rutherford1973) until ![]() $w\varDelta '\sim 1$. As we saw in Appendix D.1.4, for the fastest-growing Coppi mode,
$w\varDelta '\sim 1$. As we saw in Appendix D.1.4, for the fastest-growing Coppi mode, ![]() $\varDelta '\sim \delta _{\mathrm {in}}^{-1}$, so the secular-growth stage is skipped. The width of the islands at the onset of the nonlinear regime is, therefore,
$\varDelta '\sim \delta _{\mathrm {in}}^{-1}$, so the secular-growth stage is skipped. The width of the islands at the onset of the nonlinear regime is, therefore,
There is little overhead here for keeping ![]() $n$ general, as in (D39), so I will.
$n$ general, as in (D39), so I will.
The amplitudes ![]() $\delta b_x$ and
$\delta b_x$ and ![]() $\delta b_y$ of the tearing perturbation at the onset of nonlinearity can be worked out by observing that the typical angular distortion of a field line due to the perturbation is
$\delta b_y$ of the tearing perturbation at the onset of nonlinearity can be worked out by observing that the typical angular distortion of a field line due to the perturbation is
and that, by solenoidality,
Since, from (D12), ![]() $b_0(x)|_{x\sim w}\sim (w/\lambda )v_{\mathrm {A}y}$, we have
$b_0(x)|_{x\sim w}\sim (w/\lambda )v_{\mathrm {A}y}$, we have
Note that (D42) and (D43) together imply that ![]() $\delta b_y\sim b_0(x)|_{x\sim w}$, i.e., the perturbed field is as large as the equilibrium field is locally at
$\delta b_y\sim b_0(x)|_{x\sim w}$, i.e., the perturbed field is as large as the equilibrium field is locally at ![]() $x\sim w$ (but not as the upstream field
$x\sim w$ (but not as the upstream field ![]() $v_{\mathrm {A}y}$ at
$v_{\mathrm {A}y}$ at ![]() $x\sim \lambda$).
$x\sim \lambda$).
Let us confirm that (D41) was a good estimate for the onset of nonlinearity, i.e., that, once it is achieved, the characteristic rate of the nonlinear evolution of the tearing perturbation becomes comparable to its linear growth rate (D40). The nonlinear evolution rate can be estimated as ![]() $k_* \delta u_y$, where
$k_* \delta u_y$, where ![]() $\delta u_y$ is the outflow velocity from the tearing region. When
$\delta u_y$ is the outflow velocity from the tearing region. When ![]() $\mathrm {Pm}\lesssim 1$, this is obviously Alfvénic,
$\mathrm {Pm}\lesssim 1$, this is obviously Alfvénic, ![]() $\delta u_y\sim \delta b_y$. When
$\delta u_y\sim \delta b_y$. When ![]() $\mathrm {Pm}\gg 1$, the situation is more subtle as the viscous relaxation of the flows is in fact faster than their Alfvénic evolution (as we are about to see). Then the outflow velocity must be determined from the force balance between viscous and magnetic stresses: using (D44),
$\mathrm {Pm}\gg 1$, the situation is more subtle as the viscous relaxation of the flows is in fact faster than their Alfvénic evolution (as we are about to see). Then the outflow velocity must be determined from the force balance between viscous and magnetic stresses: using (D44),
Combining the small- and large-![]() $\mathrm {Pm}$ cases, we get
$\mathrm {Pm}$ cases, we get
In the last expression, (D44) was used to estimate ![]() $\delta b_y$ and then (D40) to ascertain that the nonlinear and linear rates are indeed the same.
$\delta b_y$ and then (D40) to ascertain that the nonlinear and linear rates are indeed the same.
D.3. What happens next?
Once nonlinear effects come in, the tearing perturbation becomes subject to ideal-MHD evolution (for ![]() $\mathrm {Pm}\gg 1$, also to viscous forces). This leads to collapse of the
$\mathrm {Pm}\gg 1$, also to viscous forces). This leads to collapse of the ![]() $X$-points separating the islands of the tearing perturbation into current sheets (Waelbroeck Reference Waelbroeck1993; Jemella et al. Reference Jemella, Shay, Drake and Rogers2003; Jemella, Drake & Shay Reference Jemella, Drake and Shay2004). The time scale for this process is the same as that for the Coppi mode's growth (Loureiro et al. Reference Loureiro, Cowley, Dorland, Haines and Schekochihin2005) (which, as we have just seen, is the same as the ideal-MHD time scale for a perturbation that is gone nonlinear).
$X$-points separating the islands of the tearing perturbation into current sheets (Waelbroeck Reference Waelbroeck1993; Jemella et al. Reference Jemella, Shay, Drake and Rogers2003; Jemella, Drake & Shay Reference Jemella, Drake and Shay2004). The time scale for this process is the same as that for the Coppi mode's growth (Loureiro et al. Reference Loureiro, Cowley, Dorland, Haines and Schekochihin2005) (which, as we have just seen, is the same as the ideal-MHD time scale for a perturbation that is gone nonlinear).
In order to explain what happens as a result of this, I must cover some essential material regarding current sheets and reconnection in them, but let me preview the main ingredients of the overall story first.
(i) The standard, resistively limited, reconnecting current sheet towards which the ![]() $X$-point collapse leads is described in Appendix D.4.1.
$X$-point collapse leads is described in Appendix D.4.1.
(ii) At asymptotically large Lundquist numbers, such a sheet is, in fact, violently unstable (Appendix D.4.2).
(iii) Therefore, it cannot, in fact, exist and will be disrupted in mid-formation, spawning a population of multiscale islands, or plasmoids (Appendix D.5).
(iv) If it does not fall apart as a result – e.g., if it is held together by some dynamics external to it – it turns into a stochastic plasmoid chain, which is a site of fast MHD reconnection (Appendix D.6).
All of these things will be described, somewhat painstakingly, in what follows – they are the background to the blithe assumptions made at the end of § 7.1 about the nature of the debris left in the wake of the tearing disruption of the aligned turbulent structures – assumptions that I needed to construct the model of the tearing-mediated turbulence presented in § 7.2.
D.4. Sweet–Parker sheet
Let me flesh out what was meant by the ![]() $X$-point collapse in Appendix D.3. The idea is that, once the nonlinearity takes hold and Alfvénic (or visco-Alfvénic) outflows from the reconnection region develop, the reconnecting site will suck plasma in, carrying the magnetic field with it, thus leading to formation of an extended sheet, which is a singularity from the ideal-MHD viewpoint, resolved, of course, by resistivity and acting as a funnel both for magnetic flux and plasma (figure 43).Footnote 98 After the collapse has occurred and a sheet has been formed, the magnetic field just outside the resistive layer (the ‘upstream field’) is now the full equilibrium field, brought in by the incoming flow
$X$-point collapse in Appendix D.3. The idea is that, once the nonlinearity takes hold and Alfvénic (or visco-Alfvénic) outflows from the reconnection region develop, the reconnecting site will suck plasma in, carrying the magnetic field with it, thus leading to formation of an extended sheet, which is a singularity from the ideal-MHD viewpoint, resolved, of course, by resistivity and acting as a funnel both for magnetic flux and plasma (figure 43).Footnote 98 After the collapse has occurred and a sheet has been formed, the magnetic field just outside the resistive layer (the ‘upstream field’) is now the full equilibrium field, brought in by the incoming flow ![]() $u_x$ of plasma (in terms of the discussion in Appendix D.2, this means that now
$u_x$ of plasma (in terms of the discussion in Appendix D.2, this means that now ![]() $\delta b_y\sim v _{\mathrm {A}y}$).
$\delta b_y\sim v _{\mathrm {A}y}$).

Figure 43. (a) An ![]() $X$-point, shown during the nonlinear stage of tearing mode, (b) SP current sheet, formed later on, upon collapse of that
$X$-point, shown during the nonlinear stage of tearing mode, (b) SP current sheet, formed later on, upon collapse of that ![]() $X$-point (adapted from a 2D RMHD numerical simulation by Loureiro et al. Reference Loureiro, Cowley, Dorland, Haines and Schekochihin2005). The black lines are magnetic-field lines (constant-flux contours). The in-plane field reverses direction along the middle of the domain that is shown. In the notation of Appendix D.4.1, the length of the sheet is
$X$-point (adapted from a 2D RMHD numerical simulation by Loureiro et al. Reference Loureiro, Cowley, Dorland, Haines and Schekochihin2005). The black lines are magnetic-field lines (constant-flux contours). The in-plane field reverses direction along the middle of the domain that is shown. In the notation of Appendix D.4.1, the length of the sheet is ![]() $\ell$ and its width is
$\ell$ and its width is ![]() $\delta$.
$\delta$.
D.4.1. Sweet–Parker reconnection
The flux brought in by the inflow ![]() $u_x$ must be destroyed by resistivity (reconnected and turned into
$u_x$ must be destroyed by resistivity (reconnected and turned into ![]() $b_x$). This translates into what formally is just a statement of balance between the advective and resistive terms in the induction equation:
$b_x$). This translates into what formally is just a statement of balance between the advective and resistive terms in the induction equation:
where ![]() $\delta$ is the resistive layer's width and
$\delta$ is the resistive layer's width and ![]() $u_x$ the inflow velocity. I have, in line with the prevailing convention (and physics) of the reconnection theory, introduced a Lundquist number based on the sheet length
$u_x$ the inflow velocity. I have, in line with the prevailing convention (and physics) of the reconnection theory, introduced a Lundquist number based on the sheet length ![]() $\ell$ (in the context of a sheet formed between two islands of a tearing perturbation, this length is
$\ell$ (in the context of a sheet formed between two islands of a tearing perturbation, this length is ![]() $\ell \sim k_*^{-1}$).
$\ell \sim k_*^{-1}$).
Since the sheet has to process matter as well as flux and since matter must be conserved, we may balance its inflow (![]() $u_x$) and outflow (
$u_x$) and outflow (![]() $u_y$):
$u_y$):
 \begin{equation} u_x \ell \sim u_y \delta \quad\Rightarrow\quad u_x \sim \frac{\delta}{\ell}u_y\quad\Rightarrow\quad \delta \sim \frac{\ell}{\sqrt{S_\ell}}\left(\frac{v_{\mathrm{A}y}}{u_y}\right)^{1/2}, \end{equation}
\begin{equation} u_x \ell \sim u_y \delta \quad\Rightarrow\quad u_x \sim \frac{\delta}{\ell}u_y\quad\Rightarrow\quad \delta \sim \frac{\ell}{\sqrt{S_\ell}}\left(\frac{v_{\mathrm{A}y}}{u_y}\right)^{1/2}, \end{equation}where the third equation is the result of combining the second with (D47).
Finally, the outflow velocity is inevitably Alfvénic in the absence of viscosity: this follows by balancing Reynolds and Maxwell stresses (inertia and tension) in the momentum equation (in either ![]() $y$ or
$y$ or ![]() $x$ direction; note that
$x$ direction; note that ![]() $b_x\sim v_{\mathrm {A}y}\delta /\ell$). Physically, this is just saying that the tension in the ‘parabolic’-shaped freshly reconnected magnetic field line (manifest in figure 43a) will accelerate plasma and propel it out of the sheet. In the presence of viscosity, i.e., when
$b_x\sim v_{\mathrm {A}y}\delta /\ell$). Physically, this is just saying that the tension in the ‘parabolic’-shaped freshly reconnected magnetic field line (manifest in figure 43a) will accelerate plasma and propel it out of the sheet. In the presence of viscosity, i.e., when ![]() $\mathrm {Pm}\gg 1$, we must instead balance the magnetic stress with the viscous one, exactly like I did in (D45), but with a narrower channel and a greater upstream field:
$\mathrm {Pm}\gg 1$, we must instead balance the magnetic stress with the viscous one, exactly like I did in (D45), but with a narrower channel and a greater upstream field:
To get the last expression, ![]() $\delta$ had to be substituted from (D48). Just as I have done everywhere else, I will combine the low- and high-
$\delta$ had to be substituted from (D48). Just as I have done everywhere else, I will combine the low- and high-![]() $\mathrm {Pm}$ cases:Footnote 99
$\mathrm {Pm}$ cases:Footnote 99
 \begin{equation} u_y \sim \frac{v_{\mathrm{A}y}}{\sqrt{1+\mathrm{Pm}}} \quad\Rightarrow\quad \frac{\delta}{\ell} \sim \frac{(1+\mathrm{Pm})^{1/4}}{\sqrt{S_\ell}} \equiv\frac{1}{\sqrt{\tilde S_\ell}}, \quad\tilde S_\ell = \frac{u_y \ell}{\eta}, \end{equation}
\begin{equation} u_y \sim \frac{v_{\mathrm{A}y}}{\sqrt{1+\mathrm{Pm}}} \quad\Rightarrow\quad \frac{\delta}{\ell} \sim \frac{(1+\mathrm{Pm})^{1/4}}{\sqrt{S_\ell}} \equiv\frac{1}{\sqrt{\tilde S_\ell}}, \quad\tilde S_\ell = \frac{u_y \ell}{\eta}, \end{equation}
where ![]() $\tilde S_\ell$, the Lundquist number based on the outflow velocity, is an obviously useful shorthand. Other relevant quantities can now be calculated, e.g., the rate at which flux is reconnected:
$\tilde S_\ell$, the Lundquist number based on the outflow velocity, is an obviously useful shorthand. Other relevant quantities can now be calculated, e.g., the rate at which flux is reconnected:
 \begin{equation} \frac{\partial\varPsi}{\partial t}\sim u_xv_{\mathrm{A}y} \sim \frac{u_yv_{\mathrm{A}y}}{\sqrt{\tilde S_\ell}} \sim \frac{v_{\mathrm{A}y}^2}{(1+\mathrm{Pm})^{1/4}\sqrt{S_\ell}}. \end{equation}
\begin{equation} \frac{\partial\varPsi}{\partial t}\sim u_xv_{\mathrm{A}y} \sim \frac{u_yv_{\mathrm{A}y}}{\sqrt{\tilde S_\ell}} \sim \frac{v_{\mathrm{A}y}^2}{(1+\mathrm{Pm})^{1/4}\sqrt{S_\ell}}. \end{equation} The argument that I have just presented is one of the enduring classics of the genre and is due to Sweet (Reference Sweet1958) and Parker (Reference Parker1957) (hereafter SP; the large-![]() $\mathrm {Pm}$ extension was done by Park, Monticello & White Reference Park, Monticello and White1984). While the argument is qualitative, it does work, in the sense both that one can construct unique solutions of the SP kind, in a manner pleasing to rigorous theoreticians (Uzdensky, Kulsrud & Yamada Reference Uzdensky, Kulsrud and Yamada1996; Uzdensky & Kulsrud Reference Uzdensky and Kulsrud2000), and that SP reconnection has been measured and confirmed experimentally (Ji et al. Reference Ji, Yamada, Hsu and Kulsrud1998, Reference Ji, Yamada, Hsu, Kulsrud, Carter and Zaharia1999) (figure 45 shows an SP sheet measured in their MRX experiment at Princeton).
$\mathrm {Pm}$ extension was done by Park, Monticello & White Reference Park, Monticello and White1984). While the argument is qualitative, it does work, in the sense both that one can construct unique solutions of the SP kind, in a manner pleasing to rigorous theoreticians (Uzdensky, Kulsrud & Yamada Reference Uzdensky, Kulsrud and Yamada1996; Uzdensky & Kulsrud Reference Uzdensky and Kulsrud2000), and that SP reconnection has been measured and confirmed experimentally (Ji et al. Reference Ji, Yamada, Hsu and Kulsrud1998, Reference Ji, Yamada, Hsu, Kulsrud, Carter and Zaharia1999) (figure 45 shows an SP sheet measured in their MRX experiment at Princeton).
D.4.2. Plasmoid instability
However, like for any sheet, one can work out a tearing instability for an SP sheet (this is not the same tearing instability as might have given rise to the sheet as suggested at the beginning of Appendix D.4 – the SP sheet is now the underlying equilibrium). The results of Appendix D.1.4 can be ported directly to this situation (with some caveats that I will discuss in the next paragraph), by identifying ![]() $\delta =\lambda$ and
$\delta =\lambda$ and ![]() $\ell = \xi$. This gives instantly
$\ell = \xi$. This gives instantly
This is the so-called plasmoid instability (Tajima & Shibata Reference Tajima and Shibata1997; Loureiro et al. Reference Loureiro, Schekochihin and Cowley2007, Reference Loureiro, Schekochihin and Uzdensky2013a; Bhattacharjee et al. Reference Bhattacharjee, Huang, Yang and Rogers2009; Comisso & Grasso Reference Comisso and Grasso2016; see figure 44). The realisation that SP sheets must be unstable can be traced back to Bulanov et al. (Reference Bulanov, Syrovatskiǐ and Sakai1978, Reference Bulanov, Sakai and Syrovatskii1979), with the first numerical demonstration achieved by Biskamp (Reference Biskamp1986) (see also Biskamp Reference Biskamp1982, Steinolfson & van Hoven Reference Steinolfson and van Hoven1984, Matthaeus & Lamkin Reference Matthaeus and Lamkin1985, Dahlburg, Zang & Montgomery Reference Dahlburg, Zang and Montgomery1986, Lee & Fu Reference Lee and Fu1986, and Malara, Veltri & Carbone Reference Malara, Veltri and Carbone1992). However, this knowledge did not seem to have impacted the field as much as it should have doneFootnote 100 until the appearance of the analytical paper by Loureiro et al. (Reference Loureiro, Schekochihin and Cowley2007) and the rise of the plasmoid-chain simulation industry in 2D (Lapenta Reference Lapenta2008; Daughton et al. Reference Daughton, Roytershteyn, Albright, Karimabadi, Yin and Bowers2009b,Reference Daughton, Roytershteyn, Albright, Karimabadi, Yin and Bowersc; Cassak, Shay & Drake Reference Cassak, Shay and Drake2009; Samtaney et al. Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009; Huang & Bhattacharjee Reference Huang and Bhattacharjee2010, Reference Huang and Bhattacharjee2012, Reference Huang and Bhattacharjee2013; Huang, Comisso & Bhattacharjee Reference Huang, Comisso and Bhattacharjee2017; Bárta et al. Reference Bárta, Büchner, Karlický and Skála2011; Loureiro et al. Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012; Shen et al. Reference Shen, Lin, Murphy and Raymond2013; Tenerani et al. Reference Tenerani, Velli, Rappazzo and Pucci2015b; Tenerani & Velli Reference Tenerani and Velli2020b), followed, more recently, by its more turbulent counterpart in 3D (Oishi et al. Reference Oishi, Mac Low, Collins and Tamura2015; Huang & Bhattacharjee Reference Huang and Bhattacharjee2016; Beresnyak Reference Beresnyak2017; Kowal et al. Reference Kowal, Falceta-Gonçalves, Lazarian and Vishniac2017; Stanier et al. Reference Stanier, Daughton, Le, Li and Bird2019; Yang et al. Reference Yang, Li, Guo, Li, Li, He, Zhang and Feng2020; Daldorff, Leake & Klimchuk Reference Daldorff, Leake and Klimchuk2022)Footnote 101 and even some experimental undertakings (Jara-Almonte et al. Reference Jara-Almonte, Ji, Yamada, Yoo and Fox2016; Hare et al. Reference Hare, Suttle, Lebedev, Loureiro, Ciardi, Burdiak, Chittenden, Clayson, Garcia and Niasse2017b,Reference Hare, Lebedev, Suttle, Loureiro, Ciardi, Burdiak, Chittenden, Clayson, Eardley and Garciab, Reference Hare, Suttle, Lebedev, Loureiro, Ciardi, Chittenden, Clayson, Eardley, Garcia and Halliday2018; Peterson et al. Reference Peterson, Endrizzi, Beidler, Bunkers, Clark, Egedal, Flanagan, McCollam, Milhone and Olson2019). Perhaps this was because plasmoids had to wait for their moment in the sun until they could be properly resolved numerically – and that required fairly large simulations. Indeed, for an SP sheet to start spawning plasmoids, a sizeable Lundquist number is needed: asking for ![]() $\delta _{\mathrm {in}}/\delta$ to be a reasonably small number, say, at least
$\delta _{\mathrm {in}}/\delta$ to be a reasonably small number, say, at least ![]() $1/3$, (D52) gives us
$1/3$, (D52) gives us
the critical Lundquist number for the plasmoid instability (Biskamp Reference Biskamp1986; Loureiro et al. Reference Loureiro, Cowley, Dorland, Haines and Schekochihin2005, Reference Loureiro, Schekochihin and Cowley2007; Samtaney et al. Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009; Ni et al. Reference Ni, Germaschewski, Huang, Sullivan, Yang and Bhattacharjee2010; Shi, Velli & Tenerani Reference Shi, Velli and Tenerani2018).

Figure 44. Plasmoid instability in current sheets with, from top to bottom, ![]() $S_\xi =10^4, 10^5, 10^6, 10^7, 10^8$. The domain shown is
$S_\xi =10^4, 10^5, 10^6, 10^7, 10^8$. The domain shown is ![]() $0.12$ of the full length of the sheet. This plot is adapted from Samtaney et al. (Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009), who confirmed the scalings (D52) numerically. (Reprinted with permission from Samtaney et al. Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009, copyright (2009) by the American Physical Society.)
$0.12$ of the full length of the sheet. This plot is adapted from Samtaney et al. (Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009), who confirmed the scalings (D52) numerically. (Reprinted with permission from Samtaney et al. Reference Samtaney, Loureiro, Uzdensky, Schekochihin and Cowley2009, copyright (2009) by the American Physical Society.)
Let me pause briefly to discuss how (in)valid the direct application of Appendix D.1 to an SP sheet in fact was. The good news is that, for such an equilibrium configuration, it is certainly true that ![]() $n=1$ in (D20), because the scale of the equilibrium field's reversal within the sheet is much smaller than that of its variation outside it, and so the derivation in Appendix D.1.2 applies – indeed it was originally invented by Loureiro et al. (Reference Loureiro, Schekochihin and Cowley2007, Reference Loureiro, Schekochihin and Uzdensky2013a) for this exact problem. The bad news is that an SP sheet is, in fact, not a static equilibrium considered in Appendix D.1, but a dynamic one, featuring an Alfvénic (or, at
$n=1$ in (D20), because the scale of the equilibrium field's reversal within the sheet is much smaller than that of its variation outside it, and so the derivation in Appendix D.1.2 applies – indeed it was originally invented by Loureiro et al. (Reference Loureiro, Schekochihin and Cowley2007, Reference Loureiro, Schekochihin and Uzdensky2013a) for this exact problem. The bad news is that an SP sheet is, in fact, not a static equilibrium considered in Appendix D.1, but a dynamic one, featuring an Alfvénic (or, at ![]() $\mathrm {Pm}\gg 1$, sub-Alfvénic) outflow
$\mathrm {Pm}\gg 1$, sub-Alfvénic) outflow ![]() $u_y$ (see (D50)), which is strongly sheared transversely to the sheet and has a positive outward gradient along it. Thus, technically, one must re-derive the tearing mode for this new equilibrium before jumping to conclusions. Doing so leads to scalings different from (D34) in the FKR regime, but does not affect the fastest-growing mode (D32) (a nice semi-qualitative derivation of this result is offered by Boldyrev & Loureiro Reference Boldyrev and Loureiro2018; the previous, quite sophisticated, if not in all cases penetrable, paper trail on tearing with flows is Bulanov et al. Reference Bulanov, Syrovatskiǐ and Sakai1978, Reference Bulanov, Sakai and Syrovatskii1979, Paris & Sy Reference Paris and Sy1983, Hofman Reference Hofman1975, Dobrowolny, Veltri & Mangeney Reference Dobrowolny, Veltri and Mangeney1983, Einaudi & Rubini Reference Einaudi and Rubini1986, Reference Einaudi and Rubini1989, Chen & Morrison Reference Chen and Morrison1990, Loureiro et al. Reference Loureiro, Schekochihin and Uzdensky2013a, Shi et al. Reference Shi, Velli and Tenerani2018, Tolman et al. Reference Tolman, Loureiro and Uzdensky2018). This gives me licence not to worry about this complication here.
$u_y$ (see (D50)), which is strongly sheared transversely to the sheet and has a positive outward gradient along it. Thus, technically, one must re-derive the tearing mode for this new equilibrium before jumping to conclusions. Doing so leads to scalings different from (D34) in the FKR regime, but does not affect the fastest-growing mode (D32) (a nice semi-qualitative derivation of this result is offered by Boldyrev & Loureiro Reference Boldyrev and Loureiro2018; the previous, quite sophisticated, if not in all cases penetrable, paper trail on tearing with flows is Bulanov et al. Reference Bulanov, Syrovatskiǐ and Sakai1978, Reference Bulanov, Sakai and Syrovatskii1979, Paris & Sy Reference Paris and Sy1983, Hofman Reference Hofman1975, Dobrowolny, Veltri & Mangeney Reference Dobrowolny, Veltri and Mangeney1983, Einaudi & Rubini Reference Einaudi and Rubini1986, Reference Einaudi and Rubini1989, Chen & Morrison Reference Chen and Morrison1990, Loureiro et al. Reference Loureiro, Schekochihin and Uzdensky2013a, Shi et al. Reference Shi, Velli and Tenerani2018, Tolman et al. Reference Tolman, Loureiro and Uzdensky2018). This gives me licence not to worry about this complication here.
Admittedly, in an extended SP sheet, the presence of flows can also lead to a very fast Kelvin–Helmholtz instability that appears at the periphery of the sheet and is actually even faster than the plasmoid instability (Loureiro et al. Reference Loureiro, Schekochihin and Uzdensky2013a). This, however, does not change the most important conclusion from (D52), and arguably the only relevant one, which is that the instability of an SP sheet is massively supercritical: at large enough ![]() $\tilde S_\xi$, it is nowhere near marginal stability. The question therefore really is whether we should expect SP sheets ever to be formed in natural circumstances. This brings us to our next topic.
$\tilde S_\xi$, it is nowhere near marginal stability. The question therefore really is whether we should expect SP sheets ever to be formed in natural circumstances. This brings us to our next topic.
D.5. Formation and disruption of sheets
Let us put SP sheets aside and talk more generally about MHD sheets of the kind envisioned in Appendix D.1 as the background equilibrium for tearing. The naturally occurring tearing-unstable ideal-MHD solutions are, of course, not static equilibria: they arise, basically, because of the dynamical tendency in MHD for ![]() $X$-points to collapse into sheets (which I invoked in Appendices D.3), illustrated in figure 45. An elementary example is the classic Chapman & Kendall (Reference Chapman and Kendall1963) collapsing solution of MHD equations:
$X$-points to collapse into sheets (which I invoked in Appendices D.3), illustrated in figure 45. An elementary example is the classic Chapman & Kendall (Reference Chapman and Kendall1963) collapsing solution of MHD equations:
Here ![]() $\varGamma (t)$ can be specified arbitrarily and then
$\varGamma (t)$ can be specified arbitrarily and then ![]() $\lambda (t)$ and
$\lambda (t)$ and ![]() $\xi (t)$ follow upon direct substitution of (D54) into the RMHD equations (D2–D3) (with
$\xi (t)$ follow upon direct substitution of (D54) into the RMHD equations (D2–D3) (with ![]() $\eta =0$). The original Chapman & Kendall (Reference Chapman and Kendall1963) version of this was the exponential collapse:
$\eta =0$). The original Chapman & Kendall (Reference Chapman and Kendall1963) version of this was the exponential collapse:
A later, perhaps more physically relevant example, due to Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016), is obtained by fixing the outflow velocity at the end of the sheet to be a constant parameter: if ![]() $u_y = \partial \varPhi _0/\partial x = u_0 y/\xi$, then
$u_y = \partial \varPhi _0/\partial x = u_0 y/\xi$, then
In this, or any other conceivable model of sheet formation, the aspect ratio increases with time as the sheet's width ![]() $\lambda$ decreases and its length
$\lambda$ decreases and its length ![]() $\xi$ increases.
$\xi$ increases.

Figure 45. Formation of a sheet from an ![]() $X$-point in the MRX experiment at Princeton (reprinted from Yamada et al. Reference Yamada, Ji, Hsu, Carter, Kulsrud, Bretz, Jobes, Ono and Perkins1997 with the permission of AIP Publishing).
$X$-point in the MRX experiment at Princeton (reprinted from Yamada et al. Reference Yamada, Ji, Hsu, Carter, Kulsrud, Bretz, Jobes, Ono and Perkins1997 with the permission of AIP Publishing).
The traditional thinking about sheets in MHD held that an ideal collapsing solution such as (D54) (or an explosively collapsing one obtained by Syrovatskiǐ Reference Syrovatskiǐ1971 for compressible MHD) would culminate in a steady-state current sheet, which, from the ideal-MHD point of view, would be a singularity, resolved in resistive MHD by Ohmic diffusion, leading to an SP sheet. One could then discuss magnetic reconnection in such a sheet (Appendix D.4.1). However, as we saw in Appendix D.4.2, this object is massively unstable to tearing perturbations and will break up into a multitude of islands (‘plasmoids’). Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) and Pucci & Velli (Reference Pucci and Velli2014) argued that, in fact, it would never form, because tearing perturbations growing against the background of a collapsing ideal-MHD solution will disrupt it before it reaches its steady-state, resistive SP limit.
The detailed demonstration of this result involves realising that not only does the instantaneous aspect ratio of a forming sheet decide what types of tearing perturbations are allowed (single-island FKR modes or multi-island fastest-growing, ‘Coppi’ modes; see Appendix D.1.4), but that, in principle, this can change as the sheet evolves, that many different modes can coexist and that these perturbations will grow on different time scales not only linearly but also nonlinearly (the FKR modes having to go through the secular Rutherford Reference Rutherford1973 regime, the Coppi ones not). A careful analysis of all this can be found in the paper by Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) (the follow-up by Tolman et al. Reference Tolman, Loureiro and Uzdensky2018 deals with the effect of the sheet-forming flows on the tearing mode). The summary that will suffice for my purposes here is that if the fastest-growing linear mode (D32) fits into the sheet, it will also be the one that first reaches the nonlinear regime and disrupts the formation of the sheet. Note that at the onset of the nonlinear regime of the tearing mode, the width of the islands is given by (D41). Since ![]() $w\ll \lambda$, islands of this size are, in fact, short of what is needed to disrupt the sheet. Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) argue that the collapse of the inter-island
$w\ll \lambda$, islands of this size are, in fact, short of what is needed to disrupt the sheet. Uzdensky & Loureiro (Reference Uzdensky and Loureiro2016) argue that the collapse of the inter-island ![]() $X$-points, already mooted in Appendix D.3, will eventually – on the same, or faster, time scale as that of the growth of the mode – produce islands of size
$X$-points, already mooted in Appendix D.3, will eventually – on the same, or faster, time scale as that of the growth of the mode – produce islands of size ![]() $w\sim \lambda$, which is what they have to get to in order to be properly disruptive. This is a key ingredient for the picture of ‘tearing-mediated turbulence’ advocated in § 7.2 (but see caveats in § 7.4.1).
$w\sim \lambda$, which is what they have to get to in order to be properly disruptive. This is a key ingredient for the picture of ‘tearing-mediated turbulence’ advocated in § 7.2 (but see caveats in § 7.4.1).
D.5.1. ‘Ideal tearing’
So what kind of sheets can form before disruption occurs? Namely, what aspect ratio can a sheet reach before the growth rate of the tearing mode triggered in the sheet becomes larger than the rate at which the sheet is collapsing via its ideal-MHD evolution? The former rate is given by (D32) and the latter is ![]() $\varGamma \sim v _{\mathrm {A}y}/\xi$, as is illustrated by the Uzdensky–Loureiro solution (D56).Footnote 102 Then
$\varGamma \sim v _{\mathrm {A}y}/\xi$, as is illustrated by the Uzdensky–Loureiro solution (D56).Footnote 102 Then
The last expression contains the Lundquist number referred to the length ![]() $\xi$ rather than to the width
$\xi$ rather than to the width ![]() $\lambda$ of the sheet, as customary in magnetic-reconnection theory (cf. Appendix D.4). Note that the assumption that it is the fastest-growing Coppi mode (D32) that should be used in this estimate is confirmed a posteriori by checking that the mode does fit into the sheet (cf. (D37)): for
$\lambda$ of the sheet, as customary in magnetic-reconnection theory (cf. Appendix D.4). Note that the assumption that it is the fastest-growing Coppi mode (D32) that should be used in this estimate is confirmed a posteriori by checking that the mode does fit into the sheet (cf. (D37)): for ![]() $\xi$ satisfying the equality in (D57),
$\xi$ satisfying the equality in (D57),
The scaling (D57) of the aspect ratio of the sheet with ![]() $S_\xi$ was proposed by Pucci & Velli (Reference Pucci and Velli2014) to be the maximum possible attainable one before the sheet is destroyed by what they termed ‘ideal tearing’, i.e., by tearing modes that grow on the same time scale as the ideal-MHD sheet evolves (this result was checked numerically by Landi et al. Reference Landi, Del Zanna, Papini, Pucci and Velli2015 and Del Zanna et al. Reference Del Zanna, Landi, Papini, Pucci and Velli2016, extended to
$S_\xi$ was proposed by Pucci & Velli (Reference Pucci and Velli2014) to be the maximum possible attainable one before the sheet is destroyed by what they termed ‘ideal tearing’, i.e., by tearing modes that grow on the same time scale as the ideal-MHD sheet evolves (this result was checked numerically by Landi et al. Reference Landi, Del Zanna, Papini, Pucci and Velli2015 and Del Zanna et al. Reference Del Zanna, Landi, Papini, Pucci and Velli2016, extended to ![]() $\mathrm {Pm}\gg 1$ by Tenerani et al. Reference Tenerani, Rappazzo, Velli and Pucci2015a, and generalised by Pucci et al. Reference Pucci, Velli, Tenerani and Del Sarto2018 to the case of arbitrary scaling of
$\mathrm {Pm}\gg 1$ by Tenerani et al. Reference Tenerani, Rappazzo, Velli and Pucci2015a, and generalised by Pucci et al. Reference Pucci, Velli, Tenerani and Del Sarto2018 to the case of arbitrary scaling of ![]() $\varDelta '$ with
$\varDelta '$ with ![]() $k_y$ that I explained in Appendix D.1.5). The conclusion that the sheet is indeed destroyed depends on the
$k_y$ that I explained in Appendix D.1.5). The conclusion that the sheet is indeed destroyed depends on the ![]() $X$-point-collapse argument described above, because the tearing modes by themselves do not produce islands as wide as the sheet (see Appendix D.2).
$X$-point-collapse argument described above, because the tearing modes by themselves do not produce islands as wide as the sheet (see Appendix D.2).
The argument in § 7.1 about the disruption of MHD turbulence by tearing is essentially the application of the criterion (D57) to the aligned structures of which Boldyrev's MHD turbulent cascade consists.
Since the aspect ratio of the sheet described by (D57) is smaller than that of the SP sheet (D50) (![]() $S_\xi ^{1/3}$, rather than
$S_\xi ^{1/3}$, rather than ![]() $S_\xi ^{1/2}$), Pucci & Velli (Reference Pucci and Velli2014) argued that global SP sheets could never form. An extensive numerical study by Huang et al. (Reference Huang, Comisso and Bhattacharjee2017) of the instability of forming current sheets has indeed confirmed explicitly that the plasmoid-instability scalings (D52) derived for an SP sheet only survive up to a certain critical value
$S_\xi ^{1/2}$), Pucci & Velli (Reference Pucci and Velli2014) argued that global SP sheets could never form. An extensive numerical study by Huang et al. (Reference Huang, Comisso and Bhattacharjee2017) of the instability of forming current sheets has indeed confirmed explicitly that the plasmoid-instability scalings (D52) derived for an SP sheet only survive up to a certain critical value
(which obviously had to be bigger than the critical Lundquist number (D53) for the plasmoid instability itself), with the ‘ideal-tearing’ scalings (D57) and (D58) taking over at ![]() $S_\xi \gtrsim S_{\xi,\mathrm {c}}^\mathrm {(ideal)}$.Footnote 103 Figure 46, taken from their paper, is an excellent illustration of the evolution of tearing perturbations and plasmoid chains.
$S_\xi \gtrsim S_{\xi,\mathrm {c}}^\mathrm {(ideal)}$.Footnote 103 Figure 46, taken from their paper, is an excellent illustration of the evolution of tearing perturbations and plasmoid chains.

Figure 46. This is a plot from Huang et al. (Reference Huang, Comisso and Bhattacharjee2017) (© AAS, reproduced with permission) illustrating the evolution of tearing perturbations of an evolving sheet in a 2D MHD simulation with ![]() $S_\xi \sim 10^6$ and
$S_\xi \sim 10^6$ and ![]() $\mathrm {Pm}\ll 1$. Their
$\mathrm {Pm}\ll 1$. Their ![]() $(x,y,z)$ are my
$(x,y,z)$ are my ![]() $(y,z,x)$, their
$(y,z,x)$, their ![]() $L$ is my
$L$ is my ![]() $\xi$ (sheet length), their
$\xi$ (sheet length), their ![]() $a$ is my
$a$ is my ![]() $\lambda$ (sheet width), their
$\lambda$ (sheet width), their ![]() $\tau _\mathrm {A}$ is my
$\tau _\mathrm {A}$ is my ![]() $\varGamma ^{-1}\sim \xi /v_{\mathrm {A}}$ (characteristic time of the sheet evolution), their
$\varGamma ^{-1}\sim \xi /v_{\mathrm {A}}$ (characteristic time of the sheet evolution), their ![]() $\delta$ is my
$\delta$ is my ![]() $\delta _{\mathrm {in}}$ (width of the tearing inner layer). The colour in the upper halves of their panels shows out-of-page current (colour bar ‘
$\delta _{\mathrm {in}}$ (width of the tearing inner layer). The colour in the upper halves of their panels shows out-of-page current (colour bar ‘![]() $J_y$’) and in the lower halves the outflow velocity along the sheet (colour bar ‘
$J_y$’) and in the lower halves the outflow velocity along the sheet (colour bar ‘![]() $v_x$’). The solid magenta lines are separatrices demarcating two ‘global’ coalescing islands that they set up to form the sheet. The four snapshots are (a) at the moment when the tearing mode goes nonlinear (
$v_x$’). The solid magenta lines are separatrices demarcating two ‘global’ coalescing islands that they set up to form the sheet. The four snapshots are (a) at the moment when the tearing mode goes nonlinear (![]() $w\sim \delta _{\mathrm {in}}$; see Appendix D.2), (b) a little later, showing formation of secondary sheets (and so collapse of inter-island
$w\sim \delta _{\mathrm {in}}$; see Appendix D.2), (b) a little later, showing formation of secondary sheets (and so collapse of inter-island ![]() $X$-points), (c) later on, with a secondary instability of these sheets manifesting itself as more plasmoids appear (cf. Appendix D.5.2), and (d) in saturation, which for them is the period of stochastic but statistically steady and fast (with a rate independent of
$X$-points), (c) later on, with a secondary instability of these sheets manifesting itself as more plasmoids appear (cf. Appendix D.5.2), and (d) in saturation, which for them is the period of stochastic but statistically steady and fast (with a rate independent of ![]() $S_\xi$) reconnection and which obviously also corresponds to islands reaching the width of the sheet and starting to form a stochastic chain, moving and coalescing (see Appendix D.6). Note that all of this evolution happens within one Alfvén time, although the initial-growth stage does need a few Alfvén times to get going.
$S_\xi$) reconnection and which obviously also corresponds to islands reaching the width of the sheet and starting to form a stochastic chain, moving and coalescing (see Appendix D.6). Note that all of this evolution happens within one Alfvén time, although the initial-growth stage does need a few Alfvén times to get going.
D.5.2. Recursive tearing
It is not a difficult leap to realise that if a collapsing ‘global’ MHD sheet-like configuration (which, the way it was introduced at the beginning of Appendix D.5, was manifestly an ![]() $X$-point configuration) is unstable to tearing, the secondary
$X$-point configuration) is unstable to tearing, the secondary ![]() $X$-points generated by this tearing can also be unstable to (secondary) tearing and thus might not ‘complete’ the collapse into ‘proper’ SP sheets that was posited for them above. This can happen if the secondary tearing has a shorter growth time than the primary one, which, as we are about to see, is always the case. This conjures up an image of recursive tearings piling up on top of each other ad infinitum or, rather, until the inter-island
$X$-points generated by this tearing can also be unstable to (secondary) tearing and thus might not ‘complete’ the collapse into ‘proper’ SP sheets that was posited for them above. This can happen if the secondary tearing has a shorter growth time than the primary one, which, as we are about to see, is always the case. This conjures up an image of recursive tearings piling up on top of each other ad infinitum or, rather, until the inter-island ![]() $X$-points have small enough Lundquist numbers to be stable. At that point, they can all collapse properly into mini-SP-sheets and we are left with a multiscale population of fully nonlinear islands, which can now break up the ‘mother sheet’ and/or start interacting with each other and form a stochastic chain described in Appendix D.6. For the purposes of the discussion in the main text (§ 7.2), the issue is whether we should be concerned that the outcome is not just a number of flux ropes of one size
$X$-points have small enough Lundquist numbers to be stable. At that point, they can all collapse properly into mini-SP-sheets and we are left with a multiscale population of fully nonlinear islands, which can now break up the ‘mother sheet’ and/or start interacting with each other and form a stochastic chain described in Appendix D.6. For the purposes of the discussion in the main text (§ 7.2), the issue is whether we should be concerned that the outcome is not just a number of flux ropes of one size ![]() $\sim \lambda$, but a whole multiscale distribution of them.
$\sim \lambda$, but a whole multiscale distribution of them.
The first model of recursive tearing was proposed in the seminal paper by Shibata & Tanuma (Reference Shibata and Tanuma2001), and was more recently numerically tested and amended by Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) (both models are usefully reviewed by Singh et al. Reference Singh, Pucci, Tenerani, Shibata, Hillier and Velli2019). I shall explain their argument first, and then propose a certain modification of it that, in my view, makes it more plausible (and does lead to one important consequence).
Let us work on the assumption that the secondary tearing of an inter-island ![]() $X$-point works in the same way as the primary tearing described in Appendix D.1.4 (see figure 47). Therefore, we can assign our old equilibrium parameters to the
$X$-point works in the same way as the primary tearing described in Appendix D.1.4 (see figure 47). Therefore, we can assign our old equilibrium parameters to the ![]() $i$-th level of tearing:
$i$-th level of tearing:
The same quantities at the ![]() $(i+1)$-st level are then determined by the resulting perturbation in a way that depends on a number of assumptions.
$(i+1)$-st level are then determined by the resulting perturbation in a way that depends on a number of assumptions.

Figure 47. A plot, adapted from Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b) (© AAS, reproduced with permission), of the ![]() $b_x=-ik_y\psi (x)$ profiles (cf. figure 41) for nested tearing modes: primary (black), secondary (red) and tertiary (blue). They extracted these from a direct numerical simulation of a recursively tearing sheet. This is a remarkably clean example of the similarity of tearing at ever smaller scales.
$b_x=-ik_y\psi (x)$ profiles (cf. figure 41) for nested tearing modes: primary (black), secondary (red) and tertiary (blue). They extracted these from a direct numerical simulation of a recursively tearing sheet. This is a remarkably clean example of the similarity of tearing at ever smaller scales.
First, as announced above, let us assume that the ‘equilibrium’ (D60) will produce a tearing perturbation with growth rate and wavenumber given by (D32):
where (cf. (D46))
This is an assumption, not a certainty, because the local ‘equilibrium’ set up by the ![]() $i$-th tearing perturbation, which features flows as well as fields, is not a priori obliged to be tearing unstable in exactly the same way as a very simple static equilibrium used in Appendix D.1. Flows are expected to have a seriously stabilising effect if
$i$-th tearing perturbation, which features flows as well as fields, is not a priori obliged to be tearing unstable in exactly the same way as a very simple static equilibrium used in Appendix D.1. Flows are expected to have a seriously stabilising effect if
(Bulanov et al. Reference Bulanov, Syrovatskiǐ and Sakai1978, Reference Bulanov, Sakai and Syrovatskii1979; Biskamp Reference Biskamp1986; Shi et al. Reference Shi, Velli and Tenerani2018; Tolman et al. Reference Tolman, Loureiro and Uzdensky2018). This is also the ‘ideal-tearing’ threshold (D57), at which the tearing only just outperforms the ![]() $X$-point collapse (except, for
$X$-point collapse (except, for ![]() $\mathrm {Pm}\gg 1$, the Alfvénic outflow here is tempered by viscosity, because secondary-sheet dynamics, as well as tearing, happen at scales where viscosity matters). Our second assumption, therefore, following both Shibata & Tanuma (Reference Shibata and Tanuma2001) and Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016), will be that (D63) holds at every level, i.e., tearing at every level is ideal and marginal. It makes sense that it should not be much faster than that, otherwise the
$\mathrm {Pm}\gg 1$, the Alfvénic outflow here is tempered by viscosity, because secondary-sheet dynamics, as well as tearing, happen at scales where viscosity matters). Our second assumption, therefore, following both Shibata & Tanuma (Reference Shibata and Tanuma2001) and Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016), will be that (D63) holds at every level, i.e., tearing at every level is ideal and marginal. It makes sense that it should not be much faster than that, otherwise the ![]() $i$-th-level ‘equilibrium’ would have gone unstable earlier in its evolution.
$i$-th-level ‘equilibrium’ would have gone unstable earlier in its evolution.
The next steps are less obvious. We need to determine three quantities at the ![]() $(i+1)$-st level of tearing:
$(i+1)$-st level of tearing: ![]() $v_{i+1}$,
$v_{i+1}$, ![]() $\lambda _{i+1}$ and
$\lambda _{i+1}$ and ![]() $\xi _{i+1}$. Imposing (D63) at every level reduces this to two, so we need two further assumptions. Shibata & Tanuma (Reference Shibata and Tanuma2001) propose, first, that the length of the
$\xi _{i+1}$. Imposing (D63) at every level reduces this to two, so we need two further assumptions. Shibata & Tanuma (Reference Shibata and Tanuma2001) propose, first, that the length of the ![]() $(i+1)$-st sheet is the wavelength of the
$(i+1)$-st sheet is the wavelength of the ![]() $i$-th tearing mode (D61), viz.,
$i$-th tearing mode (D61), viz.,
and, secondly, that the ![]() $X$-point collapse proceeds far enough for the reconnecting field to be the same at all levels of tearing:
$X$-point collapse proceeds far enough for the reconnecting field to be the same at all levels of tearing:
Both of these assumptions can be doubted and revised, but, before doing this, let us see what they imply.
Using (D65), (D63), and (D64), in that order, we get
 \begin{equation} \frac{\tilde S_{i+1}}{\tilde S_i} = \frac{v_{i+1}\lambda_{i+1}}{v_i\lambda_i} \sim \frac{\lambda_{i+1}}{\lambda_i} \sim \frac{\xi_{i+1}\tilde S_{i+1}^{{-}1/2}}{\lambda_i} \sim \tilde S_i^{1/4}\tilde S_{i+1}^{{-}1/2}\quad\Rightarrow\quad \tilde S_{i+1}\sim \tilde S_i^{5/6}. \end{equation}
\begin{equation} \frac{\tilde S_{i+1}}{\tilde S_i} = \frac{v_{i+1}\lambda_{i+1}}{v_i\lambda_i} \sim \frac{\lambda_{i+1}}{\lambda_i} \sim \frac{\xi_{i+1}\tilde S_{i+1}^{{-}1/2}}{\lambda_i} \sim \tilde S_i^{1/4}\tilde S_{i+1}^{{-}1/2}\quad\Rightarrow\quad \tilde S_{i+1}\sim \tilde S_i^{5/6}. \end{equation}
This implies, inter alia, that the ![]() $(i+1)$-st tearing starts only when islands grow a bit larger than the width
$(i+1)$-st tearing starts only when islands grow a bit larger than the width ![]() $w\sim \delta _{\mathrm {in}}$ that they achieved when the
$w\sim \delta _{\mathrm {in}}$ that they achieved when the ![]() $i$-th tearing went nonlinear: using (D41) with
$i$-th tearing went nonlinear: using (D41) with ![]() $n=1$, we can estimate
$n=1$, we can estimate
It is not obvious that it should be so, but it is reassuring that the secondary instability happens after the onset of the nonlinear stage, and not before (the conviction that, in fact, the secondary tearing must start right at the onset of nonlinearity led Tenerani et al. Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016 to their version of recursive tearing, which I will discuss at the and of this section).
The assumption (D65) is perhaps questionable. I do not see why the local ![]() $X$-point ‘equilibria’ produced in the nonlinear stage of the primary tearing should stay stable until
$X$-point ‘equilibria’ produced in the nonlinear stage of the primary tearing should stay stable until ![]() $X$-point collapse makes
$X$-point collapse makes ![]() $v_i\sim v_0$ (of course, knowing definitely whether they do so requires a careful quantitative theory of the secondary tearing, currently unavailable). It seems more natural to assume that the effective ‘equilibrium’ upstream field for the secondary tearing (
$v_i\sim v_0$ (of course, knowing definitely whether they do so requires a careful quantitative theory of the secondary tearing, currently unavailable). It seems more natural to assume that the effective ‘equilibrium’ upstream field for the secondary tearing (![]() $v_{i+1}$) is reduced from that for the primary one (
$v_{i+1}$) is reduced from that for the primary one (![]() $v_i$) by the fact that the islands generated by the latter are not as wide (
$v_i$) by the fact that the islands generated by the latter are not as wide (![]() $\lambda _{i+1}$) as the primary sheet (
$\lambda _{i+1}$) as the primary sheet (![]() $\lambda _i$). Then
$\lambda _i$). Then
This is derived in the same way as ![]() $\delta b_y/v_{\mathrm {A}y}\sim w/\lambda$ was from (D42) and (D43), but now
$\delta b_y/v_{\mathrm {A}y}\sim w/\lambda$ was from (D42) and (D43), but now ![]() $\delta b_y\to v_{i+1}$,
$\delta b_y\to v_{i+1}$, ![]() $v_{\mathrm {A}y}\to v_i$,
$v_{\mathrm {A}y}\to v_i$, ![]() $w\to \lambda _{i+1}$ and
$w\to \lambda _{i+1}$ and ![]() $\lambda \to \lambda _i$. This idea, sometimes called the ‘embedding effect’, goes back to Cassak & Drake (Reference Cassak and Drake2009), whose simulations appeared to support the notion that secondary tearing would get going in these circumstances (a more recent paper by Del Sarto & Ottaviani Reference Del Sarto and Ottaviani2017 took the same view).
$\lambda \to \lambda _i$. This idea, sometimes called the ‘embedding effect’, goes back to Cassak & Drake (Reference Cassak and Drake2009), whose simulations appeared to support the notion that secondary tearing would get going in these circumstances (a more recent paper by Del Sarto & Ottaviani Reference Del Sarto and Ottaviani2017 took the same view).
Adopting (D68) instead of (D65) in the Shibata & Tanuma (Reference Shibata and Tanuma2001) scheme for recursive tearing amounts to replacing (D66) with
This new scheme does not lose any of the properties of the old one that made the latter plausible:
(i) since (D67) becomes
the ![]() $(i+1)$-st tearing still sets on during the nonlinear stage of the
$(i+1)$-st tearing still sets on during the nonlinear stage of the ![]() $i$-th one;
$i$-th one;
(ii) the Lundquist number gets smaller at every level:
(iii) the tearing growth rate nevertheless increases:
so the perturbations always grow faster than the underlying ‘equilibria’ evolve (![]() $\gamma _{i+1}\gg \gamma _i$). Thus, the entire hierarchy of islands is created very quickly, on the time scale of (a few times)
$\gamma _{i+1}\gg \gamma _i$). Thus, the entire hierarchy of islands is created very quickly, on the time scale of (a few times) ![]() $\gamma _0^{-1}\sim \xi _0/u_0$. If there is some critical Lundquist number
$\gamma _0^{-1}\sim \xi _0/u_0$. If there is some critical Lundquist number ![]() $\tilde S_{\mathrm {c}}$ required for these tearing modes to be unstable, (D71) allows us to work out the maximum number of times that the recursive tearing will be iterated before
$\tilde S_{\mathrm {c}}$ required for these tearing modes to be unstable, (D71) allows us to work out the maximum number of times that the recursive tearing will be iterated before ![]() $X$-points can collapse unimpeded into proper, stable, reconnecting current sheets:
$X$-points can collapse unimpeded into proper, stable, reconnecting current sheets:
It is obvious that in practice this will not be a large number at all.
The embedding assumption (D68) enables me to argue that, while islands at all scales below ![]() $\lambda _0$ are produced, they do not contain much energy. Indeed, the effective energy density in the
$\lambda _0$ are produced, they do not contain much energy. Indeed, the effective energy density in the ![]() $i$-th-level islands is
$i$-th-level islands is
where the extra factor of ![]() $\lambda /\lambda _0$ represents the fraction of the volume that these islands fill, assuming that they are arranged neatly in a row. When translated into a spectral slope, this gives
$\lambda /\lambda _0$ represents the fraction of the volume that these islands fill, assuming that they are arranged neatly in a row. When translated into a spectral slope, this gives ![]() $k_{\perp }^{-4}$, which is easily dominated by the
$k_{\perp }^{-4}$, which is easily dominated by the ![]() $k_{\perp }^{-11/5}$ spectrum of the tearing-mediated turbulence derived in § 7.2.1. If this is true, we should be allowed to dismiss recursive tearing as a side show in the context of the tearing-mediated cascade.
$k_{\perp }^{-11/5}$ spectrum of the tearing-mediated turbulence derived in § 7.2.1. If this is true, we should be allowed to dismiss recursive tearing as a side show in the context of the tearing-mediated cascade.
Let me conclude this section with some further nuances and caveats. After the entire tearing hierarchy has formed, the ![]() $X$-points fully collapse and the sheet is perhaps broken up by its spawn of islands, of which only the largest ones are energetically of any consequence (see (D74)). Were it instead to persist for some time (which is not not impossible: see §§ 7.4.1 and 7.4.2), everything would change in the course of the subsequent dynamics of its plasmoid (island) population: plasmoid shapes, their number (they travel along the sheet, coalesce, and eventually get ejected from the sheet), field amplitudes in them (reconnection continues via elementary inter-plasmoid current sheets that are short enough to be stable). Such stochastic plasmoid chains have been studied numerically by many people (see references in Appendix D.4.2). Their statistical steady state is, I believe, correctly described by the theoretical model of Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010), reviewed in Appendix D.6. In this context, an important caveat to the theory of recursive tearing discussed above is that imagining that all those nonlinear processes happen after recursive tearing has run its course was surely a gross idealisation. Namely, I assumed implicitly that secondary tearing would be the first instability to kick in once the primary tearing mode became nonlinear – and thus ignored, e.g., the possibility, raised some time ago by Malara et al. (Reference Malara, Veltri and Carbone1992), that the islands produced by tearing might start coalescing before secondary tearing destabilised the inter-island
$X$-points fully collapse and the sheet is perhaps broken up by its spawn of islands, of which only the largest ones are energetically of any consequence (see (D74)). Were it instead to persist for some time (which is not not impossible: see §§ 7.4.1 and 7.4.2), everything would change in the course of the subsequent dynamics of its plasmoid (island) population: plasmoid shapes, their number (they travel along the sheet, coalesce, and eventually get ejected from the sheet), field amplitudes in them (reconnection continues via elementary inter-plasmoid current sheets that are short enough to be stable). Such stochastic plasmoid chains have been studied numerically by many people (see references in Appendix D.4.2). Their statistical steady state is, I believe, correctly described by the theoretical model of Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010), reviewed in Appendix D.6. In this context, an important caveat to the theory of recursive tearing discussed above is that imagining that all those nonlinear processes happen after recursive tearing has run its course was surely a gross idealisation. Namely, I assumed implicitly that secondary tearing would be the first instability to kick in once the primary tearing mode became nonlinear – and thus ignored, e.g., the possibility, raised some time ago by Malara et al. (Reference Malara, Veltri and Carbone1992), that the islands produced by tearing might start coalescing before secondary tearing destabilised the inter-island ![]() $X$-points. Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) claim to see this in their simulations.
$X$-points. Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) claim to see this in their simulations.
The alternative version of recursive tearing proposed by Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) was, it seems, inspired by the notion that the nonlinear rearrangements of the island population that occurred in a plasmoid chain gave one licence to discard the Shibata & Tanuma (Reference Shibata and Tanuma2001) assumption (D64) that the number of islands at each level was decided by the wavenumber of the fastest-growing tearing mode at that level. Instead they propose (and claim confirmed in their simulations) that the width of the ![]() $(i+1)$-st sheet is, in fact, the island width
$(i+1)$-st sheet is, in fact, the island width ![]() $w\sim \delta _{\mathrm {in}}$ of the
$w\sim \delta _{\mathrm {in}}$ of the ![]() $i$-th tearing mode right at the onset of nonlinearity, viz., using (D41) with
$i$-th tearing mode right at the onset of nonlinearity, viz., using (D41) with ![]() $n=1$,
$n=1$,
Then, instead of (D66),
where the assumption (D65) that the upstream field is the equilibrium field has been retained. The scaling of the Lundquist number is the same as (D71), but for a different reason. If (D65) were to be replaced by the embedding assumption (D68), one gets instead
Under this scheme, one does not need to know ![]() $\xi _i$, but if one wants to know it, it is determined from the ideal-tearing condition (D63) rather than from the wavelength of the tearing mode (D64) (otherwise one gets a tearing growth rate
$\xi _i$, but if one wants to know it, it is determined from the ideal-tearing condition (D63) rather than from the wavelength of the tearing mode (D64) (otherwise one gets a tearing growth rate ![]() $\gamma _i$ that far exceeds the marginal level
$\gamma _i$ that far exceeds the marginal level ![]() $\sim u_i/\xi _i$). One somewhat awkward implication is that the
$\sim u_i/\xi _i$). One somewhat awkward implication is that the ![]() $i$-th tearing is expected to find some way of producing more numerous, smaller islands than allowed by the wavenumber
$i$-th tearing is expected to find some way of producing more numerous, smaller islands than allowed by the wavenumber ![]() $k_*$ of its fastest-growing mode: from (D63), (D61), and (D76),
$k_*$ of its fastest-growing mode: from (D63), (D61), and (D76),
or ![]() $\xi _{i+1}k_{*,i}\sim \tilde S_i^{-1/4}$ if (D77) is used instead of (D76).
$\xi _{i+1}k_{*,i}\sim \tilde S_i^{-1/4}$ if (D77) is used instead of (D76).
Thus, four recursive-tearing scenarios are available: (D66), (D69), (D76), and (D77). Which of these you believe depends on which of the plausible assumptions discussed above you find most plausible – or best verified numerically (a hard task). Do the differences between them really matter? Certainly not for the qualitative picture of recursive tearing quickly leading to the formation of a fully nonlinear plasmoid chain. Once this has happened, i.e., once all ![]() $X$-points have fully collapsed, (D65) will certainly be true at all levels (Uzdensky et al. Reference Uzdensky, Loureiro and Schekochihin2010; Loureiro et al. Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012). However, if it were also true and, consequently, (D68) untrue, during the initial recursive tearing, as Shibata & Tanuma (Reference Shibata and Tanuma2001) and Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) would have it, then I would not be able to wave away the role of the secondary islands in the disruption process, as I did in § 7.4.1. Indeed, modifying (D74) to have
$X$-points have fully collapsed, (D65) will certainly be true at all levels (Uzdensky et al. Reference Uzdensky, Loureiro and Schekochihin2010; Loureiro et al. Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012). However, if it were also true and, consequently, (D68) untrue, during the initial recursive tearing, as Shibata & Tanuma (Reference Shibata and Tanuma2001) and Tenerani et al. (Reference Tenerani, Velli, Rappazzo and Pucci2015b, Reference Tenerani, Velli, Pucci, Landi and Rappazzo2016) would have it, then I would not be able to wave away the role of the secondary islands in the disruption process, as I did in § 7.4.1. Indeed, modifying (D74) to have ![]() $v_i\sim v_0$, one gets
$v_i\sim v_0$, one gets
which corresponds to a spectrum of ![]() $k_{\perp }^{-2}$ (cf. Appendix D.6.2). This could swamp the
$k_{\perp }^{-2}$ (cf. Appendix D.6.2). This could swamp the ![]() $k_{\perp }^{-11/5}$ spectrum of the tearing-mediated turbulence (§ 7.2.1) unless mitigated by some volume-filling effects (as, e.g., in Tenerani & Velli Reference Tenerani and Velli2020b). In any case, there would then be a legitimate question of how all these islands might modify, or even completely determine, the tearing-mediated-range statistics. These issues are discussed in § 7.4.3 and Appendix D.6.3.
$k_{\perp }^{-11/5}$ spectrum of the tearing-mediated turbulence (§ 7.2.1) unless mitigated by some volume-filling effects (as, e.g., in Tenerani & Velli Reference Tenerani and Velli2020b). In any case, there would then be a legitimate question of how all these islands might modify, or even completely determine, the tearing-mediated-range statistics. These issues are discussed in § 7.4.3 and Appendix D.6.3.
D.6. Fast MHD reconnection
I have referred several times already to a fully nonlinear plasmoid chain being a possible end result of recursive tearing (Appendix D.5.2) and making reconnection fast (§ 7.4). Let me reproduce here, in broad brush, the theory of this regime by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010).
Once all the ![]() $X$-points at all levels of recursive tearing have collapsed, the current sheet becomes a chain of plasmoids of different sizes connected by the longest SP sheets that can remain stable, i.e., ones whose ‘critical’ length and width are
$X$-points at all levels of recursive tearing have collapsed, the current sheet becomes a chain of plasmoids of different sizes connected by the longest SP sheets that can remain stable, i.e., ones whose ‘critical’ length and width are
where ![]() $u_y\sim v _{\mathrm {A}y}/\sqrt {1+\mathrm {Pm}}$ is the outflow velocity (see (D50)) and
$u_y\sim v _{\mathrm {A}y}/\sqrt {1+\mathrm {Pm}}$ is the outflow velocity (see (D50)) and ![]() $\tilde S_{\mathrm {c}}$ is the critical Lundquist number (D53) for the plasmoid instability. The inter-plasmoid sheets cannot be any longer than
$\tilde S_{\mathrm {c}}$ is the critical Lundquist number (D53) for the plasmoid instability. The inter-plasmoid sheets cannot be any longer than ![]() $\ell _{\mathrm {c}}$ because the moment they get stretched longer they go unstable and break up into more plasmoids. Thus, the number of plasmoids typically found in a sheet of length
$\ell _{\mathrm {c}}$ because the moment they get stretched longer they go unstable and break up into more plasmoids. Thus, the number of plasmoids typically found in a sheet of length ![]() $\ell$, in steady state, is just
$\ell$, in steady state, is just ![]() $N\sim \ell /\ell _{\mathrm {c}} \sim \tilde S_\ell /\tilde S_{\mathrm {c}}$. These plasmoids are all of different sizes, having been generated at various levels of recursive tearing or as a result of coalescence of earlier-generation plasmoids. One can think of them as belonging to many hierarchical levels, with plasmoids of the
$N\sim \ell /\ell _{\mathrm {c}} \sim \tilde S_\ell /\tilde S_{\mathrm {c}}$. These plasmoids are all of different sizes, having been generated at various levels of recursive tearing or as a result of coalescence of earlier-generation plasmoids. One can think of them as belonging to many hierarchical levels, with plasmoids of the ![]() $n$-th level living in ‘local’ sheets bounded by pairs of
$n$-th level living in ‘local’ sheets bounded by pairs of ![]() $(n-1)$-st-level plasmoids. At every level, they are all moving along their local sheet with a mean (visco-)Alfvénic outflow
$(n-1)$-st-level plasmoids. At every level, they are all moving along their local sheet with a mean (visco-)Alfvénic outflow ![]() $u_y$, the same at every level, eventually getting ejected into (coalesced with) the previous-level plasmoids.
$u_y$, the same at every level, eventually getting ejected into (coalesced with) the previous-level plasmoids.
It is surprisingly easy to argue that reconnection in such a system (illustrated by the lowest panel of figure 46) is fast. First notice that the plasmoids travelling along the sheet and eventually ejected from it would carry no reconnected flux (no ![]() $\delta b_x$) if they only contained closed field lines. However, since the upstream (reconnecting) field
$\delta b_x$) if they only contained closed field lines. However, since the upstream (reconnecting) field ![]() $v_{\mathrm {A}y}$ decreases gently from the centre of the sheet (
$v_{\mathrm {A}y}$ decreases gently from the centre of the sheet (![]() $y=0$) outwards along
$y=0$) outwards along ![]() $y$, the reconnection on the smaller-
$y$, the reconnection on the smaller-![]() $|y|$ side of each plasmoid is slightly faster than on the larger-
$|y|$ side of each plasmoid is slightly faster than on the larger-![]() $|y|$ side of it. Therefore, each plasmoid carries some open flux (figure 48) and it is the ejection of this open flux that contributes to the overall reconnection rate. At every level
$|y|$ side of it. Therefore, each plasmoid carries some open flux (figure 48) and it is the ejection of this open flux that contributes to the overall reconnection rate. At every level ![]() $n$ in the plasmoid hierarchy, reconnection in a sheet containing
$n$ in the plasmoid hierarchy, reconnection in a sheet containing ![]() $n$-th-and-higher-level plasmoids and bounded by two
$n$-th-and-higher-level plasmoids and bounded by two ![]() $(n-1)$-st-level ones adds to the open flux enveloping the
$(n-1)$-st-level ones adds to the open flux enveloping the ![]() $(n-1)$-st-level plasmoid on the larger-
$(n-1)$-st-level plasmoid on the larger-![]() $|y|$ side and subtracts from the one on the smaller-
$|y|$ side and subtracts from the one on the smaller-![]() $|y|$ side. The overall reconnected flux is the sum over these contributions, all of which cancel each other except for the one from the centre of the sheet. Thus, the overall reconnection rate is just the reconnection rate in the central elementary sheet, given by (D51) with the critical Lundquist number
$|y|$ side. The overall reconnected flux is the sum over these contributions, all of which cancel each other except for the one from the centre of the sheet. Thus, the overall reconnection rate is just the reconnection rate in the central elementary sheet, given by (D51) with the critical Lundquist number ![]() $\tilde S_{\mathrm {c}}$:
$\tilde S_{\mathrm {c}}$:
independent of the actual Lundquist number ![]() $\tilde S_\ell$ and the same at every level in the hierarchy.
$\tilde S_\ell$ and the same at every level in the hierarchy.

Figure 48. Contour plot of the magnetic flux function illustrating the open flux. This is taken from a section of a 2D MHD simulation of a plasmoid chain; the centre of the sheet is somewhere far away on the left. (Reprinted with permission from Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010), copyright (2010) by the American Physical Society.)
This result can be rederived (or re-interpreted) as a modification of the SP reconnection, proposed by Shibata & Tanuma (Reference Shibata and Tanuma2001), in which the effective width ![]() $\delta _{\mathrm {eff}}^{(n-1)}$ of the sheet (whose length is
$\delta _{\mathrm {eff}}^{(n-1)}$ of the sheet (whose length is ![]() $\ell ^{(n-1)}$) connecting the
$\ell ^{(n-1)}$) connecting the ![]() $(n-1)$-st-level plasmoids, for the purposes of mass (and with it, flux) ejection, is the width of the largest plasmoids in that sheet, which are the
$(n-1)$-st-level plasmoids, for the purposes of mass (and with it, flux) ejection, is the width of the largest plasmoids in that sheet, which are the ![]() $n$-th-level plasmoids. Then the reconnection rate in such a sheet, i.e., the rate of growth of the flux
$n$-th-level plasmoids. Then the reconnection rate in such a sheet, i.e., the rate of growth of the flux ![]() $\varPsi ^{(n-1)}$ in the
$\varPsi ^{(n-1)}$ in the ![]() $(n-1)$-st-level plasmoids, is
$(n-1)$-st-level plasmoids, is
where the inflow velocity ![]() $u_x^{(n-1)}$ has been calculated from mass conservation, as in (D48), and the (visco-)Alfvénic outflow
$u_x^{(n-1)}$ has been calculated from mass conservation, as in (D48), and the (visco-)Alfvénic outflow ![]() $u_y$ is the same at every level of the hierarchy, because the inter-plasmoid
$u_y$ is the same at every level of the hierarchy, because the inter-plasmoid ![]() $X$-points are all fully collapsed, so the upstream field is
$X$-points are all fully collapsed, so the upstream field is ![]() $v_{\mathrm {A}y}$ at every level. The
$v_{\mathrm {A}y}$ at every level. The ![]() $n$-th-level plasmoids’ width is then found by letting the perturbed field inside them be comparable to that upstream field:
$n$-th-level plasmoids’ width is then found by letting the perturbed field inside them be comparable to that upstream field:
Finally, the flux typically contained inside the ![]() $n$-th-level plasmoid can be estimated as the reconnection rate at that level times the time that an
$n$-th-level plasmoid can be estimated as the reconnection rate at that level times the time that an ![]() $n$-th-level plasmoid will take to travel out of the
$n$-th-level plasmoid will take to travel out of the ![]() $(n-1)$-st-level sheet:
$(n-1)$-st-level sheet:
so the reconnection rate is the same at every level and thus equal to the reconnection rate (D81) at ![]() $n\to \infty$, i.e., in the most elementary sheet, q.e.d.
$n\to \infty$, i.e., in the most elementary sheet, q.e.d.
Thus, the basic reason for reconnection becoming fast in this way is that plasmoids make the SP sheet effectively fatter, relieving the severe constraint that pumping mass and flux through a narrow funnel would otherwise impose. The only remaining constraint is the need to get the SP sheet to be at least as long as ![]() $\ell _{\mathrm {c}}$ in order for it to be able to break up into plasmoids.
$\ell _{\mathrm {c}}$ in order for it to be able to break up into plasmoids.
The fact that SP reconnection transitions to a fast, plasmoid-dominated regime at ![]() $S_\ell \gtrsim S _{\mathrm {c}}\sim 10^4$, with the reconnection rate set by
$S_\ell \gtrsim S _{\mathrm {c}}\sim 10^4$, with the reconnection rate set by ![]() $S_{\mathrm {c}}$, was confirmed numerically by Bhattacharjee et al. (Reference Bhattacharjee, Huang, Yang and Rogers2009), Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012, see figure 49a) and in numerous subsequent simulations of bespoke reconnecting configurations (many of them cited in Appendix D.4.2). Whether this kind of fast reconnection and statistically steady plasmoid chains occur as local features of MHD turbulence is a tricky question: see §§ 7.4.1 and 7.4.2. Note that, in Appendix D.7, I will discuss another, quite different, sense in which reconnection is sometimes described as fast when it occurs in a turbulent environment.
$S_{\mathrm {c}}$, was confirmed numerically by Bhattacharjee et al. (Reference Bhattacharjee, Huang, Yang and Rogers2009), Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012, see figure 49a) and in numerous subsequent simulations of bespoke reconnecting configurations (many of them cited in Appendix D.4.2). Whether this kind of fast reconnection and statistically steady plasmoid chains occur as local features of MHD turbulence is a tricky question: see §§ 7.4.1 and 7.4.2. Note that, in Appendix D.7, I will discuss another, quite different, sense in which reconnection is sometimes described as fast when it occurs in a turbulent environment.

Figure 49. (a) Reconnection rate (blue squares), normalised, in my notation, to ![]() $u_yv_{\mathrm {A}y}$, in 2D MHD
$u_yv_{\mathrm {A}y}$, in 2D MHD ![]() $\mathrm {Pm}=1$ simulations by Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012). Transition at
$\mathrm {Pm}=1$ simulations by Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012). Transition at ![]() $S_\ell \sim 10^4$ from the SP scaling to the fast-reconnection regime (D81) is manifest. (b) Plasmoid-width distribution function in the same simulations, from the same paper, confirming the scaling predicted by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010) (see Appendix D.6.1). (Reprinted from Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012) with the permission of AIP Publishing.)
$S_\ell \sim 10^4$ from the SP scaling to the fast-reconnection regime (D81) is manifest. (b) Plasmoid-width distribution function in the same simulations, from the same paper, confirming the scaling predicted by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010) (see Appendix D.6.1). (Reprinted from Loureiro et al. (Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012) with the permission of AIP Publishing.)
D.6.1. Plasmoid flux and width distribution
The relation (D84), combined with the constant reconnection rate (D81), allows one to determine the distribution function of the plasmoid fluxes – not necessary for the above argument, but a nice, testable result, which will prove useful in what follows. The number of plasmoids with ![]() $\varPsi >\varPsi ^{(n)}$, or, equivalently,
$\varPsi >\varPsi ^{(n)}$, or, equivalently, ![]() $w > w^{(n)}$, in the sheet of overall length
$w > w^{(n)}$, in the sheet of overall length ![]() $\ell$ is
$\ell$ is
the last expression following from (D83). Therefore, the plasmoid-flux distribution function is ![]() $f(\varPsi )\propto \varPsi ^{-2}$ and the plasmoid-width distribution function is
$f(\varPsi )\propto \varPsi ^{-2}$ and the plasmoid-width distribution function is ![]() $f(w)\propto w^{-2}$. These scalings are indeed corroborated numerically (Loureiro et al. Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012, see figure 49b).
$f(w)\propto w^{-2}$. These scalings are indeed corroborated numerically (Loureiro et al. Reference Loureiro, Samtaney, Schekochihin and Uzdensky2012, see figure 49b).
D.6.2. Spectrum of plasmoid chain
It is instructive to work out the spectrum of the plasmoid chain imagined by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010). This chain is a multi-scale structure, but not one that is naturally described as a local constant-flux cascade: rather, energy is brought into it by the incoming (reconnecting) field ![]() $v_{\mathrm {A}y}$ and deposited by the reconnection processes (which include coalescence) into plasmoids at all levels. The field inside these plasmoids is always
$v_{\mathrm {A}y}$ and deposited by the reconnection processes (which include coalescence) into plasmoids at all levels. The field inside these plasmoids is always ![]() $v_{\mathrm {A}y}$, independently of their size (see (D83)). However, the plasmoids are arranged in a row along the chain, rather than randomly in space, so the smaller ones fill less space than the larger ones and thus the effective energy density associated with them is smaller, viz., for
$v_{\mathrm {A}y}$, independently of their size (see (D83)). However, the plasmoids are arranged in a row along the chain, rather than randomly in space, so the smaller ones fill less space than the larger ones and thus the effective energy density associated with them is smaller, viz., for ![]() $n$-th-level plasmoids,
$n$-th-level plasmoids,
where ![]() $w^{(1)}$ is the width of the largest plasmoids and, therefore, of the chain.Footnote 104 Note that the size of the plasmoids in the
$w^{(1)}$ is the width of the largest plasmoids and, therefore, of the chain.Footnote 104 Note that the size of the plasmoids in the ![]() $y$ direction (along the chain) does not matter here because the smaller plasmoids are more numerous than the larger ones by the exact same factor by which they are shorter in length (
$y$ direction (along the chain) does not matter here because the smaller plasmoids are more numerous than the larger ones by the exact same factor by which they are shorter in length (![]() $\sim \ell /\ell ^{(n)}$). Now let
$\sim \ell /\ell ^{(n)}$). Now let ![]() $\delta b_\mathrm {eff}^{(n)}=\delta b_{\mathrm {eff},\lambda }$,
$\delta b_\mathrm {eff}^{(n)}=\delta b_{\mathrm {eff},\lambda }$, ![]() $\lambda = w^{(n)}$. By (D83), (D84), (D85) and (D81),
$\lambda = w^{(n)}$. By (D83), (D84), (D85) and (D81),
so we have ![]() $w^{(1)}\sim \tilde S_{\mathrm {c}}^{-1/2}\ell$. From (D87), therefore,
$w^{(1)}\sim \tilde S_{\mathrm {c}}^{-1/2}\ell$. From (D87), therefore,
 \begin{equation} \delta b_{\mathrm{eff},\lambda} \sim v_{\mathrm{A}y}\tilde S_{\mathrm{c}}^{1/4}\left(\frac{\lambda}{\ell}\right)^{1/2}\quad\Rightarrow\quad E(k_x) \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}k_x^{{-}2}. \end{equation}
\begin{equation} \delta b_{\mathrm{eff},\lambda} \sim v_{\mathrm{A}y}\tilde S_{\mathrm{c}}^{1/4}\left(\frac{\lambda}{\ell}\right)^{1/2}\quad\Rightarrow\quad E(k_x) \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}k_x^{{-}2}. \end{equation}
I have used ![]() $k_x$, rather than
$k_x$, rather than ![]() $k_{\perp }$, because the direction of maximum variation here is very obviously
$k_{\perp }$, because the direction of maximum variation here is very obviously ![]() $x$, transverse to the sheet; it is, of course, true that
$x$, transverse to the sheet; it is, of course, true that ![]() $k_{\perp }\sim k_x$.
$k_{\perp }\sim k_x$.
The total energy flux into the chain, per unit volume, is
It is, therefore, possible to recover (D89) formally from a constant-flux argument in which the nonlinear time is the life time (ejection time) of an ![]() $n$-th-level plasmoid (Loureiro Reference Loureiro2016):
$n$-th-level plasmoid (Loureiro Reference Loureiro2016):
 \begin{equation} \frac{[\delta b_\mathrm{eff}^{(n)}\bigr]^2}{\tau_{\mathrm{nl}}^{(n)}} \sim \varepsilon,\quad \tau_{\mathrm{nl}}^{(n)}\sim \frac{\ell^{(n-1)}}{u_y}\sim\frac{\tilde S_{\mathrm{c}}^{1/2} w^{(n)}}{u_y}\quad\Rightarrow\quad \delta b_{\mathrm{eff},\lambda} \sim \left(\frac{\varepsilon}{u_y}\right)^{1/2}\tilde S_{\mathrm{c}}^{1/4}\lambda^{1/2}, \end{equation}
\begin{equation} \frac{[\delta b_\mathrm{eff}^{(n)}\bigr]^2}{\tau_{\mathrm{nl}}^{(n)}} \sim \varepsilon,\quad \tau_{\mathrm{nl}}^{(n)}\sim \frac{\ell^{(n-1)}}{u_y}\sim\frac{\tilde S_{\mathrm{c}}^{1/2} w^{(n)}}{u_y}\quad\Rightarrow\quad \delta b_{\mathrm{eff},\lambda} \sim \left(\frac{\varepsilon}{u_y}\right)^{1/2}\tilde S_{\mathrm{c}}^{1/4}\lambda^{1/2}, \end{equation}
which is the same as (D89), by way of (D90). Yet another way to derive the same result is via the plasmoid-width distribution function (D86) (Bárta et al. Reference Bárta, Skála, Karlický and Büchner2012): the energy in the plasmoids of width ![]() $\lambda \sim k_x^{-1}$ is, per unit volume,
$\lambda \sim k_x^{-1}$ is, per unit volume,
 \begin{equation} E(k_x)\mathrm{d} k_x \sim \frac{v_{\mathrm{A}y}^2 \tilde S_{\mathrm{c}}^{1/2}\lambda^2\mathrm{d} N(\lambda)}{\tilde S_{\mathrm{c}}^{{-}1/2}\ell^2} \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}\mathrm{d}\lambda \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}k_x^{{-}2}\mathrm{d} k_x, \end{equation}
\begin{equation} E(k_x)\mathrm{d} k_x \sim \frac{v_{\mathrm{A}y}^2 \tilde S_{\mathrm{c}}^{1/2}\lambda^2\mathrm{d} N(\lambda)}{\tilde S_{\mathrm{c}}^{{-}1/2}\ell^2} \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}\mathrm{d}\lambda \sim v_{\mathrm{A}y}^2\tilde S_{\mathrm{c}}^{1/2}\ell^{{-}1}k_x^{{-}2}\mathrm{d} k_x, \end{equation}whence follows the spectrum (D89).
All of this works on the assumption that parallel (to the mean field) dynamics do not upset things (plasmoid-width distribution etc.) in a major way. By the usual CB argument, plasmoids, which are flux ropes in 3D, cannot extend much farther along the mean field than an Alfvén wave can travel in some characteristic nonlinear time associated with the plasmoid. The most obvious estimate of this time is one in (D91), whence (Loureiro Reference Loureiro2016)
 \begin{equation} \frac{l_{{\parallel}}^{(n)}}{v_{\mathrm{A}}}\sim\tau_{\mathrm{nl}}^{(n)} \sim \frac{\tilde S_{\mathrm{c}}^{1/2}w^{(n)}}{u_y} \quad\Rightarrow\quad l_{{\parallel}} \sim \frac{v_{\mathrm{A}}}{u_y}\,\tilde S_{\mathrm{c}}^{1/2}\lambda \quad\Rightarrow\quad E(k_{{\parallel}}) \sim \frac{v_{\mathrm{A}y}^2 u_y}{v_{\mathrm{A}}\ell}\,k_{{\parallel}}^{{-}2}. \end{equation}
\begin{equation} \frac{l_{{\parallel}}^{(n)}}{v_{\mathrm{A}}}\sim\tau_{\mathrm{nl}}^{(n)} \sim \frac{\tilde S_{\mathrm{c}}^{1/2}w^{(n)}}{u_y} \quad\Rightarrow\quad l_{{\parallel}} \sim \frac{v_{\mathrm{A}}}{u_y}\,\tilde S_{\mathrm{c}}^{1/2}\lambda \quad\Rightarrow\quad E(k_{{\parallel}}) \sim \frac{v_{\mathrm{A}y}^2 u_y}{v_{\mathrm{A}}\ell}\,k_{{\parallel}}^{{-}2}. \end{equation}Thus, the chain's parallel spectrum is the same as the perpendicular one – this appears to be what Huang & Bhattacharjee (Reference Huang and Bhattacharjee2016) report for their 3D turbulent plasmoid chain. The ‘fluctuation-direction’ spectrum (cf. § 6.5) is the same again, because, clearly,
The ‘turbulence’ of plasmoids in a plasmoid chain has a fixed, scale-independent alignment angle equal to the reconnection rate, ![]() $\sim \tilde S_{\mathrm {c}}^{-1/2}$.
$\sim \tilde S_{\mathrm {c}}^{-1/2}$.
A sceptical reader might observe that a ![]() $k_x^{-2}$ spectrum for a plasmoid chain is in fact no big revelation because plasmoids are connected by elementary sheets (D80), which, being step-like ‘discontinuities’ of width
$k_x^{-2}$ spectrum for a plasmoid chain is in fact no big revelation because plasmoids are connected by elementary sheets (D80), which, being step-like ‘discontinuities’ of width ![]() $\delta _{\mathrm {c}}$ in
$\delta _{\mathrm {c}}$ in ![]() $x$, already on their own should give rise to a
$x$, already on their own should give rise to a ![]() $k_x^{-2}$ spectrum for all
$k_x^{-2}$ spectrum for all ![]() $k_x\lesssim \delta _{\mathrm {c}}^{-1}$ (the same argument already appeared in §§ 10.4 and 12.8). This is true, and so the point of the above calculation is that plasmoids’ energy distribution does not swamp the
$k_x\lesssim \delta _{\mathrm {c}}^{-1}$ (the same argument already appeared in §§ 10.4 and 12.8). This is true, and so the point of the above calculation is that plasmoids’ energy distribution does not swamp the ![]() $k_x^{-2}$ scaling of the spectrum; also, the same scaling in
$k_x^{-2}$ scaling of the spectrum; also, the same scaling in ![]() $k_y$ and
$k_y$ and ![]() $k_{\parallel }$ is a property of the plasmoids, not of the inter-plasmoid sheets.
$k_{\parallel }$ is a property of the plasmoids, not of the inter-plasmoid sheets.
D.6.3. Reconnection-driven turbulence
In fact, the reconnecting sheets seen in 3D simulations do not resemble all too closely the relatively orderly procession of multi-scale plasmoids envisioned by Uzdensky et al. (Reference Uzdensky, Loureiro and Schekochihin2010) and seen quite clearly in 2D simulations (see figure 46 and papers cited in Appendix D.4.2). In 3D, the chain looks like a strip of vigorous turbulence (figure 50), even if flux ropes (3D plasmoids) may be identifiable there, at least when a mean field is imposed.Footnote 105 One can think of this situation as a result of the flux ropes constantly going unstable and thus seeding turbulent cascades on multiple scales. A naïve but perhaps instructive model of such ‘reconnection driving’ of turbulence (promised in § 7.4.3) can be constructed by assuming that the energy density (D87) in the plasmoids at each level ![]() $n$ describes the ‘outer scale’ of a mini-cascade, which then proceeds in some regular MHD manner. For this argument, it does not matter what exactly this cascade is like, just that it has some power-law scaling below its mother plasmoid's scale
$n$ describes the ‘outer scale’ of a mini-cascade, which then proceeds in some regular MHD manner. For this argument, it does not matter what exactly this cascade is like, just that it has some power-law scaling below its mother plasmoid's scale ![]() $w^{(n)}$:
$w^{(n)}$:
 \begin{equation} \delta Z^{(n)}_\lambda \sim\delta b_\mathrm{eff}^{(n)}\left(\frac{\lambda}{w^{(n)}}\right)^\gamma \sim v_{\mathrm{A}y}\tilde S_{\mathrm{c}}^{1/4}\frac{\left[w^{(n)}\right]^{(1 - 2\gamma)/2}\lambda^\gamma}{\ell^{1/2}}, \qquad\lambda < w^{(n)}, \end{equation}
\begin{equation} \delta Z^{(n)}_\lambda \sim\delta b_\mathrm{eff}^{(n)}\left(\frac{\lambda}{w^{(n)}}\right)^\gamma \sim v_{\mathrm{A}y}\tilde S_{\mathrm{c}}^{1/4}\frac{\left[w^{(n)}\right]^{(1 - 2\gamma)/2}\lambda^\gamma}{\ell^{1/2}}, \qquad\lambda < w^{(n)}, \end{equation}
where, e.g., ![]() $\gamma =1/4$ for an aligned MHD cascade (§ 6.4) and
$\gamma =1/4$ for an aligned MHD cascade (§ 6.4) and ![]() $\gamma =3/5$ for a tearing-mediated one (§ 7.2.1). The cumulative energy density at scale
$\gamma =3/5$ for a tearing-mediated one (§ 7.2.1). The cumulative energy density at scale ![]() $\lambda$ from all the mini-cascades is then
$\lambda$ from all the mini-cascades is then
 \begin{equation} \delta Z_\lambda^2 \sim \sum_{w^{(n)}>\lambda} \bigl[\delta Z^{(n)}_\lambda\bigr]^2 \sim \frac{v_{\mathrm{A}y}^2\lambda^{2\gamma}}{\ell\tilde S_{\mathrm{c}}^{{-}1/2}} \int_{\max\{\lambda,\,\delta_{\mathrm{c}}\}}^{w^{(1)}}\!\!\!\!\!\!\!\!\!\!\!\! \mathrm{d} N(w)\,w^{1-2\gamma} \sim v_{\mathrm{A}y}^2\min\left\{1,\left(\frac{\lambda}{\delta_{\mathrm{c}}}\right)^{2\gamma}\right\}, \end{equation}
\begin{equation} \delta Z_\lambda^2 \sim \sum_{w^{(n)}>\lambda} \bigl[\delta Z^{(n)}_\lambda\bigr]^2 \sim \frac{v_{\mathrm{A}y}^2\lambda^{2\gamma}}{\ell\tilde S_{\mathrm{c}}^{{-}1/2}} \int_{\max\{\lambda,\,\delta_{\mathrm{c}}\}}^{w^{(1)}}\!\!\!\!\!\!\!\!\!\!\!\! \mathrm{d} N(w)\,w^{1-2\gamma} \sim v_{\mathrm{A}y}^2\min\left\{1,\left(\frac{\lambda}{\delta_{\mathrm{c}}}\right)^{2\gamma}\right\}, \end{equation}
where ![]() $N(w)$ is the plasmoid-width distribution (D86). The upper bound of the integral is the width
$N(w)$ is the plasmoid-width distribution (D86). The upper bound of the integral is the width ![]() $w^{(1)}\sim \ell \tilde S_{\mathrm {c}}^{-1/2}$ of the largest plasmoids, and the lower bound is the larger of the scale
$w^{(1)}\sim \ell \tilde S_{\mathrm {c}}^{-1/2}$ of the largest plasmoids, and the lower bound is the larger of the scale ![]() $\lambda$ and the width
$\lambda$ and the width ![]() $\delta _{\mathrm {c}}\sim \ell _{\mathrm {c}}\tilde S_{\mathrm {c}}^{-1/2}$ of the smallest plasmoids, i.e., ones associated with the critical SP sheet (D80). This result means that there is a
$\delta _{\mathrm {c}}\sim \ell _{\mathrm {c}}\tilde S_{\mathrm {c}}^{-1/2}$ of the smallest plasmoids, i.e., ones associated with the critical SP sheet (D80). This result means that there is a ![]() $k_{\perp }^{-1}$ spectrum in the reconnection-driving range
$k_{\perp }^{-1}$ spectrum in the reconnection-driving range ![]() $k_{\perp }\delta _{\mathrm {c}}\lesssim 1$ followed by an MHD turbulence spectrum
$k_{\perp }\delta _{\mathrm {c}}\lesssim 1$ followed by an MHD turbulence spectrum ![]() $\propto k_{\perp }^{-2\gamma -1}$ at
$\propto k_{\perp }^{-2\gamma -1}$ at ![]() $k_{\perp }\delta _{\mathrm {c}} \gtrsim 1$.
$k_{\perp }\delta _{\mathrm {c}} \gtrsim 1$.

Figure 50. A 3D, turbulent plasmoid (flux-rope) chain obtained in the simulations of Huang & Bhattacharjee (Reference Huang and Bhattacharjee2016) (© AAS, reproduced with permission).
What this spectrum is depends on whether the cascade is ideal/aligned or tearing-dominated. In order to work that out with confidence, one has to know rather more than we (or, at least, I) currently do about the turbulent dynamics of flux ropes. Recall, however, that the structures in the driving range are already aligned with angle ![]() $\sim \tilde S_{\mathrm {c}}^{-1/2}$. It is, therefore, possible, and indeed plausible, that the MHD cascade seeded by unstable flux ropes might be tearing-mediated, as mooted in § 7.4.3.
$\sim \tilde S_{\mathrm {c}}^{-1/2}$. It is, therefore, possible, and indeed plausible, that the MHD cascade seeded by unstable flux ropes might be tearing-mediated, as mooted in § 7.4.3.
To conclude, under the simplistic scheme explored above, reconnection-driven turbulence at small enough scales appears to be some form of regular MHD turbulence, but with a broad scale range into which energy is injected directly by reconnection processes – broad asymptotically but certainly not captured in full asymptotic glory by any realisable numerical experiment. This might explain a degree of discord in the scalings reported in the papers cited in § 7.4.3.
D.7. Stochastic reconnection and MHD turbulence
As promised in § 8.3.3, here is a (biased) review of ‘stochastic reconnection’, the notion primarily associated today with Lazarian & Vishniac (Reference Lazarian and Vishniac1999, henceforth LV99) – a widely cited paper, which, however, has acquired the reputation of being rather hard to understand. Eyink et al. (Reference Eyink, Lazarian and Vishniac2011) seem to me to have succeeded in explaining it with a degree of clarity by adopting somewhat different terms, based on a sizeable body of precursor work by Eyink (Reference Eyink2009, Reference Eyink2011). There are many self-reviews of this school of thought, of which the most recent and comprehensive one is Lazarian et al. (Reference Lazarian, Eyink, Jafari, Kowal, Li, Xu and Vishniac2020), but my attempt below may be the first by an external observer. Their main idea is roughly as follows.
First, let us note that, instead of (D47), we may follow Kulsrud (Reference Kulsrud2005) and start our consideration of an SP sheet by stating that the width of the sheet must be equal to the typical distance that the field lines would diffuse resistively in the direction (![]() $x$) transverse to the upstream field (which points in
$x$) transverse to the upstream field (which points in ![]() $y$) over the time that it takes the plasma to transit through the sheet and be ejected out of it, viz.,
$y$) over the time that it takes the plasma to transit through the sheet and be ejected out of it, viz.,
 \begin{equation} \delta \sim (\eta t_{\mathrm{out}})^{1/2},\quad t_{\mathrm{out}} \sim \frac{\ell}{u_y}\quad\Rightarrow\quad \delta \sim \left(\frac{\eta\ell}{u_y}\right)^{1/2} \sim \frac{\ell}{\sqrt{S_\ell}}\left(\frac{v_{\mathrm{A}y}}{u_y}\right)^{1/2}, \end{equation}
\begin{equation} \delta \sim (\eta t_{\mathrm{out}})^{1/2},\quad t_{\mathrm{out}} \sim \frac{\ell}{u_y}\quad\Rightarrow\quad \delta \sim \left(\frac{\eta\ell}{u_y}\right)^{1/2} \sim \frac{\ell}{\sqrt{S_\ell}}\left(\frac{v_{\mathrm{A}y}}{u_y}\right)^{1/2}, \end{equation}which is the same expression as (D48).
LV99, as interpreted by Eyink et al. (Reference Eyink, Lazarian and Vishniac2011),Footnote 106 argue that if the sheet is embedded in a turbulent environment, ![]() $\delta$ should instead be calculated as the distance by which two magnetic field lines initially starting arbitrarily close-by, separate after time
$\delta$ should instead be calculated as the distance by which two magnetic field lines initially starting arbitrarily close-by, separate after time ![]() $t_{\mathrm {out}}$, and that this distance is the same as the distance by which two Lagrangian fluid particles separate. It is this identification between stochastic particle trajectories and field lines that requires all the work contained in Eyink (Reference Eyink2009, Reference Eyink2011). In formal terms, he is able to prove that, in the presence of resistivity, the magnetic field at any point in space and time is an average over those realisations of a certain random field that end up at that point after evolving as ‘virtual’ magnetic fields ‘frozen’ into a stochastic flow that is the superposition of the Lagrangian turbulent velocity field and a white noise with the diffusion constant
$t_{\mathrm {out}}$, and that this distance is the same as the distance by which two Lagrangian fluid particles separate. It is this identification between stochastic particle trajectories and field lines that requires all the work contained in Eyink (Reference Eyink2009, Reference Eyink2011). In formal terms, he is able to prove that, in the presence of resistivity, the magnetic field at any point in space and time is an average over those realisations of a certain random field that end up at that point after evolving as ‘virtual’ magnetic fields ‘frozen’ into a stochastic flow that is the superposition of the Lagrangian turbulent velocity field and a white noise with the diffusion constant ![]() $\eta$. However small is
$\eta$. However small is ![]() $\eta$, such fields diverge in the same way as Lagrangian trajectories do. Eyink et al. (Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013) successfully tested this proposition in a large numerical simulation of MHD turbulence.Footnote 107
$\eta$, such fields diverge in the same way as Lagrangian trajectories do. Eyink et al. (Reference Eyink, Vishniac, Lalescu, Aluie, Kanov, Bürger, Burns, Meneveau and Szalay2013) successfully tested this proposition in a large numerical simulation of MHD turbulence.Footnote 107
In fluid dynamics, the stochastic separation of Lagrangian trajectories is known as Richardson diffusion: one argues, with Richardson (Reference Richardson1926), that the rate of change of the typical square distance ![]() $\Delta r^2$ between them is the turbulent diffusivity associated with velocities at the scale
$\Delta r^2$ between them is the turbulent diffusivity associated with velocities at the scale ![]() $\Delta r$:
$\Delta r$:
The last two steps follow from ![]() $\delta u_{\Delta r}^2/\tau _{\mathrm {c}} \sim \varepsilon$ and
$\delta u_{\Delta r}^2/\tau _{\mathrm {c}} \sim \varepsilon$ and ![]() $\delta u_{\Delta r}\sim (\varepsilon \Delta r)^{1/3}$. The latter relation might appear to be valid only for standard K41 or GS95 turbulence (2.9). In fact, it is also valid for the aligned turbulence because the velocities in (D98) must be in the direction of the particle separation
$\delta u_{\Delta r}\sim (\varepsilon \Delta r)^{1/3}$. The latter relation might appear to be valid only for standard K41 or GS95 turbulence (2.9). In fact, it is also valid for the aligned turbulence because the velocities in (D98) must be in the direction of the particle separation ![]() $\Delta r$, so we must use the scaling of
$\Delta r$, so we must use the scaling of ![]() $\delta u$ with
$\delta u$ with ![]() $\xi$, not with
$\xi$, not with ![]() $\lambda$ – and that is always the Kolmogorov scaling, including for the aligned cascade (see (6.31)) and even for the tearing-mediated one (see § 7.2.3). Integrating (D98) gets us ‘superdiffusion’:
$\lambda$ – and that is always the Kolmogorov scaling, including for the aligned cascade (see (6.31)) and even for the tearing-mediated one (see § 7.2.3). Integrating (D98) gets us ‘superdiffusion’:
as long as ![]() $\Delta r(t)<\lambda _{\mathrm {CB}}$ (the outer scale of the strong turbulence), or, equivalently, as long as
$\Delta r(t)<\lambda _{\mathrm {CB}}$ (the outer scale of the strong turbulence), or, equivalently, as long as ![]() $t$ is shorter than the nonlinear time
$t$ is shorter than the nonlinear time ![]() $\tau _{\mathrm {nl}}$ at scale
$\tau _{\mathrm {nl}}$ at scale ![]() $\lambda _{\mathrm {CB}}$. A salient feature here is that
$\lambda _{\mathrm {CB}}$. A salient feature here is that ![]() $\Delta r(t)$ is independent of the initial separation, which can be arbitrarily small. Therefore, the width of the reconnecting sheet and the inflow speed are
$\Delta r(t)$ is independent of the initial separation, which can be arbitrarily small. Therefore, the width of the reconnecting sheet and the inflow speed are
 \begin{equation} \delta \sim \Delta r(t_{\mathrm{out}}) \sim \varepsilon^{1/2}\left(\frac{\ell}{u_y}\right)^{3/2}\quad\Rightarrow\quad u_x \sim \frac{\delta}{\ell}\,u_y\sim\left(\frac{\varepsilon\ell}{u_y}\right)^{1/2}.\end{equation}
\begin{equation} \delta \sim \Delta r(t_{\mathrm{out}}) \sim \varepsilon^{1/2}\left(\frac{\ell}{u_y}\right)^{3/2}\quad\Rightarrow\quad u_x \sim \frac{\delta}{\ell}\,u_y\sim\left(\frac{\varepsilon\ell}{u_y}\right)^{1/2}.\end{equation}This result is all I need to work out what LV99 means for the theories of turbulent cascade presented in the main text. I will do this in Appendices D.7.3 and D.7.4, to which a reader only interested in the effect of reconnection on turbulence can safely skip. Appendices D.7.1 and D.7.2 are for those who are also interested in the effect of turbulence on reconnection,Footnote 108 and in the status of the numerical evidence on the subject.
D.7.1. Stochastic reconnection mediated by strong turbulence
LV99 formulated their prediction for the reconnection rate not in terms of its scaling with ![]() $\varepsilon$, as in (D100), but with the Alfvénic Mach number, which, for the purposes of this exposition, I will define as
$\varepsilon$, as in (D100), but with the Alfvénic Mach number, which, for the purposes of this exposition, I will define as
where ![]() $v_{\mathrm {A}y}$ is the in-plane Alfvén speed associated with the upstream (reconnecting) magnetic field and
$v_{\mathrm {A}y}$ is the in-plane Alfvén speed associated with the upstream (reconnecting) magnetic field and ![]() $\delta u_{L_{\perp }}$ is the turbulent velocity field at the outer scale
$\delta u_{L_{\perp }}$ is the turbulent velocity field at the outer scale ![]() $L_{\perp }$. How to express the prediction (D100) in terms of
$L_{\perp }$. How to express the prediction (D100) in terms of ![]() $M_{\mathrm {A}}$ depends on how the turbulence is driven.
$M_{\mathrm {A}}$ depends on how the turbulence is driven.
In LV99, it is driven weakly, so, using the standard WT scaling (4.7), they have
 \begin{equation} \delta u_{L_{{\perp}}} \sim \left(\frac{\varepsilon v_{\mathrm{A}}}{L_{{\parallel}}}\right)^{1/4}L_{{\perp}}^{1/2} \quad\Rightarrow\quad \varepsilon \sim \frac{\delta u_{L_{{\perp}}}^4L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}^2} = M_{\mathrm{A}}^4 \frac{v_{\mathrm{A}y}^4L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}^2}. \end{equation}
\begin{equation} \delta u_{L_{{\perp}}} \sim \left(\frac{\varepsilon v_{\mathrm{A}}}{L_{{\parallel}}}\right)^{1/4}L_{{\perp}}^{1/2} \quad\Rightarrow\quad \varepsilon \sim \frac{\delta u_{L_{{\perp}}}^4L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}^2} = M_{\mathrm{A}}^4 \frac{v_{\mathrm{A}y}^4L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}^2}. \end{equation}
In their model, in fact, the driving is isotropic and the mean field is of the same order as the in-plane field: ![]() $L_{\parallel } \sim L_{\perp } \equiv L$ and
$L_{\parallel } \sim L_{\perp } \equiv L$ and ![]() $v_{\mathrm {A}y}\sim v _{\mathrm {A}}$ (but
$v_{\mathrm {A}y}\sim v _{\mathrm {A}}$ (but ![]() $M_{\mathrm {A}}\ll 1$, so the turbulence is indeed weak). This is outside the RMHD limit, but it is probably fine to extrapolate ‘twiddle’ scalings to this regime:
$M_{\mathrm {A}}\ll 1$, so the turbulence is indeed weak). This is outside the RMHD limit, but it is probably fine to extrapolate ‘twiddle’ scalings to this regime: ![]() $\varepsilon \sim M_{\mathrm {A}}^4v_{\mathrm {A}}^3/L$. In what follows, I shall keep the anisotropic scaling (D102) but show how the main results simplify in the isotropic case and reduce to LV99.
$\varepsilon \sim M_{\mathrm {A}}^4v_{\mathrm {A}}^3/L$. In what follows, I shall keep the anisotropic scaling (D102) but show how the main results simplify in the isotropic case and reduce to LV99.
There are, obviously, two distinct possibilities: when ![]() $L_{\perp }$ is much larger and much smaller than the sheet width
$L_{\perp }$ is much larger and much smaller than the sheet width ![]() $\delta$. I shall deal with the latter in Appendix D.7.2. In the former regime, the formula (D100) applies if
$\delta$. I shall deal with the latter in Appendix D.7.2. In the former regime, the formula (D100) applies if ![]() $\delta$ is small enough that turbulence is already in the strong regime at that scale, viz., if
$\delta$ is small enough that turbulence is already in the strong regime at that scale, viz., if
 \begin{equation} L_{{\perp}} \gg \lambda_{\mathrm{CB}}=\varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2} \gtrsim \delta, \end{equation}
\begin{equation} L_{{\perp}} \gg \lambda_{\mathrm{CB}}=\varepsilon^{1/2}\left(\frac{L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{3/2} \gtrsim \delta, \end{equation}
where the CB scale ![]() $\lambda _{\mathrm {CB}}$ is given by (4.3). Using (D100), we find that the second inequality in (D103) is equivalent to
$\lambda _{\mathrm {CB}}$ is given by (4.3). Using (D100), we find that the second inequality in (D103) is equivalent to
 \begin{equation} \frac{\delta}{\lambda_{\mathrm{CB}}} \sim \left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{{3/2}} \lesssim 1. \end{equation}
\begin{equation} \frac{\delta}{\lambda_{\mathrm{CB}}} \sim \left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{{3/2}} \lesssim 1. \end{equation}
If this is true, then, even though turbulence is driven weakly at scale ![]() $L_{\perp }$, stochastic reconnection is mediated by strong turbulence at scale
$L_{\perp }$, stochastic reconnection is mediated by strong turbulence at scale ![]() $\delta$. Assuming (D104) to be true and using (D100) again, one gets the reconnection rate
$\delta$. Assuming (D104) to be true and using (D100) again, one gets the reconnection rate
 \begin{equation} \frac{u_x}{v_{\mathrm{A}y}} \sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2} \sim M_{\mathrm{A}}^2\left(\frac{\ell}{L}\right)^{1/2}, \end{equation}
\begin{equation} \frac{u_x}{v_{\mathrm{A}y}} \sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2} \sim M_{\mathrm{A}}^2\left(\frac{\ell}{L}\right)^{1/2}, \end{equation}
the last expression having been obtained for the case of isotropic driving and taking also the outflow to be Alfvénic, ![]() $u_y\sim v _{\mathrm {A}y}$.
$u_y\sim v _{\mathrm {A}y}$.
This is the well-known LV99 prediction ![]() $u_x\propto M _{\mathrm {A}}^2$, which, to numericists, has all the attraction of a testable result. In a recent study by Yang et al. (Reference Yang, Li, Guo, Li, Li, He, Zhang and Feng2020), they tried to test it and found instead
$u_x\propto M _{\mathrm {A}}^2$, which, to numericists, has all the attraction of a testable result. In a recent study by Yang et al. (Reference Yang, Li, Guo, Li, Li, He, Zhang and Feng2020), they tried to test it and found instead ![]() $u_x\propto M _{\mathrm {A}}$, causing some concern about the validity of LV99. In fact, while their result disagrees with (D105), it seems to me to be perfectly consistent with (D100) if one can assume that motions comparable in size to the box-wide rms velocity exist on the scale of the sheet width – either because the sheet generates its own turbulence (as it does in such simulations when they are not externally driven: see § 7.4.3 and references therein), or because it is able locally to chop down the driven energy-containing motions to its own scale (Yang et al. Reference Yang, Li, Guo, Li, Li, He, Zhang and Feng2020 did force their turbulence externally). Namely, let us assume that, effectively, for the sheet,
$u_x\propto M _{\mathrm {A}}$, causing some concern about the validity of LV99. In fact, while their result disagrees with (D105), it seems to me to be perfectly consistent with (D100) if one can assume that motions comparable in size to the box-wide rms velocity exist on the scale of the sheet width – either because the sheet generates its own turbulence (as it does in such simulations when they are not externally driven: see § 7.4.3 and references therein), or because it is able locally to chop down the driven energy-containing motions to its own scale (Yang et al. Reference Yang, Li, Guo, Li, Li, He, Zhang and Feng2020 did force their turbulence externally). Namely, let us assume that, effectively, for the sheet,
The latter relationship is a kind of CB: the time scale for the incoming matter and flux to be taken out of the sheet is the same as for an Alfvén wave to travel the correlation length along the guide field. Using ![]() $L_{\perp }\sim \lambda _{\mathrm {CB}}$ in the strong-turbulence scaling (6.22), we get
$L_{\perp }\sim \lambda _{\mathrm {CB}}$ in the strong-turbulence scaling (6.22), we get
 \begin{equation} \delta u_{L_{{\perp}}}\sim\left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\quad\Rightarrow\quad \varepsilon \sim \frac{\delta u_{L_{{\perp}}}^2v_{\mathrm{A}}}{L_{{\parallel}}} \sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}} v_{\mathrm{A}y}^2}{L_{{\parallel}}}. \end{equation}
\begin{equation} \delta u_{L_{{\perp}}}\sim\left(\frac{\varepsilon L_{{\parallel}}}{v_{\mathrm{A}}}\right)^{1/2}\quad\Rightarrow\quad \varepsilon \sim \frac{\delta u_{L_{{\perp}}}^2v_{\mathrm{A}}}{L_{{\parallel}}} \sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}} v_{\mathrm{A}y}^2}{L_{{\parallel}}}. \end{equation}The formula (D100) now gives
 \begin{equation} \frac{u_x}{v_{\mathrm{A}y}} \sim M_{\mathrm{A}}\left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2} \sim M_{\mathrm{A}}, \end{equation}
\begin{equation} \frac{u_x}{v_{\mathrm{A}y}} \sim M_{\mathrm{A}}\left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2} \sim M_{\mathrm{A}}, \end{equation}
the last expression following from (D106). This result is almost trivial: ![]() $u_x\sim \delta u_{L_{\perp }}$, so the sheet sucks in flux at the typical velocity of turbulent motions inside it.
$u_x\sim \delta u_{L_{\perp }}$, so the sheet sucks in flux at the typical velocity of turbulent motions inside it.
D.7.2. Stochastic reconnection mediated by weak turbulence
Historically the first suite of confirmatory numerics for the LV99 theory was reported by Kowal et al. (Reference Kowal, Lazarian, Vishniac and Otmianowska-Mazur2009, Reference Kowal, Lazarian, Vishniac and Otmianowska-Mazur2012) (usefully summarised by Lazarian et al. Reference Lazarian, Eyink, Vishniac and Kowal2015), who, in their simulations of a macroscopic magnetic field reconnecting in the presence of externally injected turbulence, did indeed see the scaling ![]() $u_x\propto \varepsilon ^{1/2}$, independent of
$u_x\propto \varepsilon ^{1/2}$, independent of ![]() $\eta$ (figure 51). Most of their simulations, however, seem to be in the second regime considered by LV99, one in which
$\eta$ (figure 51). Most of their simulations, however, seem to be in the second regime considered by LV99, one in which ![]() $L_{\perp } \lesssim \delta$.
$L_{\perp } \lesssim \delta$.

Figure 51. Simulations of stochastic reconnection by Kowal et al. (Reference Kowal, Lazarian, Vishniac and Otmianowska-Mazur2009): (a) arrows are magnetic fields, colour shows (turbulent) currents; (b) reconnection rate ![]() $V_\mathrm {rec}$ vs. injected power
$V_\mathrm {rec}$ vs. injected power ![]() $P_\mathrm {inj}$, which, in my notation, are
$P_\mathrm {inj}$, which, in my notation, are ![]() $u_x$ and
$u_x$ and ![]() $\varepsilon$, respectively – this plot, taken from Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015), shows
$\varepsilon$, respectively – this plot, taken from Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015), shows ![]() $u_x\propto \varepsilon ^{1/2}$, in accordance with (D111).
$u_x\propto \varepsilon ^{1/2}$, in accordance with (D111).
Since the turbulence that they are injecting into the sheet is still weak, and its scale is smaller than the width of the sheet, the expression for the turbulent diffusion coefficient used in (D98) no longer applies (unless the sheet manages to generate its own turbulence, which, in their simulations, it does not appear to do – perhaps because it is quite short?). Instead, we have
where ![]() $\omega _{\mathrm {A}}\sim v _{\mathrm {A}}/L_{\parallel }$ is the Alfvén frequency and
$\omega _{\mathrm {A}}\sim v _{\mathrm {A}}/L_{\parallel }$ is the Alfvén frequency and ![]() $\tau _{\mathrm {c}}$ is the correlation time of WT (it is given by (4.5), but we do not need to know this to get to the last expression). An easy way to understand why these two characteristic time scales appear in (D109) in the way they do is as follows. The turbulent diffusion coefficient is just the time integral of the two-time correlation function of the velocity field, and we can calculate it by assuming a simple functional form for this correlation function characteristic of a slowly decorrelating but fast-oscillating wave field:
$\tau _{\mathrm {c}}$ is the correlation time of WT (it is given by (4.5), but we do not need to know this to get to the last expression). An easy way to understand why these two characteristic time scales appear in (D109) in the way they do is as follows. The turbulent diffusion coefficient is just the time integral of the two-time correlation function of the velocity field, and we can calculate it by assuming a simple functional form for this correlation function characteristic of a slowly decorrelating but fast-oscillating wave field:
In the absence of waves, or in CB (![]() $\omega _{\mathrm {A}}\sim \tau _{\mathrm {c}}^{-1}$), one recovers the usual formula
$\omega _{\mathrm {A}}\sim \tau _{\mathrm {c}}^{-1}$), one recovers the usual formula ![]() $D\sim \delta u_{L_{\perp }}^2\tau _{\mathrm {c}}$, analogous to (D98), whereas for
$D\sim \delta u_{L_{\perp }}^2\tau _{\mathrm {c}}$, analogous to (D98), whereas for ![]() $\omega _{\mathrm {A}}\gg \tau _{\mathrm {c}}^{-1}$, one gets (D109).
$\omega _{\mathrm {A}}\gg \tau _{\mathrm {c}}^{-1}$, one gets (D109).
Thus, adopting (D109), we get, in the same way as we did in (D100),
 \begin{equation} \Delta r(t) \sim \frac{(\varepsilon t)^{1/2}}{\omega_{\mathrm{A}}}\quad\Rightarrow\quad \delta \sim \left(\frac{\varepsilon\ell}{u_y}\right)^{1/2}\frac{L_{{\parallel}}}{v_{\mathrm{A}}}, \qquad u_x \sim \biggl(\frac{\varepsilon u_y}{\ell}\biggr)^{1/2}\frac{L_{{\parallel}}}{v_{\mathrm{A}}}. \end{equation}
\begin{equation} \Delta r(t) \sim \frac{(\varepsilon t)^{1/2}}{\omega_{\mathrm{A}}}\quad\Rightarrow\quad \delta \sim \left(\frac{\varepsilon\ell}{u_y}\right)^{1/2}\frac{L_{{\parallel}}}{v_{\mathrm{A}}}, \qquad u_x \sim \biggl(\frac{\varepsilon u_y}{\ell}\biggr)^{1/2}\frac{L_{{\parallel}}}{v_{\mathrm{A}}}. \end{equation}
With the aid of (D102), the reconnection rate in terms of ![]() $M_{\mathrm {A}}$ becomes
$M_{\mathrm {A}}$ becomes
 \begin{equation} \frac{u_x}{v_{\mathrm{A}y}}\sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \left(\frac{L_{{\parallel}} u_y}{\ell v_{\mathrm{A}}}\right)^{1/2} \sim M_{\mathrm{A}}^2\left(\frac{L}{\ell}\right)^{1/2}, \end{equation}
\begin{equation} \frac{u_x}{v_{\mathrm{A}y}}\sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \left(\frac{L_{{\parallel}} u_y}{\ell v_{\mathrm{A}}}\right)^{1/2} \sim M_{\mathrm{A}}^2\left(\frac{L}{\ell}\right)^{1/2}, \end{equation}
the last expression being for an isotropic forcing and Alfvénic outflows, as in (D105). This prediction too appears in LV99, although derived by a slightly different route. Yet again, ![]() $u_x\propto \varepsilon ^{1/2} \propto M_{\mathrm {A}}^2$, which is what Kowal et al. (Reference Kowal, Lazarian, Vishniac and Otmianowska-Mazur2009) confirmed (figure 51).
$u_x\propto \varepsilon ^{1/2} \propto M_{\mathrm {A}}^2$, which is what Kowal et al. (Reference Kowal, Lazarian, Vishniac and Otmianowska-Mazur2009) confirmed (figure 51).
Note that in (D109), the ![]() $L_{\perp }$ dependence disappeared from the expression for turbulent diffusivity. This means that actually the above calculation remains valid also for
$L_{\perp }$ dependence disappeared from the expression for turbulent diffusivity. This means that actually the above calculation remains valid also for ![]() $L_{\perp } \gg \delta$ – as long as
$L_{\perp } \gg \delta$ – as long as ![]() $\delta \gg \lambda _{\mathrm {CB}}$, i.e., as long as the turbulence is weak at the scale
$\delta \gg \lambda _{\mathrm {CB}}$, i.e., as long as the turbulence is weak at the scale ![]() $\delta$. In this case, one must replace
$\delta$. In this case, one must replace ![]() $L_{\perp }$ with
$L_{\perp }$ with ![]() $\Delta r(t)$ in (D109), but the result is the same because, as we have just seen,
$\Delta r(t)$ in (D109), but the result is the same because, as we have just seen, ![]() $D(\Delta r)$ is independent of
$D(\Delta r)$ is independent of ![]() $\Delta r$ for WT – the divergence of Lagrangian trajectories in WT is always just diffusive, Richardson superdiffusion (D99) being a distinctive property of strong turbulence. Therefore, (D112) only breaks down and transitions into (D105) when
$\Delta r$ for WT – the divergence of Lagrangian trajectories in WT is always just diffusive, Richardson superdiffusion (D99) being a distinctive property of strong turbulence. Therefore, (D112) only breaks down and transitions into (D105) when ![]() $\lambda _{\mathrm {CB}}$ becomes comparable to
$\lambda _{\mathrm {CB}}$ becomes comparable to ![]() $\delta$, i.e., when (D104) becomes true. In summary,
$\delta$, i.e., when (D104) becomes true. In summary,
 \begin{equation} \frac{u_x}{v_{\mathrm{A}y}}\sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \min\left\{\left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2}, \left(\frac{L_{{\parallel}} u_y}{\ell v_{\mathrm{A}}}\right)^{1/2}\right\}, \end{equation}
\begin{equation} \frac{u_x}{v_{\mathrm{A}y}}\sim M_{\mathrm{A}}^2\frac{v_{\mathrm{A}y} L_{{\parallel}}}{v_{\mathrm{A}} L_{{\perp}}} \min\left\{\left(\frac{\ell v_{\mathrm{A}}}{L_{{\parallel}} u_y}\right)^{1/2}, \left(\frac{L_{{\parallel}} u_y}{\ell v_{\mathrm{A}}}\right)^{1/2}\right\}, \end{equation}the LV99 magic formula for the rate of stochastic reconnection, generalised to the case of anisotropic driving.
D.7.3. Stochastic reconnection in GS95 turbulence
Let us now apply (D100) to a typical turbulent structure in which ![]() $\ell =\xi$ and
$\ell =\xi$ and ![]() $u_y\sim \delta u_\xi \sim (\varepsilon \xi )^{1/3}$. This instantly implies
$u_y\sim \delta u_\xi \sim (\varepsilon \xi )^{1/3}$. This instantly implies
For GS95 turbulence (![]() $\xi \sim \lambda$), this means that reconnection of field lines within each ‘eddy’ occurs on the same time scale as the ‘eddy’ turns over – this is, I think, what Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015) mean when they say that stochastic reconnection makes GS95 turbulence ‘self-consistent’.
$\xi \sim \lambda$), this means that reconnection of field lines within each ‘eddy’ occurs on the same time scale as the ‘eddy’ turns over – this is, I think, what Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015) mean when they say that stochastic reconnection makes GS95 turbulence ‘self-consistent’.
D.7.4. Stochastic reconnection in aligned turbulence
What if the turbulence is aligned? According to my argument above, in view of (6.31), it might seem that (D114) remains valid. This is worrisome: indeed this tells us that the width of the layer over which stochastic reconnection would be happening is larger than the width of the aligned structure: ![]() $\delta \sim \xi \gg \lambda$! If this were true, writing this review would have been a waste of time: aligned structures would be quickly broken up by stochastic reconnection, so there would be no aligned cascade. This would invalidate all of § 6 and, consequently, obviate any consideration of tearing-mediated turbulence in § 7 – the cascade would just be GS95 all the way to the dissipation scale. Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015) (and their previous papers referenced therein) might then be excused for (politely) ignoring all the newfangled turbulence theory postdating GS95, and Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019) would be vindicated much more completely than I could offer to do in § 7.2.2. I cannot prove formally that this does not or cannot happen (cf. § 8.2), but I can show that aligned turbulence is, in fact, not ruled out by stochastic reconnection.
$\delta \sim \xi \gg \lambda$! If this were true, writing this review would have been a waste of time: aligned structures would be quickly broken up by stochastic reconnection, so there would be no aligned cascade. This would invalidate all of § 6 and, consequently, obviate any consideration of tearing-mediated turbulence in § 7 – the cascade would just be GS95 all the way to the dissipation scale. Lazarian et al. (Reference Lazarian, Eyink, Vishniac and Kowal2015) (and their previous papers referenced therein) might then be excused for (politely) ignoring all the newfangled turbulence theory postdating GS95, and Beresnyak (Reference Beresnyak2011, Reference Beresnyak2012a, Reference Beresnyak2014b, Reference Beresnyak2019) would be vindicated much more completely than I could offer to do in § 7.2.2. I cannot prove formally that this does not or cannot happen (cf. § 8.2), but I can show that aligned turbulence is, in fact, not ruled out by stochastic reconnection.
Let us imagine that an aligned structure has emerged with transverse (to fluctuating fields) scale ![]() $\lambda$ and longitudinal (fluctuation-direction) scale
$\lambda$ and longitudinal (fluctuation-direction) scale ![]() $\xi \gg \lambda$. In order for stochastic reconnection to destroy it quickly, there must be turbulent structures within the layer of width
$\xi \gg \lambda$. In order for stochastic reconnection to destroy it quickly, there must be turbulent structures within the layer of width ![]() $\lambda$ whose longitudinal scales are as large as
$\lambda$ whose longitudinal scales are as large as ![]() $\sim \lambda$. But within this layer, there is an intense shear
$\sim \lambda$. But within this layer, there is an intense shear ![]() $\sim \delta u_\lambda /\lambda$, which should suppress any turbulent motions whose typical nonlinear time scales are longer than the inverse of this shear. For small enough structures, the nonlinear times will be short and eventually overcome the shear. Let us find the longitudinal scale
$\sim \delta u_\lambda /\lambda$, which should suppress any turbulent motions whose typical nonlinear time scales are longer than the inverse of this shear. For small enough structures, the nonlinear times will be short and eventually overcome the shear. Let us find the longitudinal scale ![]() $\xi '$ of the largest possible such motions: their nonlinear decorrelation rate is
$\xi '$ of the largest possible such motions: their nonlinear decorrelation rate is
 \begin{equation} \frac{\delta u_{\xi'}}{\xi'} \sim \frac{\delta u_\lambda}{\lambda}\quad\Rightarrow\quad \frac{\xi'}{\lambda} \sim\left(\frac{\lambda}{\xi}\right)^{1/2} \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/8} \ll 1, \end{equation}
\begin{equation} \frac{\delta u_{\xi'}}{\xi'} \sim \frac{\delta u_\lambda}{\lambda}\quad\Rightarrow\quad \frac{\xi'}{\lambda} \sim\left(\frac{\lambda}{\xi}\right)^{1/2} \sim \left(\frac{\lambda}{\lambda_{\mathrm{CB}}}\right)^{1/8} \ll 1, \end{equation}
where I have used (6.31) for ![]() $\delta u_{\xi '}\sim (\varepsilon \xi ')^{1/3}$ and
$\delta u_{\xi '}\sim (\varepsilon \xi ')^{1/3}$ and ![]() $\delta u_\lambda =\delta u_\xi \sim (\varepsilon \xi )^{1/3}$.Footnote 109 If these motions are aligned in the usual way, with transverse scale
$\delta u_\lambda =\delta u_\xi \sim (\varepsilon \xi )^{1/3}$.Footnote 109 If these motions are aligned in the usual way, with transverse scale ![]() $\lambda '$, then, using (6.31) again,
$\lambda '$, then, using (6.31) again, ![]() $\lambda '/\lambda \sim (\lambda /\lambda _{\mathrm {CB}})^{1/2}$.
$\lambda '/\lambda \sim (\lambda /\lambda _{\mathrm {CB}})^{1/2}$.
Going back to (D98), one must now integrate this equation up to time ![]() $t_{\mathrm {out}}\sim \xi /\delta u_\xi \sim \varepsilon ^{-1/3}\xi ^{2/3}$, which is longer than the nonlinear time
$t_{\mathrm {out}}\sim \xi /\delta u_\xi \sim \varepsilon ^{-1/3}\xi ^{2/3}$, which is longer than the nonlinear time ![]() $\xi '/\delta u_{\xi '} \sim \varepsilon ^{-1/3}\xi '^{2/3}$ of the largest turbulent structures within our layer. This gives conventional turbulent diffusion:
$\xi '/\delta u_{\xi '} \sim \varepsilon ^{-1/3}\xi '^{2/3}$ of the largest turbulent structures within our layer. This gives conventional turbulent diffusion:
where the last step follows from (D115). Just like in (D114), the width of the stochastically reconnecting layer is the same as the width of the (now aligned) structure, so the magnetic fields in the aligned cascade reconnect just as fast as the turbulent structures decorrelate. Thus, the aligned cascade is consistent with stochastic reconnection.
The same is going to be true of the tearing-mediated cascade of § 7.2 because, in the argument leading to (D116), all I needed was the Kolmogorov scaling of the turbulent velocities in the fluctuation direction, which is always preserved (§ 7.2.3). The competition between the nonlinear decorrelation rate and the tearing rate that leads to disruption (§ 7.1) is unaffected by all this because disruption happens precisely at the scale where tearing becomes ‘ideal’. Any smaller-scale turbulence, ambient or created by the tearing, can presumably be viewed as providing seed perturbations for the instability.
The overall conclusion appears to be that stochastic reconnection, while a useful notion in the treatment of large-scale magnetic-field configurations with externally imposed turbulence, does not undermine (or modify) the existing theory of the aligned or tearing-mediated turbulence, but rather plays an interpretative role: it provides a further insight into the behaviour of tangled magnetic fields in a turbulent environment and reassures us that, whatever topological rearrangements are necessary for the cascade to proceed, they can always occur on the time scales of the cascade.