Hostname: page-component-745bb68f8f-f46jp Total loading time: 0 Render date: 2025-02-06T10:10:10.920Z Has data issue: false hasContentIssue false
  • English
  • Français

Generation of strong magnetic fields from laser interaction with two-layer targets

Published online by Cambridge University Press:  17 July 2009

S.Z. Wu ,
C.T. Zhou ,
X.T. He  and
S.-P. Zhu
S.Z. Wu
Affiliation:
Graduate School of the Chinese Academy of Engineering Physics, Beijing, People's Republic of China
C.T. Zhou*
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing, People's Republic of China Center for Applied Physics and Technology, Peking University, Beijing, People's Republic of China
X.T. He
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing, People's Republic of China Center for Applied Physics and Technology, Peking University, Beijing, People's Republic of China
S.-P. Zhu
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing, People's Republic of China
*
Address correspondence and reprint requests to: C.T. Zhou, Institute of Applied Physics and Computational Mathematics, P. O. Box 8009, Beijing 100088, People's Republic of China. E-mail: zcangtao@iapcm.ac.cn
Rights & Permissions [Opens in a new window]

Abstract

A two-layer target irradiated by an intense laser to generate strong interface magnetic field is proposed. The mechanism is analyzed through a simply physical model and investigated by two-dimensional particle-in-cell simulation. The effect of laser intensity on the resulting magnetic field strength is also studied. It is found that the magnetic field can reach up to several ten megagauss for laser intensity at 1019 Wcm−2.

Keywords

Electron beamsIntense laser-plasma interactionPIC simulationStrong magnetic field
Type
Research Article
Information
Laser and Particle Beams , Volume 27 , Issue 3 , September 2009 , pp. 471 - 474
Copyright
Copyright © Cambridge University Press 2009

1. INTRODUCTION

The self-generated magnetic field (Stamper et al., Reference Stamper, Papadopoulos, Sudan, Dean, McLean and Dawson1971; Sudan, Reference Sudan1993; Tatarakis et al., Reference Tatarakis, Watts, Beg, Dangor, Krushelnick, Wagner, Norreys and Clark2002; Wu et al., Reference Wu, Liu, Zhou and Zhu2009; Zhou & He, Reference Zhou and He2008a, Reference Zhou and He2008b) during laser-plasma interaction has been widely investigated theoretically and experimentally over the past decades, because of many potential applications (Ghoranneviss et al., Reference Ghoranneviss, Malekynia, Hora, Miley and He2008; Hora et al., Reference Hora, Lalousis and Eliezer1984, 2002, Reference Hora, Malekynia, Ghoranneviss, Miley and He2008b; Hora & Hoffmann, Reference Hora and Hoffmann2008a; Tan & Min, Reference Tan and Min1985; Zhou & He, Reference Zhou and He2007a, Reference Zhou, Yu and He2007b, Reference Zhou and He2007c), such as in fusion science, beam collimation, and electron acceleration, etc. When ultra-intense (>1018 Wcm−2 µm−2) lasers interact with overdense or solid targets, relativistic electrons are generated at the relativistic critical density. These fast electron currents will penetrate into the target and become a source of strong magnetic field, which can be up to 100 megagauss (MG) for laser intensities of 1020 Wcm−2.

Recently, there have been works on the generation and application of strong interface magnetic fields. With specially designed target structures, both hybrid-Vlasov-Fokker-Planck (HVFP) (Robinson et al., Reference Robinson and Sherlock2007) and hybrid-fluid-particle-in-cell (HFPIC) (Zhou et al., Reference Zhou and He2008a, Reference Zhou and He2008b) simulations show that strong interface magnetic fields are generated because of the gradients in the resistivity and density at the material interface. However, in realistic experiments and applications, the fast electrons are generated during the laser-plasma interaction (LPI). The earlier HVFP or HFPIC simulations cannot consider this process directly. Instead, in these simulations, the electron beams are injected and are assumed to satisfy certain scaling law (Wilks et al., Reference Wilks, Kruer, Tabak and Langdon1992). By making use of the explicit two-dimensional particle-in-cell (PIC) simulation and including the LPI directly, here we investigate the generation of strong interface magnetic fields in a two-layer target irradiated by ultra-intense lasers. The effect of the laser intensity on the field strength is also studied. It is found that the interface magnetic field can reach up to tens MG for laser intensity at 1019 Wcm−2, and even hundreds of MG for 1020 Wcm−2.

2. PHYSICAL MODEL

The self-generated magnetic field from the intense laser-produced fast electron flux is given by (Bell et al., Reference Bell, Davies and Guérin1998): ∂B/∂t = η∇ × jf + ∇η × jf, where jf is the fast electron current density, and η is the space-dependent resistivity. Since the standard explicit PIC simulation cannot include the effect of resistivity, the magnetic field is due to the density gradient alone.

We now consider the target shown in Figure 1. It consists of two plasma layers of different densities (layers 1 and 2 with densities n 1 and n 2, respectively). For laser-driven electron beam with currents larger than the Alfvén limit, a return current jr moving in the opposite direction will be induced to establish charge and current equilibrium. The local net current density is thus jnet ≡ jr + jf ~ µ0−1∇ × B, corresponding to IA ≈ 17.1 βγkA (Batani et al., Reference Batani2002) in a width on the order of 0.1 µm.

Fig. 1. A laser pulse incident on a two-layer target (layers 1 and 2 with plasma densities n 1 and n 2, respectively), and the self-generated interface magnetic field.

The net currents on the two sides of the interface are different due to the density difference, but as a whole, there is a current balance: j net(1) ~ − j net(2) (see Fig. 1). Therefore, a magnetic field B z = B z(1) + B z(2) will be generated near the interface according to Ampere's Law. We can write

(1)
B_z \sim \mu _0\, j_{\,\rm net}^{\lpar 1\rpar } L_1 + \mu_0\, j_{\rm net}^{\lpar 2\rpar } L_2\comma \; \eqno\lpar 1\rpar

where L i (i = 1, 2) is the transverse width of the current. The fast electron current j f can be estimated from the scaling law (Wilks et al., Reference Wilks, Kruer, Tabak and Langdon1992):

(2)
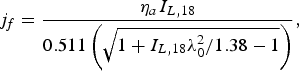
\,j_f = {\eta_a I_{L\comma 18} \over 0.511 \left( \sqrt{{1 + I_{L\comma 18} \lambda_0^2/1.38} - 1}\right) }\comma \; \eqno\lpar 2\rpar

where I L,18, λ0, j f represent the laser intensity, wavelength, and current density, in units of 1018 Wcm−2, µm, and 1012 Acm−2, respectively, and ηa is the laser absorption rate. For a typical intensity of I L = 1019 Wcm−2 and 30% absorption rate, the magnetic field strength can reach more than 30 MG according to Eqs. (1) and (2). It is also expected that the field strength will increase with increasing incident laser intensity.

3. SIMULATION RESULTS

Since we are mainly interested in the generation of the interface magnetic field by the density effect, the standard two-dimensional PIC technique is sufficient (Birdsall & Langdon, Reference Birdsall and Langdon1985). Our target configuration is shown in Figure 1. The two-dimensional (x, y) simulation box is 20 µm × 20 µm. The mesh resolution is 1200 × 1200 cells with 50 electrons and 50 ions in each cell. The initial temperature of the plasma electrons and ions is 1 keV. As shown in Figure 1, there are vacuum regions on the four sides of the target, 1 µm in the left and the right sides of the plasma (in the x direction), 2 µm in the front, and 1 μm behind the target (in the y direction). The periodic boundary condition is used in the x direction and the outgoing boundary condition in the y direction. The width of the inner layer (layer 1) is 4 μm in the x direction. The laser is incident from the bottom along the y direction with a rise time of about 4 fs, after which it remains at the constant peak intensity. The laser transverse profile (along the x direction) is super-gaussian with a 2.4 µm spot size. The laser wavelength is chosen to be λ = 1.05 µm.

We now consider two cases. The peak intensity of the incident laser is I L = 1019 Wcm−2 in case I, and 6 × 1019 Wcm−2 in case II. The target density profiles for the two cases are the same: n 1 = 20n c, n 2 = 50n c, where n c is the critical density.

Figures 2a and 2c show the net current density and the corresponding magnetic field at t = 160 fs for case I. It is clearly seen that the net currents on the two sides of the interface have opposite signs, and the values are up to the order of 1012 Acm−2. Such strong opposite currents generate a strong magnetic field along the interface (see Fig. 2c). In case I, the interface magnetic field can be more than 30 MG. The similar net currents and interface magnetic fields also appear in case II, as shown in Figures 2b and 2d. It is noted that the values for the current and magnetic field in case II are much larger than that in case I, as shown in Figure 2. According to Eqs. (1) and (2), higher laser intensity will generate higher current, and thus higher magnetic field.

Fig. 2. (Color online) The net current density in the y direction j net (in 1012 Acm−2) at 160 fs: (a) for case I and (b) case II. The corresponding magnetic fields B z (in MG): (c) case I and (d) case II.

In order to show the effect of laser intensity on the interface magnetic generation more quantitatively, several other cases have also been considered: 2 × 1019 Wcm−2, 4 × 1019 Wcm−2, 8 × 1019 Wcm−2, and 1020 Wcm−2. The other simulation parameters are identical to these in cases I and II. The strength of the resulting magnetic fields as a function of the incident laser intensity is shown in Figure 3. The line with the error bars is from the simulation, confirming that the field strength increases with the laser intensity. The analytical estimate based on Eqs. (1) and (2) is given in Figure 3 by the line with triangles. The good agreement demonstrates that the simple physical model is useful for estimating the interface magnetic field strength.

Fig. 3. (Color online) The interface magnetic field B z (in MG) against the incident laser intensity: simulation results (squares with the error bars) and physical estimate from Eqs. (1) and (2) (the line with triangles).

4. SUMMARY

To summarize, we have proposed a two-layer target for generating strong interface magnetic fields. The scheme is analyzed with a simple analytical model and the process is investigated using two-dimensional PIC simulation. It is found that the interface field can reach several tens MG at laser intensities of 1019 Wcm−2. Furthermore, we have also studied the effect of laser intensity on the magnetic field strength. Our simulation results agree very well with that from the analytical model. Thus, the proposed scheme as well as the physical model should be useful in the applications such as in fast electron collimation and high energy density physics, where strong magnetic fields are required.

ACKNOWLEDGMENTS

Wu would like to thank Z.J. Liu for his helpful discussions on PIC simulation. This work is supported by the National Natural Science Foundation of China Grant Nos. 10835003 and 10575013 and the National High-Tech ICF Committee and partially by the National Basic Research Program of China (973)(2007CB815101).

References

REFERENCES

Batani, D. (2002). Transport in dense matter of relativistic electrons produced in ultra-high-intensity laser interactions. Laser Part. Beams 20, 321–336.Google Scholar
Bell, A.R., Davies, J.R. & Guérin, S.M. (1998). Magnetic field in short-pulse high-intensity laser-solid experiments. Phys. Rev. E 58, 2471–2473.Google Scholar
Birdsall, C.K. & Langdon, A.B. (1985). Plasma Physics via Computer Simulation. New York: McGraw-Hill Inc.Google Scholar
Ghoranneviss, M., Malekynia, B., Hora, H., Miley, G.H. & He, X.T. (2008). Inhibition factor reduces fast ignition threshold of laser fusion using nonlinear force driven block ignition. Laser Part. Beams 26, 105–111.CrossRefGoogle Scholar
Hora, H., Lalousis, P. & Eliezer, S. (1984). Analysis of the inverted double-layers produced by nonlinear forces in laser-produced plasmas. Phys. Rev. Lett. 53, 1650–1652.Google Scholar
Hora, H., Hoelss, M., Scheid, W., Wang, J.X., HO, Y.K., Osman, F. & Castillo, R. (2000). Principle of high accuracy of the nonlinear theory for electron acceleration in vacuum by lasers at relativistic intensities. Laser Part. Beams 18, 135–144.Google Scholar
Hora, H. & Hoffmann, D.H.H. (2008 a). Using petawatt laser pulses of picosecond duration for detailed diagnostics of creation and decay processes of B-mesons in the LHC. Laser Part. Beams 26, 503–505.Google Scholar
Hora, H., Malekynia, B., Ghoranneviss, M., Miley, G.H. & He, X.T. (2008 b). Twenty times lower ignition threshold for laser driven fusion using collective effects and the inhibition factor. Appl. Phys. Lett. 93, 0111011-3.CrossRefGoogle Scholar
Robinson, A.P.L. & Sherlock, M. (2007). Magnetic collimation of fast electrons produced by ultraintense laser irradiation by structuring the target composition. Phys. Plasams 14, 083105.Google Scholar
Stamper, J.A., Papadopoulos, K., Sudan, R.N., Dean, S.O., McLean, E.A. & Dawson, J.M. (1971). Electron acceleration by high current-density relativistic electron bunch in plasmas. Laser Part. Beams 26, 1012–1015.Google Scholar
Sudan, R. (1993). Mechanism for the generation of 109 G magnetic fields in the interaction of ultraintense short laser pulse with an overdense plasma target. Phys. Rev. Lett. 70, 3075–3078.CrossRefGoogle ScholarPubMed
Tan, W. & Min, G. (1985). Thermal flux limitation and thermal conduction inhibition in laser plasmas. Laser Part. Beams 3, 243–250.Google Scholar
Tatarakis, M., Watts, I., Beg, F.N., Dangor, A.E., Krushelnick, K., Wagner, U., Norreys, P.A. & Clark, E.L. (2002). Measurements of ultrastrong magnetic fields during relativistic laseplasma interactions. Phys. Plasmas 9, 2244.CrossRefGoogle Scholar
Wilks, S.C., Kruer, W.L., Tabak, M. & Langdon, A.B. (1992). Absorption of ultra-intense laser pulses, Phys. Rev. Lett. 69, 1383–1386.Google Scholar
Wu, S.Z., Liu, Z.J., Zhou, C.T. & Zhu, S.P. (2009). Density effects on collimation of energetic electron beams driven by two intense laser pulses. Phys. Plasmas 16, 043106.Google Scholar
Yu, M.Y. & Luo, H.Q. (2008). A note on the multispecies model for identical particles. Phys. Plasmas 15, 024504.CrossRefGoogle Scholar
Zhou, C.T. & He, X.T. (2007 a). Influence of a large oblique incident angle on energetic protons accelerated from solid-density plasmas by ultraintense laser pulses. Appl. Phys. Lett. 90, 031503.CrossRefGoogle Scholar
Zhou, C.T., Yu, M.Y. & He, X.T. (2007 b). Electron acceleration by high current-density relativistic electron bunch in plasmas. Laser Part. Beams 25, 313–319.CrossRefGoogle Scholar
Zhou, C.T. & He, X.T. (2007 c). Intense laser-driven energetic proton beams from solid density targets. Opt. Lett. 32 2444–2446.Google Scholar
Zhou, C.T. & He, X.T. (2008 a). Intense-laser generated relativistic electron transport in coaxial two-layer targets., Appl. Phys. Lett. 92, 0715021-3.Google Scholar
Zhou, C.T. & He, X.T. (2008 b). Laser-produced energetic electron transport in overdense plasmas by wire guiding. Appl. Phys. Lett. 92, 1515021-3.Google Scholar
Figure 0

Fig. 1. A laser pulse incident on a two-layer target (layers 1 and 2 with plasma densities n1 and n2, respectively), and the self-generated interface magnetic field.

Figure 1

Fig. 2. (Color online) The net current density in the y direction jnet (in 1012 Acm−2) at 160 fs: (a) for case I and (b) case II. The corresponding magnetic fields Bz (in MG): (c) case I and (d) case II.

Figure 2

Fig. 3. (Color online) The interface magnetic field Bz (in MG) against the incident laser intensity: simulation results (squares with the error bars) and physical estimate from Eqs. (1) and (2) (the line with triangles).