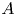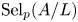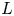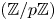1. Introduction
Let 
$p$ be a prime number and 
$K$ be a number field. There is an important result in algebraic number theory (see [Reference MadanMad72, Theorem 3]):
The 
$p$-torsion subgroup of the ideal class group of 
$L$ is unbounded as 
$L$ varies over 
$(\mathbb {Z}/p\mathbb {Z})$-extensions of 
$K$.
The first result of such a kind is due to Gauss, who proved the case 
$K=\mathbb {Q}$ and 
$p=2$. Since the growth problems for ideal class groups and for Selmer groups of abelian varieties are closely related (see [Reference ČesnavičiusČes15a]), it is naturally expected that similar results hold for 
$p$-Selmer groups of abelian varieties. Based on his generalization of the Cassels–Poitou–Tate sequence, Kęstutis Česnavičius successfully proved the following result.
Theorem 1.1 [Reference ČesnavičiusČes17, Theorem 1.2]
Let 
$p$ be a prime number, and 
$A$ be an abelian variety over a global field 
$K$. If either 
$A[p](\bar {K}) \neq 0$ or 
$A$ is supersingular, then
\[ \dim_{\mathbb{F}_{p}} \mathrm{Sel}_{p}(A/L) \]
is unbounded as 
$L$ varies over 
$( \mathbb {Z}/p\mathbb {Z} )$-extensions of 
$K$.
For the Tate–Shafarevich groups, when 
$L$ ranges over degree 
$p$ extensions of 
$K$, Clark and Sharif [Reference Clark and SharifCS10] proved that 
$\dim _{\mathbb {F}_{p}} \text{III} (A/L) [p]$ is unbounded in the case 
$\dim A=1$ and 
$p\neq \mathrm {char}\, K$; later Creutz [Reference CreutzCre11] showed the unboundedness of 
$\dim _{\mathbb {F}_{p}} \text{III} (A/L) [p]$ when 
$A$ is strongly principally polarized over a number field 
$K$ and the 
$G_{K}$-action on the Néron–Severi group of 
$A$ is trivial. Note that the extensions 
$L/K$ they constructed are not necessarily Galois. When fixing a 
$(\mathbb {Z}/n\mathbb {Z})$-extension 
$K/\mathbb {Q}$, Matsuno [Reference MatsunoMat09] proved that there exist elliptic curves 
$E/\mathbb {Q}$ with the 
$n$-rank of 
$\text{III} (E/K)[n]$ being arbitrarily large. Based on these results, Česnavičius proposed the following problem [Reference ČesnavičiusČes17, Problem 1.8].
Problem 1.2 Is 
$\dim _{\mathbb {F}_{p}} \text{III} (A/L) [p]$ unbounded as 
$L$ varies over 
$(\mathbb {Z}/p\mathbb {Z})$-extensions of 
$K$?
To attack this problem, one may try to generalize the methods of Clark and Sharif and of Creutz. However, we remark that these are based on the study of the period and index problem in 
$\text {H}^{1}(K,A)$, whose generalization to general abelian varieties seems rather difficult.
In this paper we shall present another idea to treat Problem 1.2, which is a combination of the machinery developed by Mazur and Rubin in [Reference Mazur and RubinMR18] and the method invented by Česnavičius in [Reference ČesnavičiusČes17]. Our main result is the following theorem.
Theorem 1.3 Let 
$A$ be an abelian variety over a global field 
$K$. If 
$p$ is a prime number not equal to 
$\mathrm {char}\, K$, then there exists a sequence of 
$(\mathbb {Z}/p\mathbb {Z})$-extensions 
$\{ L_{i}/K \}_{i=1}^{\infty }$ satisfying
\[ \mathrm{rank}_{\mathbb{Z}} A(L_{i}) \leq \begin{cases} \mathrm{rank}_{\mathbb{Z}} (A(K))+3(r_{0}+4g), & \text{if} \ p=2,\\ \mathrm{rank}_{\mathbb{Z}} (A(K))+(p-1)(r_{0}+4g), & \text{otherwise}, \end{cases} \]and
\[ \lim_{i\rightarrow \infty}\dim_{\mathbb{F}_{p}} \mathrm{Sel}_{p}(A/L_{i})= \infty, \]
where 
$r_{0}:=\dim _{\mathbb {F}_{p}} \mathrm {Sel}_{p}(A/K)$ and 
$g:=\dim A$.
This result gives a positive answer to Problem 1.2 when 
$p\neq \mathrm {char}\, K$. Indeed, by the well-known exact sequence
\begin{equation} 0 \rightarrow A(L)/pA(L)\rightarrow \mathrm{Sel}_{p}(A/L) \rightarrow \text{III} (A/L) [p]\rightarrow 0 \end{equation}
and the inequality 
$\dim _{\mathbb {F}_{p}} A(L)/pA(L) \leq \mathrm {rank}_{\mathbb {Z}} A(L) +2\dim (A)$, we have the following result.
Theorem 1.4 If 
$p \neq \mathrm {char}\, K$, then
\[ \dim_{\mathbb{F}_{p}} \text{III} (A/L)[p] \]
can be arbitrarily large as 
$L$ ranges over 
$(\mathbb {Z}/p\mathbb {Z})$-extensions of 
$K$.
The idea behind the proof of the above results is in fact rather simple. In [Reference ČesnavičiusČes17], Česnavičius found a method to construct a sequence of 
$(\mathbb {Z}/p\mathbb {Z})$-extensions 
$\{ L_{i} \}_{i=1}^{\infty }$ such that
\[ \lim_{i\rightarrow \infty}\dim_{\mathbb{F}_{p}} \mathrm{Sel}_{p}(A/L_{i})= \infty . \]
However, he did not bound 
$\mathrm {rank}_{\mathbb {Z}} A(L_{i})$. We will use the tools developed by Mazur and Rubin in [Reference Mazur and RubinMR18] to bound the Mordell–Weil ranks.
1.1 Layout of this paper
Section 2 is devoted to introducing the machinery of Mazur and Rubin. In § 3 we generalize Česnavičius's idea to construct 
$(\mathbb {Z}/n\mathbb {Z})$-extensions 
$L/K$ with large 
$n$-rank of 
$\mathrm {Sel}_{n}(A/L)$. We prove our main result, Theorem 4.10, in § 4 and then give applications of this result in the rest of this paper. In § 5 we obtain a result on the growth of the potential 
$\text{III}$ in cyclic extensions, which generalizes the work of Clark, Sharif and Creutz in [Reference Clark and SharifCS10, Reference CreutzCre11]. In § 6 we solve a problem raised by Lim and Murty in [Reference Lim and MurtyLM16] concerning the growth of the fine Tate–Shafarevich groups.
1.2 Notation and convention
•
$p$ will always be a prime number.• A
$(\mathbb {Z}/n\mathbb {Z})$-extension is a Galois extension whose Galois group is cyclic of order $n$, that is, isomorphic to $\mathbb {Z}/n\mathbb {Z}$.• For a
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension $L/K$ and $0\leq i\leq k$, $L^{(i)}$ is the unique $(\mathbb {Z}/p^{i}\mathbb {Z})$-subextension of $K$ inside $L$. Thus $\mathrm {Gal}(L/L^{(i)})\cong \mathbb {Z}/p^{k-i}\mathbb {Z}$ and $\mathrm {Gal}(L^{(i)}/K)\cong \mathbb {Z}/p^{i}\mathbb {Z}$.• For
$n \in \mathbb {Z}_{\geq 2}$, the $n$-rank of an abelian group $H$, denoted by $r_n(H)$, is the largest $r \in \mathbb {N}$ such that $(\mathbb {Z}/n\mathbb {Z})^{r}$ can be viewed as a subgroup of $H$. In particular, $r_{p^{k}}(H)=\dim _{\mathbb {F}_p} p^{k-1}H/p^{k} H$.• If
$K$ is a global field, let $\mathcal {P}\ell _{K}$ denote the set of places of $K$.• For any place
$v$ of a global field $K$, we fix a $K$-embedding $\sigma : \overline {K}\hookrightarrow \overline {K_{v}}$ and let $L_v=L K_v$ for any finite extension $L$ of $K$, which is the completion of $L$ with respect to the unique valuation extending $v$ corresponding to the embedding $L\stackrel {\mathrm {id}}{\hookrightarrow }\overline {K} \stackrel {\sigma }{\hookrightarrow } \overline {K_{v}}$.• If
$K$ is a local field, let $K^{\mathrm {ur}}$ denote the maximal unramified extension of $K$.• For an abelian variety
$A$ over a global field $K$, let $\mathrm {Sel}_{n}(A/K)$ denote the $n$-Selmer group of $A$ over $K$.
2. The machinery of Mazur and Rubin
Suppose 
$K$ is a field and 
$A$ is an abelian variety over 
$K$. In this section we introduce the machinery developed by Mazur and Rubin in [Reference Mazur and RubinMR18], which plays a key role in our proof of bounding the Mordell–Weil ranks in 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions. However, in [Reference Mazur and RubinMR18], this machinery requires 
$A$ to be a simple abelian variety and 
$p$ be unramified in the center of the endomorphism ring 
$\mathrm {End}_{K}(A)$ (see [Reference Mazur and RubinMR18, § 5] for details). In order to deal with all prime numbers and arbitrary abelian varieties, we revise this machinery slightly so that our revision is closer to the treatment in [Reference Mazur and RubinMR07]. One should keep in mind the small difference between our setting and that in [Reference Mazur and RubinMR18]. The results in this section are analogous to those in [Reference Mazur and RubinMR18, §§ 6–8] and can be proved similarly.
2.1 Twists of abelian varieties
We recall some basic knowledge about twists of abelian varieties. This conception was first discussed by Milne in [Reference MilneMil72], and later generalized by Mazur and Rubin to the case of commutative algebraic groups in [Reference Mazur, Rubin and SilverbergMRS07].
Suppose 
$k\geq 1$ and 
$L/K$ is a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension. Recall that 
$L^{(i)}$ is the unique 
$(\mathbb {Z}/p^{i}\mathbb {Z})$-subextension of 
$K$ inside 
$L$. Denote 
$G:=\mathrm {Gal}(L/K)$. We have the following definition in the sense of [Reference Mazur, Rubin and SilverbergMRS07, Definition 1.1].
Definition 2.1 The 
$( L/K)$-twist 
$A_{L}$ of 
$A$ is the abelian variety
\[ A_{L}:=\mathrm{Ker}\,(\mathbb{Z}[G]\rightarrow\mathbb{Z}[\mathrm{Gal}(L^{(k-1)}/K)])\otimes A \]
over 
$K$. More concretely, by [Reference Mazur, Rubin and SilverbergMRS07, Theorem 5.8], 
$A_{L}=\mathrm {Ker}\, (\mathrm {Res}_{K}^{L} A \rightarrow \mathrm {Res}_{K}^{L^{(k-1)}} A )$. We also set 
$A_{K}=A$.
Remark 2.2 From now on in this paper, 
$A_{L}$ always means the 
$(L/K)$-twist of 
$A$, not to be confused with 
$A \times _{K} L$, the base change of 
$A$ to 
$L$.
Notation 2.3 Set
\[ \mathbb{Z}_{L}:=\mathbb{Z}[G]/\mathcal{N} \mathbb{Z}[G],\quad\text{where}\ \mathcal{N}:=\sum_{\sigma \in \mathrm{Gal}(L/L^{(k-1)})} \sigma \in \mathbb{Z}[G], \]
which is a free 
$\mathbb {Z}$-module of rank 
$\varphi (p^{k})=p^{k-1}(p-1)$. Set 
$\mathbb {Z}_K=\mathbb {Z}$.
By fixing an isomorphism 
$G\cong \mu _{p^{k}}$, we have 
$\mathbb {Z}_{L} \cong \mathbb {Z}[\mu _{p^{k}}]$ and we identify these two through this isomorphism, which gives an inclusion 
$\mathbb {Z}_{L}\hookrightarrow \mathbb {Q}(\mu _{p^{k}})$. Let 
$\mathfrak {p}_{L}$ be the unique prime ideal of 
$\mathbb {Z}_{L}$ above 
$p$. Let 
$\mathfrak {p}_K=(p)$.
Theorem 2.4 There is an isomorphism of 
$\mathbb {Z}[G_{K}]$-modules
\[ A[p]\cong A_{L}[\mathfrak{p}_{L}]. \]Proof. The case where 
$A$ is an elliptic curve was proved in [Reference Mazur and RubinMR07, Proposition 4.1]. The proof for the general case is essentially the same. One can also deduce the isomorphism by a similar argument to that in [Reference Mazur and RubinMR18, Corollary 6.4].
2.2 Local conditions
In this subsection we suppose 
$K$ is a local field whose residue field is 
$\mathbb {F}_{q}$ with 
$p\neq \mathrm {char}\, \mathbb {F}_{q}$. Let 
$A$ be an abelian variety over 
$K$. Let 
$L/K$ be a 
$(\mathbb {Z}/p^{k}\mathbb {Z} )$-extension, 
$k\geq 0$.
Notation 2.5 The group 
$\mathcal {H}( L/K )$ is the subgroup of 
$\text {H}^{1}(K, A[p])$ given by
\[ \mathcal{H}( L/K ):=\mathrm{Im}\,( A_{L}(K)/\mathfrak{p}_{L}A_{L}(K) \hookrightarrow \text{H}^{1}(K,A_{L}[\mathfrak{p}_{L}])\cong \text{H}^{1}(K,A[p])), \]
where the first inclusion is the Kummer map and the second isomorphism is induced by the isomorphism 
$A_{L}[\mathfrak {p}_L{}]\cong A[p]$ of 
$G_K$-modules. In particular, 
$\mathcal {H}( K ) =\mathcal {H}( K/K )$ is the image of the Kummer map
\[ \mathcal{H}( K ) =\mathcal{H}( K/K ) :=\mathrm{Im}\,(A(K)/p A(K) \hookrightarrow \text{H}^{1}(K,A[p])). \] Recall that if 
$p \neq \mathrm {char}\, \mathbb {F}_{q}$ and 
$A/K$ has good reduction, the unramified subgroup of 
$\text {H}^{1}(K,A[p])$ is given by
\[ \text{H}_{\mathrm{ur}}^{1}(K,A[p]) :=\ker(\text{H}^{1}(K,A[p])\rightarrow \text{H}^{1}(K^{\mathrm{ur}},A[p]) )=\text{H}^{1}(K^{\mathrm{ur}}/K,A[p]) . \]
We have the following basic facts about the subgroups 
$\mathcal {H}( L/K )$ and 
$H_{\mathrm {ur}}^{1}(K,A[p])$ of 
$\text {H}^{1}(K, A[p])$.
Lemma 2.6 Suppose 
$p\neq \mathrm {char}\, \mathbb {F}_{q}$. Then the following assertions hold.
(1)
$\dim _{\mathbb {F}_{p}} \mathcal {H}( L/K )= \dim _{\mathbb {F}_{p}} A(K)[p]$.(2) If
$A/K$ has good reduction, and $\phi \in G_{K}$ is an element whose restriction in $\mathrm {Gal}(K^{\mathrm {ur}}/K)$ is the Frobenius, then
\[ \dim_{\mathbb{F}_{p}} \mathcal{H}( L/K )= \dim_{\mathbb{F}_{p}} A[p]/(\phi-1) A[p]. \]
Proof. See [Reference Mazur and RubinMR18, Lemma 7.2].
Lemma 2.7 Suppose 
$p\neq \mathrm {char}\, \mathbb {F}_{q}$, 
$L/K$ is unramified, and 
$A/K$ has good reduction.
(1) If
$\phi \in G_{K}$ is an element that restricts to Frobenius in $\mathrm {Gal}(K^{\mathrm {ur}}/K)$, then evaluation of cocycles at $\phi$ gives an isomorphism
\[ \text{H}_{\mathrm{ur}}^{1}(K,A[p]) \simeq A[p]/(\phi-1) A[p]. \](2) The twist
$A_{L}$ has good reduction over $K$, and
Thus under these assumptions\[ \mathcal{H}( L/K )=\text{H}_{\mathrm{ur}}^{1}(K,A[p]). \]$\mathcal {H}( L/K )$ is independent of $L$.
Proof. See [Reference Mazur and RubinMR18, Lemma 7.3].
Proposition 2.8 Suppose 
$p\neq \mathrm {char}\, \mathbb {F}_{q}$, 
$L/K$ is nontrivial and totally ramified, and 
$A/K$ has good reduction. Recall that 
$L^{(1)}$ is the unique 
$(\mathbb {Z}/p\mathbb {Z})$-extension of 
$K$ contained in 
$L$.
(1) The map
induced by the inclusion\[ A_{L}(K)/\mathfrak{p}_{L}A_{L}(K)\rightarrow A_{L}(L^{(1)})/ \mathfrak{p}_{L}A_{L}(L^{(1)}) \]$A_{L}(K)\hookrightarrow A_{L}(L^{(1)})$ is the zero map.(2)
$\mathcal {H}( L/K )=\mathrm {Hom}(\mathrm {Gal}(L^{(1)}/K),A(K)[p] )$.
Proof. The first assertion is essential for the proof of the second, which plays an important role in the proof of Theorem 4.10. One can refer to [Reference Mazur and RubinMR18, Lemma 7.4] for the proof of (1). Assertion (2) was implied in the proof of [Reference Mazur and RubinMR18, Proposition 7.8], but, because of its importance, we include its proof here.
Consider the following commutative diagram.
We have 
$a=0$ by the first assertion, thus
\[ \mathcal{H}(L/K) \subset \ker c=\mathrm{Hom}(\mathrm{Gal}(L^{(1)}/K),A(K)[p]). \]According to Lemma 2.6, we obtain
\[ \dim_{\mathbb{F}_{p}} \mathcal{H}( L/K )= \dim_{\mathbb{F}_{p}} A(K)[p]= \dim_{\mathbb{F}_{p}}\mathrm{Hom}(\mathrm{Gal}(L^{(1)}/K),A(K)[p]) , \]
so 
$\mathcal {H}( L/K )=\mathrm {Hom}(\mathrm {Gal}(L^{(1)}/K),A(K)[p] )$.
2.3 Relative Selmer groups
In this subsection we fix a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L/K$ of global fields with 
$p\neq \mathrm {char}\, K$, and we allow the case 
$L=K$.
Definition 2.9 The relative Selmer group 
$\mathrm {Sel}(L/K,A[p])$ is the subgroup of 
$\text {H}^{1}(K,A[p])$ defined by the exact sequence
\[ 0 \longrightarrow \mathrm{Sel}(L/K,A[p]) \longrightarrow \text{H}^{1}(K,A[p]) \longrightarrow \displaystyle \prod_{v \in \mathcal{P}\ell_{K}}\dfrac{\text{H}^{1}(K_{v},A[p])}{\mathcal{H}(L_{v}/K_{v})} , \]
where (in our notation) 
$L_{v}$ is the completion of 
$L$ at some place of 
$L$ above 
$v$. Note that 
$\mathrm {Sel}(K/K,A[p])$ is nothing more than 
$\mathrm {Sel}_{p}(A/K)$.
Lemma 2.10 The isomorphism 
$\text {H}^{1}(K,A[p])\cong \text {H}^{1}(K,A_L[\mathfrak {p}_{L}])$ identifies the standard 
$\mathfrak {p}_{L}$-Selmer group 
$\mathrm {Sel}_{\mathfrak {p}_{L}}(A_{L}/K)$ of 
$A_{L}$ with 
$\mathrm {Sel}(L/K,A[p])$, that is, there exists an isomorphism 
$\phi$ making the following diagram commutative.
Proof. This follows almost verbatim from the argument to prove [Reference Mazur and RubinMR18, Lemma 8.4].
The important fact that enables us to bound the Mordell Weil ranks in 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions is that there is an isogeny (see [Reference Mazur, Rubin and SilverbergMRS07, Theorem 4.5])
\[ \bigoplus_{i=0}^{k}A_{L^{(i)}}\rightarrow \mathrm{Res}_{K}^{L}A \]
over 
$K$. Then, taking 
$K$-rational points, we get
\begin{equation} \mathrm{rank}_{\mathbb{Z}} (A(L)) = \mathrm{rank}_{\mathbb{Z}} (A(K))+ \sum_{i=1}^{k}\mathrm{rank}_{\mathbb{Z}} A_{L^{(i)}}(K). \end{equation} By Lemma 2.10 one can use the relative Selmer groups to bound the ranks of 
$A_{L^{(i)}}(K)$, which gives the following theorem.
Theorem 2.11 Suppose 
$L/K$ is a nontrivial 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension, and let 
$L^{(i)}$ denote the extension of 
$K$ of degree 
$p^{i}$ inside 
$L$. Then
\begin{equation} \mathrm{rank}_{\mathbb{Z}} (A(L)) \leq \mathrm{rank}_{\mathbb{Z}} (A(K))+ \sum_{i=1}^{k} \varphi(p^{i}) \dim_{\mathbb{F}_{p}}(\mathrm{Sel}(L^{(i)}/K,A[p])). \end{equation}Proof. The proof is very close to that of [Reference Mazur and RubinMR18, Proposition 8.8]. By Lemma 2.10, the Kummer map induces an inclusion
\[ A_{L^{(i)}}(K)\otimes (\mathbb{Z}_{L^{(i)}}/\mathfrak{p}_{L^{(i)}}) \hookrightarrow \mathrm{Sel}(L^{(i)}/K,A[p]). \]
Note also that 
$\mathbb {Z}/p\mathbb {Z} =\mathbb {Z}_{L^{(i)}}/\mathfrak {p}_{L^{(i)}}\mathbb {Z}_{L^{(i)}}$. Thus,
\begin{align*} \mathrm{rank}_{\mathbb{Z}} A_{L^{(i)}}(K) &= \varphi(p^{i}) \mathrm{rank}_{\mathbb{Z}_{L^{(i)}}}A_{L^{(i)}}(K) \\ &\leq \varphi(p^{i}) \dim_{\mathbb{F}_{p}} A_{L^{(i)}}(K)\otimes (\mathbb{Z}_{L^{(i)}}/\mathfrak{p}_{L^{(i)}}) \\ & \leq \varphi(p^{i}) \dim_{\mathbb{F}_{p}}(\mathrm{Sel}(L^{(i)}/K,A[p])). \end{align*}The above inequality combined with (2) completes the proof.
3. Growth of $n$-Selmer ranks in degree $n$ cyclic extensions
In this section, let 
$n \geq 2$ be a fixed integer and 
$A$ be an abelian variety over a global field 
$K$ with 
$\mathrm {char}\, K=0$ or 
$\mathrm {char}\, K \nmid n$. Based on Česnavičius's idea in [Reference ČesnavičiusČes17], we explain how to construct 
$(\mathbb {Z}/n\mathbb {Z})$-extensions 
$L/K$ with large 
$r_{n}(\mathrm {Sel}_n (A/L))$. The following results hold in a more general setting, but the special case is enough for our applications. One can refer [Reference ČesnavičiusČes17, §§ 4 and 5] for more general statements.
Theorem 3.1 [Reference ČesnavičiusČes17, Theorem 4.2]
Let 
$S$ be a finite subset of 
$\mathcal {P}\ell _{K}$ containing the places above 
$n\infty$ or where 
$A$ has bad reduction, and 
$(\cdot ) ^{\ast }$ denote the Pontryagin dual of 
$(\cdot )$. Then there is an exact sequence
\begin{align*} 0 \rightarrow \mathrm{Sel}_{n}(A/K) &\rightarrow \text{H}^{1}(\mathrm{Gal}(K^{S}/K),A[n]) \rightarrow \bigoplus_{v \in S} \dfrac{ \text{H}^{1}(K_{v},A[n])}{\mathcal{\mathcal{H}}(K_{v})} \rightarrow \mathrm{Sel}_{n}(A^{\vee}/K)^{\ast} \\ &\rightarrow \text{H}^{1}(\mathrm{Gal}(K^{S}/K),A[n])\rightarrow \bigoplus_{v \in S} \text{H}^{2}(K_{v},A[n]), \end{align*}
where 
$K^{S}$ is the maximal extension of 
$K$ unramified outside 
$S$.
Remark 3.2 The fppf cohomology was used in the original statement of [Reference ČesnavičiusČes17, Theorem 4.2]. Here we rephrase it in the language of Galois cohomology, due to the canonical isomorphism
\[ \text{H}_{\mathrm{fppf}}^{i}(U,\mathcal{A}[n])\cong \text{H}^{i}(\mathrm{Gal}(K^{S}/K),A[n]) \]
(see [Reference ČesnavičiusČes15b, p. 1661, equation (1)]), where 
$U:=X- S$, 
$\mathcal {A}$ is the Néron model of 
$A$ and
\[ X:= \begin{cases} \mathrm{Spec}\, O_{K}, & \text{if} \ \mathrm{char}\, K=0;\\ C_{K}, & \text{if} \ \mathrm{char}\, K>0, \end{cases} \]
with 
$C_{K}$ the proper smooth curve over a finite field whose function field is 
$K$.
We also note that if 
$K$ is a global function field, the condition ‘above 
$n\infty$’ is an empty one.
From now on, we shall use the following notation.
Notation 3.3 The set 
$\Sigma$ is a fixed finite subset of 
$\mathcal {P}\ell _{K}$ such that:
(1)
$\{v \in \mathcal {P}\ell _{K} \mid A \ \text {has bad reduction at} \ v \ \text {or} \ v\mid n\infty \} \subset \Sigma$;(2) the primes in
$\Sigma$ generate the class group of $K$.
For a 
$(\mathbb {Z}/n\mathbb {Z})$-extension 
$L/K$, define
\[ S_{L}:=\{v \in \mathcal{P}\ell_{K} \mid v\notin \Sigma \ \text{is totally ramified in} \ L,\ \text{and splits completely in}\ K(A[n])\}. \]The following result is based on Česnavičius's idea presented in the proof of [Reference ČesnavičiusČes17, Theorem 5.2].
Theorem 3.4 For any 
$(\mathbb {Z}/n\mathbb {Z})$-extension 
$L/K$, let 
$X_{L}:=\mathrm {res}_{L/K}(\text {H}^{1}(K,A[n])) \cap \mathrm {Sel}_{n}(A/L)$. Then there exists a constant 
$c>0$ independent of 
$L$ such that
\[ r_{n}(X_{L}) \geq |S_{L}|-c. \]Proof. Let 
$\Sigma$ be as in Notation 3.3, 
$S:=\Sigma \cup S_{L}$, and 
$S^{\prime }$ (respectively, 
$\Sigma ^{\prime }$) be the set of places of 
$L$ above 
$S$ (respectively, 
$\Sigma$). According to [Reference ČesnavičiusČes16, Proposition 2.5(d)], for all 
$v \in S_{L}$, 
$\mathcal {H}(K_{v})=\text {H}^{1}_{\mathrm {ur}}(K_{v},A[n])$. Thus by Theorem 3.1, we have a commutative diagram with exact rows as follows.
Then the snake lemma yields the exact sequence
\begin{equation} \mathrm{Ker}\, b_{L}\rightarrow \mathrm{Ker}\,(c_L|_{\mathrm{Im}\, d_{L}}) \stackrel{h_{L}}\rightarrow\mathrm{Coker}\, a_{L} \stackrel{j_{L}}\rightarrow \mathrm{Coker}\, b_{L}. \end{equation}
Note that 
$\mathrm {Ker}\, b_{L} \subset \ker (\text {H}^{1}(K,A[n])\rightarrow \text {H}^{1}(L,A[n]) )=\text {H}^{1}(\mathrm {Gal}(L/K),A[n](L))$, whose order is bounded by a constant independent of 
$L$. Along with (4), this implies
\begin{equation} r_{n}(\mathrm{Im}\, h_{L}) \geq r_{n}( \mathrm{Ker}\, (c_{L}|_{\mathrm{Im}\, d_{L}}))-c_1 \end{equation}
for some constant 
$c_1$ independent of 
$L$.
Note that 
$\mathrm {Ker}\, c_{L}/\mathrm {Ker}\, (c_{L}|_{\mathrm {Im}\, d_{L}}) \hookrightarrow \mathrm {Coker}\, d_{L} \hookrightarrow \mathrm {Sel}_{n}(A^{\vee }/K)^{\ast }$, whose order is finite and independent of 
$L$. This implies that
\begin{equation} r_{n}( \mathrm{Ker}\, (c_{L}|_{\mathrm{Im}\, d_{L}}))\geq r_{n}( \mathrm{Ker}\, c_{L})-c_2 \end{equation}
for some constant 
$c_2$ independent of 
$L$.
Let 
$\pi _{L}:\mathrm {Sel}_{n}(A/L)\longrightarrow \mathrm {Coker}\, a_{L}$ be the projection. Then one can easily check that
\begin{equation} \mathrm{Ker}\, (j_{L}\circ\pi_{L}) \subset X_{L}. \end{equation}
Note that 
$\pi _{L}:\mathrm {Ker}\,(j_{L}\circ \pi _{L})\twoheadrightarrow \mathrm {Ker}\, j_{L}$ is surjective, so we have
\begin{equation} r_{n}(\mathrm{Ker}\,(j_{L}\circ \pi_{L})) \geq r_{n}(\mathrm{Ker}\, j_{L}) =r_{n}(\mathrm{Im}\, h_{L}). \end{equation}Applying (5)–(8), we then have
\[ r_{n}(X_{L}) \geq r_{n}(\mathrm{Ker}\,(j_{L}\circ \pi_{L})) \geq r_{n}(\mathrm{Im}\, h_{L})\geq r_{n}(\mathrm{Ker}\, c_{L})-c \]
for some constant 
$c$ independent of 
$L$. It remains to show the following claim.
Claim 
$r_{n}(\mathrm {Ker}\, c_{L}) \geq |S_L|$.
Note that for 
$v \in S_{L}$, 
$A[n]$ is isomorphic to 
$( \mathbb {Z}/n\mathbb {Z})^{2g}$ over 
$K_{v}$. So 
$\text {H}^{1}(K_{v},\mathbb {Z}/n\mathbb {Z})$ is a direct factor of 
$\text {H}^{1}(K_{v},A[n])$, and
\[ \text{H}^{1}(K_{v},\mathbb{Z}/n\mathbb{Z})=\mathrm{Hom}(G_{K_{v}}, \mathbb{Z}/n\mathbb{Z} ) \supset \text{H}_{\mathrm{ur}}^{1}(K_{v}, \mathbb{Z}/n\mathbb{Z}). \]
Since 
$L_{v}/K_{v}$ is a totally ramified 
$( \mathbb {Z}/n\mathbb {Z})$-extension, 
$I_{K_{v}}/I_{L_{v}}\cong \mathbb {Z}/n\mathbb {Z}$. Choose a continuous homomorphism 
$f: G_{K_{v}}/I_{L_{v}} \rightarrow \mathbb {Z}/n\mathbb {Z}$ whose restriction on 
$I_{K_{v}}/I_{L_{v}}$ is an isomorphism to 
$\mathbb {Z}/n\mathbb {Z}$. Let 
$\bar {f}$ be its image in 
$\text {H}^{1}(K_{v},\mathbb {Z}/n\mathbb {Z})/\text {H}_{\mathrm {ur}}^{1}(K_{v}, \mathbb {Z}/n\mathbb {Z})$. Then
\[ 0 \neq \bar{f} \in \text{H}^{1}(K_{v},\mathbb{Z}/n\mathbb{Z})/ \text{H}_{\mathrm{ur}}^{1}(K_{v}, \mathbb{Z}/n\mathbb{Z}),\ 0=\bar{f}|_{G_{L_{v}}} \in \text{H}^{1}(L_{v},\mathbb{Z}/n\mathbb{Z})/\text{H}_{\mathrm{ur}}^{1}(L_{v}, \mathbb{Z}/n\mathbb{Z}). \]
Thus 
$0 \neq \bar {f} \in \mathrm {Ker}\, c_{L}$. We check that the order of 
$\bar {f}$ is exactly 
$n$. Let 
$g \in I_{K_{v}}/I_{L_{v}}$ be the preimage of 
$1\in \mathbb {Z}/n\mathbb {Z}$. Then, for 
$1\leq m < n$, 
$(mf)(g)=m\neq 0$ in 
$\mathbb {Z}/n\mathbb {Z}$, hence 
$m\bar {f}\neq 0$.
Such a construction is valid for every 
$v \in S_{L}$, so we obtain a set of 
$|S_L|$ nonzero elements of order 
$n$ in 
$\mathrm {Ker}\, c_{L}$, each lying in different direct summand hence 
$(\mathbb {Z}/n\mathbb {Z})$-linearly independent. Thus we have 
$r_{n}(\mathrm {Ker}\, c_{L}) \geq |S_L|$. This completes the proof.
4. Bounding the Mordell–Weil ranks in $(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions
In this section we always assume 
$K$ is a global field with 
$p \neq \mathrm {char}\, K$.
If 
$L/K$ is a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension with large 
$| S_{L} |$, then Theorem 3.4 implies that 
$\dim _{\mathbb {F}_{p}} \mathrm {Sel}_{p} (A/L)$ is also large. However, it could be possible that 
$\mathrm {rank}_{\mathbb {Z}} (A(L))$ is very large, which leads to small 
$\text{III} (A/L)[p]$. By Theorem 2.11, we can bound 
$\mathrm {rank}_{\mathbb {Z}} (A(L))$ by the relative Selmer groups 
$\mathrm {Sel}(L^{(i)}/K,A[p])$. So the 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions we need are those 
$L/K$ with
\[ {\rm large}\ |S_{L}|\ {\rm and\ also\ small}\ \mathrm {Sel}(L^{(i)}/K,A[p]). \]We give a method for finding such extensions in this section, which enables us to prove our main result.
4.1 $(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions of global fields with given completions at local places
In [Reference Mazur and RubinMR18], Mazur and Rubin defined the so-called 
$T$-ramified, 
$\Sigma$-split extensions and showed the existence of such extensions. Under mild hypotheses, they proved that, if 
$T$ is chosen properly, then any 
$T$-ramified, 
$\Sigma$-split extension 
$L/K$ satisfies
\[ \mathrm{Sel}(L/K, A[\lambda]) =0\quad (\text{thus } \mathrm{rank}_{\mathbb{Z}}A(K)=\mathrm{rank}_{\mathbb{Z}}A(L) ), \]
in which 
$A[\lambda ]$ is a subgroup scheme of 
$A[p]$ defined in [Reference Mazur and RubinMR18, Definition 6.2].
However, these mild hypotheses may not hold in general. As one will see in the proof of Theorem 4.10, in order to seek 
$L/K$ with small 
$\dim _{\mathbb {F}_{p}}\mathrm {Sel}(L/K,A[p])$ and large 
$|S_{L} |$, only requiring 
$L/K$ to be 
$T$-ramified, 
$\Sigma$-split seems insufficient, and we need to require more: that 
$L$ should have given completions at all 
$v \in T \setminus \{ v_{1},v_{n}\}$, in which 
$T=\{v_{1},v_{2},\dots,v_{n}\}$ with 
$v_{1},v_{n}$ two special elements in 
$T$. This leads to the following discussion in this subsection, and our main result is Lemma 4.6.
Definition 4.1 Let 
$\Sigma ^{\prime }$ be the set of all places of 
$K^{\prime }:=K(\mu _{p^{k}})$ above those in 
$\Sigma$. Suppose 
$\Sigma$ and 
$\Sigma ^{\prime }$ satisfy the respective conditions in Notation 3.3. Denote
\begin{equation} \mathcal{P}:=\left\{ v \in \mathcal{P}\ell_{K} \mid v \notin \Sigma, v\, \text{splits completely in} \, K^{\prime}(\sqrt[p^{k}]{O_{K^{\prime},\Sigma^{\prime}}^{\times}})\right\}. \end{equation}
Suppose 
$T$ is a non-empty finite subset of 
$\mathcal {P}$. A 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L/K$ is called 
$T$-ramified and 
$\Sigma$-split if:
(1)
$L/K$ is totally ramified at $v \in T$ and unramified outside $T$;(2)
$L/K$ splits completely at $v \in \Sigma$.
For 
$v \in \mathcal {P}$, let
\begin{equation} Y_{v}:=K^{\times}(O_{v}^{\times})^{p^{k}}\prod_{w \in \Sigma} K_{w}^{\times} \prod_{w \notin \Sigma \cup \{v\}}O_{w}^{\times}. \end{equation}
Then we have a surjective map 
$O_{v}^{\times }\twoheadrightarrow \textbf {A}_{K}^{\times }/Y_{v}$, which induces isomorphisms
\[ \mathbb{Z}/p^{k}\mathbb{Z}\cong O_{v}^{\times}/(O_{v}^{\times})^{p^{k}}\cong \textbf{A}_{K}^{\times}/Y_{v} \]
by the definition of 
$\mathcal {P}$. Let
\begin{equation} K(v):= \text{ the abelian extension of $ K $ corresponding to}\ Y_{v} . \end{equation}
Then by class field theory we see that 
$K(v )/K$ is a 
$\{ v\}$-ramified, 
$\Sigma$-split 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension.
Theorem 4.2 Suppose 
$T= \{ v_{1},\ldots,v_{n} \} \subset \mathcal {P}$. If 
$L/K$ is a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension which is 
$T$-ramified and 
$\Sigma$-split, then 
$L \subset K(v_{1})\cdots K(v_{n})$. In particular, for every 
$v \in \mathcal {P}$, 
$K(v)$ is the only 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension which is 
$\{v \}$-ramified and 
$\Sigma$-split.
Proof. Let 
$Y_{L}$ be the norm group of 
$\textbf {A}_{K}^{\times }$ corresponding to 
$L$. By class field theory, since 
$L/K$ is unramified outside 
$T$,
\[ (\cdots,1,O_{v}^{\times},1,\cdots) \subset Y_{L}\quad \text{for all}\ v \notin T; \]
since 
$L_{v}=K_{v}$ for 
$v \in \Sigma$,
\[ (\cdots,1,K_{v}^{\times},1,\cdots) \subset Y_{L}\quad \text{for all}\ v \in \Sigma; \]
since 
$L/K$ is a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension,
\[ \prod_{i=1}^{n}(O_{v_{i}}^{\times})^{p^{k}}\subset Y_L. \]Combining these facts yields
\[ Y=K^{\times}\prod_{i=1}^{n}(O_{v_{i}}^{\times})^{p^{k}}\prod_{v \in \Sigma} K_{v}^{\times} \prod_{v \notin \Sigma \bigcup T}O_{v}^{\times} \subset Y_{L} . \]
Note that 
$Y$ is exactly the norm group corresponding to 
$K(v_{1})\cdots K(v_{n})$. Thus
\[ L \subset K(v_{1})\cdots K(v_{n}). \] If 
$\mu _{p^{k}} \subset K$, then by Kummer theory, every 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L/K$ can be written as 
$L=K\big (\sqrt [p^{k}]{a}\big )$ for some 
$a \in K^{\times }$. We have the following lemma.
Lemma 4.3 Suppose 
$\mu _{p^{k}} \subset K$ and 
$v\in \mathcal {P}$. Let 
$\Sigma _{0}\not \ni v$ be any finite subset of 
$\mathcal {P}\ell _{K}$ which generates the class group of 
$K$. Then there exists 
$a \in O_{K,\Sigma _{0}\cup \{v \}}^{\times }$ such that 
$K(v)=K(\sqrt [p^{k}]{a})$.
Proof. Suppose 
$K(v)=K\bigl (\sqrt [p^{k}]{b}\bigr )$ for some 
$b \in K^{\times }$. Then, for every place 
$w \neq v$, we have 
$p^{k} \mid \mathrm {ord}_w(b)$ since 
$K(v) /K$ is unramified outside 
$\{ v \}$, and 
$\mathrm {ord}_w(b)=0$ for almost all places. Denote
\[ \{w_{1},\ldots,w_{n} \} :=\{ w \neq v, w\notin \Sigma_{0} \mid \mathrm{ord}_w(b)\neq 0 \} . \]
For each 
$w_{i}$, the sequence
\[ O_{K,\Sigma_{0}\cup \{w_i\}}^{\times} \xrightarrow{ \mathrm{ord}_{w_{i}} } \mathbb{Z} \rightarrow 0 \]
is exact since 
$\Sigma _{0}$ generates the class group of 
$K$. Let 
$\beta _{i}\in O_{K,\Sigma _{0}\cup \{w_i\}}^{\times }$ such that 
$\mathrm {ord}_{w_i}(b)=1$. Write 
$\mathrm {ord}_{w_{i}}(b)=p^{k}k_{i}$. Then
\[ a :=\dfrac{b}{\beta_{1}^{p^{k}k_{1}}\cdots \beta_{n}^{p^{k}k_{n}}} \in O_{K,\Sigma_{0}\cup\{v \}}^{\times} \quad \text{and} \quad K(v)=K\bigl(\sqrt[p^{k}]{a}\bigr). \] Recall that 
$(\cdotp,\cdotp ) _{v}$ is the Hilbert symbol, and 
$(\frac {\cdotp }{\cdotp })_{p^{k}}$ is the 
$p^{k}$th power residue symbol, whose definitions and properties can be found in [Reference NeukirchNeu13, Chapter V, § 3].
Lemma 4.4 Suppose 
$\mu _{p^{k}} \subset K$ and 
$p^{k}\neq 2$. Let 
$\{v_{1},v_{2}\} \subset \mathcal {P}$ such that 
$K(v_{i}) =K(\sqrt [p^{k}]{a_{i}})$ with 
$p, a_{1}$ and 
$a_{2}$ relatively prime. Then:
(1)
$(\frac {a_{1}}{a_{2}})_{p^{k}}= (\frac {a_{2}}{a_{1}})_{p^{k}}$;(2)
$(\frac {a_{1}}{v_{2}})_{p^{k}} =1 \Leftrightarrow (\frac {a_{2}}{v_{1}})_{p^{k}}=1$.
Consequently, 
$v_{1}$ splits completely in 
$K(v_{2})$ if and only if 
$v_{2}$ splits completely in 
$K(v_{1})$.
Proof. (1) According to the reciprocity law of the 
$p^{k}$th power residue (see [Reference NeukirchNeu13, Chapter VI, Theorem 8.3]), we have
\[ \bigg(\dfrac{a_{1}}{a_{2}}\bigg)_{p^{k}}\bigg(\dfrac{a_{2}}{a_{1}}\bigg)_{p^{k}}^{-1}= \prod_{v \mid p\infty} (a_{1},a_{2})_{v}. \]
If 
$v \mid \infty$, then 
$v$ must be a complex place by our assumption, so 
$(a_{1},a_{2})_{v}=1$; if 
$v \mid p$, then 
$a_{1} \in O_{v}^{\times }$ and 
$K(\sqrt [p^{k}]{a_{2}}) /K$ is unramified at 
$v$, so 
$a_{1} \in \mathrm {\textbf {Nm}} \ K_{v}(\sqrt [p^{k}]{a_{2}})$, which implies that 
$(a_{1},a_{2})_{v}=1$. Thus,
\[ \bigg(\dfrac{a_{1}}{a_{2}}\bigg)_{p^{k}}\bigg(\dfrac{a_{2}}{a_{1}}\bigg)_{p^{k}}^{-1}=1. \] (2) As 
$K(v_i)$ is totally ramified at 
$v_i$ and unramified outside 
$v_i$, 
$(a_{i})= v_{i}^{k_{i}}I_{i}^{p^{k}}$ with 
$p \nmid k_{i} \in \mathbb {Z}$ and 
$I_{i}$ a fractional ideal relatively prime to 
$(p)v_{i}$. If 
$(\frac {a_{1}}{v_{2}})_{p^{k}}=1$, then 
$(\frac {a_{1}}{a_{2}})_{p^{k}}=1$ and by (1) we get 
$1=(\frac {a_{2}}{a_{1}}) _{p^{k}}=(\frac {a_{2}}{v_{1}})_{p^{k}}^{k_{1}}$. Thus we have 
$(\frac {a_{2}}{v_{1}})_{p^{k}}=1$ since 
$p \nmid k_{1}$. The converse is similar.
Lemma 4.5 Suppose 
$\mu _{p^{k}} \subset K$ and 
$T=\{ v_{1},\ldots,v_{n} \} \subset \mathcal {P}$. Then any 
$T$-ramified and 
$\Sigma$-split 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension of 
$K$ has the form
\[ L= K(\sqrt[p^{k}]{a_{1}^{x_{1}}\cdots a_{n}^{x_{n}}}) \]
where 
$x_{i} \in (\mathbb {Z}/p^{k}\mathbb {Z})^{\times }$ and 
$a_{i} \in K^{\times }$ such that 
$K(v_i)=K(\sqrt [p^{k}]{a_{i}})$.
Proof. This is clear from Theorem 4.2.
Lemma 4.6 Suppose 
$n\geq 2$, 
$T=\{ v_{1},\ldots,v_{n} \} \subset \mathcal {P}$ and 
$p^{k}\neq 2$. Let 
$v_{n+1} \in \mathcal {P} \setminus T$ be a place splitting completely in 
$K(v_{2})\cdots K(v_{n})$ and 
$T_{1}=T\cup \{v_{n+1}\}$. If 
$L/K$ is a 
$T$-ramified and 
$\Sigma$-split 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension, then there exists a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L_{1}/K$ such that:
(1)
$L_{1}/ K$ is $T_{1}$-ramified and $\Sigma$-split;(2)
$(L_{1})_{v_{i}}=L_{v_{i}}$ for $2 \leq i \leq n$.
Proof. For convenience, we denote 
$K_{i}:=K(v_{i})$ for 
$1 \leq i \leq n+1$. Let 
$K^{\prime }:=K(\mu _{p^{k}})$, 
$L^{\prime }:=LK^{\prime }$ and 
$d:=[K^{\prime }:K]$. Let 
$\Sigma ^{\prime }$ be the set of primes of 
$K^{\prime }$ above 
$\Sigma$. By definition of 
$\mathcal {P}$, the place 
$v_i$ splits completely in 
$K'$. Let 
$v_i^{j}\ (1\leq j\leq d)$ be the primes of 
$K'$ above 
$v_i$. The sets of primes in 
$K'$ above 
$T$, 
$v_{n+1}$ and 
$T_1$ are
\[ T'=\{v_i^{j}\mid 1\leq i\leq n, 1\leq j\leq d\},\quad \Pi=\{v_{n+1}^{j}\mid 1\leq j\leq d\}\quad \text{and}\quad T_1'=T'\cup \Pi, \]
respectively. Then 
$L^{\prime }/K^{\prime }$ is 
$T^{\prime }$-ramified and 
$\Sigma ^{\prime }$-split.
Choosing finite pairwise disjoint sets of primes 
$\Sigma _{v'}\ (v'\in T'_1)$ of 
$K'$ which generate the class group of 
$K'$ and contain no prime above 
$p$, and then applying Lemma 4.3, we obtain 
$a_{v'} \in O_{K^{\prime },\Sigma _{v'}}$ such that
•
$K^{\prime }(v')=K^{\prime }(\sqrt [p^{k}]{a_{v'}})$, $p$ and all $a_{v'}$ are pairwise coprime.
Let 
$a_{(i-1)d+j}=a_{v_i^{j}}$ for 
$1\leq i\leq n+1$ and 
$1\leq j\leq d$.
By Lemma 4.5, 
$L^{\prime }= K^{\prime }\Bigl(\sqrt [p^{k}]{a_{1}^{x_{1}}\cdots a_{nd}^{x_{nd}}}\,\Bigr)$ with 
$x_{i} \in (\mathbb {Z}/p^{k}\mathbb {Z})^{\times }$ for each 
$i$. Note that 
$K^{\prime }K_{n+1}/K^{\prime }$ is 
$\Pi$-ramified and 
$\Sigma ^{\prime }$-split. Then 
$K_{n+1}K^{\prime } =K^{\prime }\Bigl(\sqrt [p^{k}]{a_{nd+1}^{x_{nd+1}}\cdots a_{(n+1)d}^{x_{(n+1)d}}}\,\Bigr)$. Let
\[ L_{1}^{\prime}:=K^{\prime}\Big(\sqrt[p^{k}]{ a_{1}^{x_{1}}\cdots a_{nd}^{x_{nd}}a_{nd+1}^{x_{nd+1}}\cdots a_{(n+1)d}^{x_{(n+1)d}} }\Big). \]
Then 
$L_{1}^{\prime } \subset K_{1}\cdots K_{n}K_{n+1}K^{\prime }$ is 
$T^{\prime }_1$-ramified and 
$\Sigma ^{\prime }$-split. Since 
$v_{n+1}$ splits completely in 
$K_{i}$ for 
$i\geq 2$, we have
\[ \bigg(\frac{a_{(i-1)d+j}}{v_{n+1}^{j'}}\bigg) _{p^{k}}=1\ \Longrightarrow \bigg(\frac{a_{nd+j'}}{v_i^{j}}\bigg)_{p^{k}}=1 \]by Lemma 4.4. This implies
\begin{equation} (L_{1}^{\prime})_{v_i^{j}}=L^{\prime}_{v_i^{j}}=L_{v_{i}},\quad 2 \leq i \leq n,\ 1 \leq j \leq d. \end{equation} For any nonempty subset 
$I\subset [n+1]=\{1,\ldots, n+1\}$, let 
$K_I=\prod _{i\in I} K_i$. By considering the ramification of primes we see that 
$K_I\cap K_J=K$ if 
$I\cap J=\emptyset$ and 
$K_I\cap K'=K$. Now by induction we have the canonical isomorphisms
\begin{align*} &\mathrm{Gal}(K_I/K)\cong \prod_{i\in I} \mathrm{Gal}(K_i/K),\quad\mathrm{Gal}(K_I K'/K')\cong \mathrm{Gal}(K_I/K), \\ &\mathrm{Gal}(K_IK'/K)\cong \mathrm{Gal}(K_I/K)\times \mathrm{Gal}(K'/K). \end{align*}
Let 
$H=\mathrm {Gal}(K_{[n+1]}K'/L_1')$ and 
$L_1= K_{[n+1]}^{H}$. Then one can check that 
$L_{1}^{\prime }=L_{1}K'$ and 
$L_{1}/K$ is a 
$T_{1}$-ramified and 
$\Sigma$-split 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension.
\begin{gather*} w\ {\rm is\ totally\ ramified\ (respectively,\ unramified,\ splits\ completely)}\ \Rightarrow\\ v\ {\rm is\ totally\ ramified\ (respectively,\ unramified,\ splits\ completely)} \end{gather*} Moreover, (12) implies that for 
$2 \leq i \leq n$, we have
\[ L_{v_{i}}=(L_{1}^{\prime})_{v_{i}}=(L_{1})_{v_{i}}(K^{\prime})_{v_{i}}=(L_{1})_{v_{i}}. \]This completes the proof.
Remark 4.7 It is natural to ask whether, for each 
$v_{i} \in T$, given a totally ramified 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$\mathcal {L}_{i} /K_{v_{i}}$, there exists a global 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L/K$ which is 
$T$-ramified, 
$\Sigma$-split and 
$L_{v_{i}}= \mathcal {L}_{i}$ for 
$v_i\in T$. The famous Grunwald–Wang theorem [Reference Neukirch, Schmidt and WingbergNSW08, Theorem 9.2.8] asserts that there does exist an extension 
$L/K$ such that 
$L_{v_{i}}= \mathcal {L}_{i}$ for 
$1\leq i\leq n$; however, it may be ramified at some 
$v \notin T$. And one can prove that an extension which is 
$T$-ramified, 
$\Sigma$-split and 
$L_{v_{i}}= \mathcal {L}_{i}$ may not exist in general.
4.2 Bounding the Mordell–Weil ranks in $(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions
Now fix an abelian variety 
$A$ over 
$K$. We denote
\begin{align*} F&:=K(A[p],(O_{K,\Sigma}^{\times})^{1/p^{k}}),\\ T_{F}&:=\{v \in \mathcal{P}\ell_{K}\mid v \notin \Sigma, v \ \text{splits completely in } F/K \},\\ S_{L}&:=\{v \in \mathcal{P}\ell_{K} \mid v\notin \Sigma, v \ \text{splits completely in } K(A[p])\ \text{and is totally ramified in} \ L \}. \end{align*}
Obviously we have 
$T_{F} \subset \mathcal {P}$ and the density theorem ensures that 
$T_{F}$ has positive density.
Definition 4.8 Suppose that 
$T=\{ v_{1},\ldots,v_{n} \} \subset \mathcal {P}$ and, for each 
$v_{i}$, 
$\mathcal {L}_{i}/K_{v_{i}}$ is a totally ramified 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension. Let 
$W_{i}:=\mathcal {H}( \mathcal {L}_{i}/K_{v_{i}})$ and 
$\mathcal {W}_{n}:=\prod _{1\leq i\leq n} W_{i}$. The artificial Selmer group 
$\mathrm {Sel}(W_{1}\times \cdots \times W_{n},A[p])=\mathrm {Sel}(\mathcal {W}_{n}, A[p] )$ is defined by the exact sequence
\[ 0 \longrightarrow \mathrm{Sel}(\mathcal{W}_{n}, A[p]) \longrightarrow \text{H}^{1}(K, A[p] ) \longrightarrow \displaystyle \prod_{i=1 }^{n}\dfrac{\text{H}^{1}(K_{v_{i}},A[p])}{W_{i}} \displaystyle \prod_{v \notin T}\dfrac{\text{H}^{1}(K_{v},A[p])}{\mathcal{H}(K_{v})} . \]
For 
$v_{i} \in T$, the strict Selmer group 
$\mathrm {Sel}(\mathcal {W}_{n}, A[p]) _{v_{i}}$ at 
$v _{i}$ is the group
\[ \mathrm{Sel}(\mathcal{W}_{n}, A[p]) _{v_{i}}:=\ker( \mathrm{Sel}(\mathcal{W}_{n}, A[p])\longrightarrow \text{H}^{1}(K_{v_{i}},A[p]) ). \] One can deduce the finiteness of 
$\mathrm {Sel}(\mathcal {W}_{n},A[p])$ from the finiteness of 
$\mathrm {Sel}(K,A[p])$.
Lemma 4.9 Let 
$T$ be a finite subset of 
$\mathcal {P}$, and 
$L/K$ be a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension which is 
$T$-ramified and 
$\Sigma$-split. If 
$K \subsetneq L' \subset L$, then
\[ \mathrm{Sel}(L'/K,A[p])=\mathrm{Sel}(L/K,A[p]). \]Proof. See [Reference Mazur and RubinMR18, Lemma 9.16].
Theorem 4.10 Suppose 
$K$ is a global field, 
$p \neq \mathrm {char}\, K$. Let 
$A$ be an abelian variety of dimension 
$g$ over 
$K$ and 
$r_0=\dim _{\mathbb {F}_{p}} \mathrm {Sel}_{p}(A/K)$. Then there exists a sequence of 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions 
$\{ L_{i} \}_{i=1}^{\infty }$ such that:
(1)
$|S_{L_{i}}| \geq i$;(2)
$\mathrm {rank}_{\mathbb {Z}} (A(L_{i})) \leq \begin {cases} \mathrm {rank}_{\mathbb {Z}} (A(K))+3(r_{0}+4g), & \text {if} \ p^{k}=2,\\ \mathrm {rank}_{\mathbb {Z}} (A(K))+(p^{k}-1)(r_{0}+4g), & \text {if otherwise}. \end {cases}$
Proof. We shall construct by induction a set of primes 
$\{v_i\}_{i= 1}^{\infty }\subset T_F$ and a sequence of 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extensions 
$\{L_i\}_{i= 1}^{\infty }$ of 
$K$ such that the following assertions hold.
(i)
$L_{i}$ is $T_{i}$-ramified and $\Sigma$-split, where $T_{i}:=\{v_1,\ldots, v_{i}\}$; in particular, $S_{L_i}\supseteq T_i$ and $|S_{L_{i}}|\geq i$.(ii) If
$i >2$, then $v_{i}$ splits completely in $K_{2}\cdots K_{i-1}$ and is inert in $K_{1}$, where $K_i:=K(v_i)$;(iii)
$v_{2}$ is inert in $K_{1}$.(iv) The inequalities
(13)are always satisfied, where\begin{equation} r_{i} \leq r_{0}+s_{i}\leq r_0+2g \end{equation}\begin{align*} W_{i}&:=\mathcal{H}((L_{i})_{v_{i}} / K_{v_{i}}),\quad \mathcal{W}_i:=W_1\times\cdots\times W_i,\\ r_{i}&:=\dim_{\mathbb{F}_{p}}\mathrm{Sel}(\mathcal{W}_{i},A[p]),\\ t_{i+1}&:=\dim_{\mathbb{F}_{p}} \mathrm{Im}\,(\mathrm{Sel}(\mathcal{W}_{i},A[p]) \rightarrow H_{\mathrm{ur}}^{1}(K_{v_{i+1}},A[p] )),\\ s_{i}&:=\dim_{\mathbb{F}_{p}} \mathrm{Im}\,(\mathrm{Sel}(\mathcal{W}_{i},A[p]) \rightarrow \mathcal{H}(L_{v_{i}}/K_{v_{i}}) ). \end{align*}
Note that 
$s_i, t_i\leq 2g$, so only the first inequality in (13) needs to be addressed.
For the base step, choose an arbitrary 
$v_{1} \in T_{F}$ and let 
$L_{1}:=K(v_{1})=K_1$. One can deduce from the exact sequence (18) below that
\[ r_{1}=r_{0}-t_{1}+s_{1} \leq r_{0}+s_{1}. \]
Assume that we have already constructed 
$\{v_i\}_{i=1}^{n}$ and 
$\{ L_{i} \}_{i=1}^{n}$, 
$n \geq 1$.
If 
$s_{n}=0$, we choose an arbitrary 
$v_{n+1} \in T_{F}$ which splits completely in 
$K_2\cdots K_{n}$ and is inert in 
$K_{1}$.
If 
$s_{n} \geq 1$, let 
$L_{1,n}$ be the unique 
$(\mathbb {Z}/p\mathbb {Z})$-extension of 
$K_{v_{n}}$ contained in 
$(L_{n})_{v_{n}}$. Then, by Proposition 2.8,
\[ \mathcal{H}( (L_{n})_{v_{n}}/K_{v_{n}})=\mathrm{Hom}(\mathrm{Gal}(L_{1,n}/K_{v_{n}}),A(K_{v_{n}})[p] ). \]
Let 
$\bar {\sigma }$ be a generator of 
$\mathrm {Gal}(L_{1,n}/K_{v_{n}})$. So we can pick linearly independent 
$c_{n,1},\ldots,c_{n,s_{n}} \in \mathrm {Sel}(\mathcal {W}_{n},A[p])$ such that
\begin{equation} c_{n,1}(\bar{\sigma}) ,\ldots,c_{n,s_{n}}(\bar{\sigma}) \subset A[p]\ \text{are linear independent over}\ \mathbb{F}_{p}. \end{equation}Note that by the induction assumption, we have:
•
$(K_{1})_{v_{n}}/K_{v_{n}}$ is nontrivial and unramified;•
$(K_{n})_{v_{n}}/K_{v_{n}}$ is totally ramified;•
$(K_{2} \cdots K_{n-1})_{v_{n}}=K_{v_{n}}$.
Thus, there exists a pre-image 
$\sigma \in G_{K_{v_{n}}}= G_{F_{v_{n}}} \subset G_{F}$ of 
$\bar {\sigma }$ such that
\[ \sigma|_{K_{1}}\neq 1,\quad \sigma| _{K_{2} \cdots K_{n}} =1\ (\text{if} \ n\geq 2) . \]
One should note that if 
$n=1$, then we only require 
$\sigma |_{K_{1} }\neq 1$. Denote 
$d_{n,j}:=c_{n,j}|_{F}$ for 
$1\leq j \leq s_{n}$. Choose a Galois extension 
$N/K$ such that
\[ G_{N} \subset \bigcap_{i=1}^{s_{n}}\ker(d_{n,i})\quad \text{and}\quad FK_1\cdots K_n \subset N. \]
By the Chebotarev density theorem, there exists 
$v_{n+1} \notin T_{n}\cup \Sigma$ such that
$v_{n+1}$ is unramified in 
$N/K$ and 
$\mathrm {Frob}_{v_{n+1}}|_{N}=\sigma |_{N}$.
In particular, 
$\mathrm {Frob}_{v_{n+1}}|_{F}=\sigma |_{F}=1$ and thus 
$v_{n+1} \in T_{F}$. By our choice,
\[ c_{n,i}(\mathrm{Frob}_{v_{n+1}}) =d_{n,i}(\mathrm{Frob}_{v_{n+1}})=d_{n,i}(\sigma)=d_{n,i}(\bar{\sigma})=c_{n,i}(\bar{\sigma}),\quad 1\leq i \leq s_{n}. \]Thus, by (14) we conclude that
\begin{equation} c_{n,1}(\mathrm{Frob}_{v_{n+1}}) ,\ldots,c_{n,s_{n}}(\mathrm{Frob}_{v_{n+1}}) \subset A[p] \ \text{ are linearly independent over} \ \mathbb{F}_{p}. \end{equation}
According to our choice, 
$v_{n+1}$ splits completely in 
$K_2\cdots K_{n}$ and is inert in 
$K_{1}$, so by Lemma 4.6, there exists a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L_{n+1}/K$ which is 
$T_{n+1}$-ramified, 
$\Sigma$-split and
\begin{equation} ( L_{n+1})_{v_{i}}=(L_{n})_{v_{i}},\quad 2 \leq i \leq n. \end{equation}Since the restrict map
\[ \mathrm{\textbf{loc}}_{v_{n+1}}: \mathrm{Sel}(\mathcal{W}_{n},A[p]) \rightarrow H_{\mathrm{ur}}^{1}(K_{v_{n+1}},A[p] ) \]
can be regarded as the evaluation of cocycles at 
$\mathrm {Frob}_{v_{n+1}}$, (15) implies that
\begin{equation} t_{n+1}= \dim_{\mathbb{F}_{p}} \mathrm{Im}\,( \mathrm{\textbf{loc}}_{v_{n+1}})\geq s_{n}. \end{equation}Observe the following diagram with exact rows.
Recall that 
$s_{n+1}=\dim _{\mathbb {F}_{p}}\mathrm {Im}\, \mathrm {\textbf {loc}}^{\prime }_{v_{n+1}}, t_{n+1}=\dim _{\mathbb {F}_{p}}\mathrm {Im}\,\mathrm {\textbf {loc}}_{v_{n+1}}$, thus
\[ r_{n+1}- s_{n+1}=\dim_{\mathbb{F}_{p}}\mathrm{Sel}(\mathcal{W}_{n+1},A[p] )_{v_{n+1}}=\dim_{\mathbb{F}_{p}}\mathrm{Sel}(\mathcal{W}_{n}, A[p])_{v_{n+1}}=r_{n}-t_{n+1}, \]which implies that
\[ r_{n+1}=r_{n}-t_{n+1}+s_{n+1}. \]Using the induction assumption and (17), we obtain
\[ r_{n+1}=r_{n}-t_{n+1}+s_{n+1}\leq r_{0}+s_{n}-t_{n+1}+s_{n+1}\leq r_{0}+s_{n+1}. \]
This completes our construction. We claim that for all 
$m\geq 1$,
\[ \mathrm{rank}_{\mathbb{Z}}(A(L_{m})) \leq \mathrm{rank}_{\mathbb{Z}} (A(K))+(p^{k}-1) (r_{0}+4g). \]By (16), we have
\begin{equation} W_{i}=\mathcal{H}((L_{i})_{v_{i}}/K_{v_{i}}) =\mathcal{H}((L_{m})_{v_{i}}/K_{v_{i}}),\quad 2\leq i \leq m . \end{equation}Consider the following diagram with exact rows.
in which (19) gives the vertical equality. Thus, we have
\[ \dim_{\mathbb{F}_{p}}\mathrm{Sel}(L_{m}/K, A[p] ) \leq\dim_{\mathbb{F}_{p}} \mathrm{Sel}(\mathcal{W}_{m} , A[p] )_{v_{1}}+2g\leq r_{0}+4g. \]Recall that by Lemma 4.9,
\[ \mathrm{Sel}(L_{m}^{(i)}/K,A[p]) =\mathrm{Sel}(L_{m}/K,A[p]). \]It follows from Theorem 2.11 that
\begin{align*} \mathrm{rank}_{\mathbb{Z}} (A(L_{m})) & \leq \mathrm{rank}_{\mathbb{Z}} (A(K))+\sum_{i=1}^{k} \varphi(p^{i}) \dim_{\mathbb{F}_{p}}\mathrm{Sel}(L_{m}^{(i)}/K,A[p]) \\ & \leq \mathrm{rank}_{\mathbb{Z}} (A(K))+(p^{k}-1) (r_{0}+4g). \end{align*}
The proof of theorem is completed except the case 
$p^{k}=2$.
As for the case 
$p^{k}=2$, by previous discussion we can find 
$(\mathbb {Z}/2^{2}\mathbb {Z})$-extensions 
$\{L_{i}/K \}_{i=1}^{\infty }$ such that:
(i)
$| S_{L_{i}} | \geq i$;(ii)
$\mathrm {rank}_{\mathbb {Z}} A(L_{i}) \leq \mathrm {rank}_{\mathbb {Z}} (A(K))+3 (r_{0}+4g)$.
Furthermore, by our construction, for all 
$v \in S_{L_{i}}$, 
$v$ is totally ramified in 
$L_{i}/K$, thus 
$v$ is totally ramified in 
$L_{i}^{(1)} /K$. Then 
$\{L_{i}^{(1)}/K \}_{i=1}^{\infty }$ is the sequence of quadratic extensions we require.
Theorem 4.11 Let 
$n\geq 2$ be an integer. If 
$K$ is a global field such that 
$\mathrm {char}\, K=0$ or 
$\mathrm {char}\, K \nmid n$, then
\[ r_{n}(\text{III} (A/L)) \]
can be arbitrarily large as 
$L$ ranges over 
$(\mathbb {Z}/n\mathbb {Z})$-extensions of 
$K$.
Proof. We first treat the case 
$n=p^{k}$. In this case, the result follows from Theorem 4.10, Theorem 3.4 and the exact sequence (1).
Next suppose 
$n=\prod _{i=1}^{t}p_{i}^{k_{i}}$ is the prime decomposition of 
$n$. By the first step we can find a 
$(\mathbb {Z}/p_{i}^{k_{i}} \mathbb {Z})$-extension 
$L_{i}/K$ for each 
$i$ such that
\[ r_{p_{i}^{k_{i}}}(\text{III}(A/L_{i}))\geq m. \]
Let 
$L=L_{1}\cdots L_{t}$. Note that the 
$p_{i}$-primary part of
\[ \mathrm{Ker}\,( \text{H}^{1}(L_{i},A) \rightarrow \text{H}^{1}(L,A) ) =\text{H}^{1}(\mathrm{Gal}(L/L_{i}),A(L)) \]is zero, then the restriction homomorphism
\[ \text{III}(A/L_{i})[p_{i}^{k_{i}}] \rightarrow \text{III}(A/L) \]is injective. This implies
\[ r_{p_{i}^{k_{i}}}(\text{III}(A/L))\geq r_{p_{i}^{k_{i}}}( \text{III}(A/L_{i})[p_{i}^{k_{i}}] ) \geq m,\quad 1\leq i \leq t. \]
Then 
$L/K$ is a 
$(\mathbb {Z}/n\mathbb {Z})$-extension we require.
5. Growth of potential $\text{III}$
In this section we fix an abelian variety 
$A$ defined over a global field 
$K$. We study the growth of 
$n$-rank of the potential 
$\text{III}$ of 
$A$ over 
$K$, whose definition we now recall.
Definition 5.1 Let 
$L/K$ be a finite extension of global fields. The potential 
$\text{III}$ of 
$A/K$ in 
$L$ is
\[ \text{III}_{K}(A/L):=\mathrm{res}_{L/K}(\text{H}^{1}(K,A) )\cap \text{III}(A/L). \]Clark and Sharif proved the following result.
Theorem 5.2 [Reference Clark and SharifCS10, Theorem 3]
Let 
$E/K$ be an elliptic curve and 
$n$ be an positive integer such that 
$\mathrm {char}\, K=0$ or 
$\mathrm {char}\, K \nmid n$. Then, for any positive integer 
$r$, there exists a field extension 
$L/K$ of degree 
$n$ such that
\[ r_{n}( \text{III}_{K}(A/L) ) \geq r. \]Creutz later considered the case of abelian varieties. He proved the following result.
Theorem 5.3 [Reference CreutzCre11, Theorem 1]
Let 
$A$ be a strongly principally polarized abelian variety over a number field 
$K$ such that the 
$G_{K}$-action on the Néron–Severi group is trivial. Then, for any prime 
$p$ and any integer 
$N$, there exists a degree 
$p$ extension 
$L/K$ for which
\[ r_{p}( \text{III}_{K}(A/L) ) >N. \]The method used to prove the above theorems is closely related to the study of the period and index problem in the Weil–Châtelet group. Here we apply a different method, which can treat high-dimensional abelian varieties, to generalize the above two results.
Theorem 5.4 Let 
$n\geq 2$ be a positive integer and 
$K$ be a global field with 
$\mathrm {char}\, K =0$ or 
$\mathrm {char}\, K\nmid n$. Then, for an abelian variety 
$A$ over 
$K$ and an arbitrary positive integer 
$m$, there exists a 
$(\mathbb {Z}/n\mathbb {Z})$-extension 
$L/K$ such that
\[ r_{n}(\text{III}_{K}(A/L)) \geq m. \]Proof. First consider the case 
$n=p^{k}$. By Theorems 4.10 and 3.4, there exists a 
$(\mathbb {Z}/p^{k}\mathbb {Z})$-extension 
$L/K$ such that
\[ r_{p^{k}}(X_{L}) \geq M+2g+m \quad \text{and} \quad r:=\mathrm{rank}_{\mathbb{Z}}A(L)\leq M, \]
where 
$X_{L}=\mathrm {res}_{L/K}(\text {H}^{1}(K,A[n])) \cap \mathrm {Sel}_{n}(A/L)$. Denote 
$\psi _{L}:\text {H}^{1}(L,A[p^{k}]) \rightarrow \text {H}^{1}(L,A)[p^{k}]$. Then 
$\mathrm {Ker}\, \psi _{L}=A(L)/p^{k}A(L)$. Since 
$r \leq M$, we have 
$r_{p^{k}}(\psi _{L}(X_{L})) \geq m$. So it suffices to show that 
$\psi _{L}(X_{L}) \subset \text{III} _{K}(A/L)$. Since 
$X_{L} \subset \mathrm {Sel}_{p^{k}}(A/L)$, from the exact sequence
we obtain 
$\psi _{L}(X_{L}) \subset \text{III} (A/L)$. Consider the following commutative diagram.
The fact that 
$X_{L}\subset \mathrm {Im}\, \, \mathrm {res}_{L/K}^{\prime }$ implies that 
$\psi _{L}(X_{L})\subset \mathrm {res}_{L/K}(\text {H}^{1}(K,A)[p^{k}])$. Thus
\[ \psi_{L}(X_{L}) \subset \text{III}_{K}(A/L). \]
The general case follows by replacing 
$\text{III} (A/L)$ by 
$\text{III} _{K}(A/L)$, and applying the same argument as in the proof of Theorem 4.11.
6. Growth of fine Selmer groups and fine Tate–Shafarevich groups
The aim of this section is to solve a problem raised by Lim and Murty in [Reference Lim and MurtyLM16]. Our answer also generalizes their results about the growth of fine Selmer groups in 
$(\mathbb {Z}/p\mathbb {Z})$-extensions.
Definition 6.1 Let 
$A$ be an abelian variety over a number field 
$K$, and recall that
\[ \mathrm{Sel}_{p^{k}}(A/K):=\mathrm{Ker}\,( \text{H}^{1}(K,A[p^{\infty}]))\rightarrow \bigoplus_{v \in \mathcal{P}\ell_{K}}\text{H}^{1}(K_{v},A)[p^{\infty}]). \]
The 
$p^{k}$-fine Selmer group 
$R_{p^{k}}(A/K)$ of 
$A$ over 
$K$ is defined by the exact sequence
\[ 0 \rightarrow R_{p^{k}}(A/K)\rightarrow \mathrm{Sel}_{p^{k}}(A/K)\rightarrow\bigoplus_{v \mid p}H^{1}(K_{v},A[p^{k}]). \]
Similarly the 
$p^{\infty }$-fine Selmer group 
$R_{p^{\infty }}(A/K)$ is defined by the exact sequence
\[ 0 \rightarrow R_{p^{\infty}}(A/K)\rightarrow \mathrm{Sel}_{p^{\infty}}(A/K)\rightarrow\bigoplus_{v \mid p}H^{1}(K_{v},A[p^{\infty}]). \]In [Reference WuthrichWut07], Wuthrich introduced the fine Tate–Shafarevich groups, which we now recall.
Definition 6.2 The fine Mordell–Weil group 
$M_{p^{k}}(A/K)$ is defined by the exact sequence
\[ 0 \rightarrow M_{p^{k}}(A/K)\rightarrow A(K)/p^{k}A(K)\rightarrow\bigoplus_{v \mid p}A(K_{v})/p^{k}A(K_{v}). \]Then the fine Tate–Shafarevich group
is defined by
One can similarly define 
$M_{p^{\infty }}(A/K)$ and
.
Lim and Murty proved the following result in [Reference Lim and MurtyLM16].
Theorem 6.3 [Reference Lim and MurtyLM16, Theorem 6.3]
Let 
$A$ be an abelian variety over a number field 
$K$. Suppose that 
$A(K)[p] \neq 0$. Then
\[ \sup\{ r_{p}(R_{p^{\infty}}(A/L))\mid L/K \text{ is a cyclic extension of degree } p \} = \infty. \]Furthermore, they posed the following problem.
Problem 6.4 Retaining the assumptions of above theorem, do we also have
Based on Theorem 4.11, we give a positive answer to the problem above in a more general setting.
Wuthrich has already observed that 
is a subgroup of 
$\text{III} (A/L)$ with finite index, and later Kundu observed that this index has a uniform upper bound independent of 
$L$, as a result of which we have the following proposition.
Proposition 6.5 [Reference KunduKun21, Proposition 4.7]
Let 
$A$ be an abelian variety over a number field 
$K$, varying over all 
$(\mathbb {Z}/p\mathbb {Z})$-extensions 
$L/K$. Then 
is unbound if and only if 
$\text{III} (A/L)$ is unbounded.
Since the unboundedness of 
$\text{III} (A/L)$ is proved by Theorem 4.11, we obtain the following result.
Theorem 6.6 Let 
$A$ be an abelian variety defined over a number field 
$K$. Then
Note that 
(respectively, 
) is a quotient group of 
$R_{p}(A/L)$ (respectively, 
$R_{p^{\infty }}(A/L)$), so we also get the unboundedness of fine Selmer group, which generalizes the result of Lim and Murty mentioned above by removing the condition 
$A(K)[p]\neq 0$.
Corollary 6.7 Let 
$A$ be an abelian variety over a number field 
$K$. Then
\begin{align*} \sup\{ r_{p}(R_{p}(A/L))\mid L/K \text{ is a cyclic extension of degree } p \} = \infty,\\ \sup\{ r_{p}(R_{p^{\infty}}(A/L))\mid L/K \text{ is a cyclic extension of degree } p \} = \infty. \end{align*}Acknowledgements
The authors would like to thank the anonymous referees heartily for their invaluable comments and suggestions which greatly improved this paper. In particular, their suggestion to prove a stronger unboundedness result in Theorem 3.4 than the original version enabled us to simplify and improve the presentation in § 5. We also thank Kęstutis Česnavičius for helpful correspondence.
This work is partially supported by the Innovation Program for Quantum Science and Technology (grant no. 2021ZD0302904) and the Anhui Initiative in Quantum Information Technologies (grant no. AHY150200).
