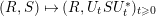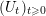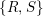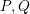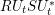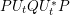1 Introduction
Let ![]() $(\mathscr{A},\unicode[STIX]{x1D70F})$ be a
$(\mathscr{A},\unicode[STIX]{x1D70F})$ be a ![]() $W^{\ast }$-probability space and let
$W^{\ast }$-probability space and let ![]() $(U_{t})_{t\geqslant 0}$ be a free unitary Brownian motion in
$(U_{t})_{t\geqslant 0}$ be a free unitary Brownian motion in ![]() $(\mathscr{A},\unicode[STIX]{x1D70F})$ with
$(\mathscr{A},\unicode[STIX]{x1D70F})$ with ![]() $U_{0}=\mathbf{1}$. For a given pair of orthogonal projections
$U_{0}=\mathbf{1}$. For a given pair of orthogonal projections ![]() $\{P,Q\}$ in
$\{P,Q\}$ in ![]() $\mathscr{A}$ that are freely independent from
$\mathscr{A}$ that are freely independent from ![]() $(U_{t})_{t\geqslant 0}$, the so-called liberation process
$(U_{t})_{t\geqslant 0}$, the so-called liberation process ![]() $(P,Q)\mapsto (P,U_{t}QU_{t}^{\ast })$ was introduced in [Reference Voiculescu19] in relation with the free entropy and the free Fisher information. In this paper, we are interested in the following variant of the liberation process:
$(P,Q)\mapsto (P,U_{t}QU_{t}^{\ast })$ was introduced in [Reference Voiculescu19] in relation with the free entropy and the free Fisher information. In this paper, we are interested in the following variant of the liberation process: ![]() $(R,S)\mapsto (R,U_{t}SU_{t}^{\ast })$ where
$(R,S)\mapsto (R,U_{t}SU_{t}^{\ast })$ where ![]() $\{R,S\}$ are the symmetries associated with
$\{R,S\}$ are the symmetries associated with ![]() $\{P,Q\}$, namely
$\{P,Q\}$, namely ![]() $R=2P-\mathbf{1},S=2Q-\mathbf{1}$. It is known, as a consequence of the asymptotic freeness of
$R=2P-\mathbf{1},S=2Q-\mathbf{1}$. It is known, as a consequence of the asymptotic freeness of ![]() $P$ and
$P$ and ![]() $U_{t}QU_{t}^{\ast }$, that the pair
$U_{t}QU_{t}^{\ast }$, that the pair ![]() $(R,U_{t}SU_{t}^{\ast })$ tends, as
$(R,U_{t}SU_{t}^{\ast })$ tends, as ![]() $t\rightarrow \infty$, to
$t\rightarrow \infty$, to ![]() $(R,USU^{\ast })$ where
$(R,USU^{\ast })$ where ![]() $U$ is a Haar unitary free from
$U$ is a Haar unitary free from ![]() $\{R,S\}$ and hence
$\{R,S\}$ and hence ![]() $R,USU^{\ast }$ are free (see [Reference Nica and Speicher17]). The connection between the two liberation processes can be understood by studying the relationship between their actions on the operators
$R,USU^{\ast }$ are free (see [Reference Nica and Speicher17]). The connection between the two liberation processes can be understood by studying the relationship between their actions on the operators ![]() $X_{t}:=PU_{t}QU_{t}^{\ast }P$ and
$X_{t}:=PU_{t}QU_{t}^{\ast }P$ and ![]() $Y_{t}:=RU_{t}SU_{t}^{\ast }$. This study is actually motivated by the important problem of proving the conjectured identity
$Y_{t}:=RU_{t}SU_{t}^{\ast }$. This study is actually motivated by the important problem of proving the conjectured identity ![]() $i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$ for two projections with arbitrary ranks. Here,
$i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$ for two projections with arbitrary ranks. Here, ![]() $i^{\ast }$ is the free mutual information introduced by Voiculescu (see [Reference Voiculescu19]) and
$i^{\ast }$ is the free mutual information introduced by Voiculescu (see [Reference Voiculescu19]) and ![]() $\unicode[STIX]{x1D712}_{\text{orb}}$ is the orbital free entropy due to Hiai, Miyamoto and Ueda (see [Reference Hiai, Miyamoto and Ueda12, Reference Ueda18]). A heuristic argument provided in [Reference Hiai and Ueda14, Section 3.2] supports this conjecture which was proved in [Reference Collins and Kemp4] in the special case
$\unicode[STIX]{x1D712}_{\text{orb}}$ is the orbital free entropy due to Hiai, Miyamoto and Ueda (see [Reference Hiai, Miyamoto and Ueda12, Reference Ueda18]). A heuristic argument provided in [Reference Hiai and Ueda14, Section 3.2] supports this conjecture which was proved in [Reference Collins and Kemp4] in the special case ![]() $\unicode[STIX]{x1D70F}(P)=\unicode[STIX]{x1D70F}(Q)=1/2$, then subsequently in [Reference Izumi and Ueda15]. In this last paper, the authors used a subordination relation to give more partial results for arbitrary ranks.
$\unicode[STIX]{x1D70F}(P)=\unicode[STIX]{x1D70F}(Q)=1/2$, then subsequently in [Reference Izumi and Ueda15]. In this last paper, the authors used a subordination relation to give more partial results for arbitrary ranks.
Here, we improve the results in [Reference Izumi and Ueda15] by providing a detailed explicit study of the unique subordinate family. To this end, we use stochastic calculus in order to derive a PDE for the Herglotz transform ![]() $H(t,z)$ of the spectral measure, says
$H(t,z)$ of the spectral measure, says ![]() $\unicode[STIX]{x1D708}_{t}$, of
$\unicode[STIX]{x1D708}_{t}$, of ![]() $Y_{t}$. It turns out that this is exactly the PDE governing the flow of an analytic function transform of the spectral measure, says
$Y_{t}$. It turns out that this is exactly the PDE governing the flow of an analytic function transform of the spectral measure, says ![]() $\unicode[STIX]{x1D707}_{t}$, of
$\unicode[STIX]{x1D707}_{t}$, of ![]() $X_{t}$ (see Remark 3.6 below). This allows us to develop a theory of subordination for the process
$X_{t}$ (see Remark 3.6 below). This allows us to develop a theory of subordination for the process ![]() $Y_{t}$ akin to [Reference Izumi and Ueda15]. In particular, we obtain an explicit computation of the subordinate function (which is a one-to-one map in the unit disc) and use it to show that its inverse extends continuously to the unit circle. As an application of our results, we prove under mild assumptions the identity
$Y_{t}$ akin to [Reference Izumi and Ueda15]. In particular, we obtain an explicit computation of the subordinate function (which is a one-to-one map in the unit disc) and use it to show that its inverse extends continuously to the unit circle. As an application of our results, we prove under mild assumptions the identity ![]() $i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$ for arbitrary ranks
$i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$ for arbitrary ranks ![]() $\unicode[STIX]{x1D70F}(P)$ and
$\unicode[STIX]{x1D70F}(P)$ and ![]() $\unicode[STIX]{x1D70F}(Q)$.
$\unicode[STIX]{x1D70F}(Q)$.
2 Analysis of the spectral measure of  $Y_{t}$
$Y_{t}$
2.1 Sequence of moments
Let ![]() $R,S\in \mathscr{A}$ be two orthogonal symmetries and
$R,S\in \mathscr{A}$ be two orthogonal symmetries and ![]() $U_{t},t\in [0,\infty )$ a free unitary Brownian motion freely independent from
$U_{t},t\in [0,\infty )$ a free unitary Brownian motion freely independent from ![]() $\{R,S\}$. Our goal here is to derive a system of ODEs satisfied by the sequence of moments of
$\{R,S\}$. Our goal here is to derive a system of ODEs satisfied by the sequence of moments of ![]() $\unicode[STIX]{x1D708}_{t}$ via free stochastic calculus.
$\unicode[STIX]{x1D708}_{t}$ via free stochastic calculus.
Proposition 2.1. Let ![]() $f_{n}(t):=\unicode[STIX]{x1D70F}([RU_{t}SU_{t}^{\ast }]^{n})\;n\geqslant 1,t\geqslant 0$, then
$f_{n}(t):=\unicode[STIX]{x1D70F}([RU_{t}SU_{t}^{\ast }]^{n})\;n\geqslant 1,t\geqslant 0$, then
 $$\begin{eqnarray}\displaystyle & \unicode[STIX]{x2202}_{t}f_{1}=-f_{1}+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}, & \displaystyle \nonumber\\ \displaystyle & \unicode[STIX]{x2202}_{t}f_{n}=-nf_{n}-n\displaystyle \mathop{\sum }_{k=1}^{n-1}f_{k}f_{n-k}+\left\{\begin{array}{@{}ll@{}}\displaystyle n^{2}\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\quad & \text{if }n\text{ is odd}\\ \displaystyle n^{2}\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}\quad & \text{if }n\text{ is even,}\end{array}\right.\quad n\geqslant 2 & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \unicode[STIX]{x2202}_{t}f_{1}=-f_{1}+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}, & \displaystyle \nonumber\\ \displaystyle & \unicode[STIX]{x2202}_{t}f_{n}=-nf_{n}-n\displaystyle \mathop{\sum }_{k=1}^{n-1}f_{k}f_{n-k}+\left\{\begin{array}{@{}ll@{}}\displaystyle n^{2}\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\quad & \text{if }n\text{ is odd}\\ \displaystyle n^{2}\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}\quad & \text{if }n\text{ is even,}\end{array}\right.\quad n\geqslant 2 & \displaystyle \nonumber\end{eqnarray}$$ where ![]() $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D70F}(R)$ and
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D70F}(R)$ and ![]() $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D70F}(S)$.
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D70F}(S)$.
Proof. Let ![]() $A_{t}=RU_{t}SU_{t}^{\ast }$, then using Ito’s formula, we have
$A_{t}=RU_{t}SU_{t}^{\ast }$, then using Ito’s formula, we have
Taking the trace in both sides and use the trace property, we get
The first summands do not depend on the summation variable ![]() $k$, while the second summands depend on the summation variable
$k$, while the second summands depend on the summation variable ![]() $j,k$ only through their difference
$j,k$ only through their difference ![]() $k-j$. Then reindexing by
$k-j$. Then reindexing by ![]() $l=k-j$, we get
$l=k-j$, we get
Since the number of pairs ![]() $(j,k)$ such that
$(j,k)$ such that ![]() $k-j=l$ for fixed
$k-j=l$ for fixed ![]() $l$ is equal to
$l$ is equal to ![]() $n-l$, then the second summation becomes
$n-l$, then the second summation becomes
This sum rewrites, after reindexing ![]() $k=n-l$, as
$k=n-l$, as
Using the trace property and adding the summations (2.1) and (2.2), we get
Thus, we have
Now since ![]() $R$ and
$R$ and ![]() $S$ are independent from
$S$ are independent from ![]() $t$, the free Ito’s formula implies
$t$, the free Ito’s formula implies
But, since
Then substituting these equations in the expression of ![]() $dA_{t}$ we get
$dA_{t}$ we get
The first two terms simplify to
while the last term is reduced to
Thus, we have
So that,
Since the trace of a stochastic integral is zero, then the first term in equation (2.3) is given by
Using the trace property and the relations ![]() $R^{2}=S^{2}=U_{t}U_{t}^{\ast }=1$, we have
$R^{2}=S^{2}=U_{t}U_{t}^{\ast }=1$, we have ![]() $\unicode[STIX]{x1D70F}(A_{t}^{n-1}R)=\unicode[STIX]{x1D70F}(R)=\unicode[STIX]{x1D6FC}$ if
$\unicode[STIX]{x1D70F}(A_{t}^{n-1}R)=\unicode[STIX]{x1D70F}(R)=\unicode[STIX]{x1D6FC}$ if ![]() $n$ is odd and
$n$ is odd and ![]() $\unicode[STIX]{x1D70F}(A_{t}^{n-1}R)=\unicode[STIX]{x1D70F}(S)=\unicode[STIX]{x1D6FD}$ otherwise.
$\unicode[STIX]{x1D70F}(A_{t}^{n-1}R)=\unicode[STIX]{x1D70F}(S)=\unicode[STIX]{x1D6FD}$ otherwise.
Hence, the first term in equation (2.3) is equal to
For the second term in equation (2.3), we shall use the following result.
Lemma 2.2. Let
Then
and for any adapted process ![]() $V_{t}$, we have
$V_{t}$, we have
Proof. The first statement is a consequence of Itô rules since ![]() $Z_{t}$ is a stochastic integral. For the last, we expand
$Z_{t}$ is a stochastic integral. For the last, we expand
 $$\begin{eqnarray}\displaystyle dZ_{t}\,V_{t}\,dZ_{t} & = & \displaystyle (iRU_{t}dB_{t}SU_{t}^{\ast }-iRU_{t}SdB_{t}U_{t}^{\ast })V_{t}(iRU_{t}dB_{t}SU_{t}^{\ast }-iRU_{t}SdB_{t}U_{t}^{\ast })\nonumber\\ \displaystyle & = & \displaystyle -RU_{t}dB_{t}SU_{t}^{\ast }V_{t}RU_{t}dB_{t}SU_{t}^{\ast }+RU_{t}dB_{t}SU_{t}^{\ast }V_{t}RU_{t}SdB_{t}U_{t}^{\ast }\nonumber\\ \displaystyle & & \displaystyle +\,RU_{t}SdB_{t}U_{t}^{\ast }V_{t}RU_{t}dB_{t}SU_{t}^{\ast }-RU_{t}SdB_{t}U_{t}^{\ast }V_{t}RU_{t}SdB_{t}U_{t}^{\ast }.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle dZ_{t}\,V_{t}\,dZ_{t} & = & \displaystyle (iRU_{t}dB_{t}SU_{t}^{\ast }-iRU_{t}SdB_{t}U_{t}^{\ast })V_{t}(iRU_{t}dB_{t}SU_{t}^{\ast }-iRU_{t}SdB_{t}U_{t}^{\ast })\nonumber\\ \displaystyle & = & \displaystyle -RU_{t}dB_{t}SU_{t}^{\ast }V_{t}RU_{t}dB_{t}SU_{t}^{\ast }+RU_{t}dB_{t}SU_{t}^{\ast }V_{t}RU_{t}SdB_{t}U_{t}^{\ast }\nonumber\\ \displaystyle & & \displaystyle +\,RU_{t}SdB_{t}U_{t}^{\ast }V_{t}RU_{t}dB_{t}SU_{t}^{\ast }-RU_{t}SdB_{t}U_{t}^{\ast }V_{t}RU_{t}SdB_{t}U_{t}^{\ast }.\nonumber\end{eqnarray}$$Applying the Itô rule
to each of these terms yields
Using the trace property and the relations ![]() $S^{2}=U_{t}U_{t}^{\ast }=1,A_{t}=RU_{t}SU_{t}^{\ast }$, we get
$S^{2}=U_{t}U_{t}^{\ast }=1,A_{t}=RU_{t}SU_{t}^{\ast }$, we get
which simplifies to give the equality (2.7).◻
It follows from (2.4) and (2.6) that for ![]() $n\geqslant 2$ and
$n\geqslant 2$ and ![]() $k\in \{1,\ldots ,n-1\}$,
$k\in \{1,\ldots ,n-1\}$,
which expands into four terms. But by use of Lemma 2.2, the only surviving term is
Taking the trace, we get
Using the same consideration leading to (2.1) and the fact that if ![]() $n$ is even then
$n$ is even then ![]() $k,n-k$ have the same parity and if
$k,n-k$ have the same parity and if ![]() $n$ is odd then
$n$ is odd then ![]() $k,n-k$ have opposite parity, we have
$k,n-k$ have opposite parity, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D70F}(A_{t}^{n-k-1}\,dA_{t}\,A_{t}^{k-1}dA_{t})=\left\{\begin{array}{@{}ll@{}}(2\unicode[STIX]{x1D6FC}^{2}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ even},k\text{ odd}\\ (2\unicode[STIX]{x1D6FD}^{2}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ even},k\text{ even}\\ (2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ odd},k\text{ odd}\\ (2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ odd},k\text{ even.}\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70F}(A_{t}^{n-k-1}\,dA_{t}\,A_{t}^{k-1}dA_{t})=\left\{\begin{array}{@{}ll@{}}(2\unicode[STIX]{x1D6FC}^{2}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ even},k\text{ odd}\\ (2\unicode[STIX]{x1D6FD}^{2}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ even},k\text{ even}\\ (2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ odd},k\text{ odd}\\ (2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-2\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k}))\,dt;\quad & n\text{ odd},k\text{ even.}\end{array}\right.\end{eqnarray}$$Hence, the second term on the RHS of (2.3) is equal to
 $$\begin{eqnarray}\displaystyle \displaystyle \left\{\begin{array}{@{}ll@{}}\left(-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+\frac{n^{2}}{2}\unicode[STIX]{x1D6FC}^{2}+\frac{n(n-2)}{2}\unicode[STIX]{x1D6FD}^{2}\right)\,dt\quad & \text{if }n\text{ is even}\\ \left(-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+n(n-1)\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\right)\,dt\quad & \text{if }n\text{ is odd}\end{array}\right. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \displaystyle \left\{\begin{array}{@{}ll@{}}\left(-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+\frac{n^{2}}{2}\unicode[STIX]{x1D6FC}^{2}+\frac{n(n-2)}{2}\unicode[STIX]{x1D6FD}^{2}\right)\,dt\quad & \text{if }n\text{ is even}\\ \left(-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+n(n-1)\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\right)\,dt\quad & \text{if }n\text{ is odd}\end{array}\right. & & \displaystyle \nonumber\end{eqnarray}$$which simplifies to
 $$\begin{eqnarray}-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+\left\{\begin{array}{@{}ll@{}}\left({\displaystyle \frac{n^{2}}{2}}\unicode[STIX]{x1D6FC}^{2}+{\displaystyle \frac{n(n-2)}{2}}\unicode[STIX]{x1D6FD}^{2}\right)\,dt\quad & \text{if }n\text{ is even}\\ \left(n(n-1)\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\right)\,dt\quad & \text{if }n\text{ is odd}\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}-n\displaystyle \mathop{\sum }_{k=1}^{n-1}\unicode[STIX]{x1D70F}(A_{t}^{k})\unicode[STIX]{x1D70F}(A_{t}^{n-k})+\left\{\begin{array}{@{}ll@{}}\left({\displaystyle \frac{n^{2}}{2}}\unicode[STIX]{x1D6FC}^{2}+{\displaystyle \frac{n(n-2)}{2}}\unicode[STIX]{x1D6FD}^{2}\right)\,dt\quad & \text{if }n\text{ is even}\\ \left(n(n-1)\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\right)\,dt\quad & \text{if }n\text{ is odd}\end{array}\right.\end{eqnarray}$$and hence the desired assertions follow after summing (2.5) and (2.8).◻
2.2 The Herglotz transform of  $\unicode[STIX]{x1D708}_{t}$
$\unicode[STIX]{x1D708}_{t}$
Here, we derive a PDE governing the Herglotz transform of the spectral measure ![]() $\unicode[STIX]{x1D708}_{t}$:
$\unicode[STIX]{x1D708}_{t}$:
Recall that, this is an analytic function on ![]() $\mathbb{D}$ (the open unit disc of
$\mathbb{D}$ (the open unit disc of ![]() $\mathbb{C}$).
$\mathbb{C}$).
Proposition 2.3. The function ![]() $H(t,z)$ satisfies the PDE
$H(t,z)$ satisfies the PDE
Proof. By direct calculation from Proposition 2.1, we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x2202}_{t}H & = & \displaystyle 2\mathop{\sum }_{n\geqslant 1}\unicode[STIX]{x2202}_{t}f_{n}(t)z^{n}\nonumber\\ \displaystyle & = & \displaystyle -2\mathop{\sum }_{n\geqslant 1}nf_{n}z^{n}-2\mathop{\sum }_{n\geqslant 1}n\mathop{\sum }_{k=1}^{n-1}f_{k}f_{n-k}z^{n}+(\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2})\mathop{\sum }_{n\geqslant 1,~n~\text{even}}n^{2}z^{n}\nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\mathop{\sum }_{n\geqslant 1,~n~\text{odd}}n^{2}z^{n}\nonumber\\ \displaystyle & = & \displaystyle -z\unicode[STIX]{x2202}_{z}H-2\mathop{\sum }_{k\geqslant 1}f_{k}z^{k}\mathop{\sum }_{n\geqslant k+1}nf_{n-k}z^{n-k}+4(\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2})\frac{z^{2}(1+z^{2})}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}z\frac{1+6z^{2}+z^{4}}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & = & \displaystyle -z\unicode[STIX]{x2202}_{z}H-4z\frac{H-1}{2}\frac{\unicode[STIX]{x2202}_{z}H}{2}+\frac{2z(\unicode[STIX]{x1D6FC}z^{2}+2\unicode[STIX]{x1D6FD}z+\unicode[STIX]{x1D6FC})(\unicode[STIX]{x1D6FD}z^{2}+2\unicode[STIX]{x1D6FC}z+\unicode[STIX]{x1D6FD})}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & = & \displaystyle -zH\unicode[STIX]{x2202}_{z}H+\frac{2z(\unicode[STIX]{x1D6FC}z^{2}+2\unicode[STIX]{x1D6FD}z+\unicode[STIX]{x1D6FC})(\unicode[STIX]{x1D6FD}z^{2}+2\unicode[STIX]{x1D6FC}z+\unicode[STIX]{x1D6FD})}{(1-z^{2})^{3}}.\square\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x2202}_{t}H & = & \displaystyle 2\mathop{\sum }_{n\geqslant 1}\unicode[STIX]{x2202}_{t}f_{n}(t)z^{n}\nonumber\\ \displaystyle & = & \displaystyle -2\mathop{\sum }_{n\geqslant 1}nf_{n}z^{n}-2\mathop{\sum }_{n\geqslant 1}n\mathop{\sum }_{k=1}^{n-1}f_{k}f_{n-k}z^{n}+(\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2})\mathop{\sum }_{n\geqslant 1,~n~\text{even}}n^{2}z^{n}\nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\mathop{\sum }_{n\geqslant 1,~n~\text{odd}}n^{2}z^{n}\nonumber\\ \displaystyle & = & \displaystyle -z\unicode[STIX]{x2202}_{z}H-2\mathop{\sum }_{k\geqslant 1}f_{k}z^{k}\mathop{\sum }_{n\geqslant k+1}nf_{n-k}z^{n-k}+4(\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2})\frac{z^{2}(1+z^{2})}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}z\frac{1+6z^{2}+z^{4}}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & = & \displaystyle -z\unicode[STIX]{x2202}_{z}H-4z\frac{H-1}{2}\frac{\unicode[STIX]{x2202}_{z}H}{2}+\frac{2z(\unicode[STIX]{x1D6FC}z^{2}+2\unicode[STIX]{x1D6FD}z+\unicode[STIX]{x1D6FC})(\unicode[STIX]{x1D6FD}z^{2}+2\unicode[STIX]{x1D6FC}z+\unicode[STIX]{x1D6FD})}{(1-z^{2})^{3}}\nonumber\\ \displaystyle & = & \displaystyle -zH\unicode[STIX]{x2202}_{z}H+\frac{2z(\unicode[STIX]{x1D6FC}z^{2}+2\unicode[STIX]{x1D6FD}z+\unicode[STIX]{x1D6FC})(\unicode[STIX]{x1D6FD}z^{2}+2\unicode[STIX]{x1D6FC}z+\unicode[STIX]{x1D6FD})}{(1-z^{2})^{3}}.\square\nonumber\end{eqnarray}$$2.3 Steady-state solution
As mentioned in the Introduction, it is known from the asymptotic freeness of ![]() $P$ and
$P$ and ![]() $U_{t}QU_{t}^{\ast }$ that
$U_{t}QU_{t}^{\ast }$ that
Proposition 2.4. The spectral measure ![]() $\unicode[STIX]{x1D708}_{t}$ of
$\unicode[STIX]{x1D708}_{t}$ of ![]() $RU_{t}SU_{t}^{\ast }$ converges weakly, as
$RU_{t}SU_{t}^{\ast }$ converges weakly, as ![]() $t\rightarrow \infty$, to the free multiplicative convolution of the spectral measures of
$t\rightarrow \infty$, to the free multiplicative convolution of the spectral measures of ![]() $R$ and
$R$ and ![]() $USU^{\ast }$, where
$USU^{\ast }$, where ![]() $U\in \mathscr{A}$ is a Haar unitary operator free from
$U\in \mathscr{A}$ is a Haar unitary operator free from ![]() $\{R,S\}$.
$\{R,S\}$.
We will see this directly from the PDE (2.9). Let ![]() $H(\infty ,.)$ be the state solution of (2.9), then it satisfies
$H(\infty ,.)$ be the state solution of (2.9), then it satisfies
After integration and taking into account ![]() $H(\infty ,0)=1$, we get
$H(\infty ,0)=1$, we get
where the principal branch of the square root is taken. On the other hand, the next technical proposition gives an explicit calculation for the Herglotz transform of ![]() $\unicode[STIX]{x1D708}_{R}\boxtimes \unicode[STIX]{x1D708}_{S}$.
$\unicode[STIX]{x1D708}_{R}\boxtimes \unicode[STIX]{x1D708}_{S}$.
Proposition 2.5. Let ![]() $\unicode[STIX]{x1D707}=((1+\unicode[STIX]{x1D6FC})/2)\unicode[STIX]{x1D6FF}_{1}+((1-\unicode[STIX]{x1D6FC})/2)\unicode[STIX]{x1D6FF}_{-1}$ and
$\unicode[STIX]{x1D707}=((1+\unicode[STIX]{x1D6FC})/2)\unicode[STIX]{x1D6FF}_{1}+((1-\unicode[STIX]{x1D6FC})/2)\unicode[STIX]{x1D6FF}_{-1}$ and
for ![]() $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}\in (-1,1]$. Then the Herglotz transform of
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}\in (-1,1]$. Then the Herglotz transform of ![]() $\unicode[STIX]{x1D708}$ is given by
$\unicode[STIX]{x1D708}$ is given by
Proof. Using the analytic machinery for multiplicative convolution (see [Reference Dykema, Nica and Voiculescu10]), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D713}_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{z(z+\unicode[STIX]{x1D6FC})}{1-z^{2}},\nonumber\\ \displaystyle \unicode[STIX]{x1D712}_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}}{2(z+1)},\nonumber\\ \displaystyle S_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}}{2z}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D713}_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{z(z+\unicode[STIX]{x1D6FC})}{1-z^{2}},\nonumber\\ \displaystyle \unicode[STIX]{x1D712}_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}}{2(z+1)},\nonumber\\ \displaystyle S_{\unicode[STIX]{x1D707}}(z) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}}{2z}.\nonumber\end{eqnarray}$$So that
 $$\begin{eqnarray}\displaystyle S_{\unicode[STIX]{x1D708}}(z) & = & \displaystyle \frac{\left(-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}\right)\left(-\unicode[STIX]{x1D6FD}\pm \sqrt{\unicode[STIX]{x1D6FD}^{2}+4z(z+1)}\right)}{4z^{2}},\nonumber\\ \displaystyle \unicode[STIX]{x1D712}_{\unicode[STIX]{x1D708}}(z) & = & \displaystyle \frac{\left(-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}\right)\left(-\unicode[STIX]{x1D6FD}\pm \sqrt{\unicode[STIX]{x1D6FD}^{2}+4z(z+1)}\right)}{4z(z+1)},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle S_{\unicode[STIX]{x1D708}}(z) & = & \displaystyle \frac{\left(-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}\right)\left(-\unicode[STIX]{x1D6FD}\pm \sqrt{\unicode[STIX]{x1D6FD}^{2}+4z(z+1)}\right)}{4z^{2}},\nonumber\\ \displaystyle \unicode[STIX]{x1D712}_{\unicode[STIX]{x1D708}}(z) & = & \displaystyle \frac{\left(-\unicode[STIX]{x1D6FC}\pm \sqrt{\unicode[STIX]{x1D6FC}^{2}+4z(z+1)}\right)\left(-\unicode[STIX]{x1D6FD}\pm \sqrt{\unicode[STIX]{x1D6FD}^{2}+4z(z+1)}\right)}{4z(z+1)},\nonumber\end{eqnarray}$$ and ![]() $\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}$ satisfies
$\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}$ satisfies
Letting ![]() $\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}=\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}(\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}+1)$, we get
$\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}=\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}(\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}+1)$, we get ![]() $\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}=(-1\pm \sqrt{1+4\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}})/2$ and since the Herglotz transform has a positive real part,
$\unicode[STIX]{x1D713}_{\unicode[STIX]{x1D708}}=(-1\pm \sqrt{1+4\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}})/2$ and since the Herglotz transform has a positive real part, ![]() $H_{\unicode[STIX]{x1D708}}=\sqrt{1+4\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}}$ where
$H_{\unicode[STIX]{x1D708}}=\sqrt{1+4\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}}$ where ![]() $\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}$ is given by
$\unicode[STIX]{x1D711}_{\unicode[STIX]{x1D708}}$ is given by
Or equivalently
Rearranging this last equality and raising it to the square, we get
So we raise it to the square once again, to get
Which simplifies to
Finally,
as desired. ◻
The next proposition provides a Lebesgue decomposition of the spectral measure ![]() $\unicode[STIX]{x1D708}_{\infty }$.
$\unicode[STIX]{x1D708}_{\infty }$.
Proposition 2.6. One has
with
 $$\begin{eqnarray}\displaystyle & & \displaystyle a=\frac{|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2},\qquad b=\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2},\qquad r_{\pm }=-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\pm \sqrt{(1-\unicode[STIX]{x1D6FC}^{2})(1-\unicode[STIX]{x1D6FD}^{2})}\nonumber\\ \displaystyle & & \displaystyle \qquad \text{and}\qquad \unicode[STIX]{x1D703}_{\pm }=\arccos r_{\pm }.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle a=\frac{|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2},\qquad b=\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2},\qquad r_{\pm }=-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\pm \sqrt{(1-\unicode[STIX]{x1D6FC}^{2})(1-\unicode[STIX]{x1D6FD}^{2})}\nonumber\\ \displaystyle & & \displaystyle \qquad \text{and}\qquad \unicode[STIX]{x1D703}_{\pm }=\arccos r_{\pm }.\nonumber\end{eqnarray}$$Proof. Writing (2.10) as
it follows that ![]() $H(\infty ,.)$ admits two simple poles at
$H(\infty ,.)$ admits two simple poles at ![]() $z=1$ and
$z=1$ and ![]() $z=-1$. So that, the decomposition of
$z=-1$. So that, the decomposition of ![]() $\unicode[STIX]{x1D708}_{\infty }$ is given by
$\unicode[STIX]{x1D708}_{\infty }$ is given by
where ![]() $d\unicode[STIX]{x1D703}$ denotes the (no-normalized) Lebesgue measure on
$d\unicode[STIX]{x1D703}$ denotes the (no-normalized) Lebesgue measure on ![]() $\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ and
$\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ and ![]() $a,b$ are the residue of
$a,b$ are the residue of ![]() $\frac{1}{2}H(\infty ,.)$ at
$\frac{1}{2}H(\infty ,.)$ at ![]() $-1,1$. Thus, we have
$-1,1$. Thus, we have
 $$\begin{eqnarray}\displaystyle a & = & \displaystyle \lim _{z\rightarrow -1}\frac{\sqrt{(1-z^{2})^{2}+4z[\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+z)^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}z]}}{2(1+z)}=\frac{|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2},\nonumber\\ \displaystyle b & = & \displaystyle \lim _{z\rightarrow 1}\frac{\sqrt{(1-z^{2})^{2}+4z[\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+z)^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}z]}}{2(1-z)}=\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2}\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle a & = & \displaystyle \lim _{z\rightarrow -1}\frac{\sqrt{(1-z^{2})^{2}+4z[\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+z)^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}z]}}{2(1+z)}=\frac{|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2},\nonumber\\ \displaystyle b & = & \displaystyle \lim _{z\rightarrow 1}\frac{\sqrt{(1-z^{2})^{2}+4z[\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+z)^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}z]}}{2(1-z)}=\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2}\nonumber\end{eqnarray}$$and the density is given by direct calculation
 $$\begin{eqnarray}\displaystyle \Re [H(\infty ,e^{i\unicode[STIX]{x1D703}})] & = & \displaystyle \Re \left[\sqrt{1+4e^{i\unicode[STIX]{x1D703}}\frac{\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+e^{i\unicode[STIX]{x1D703}})^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}e^{i\unicode[STIX]{x1D703}}}{(1-e^{2i\unicode[STIX]{x1D703}})^{2}}}\right]\nonumber\\ \displaystyle & = & \displaystyle \Re \left[\sqrt{1+\frac{4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}e^{i\unicode[STIX]{x1D703}}}{(1-e^{i\unicode[STIX]{x1D703}})^{2}}+\frac{4(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}e^{2i\unicode[STIX]{x1D703}}}{(1-e^{2i\unicode[STIX]{x1D703}})^{2}}}\right]\nonumber\\ \displaystyle & = & \displaystyle \sqrt{1-\frac{\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}}{\sin ^{2}(\unicode[STIX]{x1D703}/2)}-\frac{(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{\sin ^{2}\unicode[STIX]{x1D703}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{\sqrt{ sin^{2}\unicode[STIX]{x1D703}-4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos ^{2}(\unicode[STIX]{x1D703}/2)-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}}{|\text{sin}\unicode[STIX]{x1D703}|},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \Re [H(\infty ,e^{i\unicode[STIX]{x1D703}})] & = & \displaystyle \Re \left[\sqrt{1+4e^{i\unicode[STIX]{x1D703}}\frac{\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}(1+e^{i\unicode[STIX]{x1D703}})^{2}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}e^{i\unicode[STIX]{x1D703}}}{(1-e^{2i\unicode[STIX]{x1D703}})^{2}}}\right]\nonumber\\ \displaystyle & = & \displaystyle \Re \left[\sqrt{1+\frac{4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}e^{i\unicode[STIX]{x1D703}}}{(1-e^{i\unicode[STIX]{x1D703}})^{2}}+\frac{4(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}e^{2i\unicode[STIX]{x1D703}}}{(1-e^{2i\unicode[STIX]{x1D703}})^{2}}}\right]\nonumber\\ \displaystyle & = & \displaystyle \sqrt{1-\frac{\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}}{\sin ^{2}(\unicode[STIX]{x1D703}/2)}-\frac{(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{\sin ^{2}\unicode[STIX]{x1D703}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{\sqrt{ sin^{2}\unicode[STIX]{x1D703}-4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos ^{2}(\unicode[STIX]{x1D703}/2)-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}}{|\text{sin}\unicode[STIX]{x1D703}|},\nonumber\end{eqnarray}$$where we have used in the last equality the relation
Finally, by use of the basic trigonometric identities:
the denominator rewrites as
 $$\begin{eqnarray}\displaystyle \sin ^{2}\unicode[STIX]{x1D703}-4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos ^{2}\frac{\unicode[STIX]{x1D703}}{2}-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2} & = & \displaystyle 1-\cos ^{2}\unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos \unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}\nonumber\\ \displaystyle & = & \displaystyle -\cos ^{2}\unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos \unicode[STIX]{x1D703}+1-\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \sin ^{2}\unicode[STIX]{x1D703}-4\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos ^{2}\frac{\unicode[STIX]{x1D703}}{2}-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2} & = & \displaystyle 1-\cos ^{2}\unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos \unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}-(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}\nonumber\\ \displaystyle & = & \displaystyle -\cos ^{2}\unicode[STIX]{x1D703}-2\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}\cos \unicode[STIX]{x1D703}+1-\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2}.\nonumber\end{eqnarray}$$ Using the discriminant ![]() $\unicode[STIX]{x1D6E5}=4(\unicode[STIX]{x1D6FC}^{2}\unicode[STIX]{x1D6FD}^{2}+1-\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2})=4(1-\unicode[STIX]{x1D6FC}^{2})(1-\unicode[STIX]{x1D6FD}^{2})\geqslant 0$, we get the factorization
$\unicode[STIX]{x1D6E5}=4(\unicode[STIX]{x1D6FC}^{2}\unicode[STIX]{x1D6FD}^{2}+1-\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2})=4(1-\unicode[STIX]{x1D6FC}^{2})(1-\unicode[STIX]{x1D6FD}^{2})\geqslant 0$, we get the factorization ![]() $-(\cos \unicode[STIX]{x1D703}-r_{+})(\cos \unicode[STIX]{x1D703}-r_{-})$ with
$-(\cos \unicode[STIX]{x1D703}-r_{+})(\cos \unicode[STIX]{x1D703}-r_{-})$ with
Remark 2.7. It should be noted that this measure appears in [Reference Hiai and Petz13, Example 4.5] as the distribution of ![]() $e^{i\unicode[STIX]{x1D70B}P}e^{-i\unicode[STIX]{x1D70B}Q}$ for a pair of free projections
$e^{i\unicode[STIX]{x1D70B}P}e^{-i\unicode[STIX]{x1D70B}Q}$ for a pair of free projections ![]() $\{P,Q\}$ in
$\{P,Q\}$ in ![]() $\mathscr{A}$. In particular, when
$\mathscr{A}$. In particular, when ![]() $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FD}=0$ (i.e.,
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FD}=0$ (i.e., ![]() $\unicode[STIX]{x1D70F}(P)=\unicode[STIX]{x1D70F}(Q)=1/2$), it coincides with the uniform measure on
$\unicode[STIX]{x1D70F}(P)=\unicode[STIX]{x1D70F}(Q)=1/2$), it coincides with the uniform measure on ![]() $\mathbb{T}$.
$\mathbb{T}$.
3 Relationship between  $\unicode[STIX]{x1D707}_{t}$ and
$\unicode[STIX]{x1D707}_{t}$ and  $\unicode[STIX]{x1D708}_{t}$
$\unicode[STIX]{x1D708}_{t}$
Keep the symbols ![]() $P,Q,R,S,\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},a,b$ and
$P,Q,R,S,\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},a,b$ and ![]() $\unicode[STIX]{x1D707}_{t},\unicode[STIX]{x1D708}_{t}$ as above. In what follows
$\unicode[STIX]{x1D707}_{t},\unicode[STIX]{x1D708}_{t}$ as above. In what follows ![]() $P,Q$ and
$P,Q$ and ![]() $R,S$ are associated. Our goal here is to derive a relationship between
$R,S$ are associated. Our goal here is to derive a relationship between ![]() $\unicode[STIX]{x1D707}_{t}$ and
$\unicode[STIX]{x1D707}_{t}$ and ![]() $\unicode[STIX]{x1D708}_{t}$ and give more detailed properties of
$\unicode[STIX]{x1D708}_{t}$ and give more detailed properties of ![]() $\unicode[STIX]{x1D708}_{t}$. Here is a relationship between the corresponding sequence of moments.
$\unicode[STIX]{x1D708}_{t}$. Here is a relationship between the corresponding sequence of moments.
Proposition 3.1. For any ![]() $n\geqslant 1$, one has:
$n\geqslant 1$, one has:
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70F}([PU_{t}QU_{t}^{\ast }P]^{n}) & = & \displaystyle \frac{1}{2^{2n+1}}\binom{2n}{n}+\frac{\unicode[STIX]{x1D70F}(R+S)}{4}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{1}{2^{2n}}\mathop{\sum }_{k=1}^{n}\binom{2n}{n-k}\unicode[STIX]{x1D70F}((RU_{t}SU_{t}^{\ast })^{k}).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70F}([PU_{t}QU_{t}^{\ast }P]^{n}) & = & \displaystyle \frac{1}{2^{2n+1}}\binom{2n}{n}+\frac{\unicode[STIX]{x1D70F}(R+S)}{4}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{1}{2^{2n}}\mathop{\sum }_{k=1}^{n}\binom{2n}{n-k}\unicode[STIX]{x1D70F}((RU_{t}SU_{t}^{\ast })^{k}).\end{eqnarray}$$Proof. Since ![]() $P$ is idempotent and since
$P$ is idempotent and since ![]() $\unicode[STIX]{x1D70F}$ is a trace, we write
$\unicode[STIX]{x1D70F}$ is a trace, we write
Let ![]() $\tilde{S}:=U_{t}SU_{t}^{\ast }$. Then writing
$\tilde{S}:=U_{t}SU_{t}^{\ast }$. Then writing
one easily can see that the same enumeration techniques used in [Reference Demni, Hamdi and Hmidi7, Proposition 4.1] to expand ![]() $\unicode[STIX]{x1D70F}[((\mathbf{1}+R)(\mathbf{1}+\tilde{S}))^{n}]$ remain valid, but here we will take into account the contribution of words formed by an odd number of letters. Using the trace property and the relations
$\unicode[STIX]{x1D70F}[((\mathbf{1}+R)(\mathbf{1}+\tilde{S}))^{n}]$ remain valid, but here we will take into account the contribution of words formed by an odd number of letters. Using the trace property and the relations ![]() $R^{2}=\tilde{S}^{2}=\mathbf{1}$, this contribution is
$R^{2}=\tilde{S}^{2}=\mathbf{1}$, this contribution is ![]() $\unicode[STIX]{x1D70F}(R)+\unicode[STIX]{x1D70F}(S)$ up to a positive integer
$\unicode[STIX]{x1D70F}(R)+\unicode[STIX]{x1D70F}(S)$ up to a positive integer ![]() $N$. By letting
$N$. By letting ![]() $R=S$ and using the expansion in [Reference Demni, Hamdi and Hmidi7, p. 1366], we get
$R=S$ and using the expansion in [Reference Demni, Hamdi and Hmidi7, p. 1366], we get ![]() $2N=2^{2n-1}$ and hence the desired equality follows.◻
$2N=2^{2n-1}$ and hence the desired equality follows.◻
Let
be the Cauchy transform of the process ![]() $X_{t}$. The following corollary gives a relationship between
$X_{t}$. The following corollary gives a relationship between ![]() $G$ and the Herglotz transform of
$G$ and the Herglotz transform of ![]() $\unicode[STIX]{x1D708}_{t}$.
$\unicode[STIX]{x1D708}_{t}$.
Corollary 3.2. One has
whereFootnote 1
Proof. We will prove the following equivalent relation
satisfied by the moment generating function of the process ![]() $X_{t}$
$X_{t}$
Before going into the details, recall from [Reference Demni, Hamdi and Hmidi7, p. 1359] that ![]() $|g(1/z)|\leqslant |z|<1$ in the open unit disc, then this last relation makes sense for all
$|g(1/z)|\leqslant |z|<1$ in the open unit disc, then this last relation makes sense for all ![]() $|z|<1$. Now multiplying (3.1) by
$|z|<1$. Now multiplying (3.1) by ![]() $z^{n}$ and summing over
$z^{n}$ and summing over ![]() $n\geqslant 1$, we get
$n\geqslant 1$, we get
But, this last term rewrites, after permutation of sums and reindexing ![]() $j=n-k$, as
$j=n-k$, as
 $$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{n\geqslant 1}\frac{z^{n}}{2^{2n}}\mathop{\sum }_{k=1}^{n}\binom{2n}{n-k}\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{k\geqslant 1}\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]\mathop{\sum }_{j\geqslant 0}\frac{z^{j+k}}{2^{2j+2k}}\binom{2j+2k}{j}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{n\geqslant 1}\frac{z^{n}}{2^{2n}}\mathop{\sum }_{k=1}^{n}\binom{2n}{n-k}\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{k\geqslant 1}\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]\mathop{\sum }_{j\geqslant 0}\frac{z^{j+k}}{2^{2j+2k}}\binom{2j+2k}{j}.\nonumber\end{eqnarray}$$Using the identity (see, e.g., [Reference Demni, Hamdi and Hmidi7])
we get
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D713}_{\unicode[STIX]{x1D707}_{t}}(z) & = & \displaystyle \frac{1}{2\sqrt{1-z}}-\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{1}{\sqrt{1-z}}\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]z^{k}}{(1+\sqrt{1-z})^{2k}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2\sqrt{1-z}}-\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{1}{\sqrt{1-z}}\frac{H(t,g(1/z))-1}{2}\nonumber\\ \displaystyle & = & \displaystyle -\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{H(t,g(1/z))}{2\sqrt{1-z}},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D713}_{\unicode[STIX]{x1D707}_{t}}(z) & = & \displaystyle \frac{1}{2\sqrt{1-z}}-\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{1}{\sqrt{1-z}}\mathop{\sum }_{k\geqslant 1}\frac{\unicode[STIX]{x1D70F}[(RU_{t}SU_{t}^{\ast })^{k}]z^{k}}{(1+\sqrt{1-z})^{2k}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2\sqrt{1-z}}-\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{1}{\sqrt{1-z}}\frac{H(t,g(1/z))-1}{2}\nonumber\\ \displaystyle & = & \displaystyle -\frac{1}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})z}{4(1-z)}+\frac{H(t,g(1/z))}{2\sqrt{1-z}},\nonumber\end{eqnarray}$$which proves the corollary. ◻
We are now ready to prove the relationship between the spectral measure of ![]() $X_{t}$ and
$X_{t}$ and ![]() $Y_{t}$:
$Y_{t}$: ![]() $\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$.
$\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$.
Theorem 3.3. Let ![]() $\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})$ be the positive measure on
$\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})$ be the positive measure on ![]() $[0,\unicode[STIX]{x1D70B}]$ obtained from
$[0,\unicode[STIX]{x1D70B}]$ obtained from ![]() $\unicode[STIX]{x1D707}_{t}(dx)$ via the variable change
$\unicode[STIX]{x1D707}_{t}(dx)$ via the variable change ![]() $x=\cos ^{2}(\unicode[STIX]{x1D703}/2)$ and
$x=\cos ^{2}(\unicode[STIX]{x1D703}/2)$ and
its symmetrization on ![]() $(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B})$ with the mapping
$(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B})$ with the mapping ![]() $j:\unicode[STIX]{x1D703}\in (0,\unicode[STIX]{x1D70B})\mapsto -\unicode[STIX]{x1D703}\in (-\unicode[STIX]{x1D70B},0)$. Then, the two measures
$j:\unicode[STIX]{x1D703}\in (0,\unicode[STIX]{x1D70B})\mapsto -\unicode[STIX]{x1D703}\in (-\unicode[STIX]{x1D70B},0)$. Then, the two measures ![]() $\unicode[STIX]{x1D707}_{t}$ and
$\unicode[STIX]{x1D707}_{t}$ and ![]() $\unicode[STIX]{x1D708}_{t}$ are related via
$\unicode[STIX]{x1D708}_{t}$ are related via
Proof. By (3.2), we have
Letting ![]() $\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})=\unicode[STIX]{x1D707}_{t}(dx)$ with
$\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})=\unicode[STIX]{x1D707}_{t}(dx)$ with ![]() $x=\cos ^{2}(\unicode[STIX]{x1D703}/2),\unicode[STIX]{x1D703}\in [0,\unicode[STIX]{x1D70B}]$, we get
$x=\cos ^{2}(\unicode[STIX]{x1D703}/2),\unicode[STIX]{x1D703}\in [0,\unicode[STIX]{x1D70B}]$, we get
 $$\begin{eqnarray}\displaystyle H(t,g(z)) & = & \displaystyle -2\sqrt{z^{2}-z}\left(\int _{0}^{\unicode[STIX]{x1D70B}}\frac{1}{z-\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{4z}-\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{4(z-1)}\right).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle H(t,g(z)) & = & \displaystyle -2\sqrt{z^{2}-z}\left(\int _{0}^{\unicode[STIX]{x1D70B}}\frac{1}{z-\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{4z}-\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{4(z-1)}\right).\nonumber\end{eqnarray}$$Next, we perform the variable change
to get
 $$\begin{eqnarray}\displaystyle H(t,\unicode[STIX]{x1D701}) & = & \displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{2}\left(\int _{0}^{\unicode[STIX]{x1D70B}}\frac{1}{\frac{(2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1})}{4}-\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}}\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{-2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}}\vphantom{\frac{1}{\frac{(2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1})}{4}-\cos ^{2}(\unicode[STIX]{x1D703}/2)}}\right)\nonumber\\ \displaystyle & = & \displaystyle \int _{0}^{\unicode[STIX]{x1D70B}}\frac{2(\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701})}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-4\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{(2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})(1-\unicode[STIX]{x1D701})}{2(1+\unicode[STIX]{x1D701})}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})(1+\unicode[STIX]{x1D701})}{2(1-\unicode[STIX]{x1D701})}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle H(t,\unicode[STIX]{x1D701}) & = & \displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{2}\left(\int _{0}^{\unicode[STIX]{x1D70B}}\frac{1}{\frac{(2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1})}{4}-\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}}\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{-2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}}\vphantom{\frac{1}{\frac{(2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1})}{4}-\cos ^{2}(\unicode[STIX]{x1D703}/2)}}\right)\nonumber\\ \displaystyle & = & \displaystyle \int _{0}^{\unicode[STIX]{x1D70B}}\frac{2(\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701})}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-4\cos ^{2}(\unicode[STIX]{x1D703}/2)}\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{(2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})(1-\unicode[STIX]{x1D701})}{2(1+\unicode[STIX]{x1D701})}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})(1+\unicode[STIX]{x1D701})}{2(1-\unicode[STIX]{x1D701})}.\nonumber\end{eqnarray}$$But since
 $$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-4\cos ^{2}(\unicode[STIX]{x1D703}/2)} & = & \displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-2\cos \unicode[STIX]{x1D703}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1-\unicode[STIX]{x1D701}^{2}}{\unicode[STIX]{x1D701}^{2}-2\unicode[STIX]{x1D701}\cos \unicode[STIX]{x1D703}+1}\nonumber\\ \displaystyle & = & \displaystyle \frac{e^{i\unicode[STIX]{x1D703}}}{e^{i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}+\frac{e^{i\unicode[STIX]{x1D703}}}{e^{-i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}-1,\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{2+\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-4\cos ^{2}(\unicode[STIX]{x1D703}/2)} & = & \displaystyle \frac{\unicode[STIX]{x1D701}^{-1}-\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{-1}-2\cos \unicode[STIX]{x1D703}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1-\unicode[STIX]{x1D701}^{2}}{\unicode[STIX]{x1D701}^{2}-2\unicode[STIX]{x1D701}\cos \unicode[STIX]{x1D703}+1}\nonumber\\ \displaystyle & = & \displaystyle \frac{e^{i\unicode[STIX]{x1D703}}}{e^{i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}+\frac{e^{i\unicode[STIX]{x1D703}}}{e^{-i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}-1,\nonumber\end{eqnarray}$$then
 $$\begin{eqnarray}\displaystyle H(t,\unicode[STIX]{x1D701}) & = & \displaystyle 2\int _{0}^{\unicode[STIX]{x1D70B}}\left(\frac{e^{i\unicode[STIX]{x1D703}}}{e^{i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}+\frac{e^{-i\unicode[STIX]{x1D703}}}{e^{-i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}-1\right)\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{(2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})(1-\unicode[STIX]{x1D701})}{2(1+\unicode[STIX]{x1D701})}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})(1+\unicode[STIX]{x1D701})}{2(1-\unicode[STIX]{x1D701})}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle H(t,\unicode[STIX]{x1D701}) & = & \displaystyle 2\int _{0}^{\unicode[STIX]{x1D70B}}\left(\frac{e^{i\unicode[STIX]{x1D703}}}{e^{i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}+\frac{e^{-i\unicode[STIX]{x1D703}}}{e^{-i\unicode[STIX]{x1D703}}-\unicode[STIX]{x1D701}}-1\right)\tilde{\unicode[STIX]{x1D707}}_{t}(d\unicode[STIX]{x1D703})-\frac{(2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})(1-\unicode[STIX]{x1D701})}{2(1+\unicode[STIX]{x1D701})}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})(1+\unicode[STIX]{x1D701})}{2(1-\unicode[STIX]{x1D701})}.\nonumber\end{eqnarray}$$ Thus, using the symmetrization ![]() $\hat{\unicode[STIX]{x1D707}}_{t}:={\textstyle \frac{1}{2}}(\tilde{\unicode[STIX]{x1D707}}_{t}+(\tilde{\unicode[STIX]{x1D707}}_{t}|_{(0,\unicode[STIX]{x1D70B})})\circ j^{-1})$ with
$\hat{\unicode[STIX]{x1D707}}_{t}:={\textstyle \frac{1}{2}}(\tilde{\unicode[STIX]{x1D707}}_{t}+(\tilde{\unicode[STIX]{x1D707}}_{t}|_{(0,\unicode[STIX]{x1D70B})})\circ j^{-1})$ with ![]() $j:\unicode[STIX]{x1D703}\in (0,\unicode[STIX]{x1D70B})\mapsto -\unicode[STIX]{x1D703}\in (-\unicode[STIX]{x1D70B},0)$, we get
$j:\unicode[STIX]{x1D703}\in (0,\unicode[STIX]{x1D70B})\mapsto -\unicode[STIX]{x1D703}\in (-\unicode[STIX]{x1D70B},0)$, we get
This proves the theorem. ◻
Remark 3.4. The relationship ![]() $\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$ enables us, in particular, to retrieve the decomposition of
$\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$ enables us, in particular, to retrieve the decomposition of ![]() $\unicode[STIX]{x1D708}_{\infty }$ already obtained in Section 2 from the spectral measure
$\unicode[STIX]{x1D708}_{\infty }$ already obtained in Section 2 from the spectral measure ![]() $\unicode[STIX]{x1D707}_{\infty }$ (given by the free multiplicative convolution of the spectral measure of
$\unicode[STIX]{x1D707}_{\infty }$ (given by the free multiplicative convolution of the spectral measure of ![]() $P$ and
$P$ and ![]() $UQU^{\ast }$ with
$UQU^{\ast }$ with ![]() $U$ is a Haar unitary free from
$U$ is a Haar unitary free from ![]() $\{P,Q\}$ (see, [Reference Dykema, Nica and Voiculescu10, Example 3.6.7])). Indeed, we have
$\{P,Q\}$ (see, [Reference Dykema, Nica and Voiculescu10, Example 3.6.7])). Indeed, we have ![]() $\hat{\unicode[STIX]{x1D6FF}_{0}}=\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}},\hat{\unicode[STIX]{x1D6FF}_{1}}=\unicode[STIX]{x1D6FF}_{0}$ and if
$\hat{\unicode[STIX]{x1D6FF}_{0}}=\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}},\hat{\unicode[STIX]{x1D6FF}_{1}}=\unicode[STIX]{x1D6FF}_{0}$ and if ![]() $\unicode[STIX]{x1D707}_{t}$ has the density
$\unicode[STIX]{x1D707}_{t}$ has the density ![]() $h(x)$ with respect to
$h(x)$ with respect to ![]() $dx$ on
$dx$ on ![]() $[0,1]$, then
$[0,1]$, then ![]() $\unicode[STIX]{x1D708}_{t}$ has the density
$\unicode[STIX]{x1D708}_{t}$ has the density ![]() ${\hat{h}}(\unicode[STIX]{x1D703})$ with respect to the (no-normalized) Lebesgue measure
${\hat{h}}(\unicode[STIX]{x1D703})$ with respect to the (no-normalized) Lebesgue measure ![]() $d\unicode[STIX]{x1D703}$ on
$d\unicode[STIX]{x1D703}$ on ![]() $\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ with
$\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ with ![]() ${\hat{h}}(\unicode[STIX]{x1D703})=h(\cos ^{2}(\unicode[STIX]{x1D703}/2))|\sin \unicode[STIX]{x1D703}|/4$.
${\hat{h}}(\unicode[STIX]{x1D703})=h(\cos ^{2}(\unicode[STIX]{x1D703}/2))|\sin \unicode[STIX]{x1D703}|/4$.
Corollary 3.5. For every ![]() $t>0$ and
$t>0$ and ![]() $z\in \mathbb{D}$, we have
$z\in \mathbb{D}$, we have
where ![]() $L(t,z)$ is the function defined in [Reference Izumi and Ueda15, Section 3] by
$L(t,z)$ is the function defined in [Reference Izumi and Ueda15, Section 3] by
 $$\begin{eqnarray}\displaystyle & & \displaystyle \int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}\nonumber\\ \displaystyle & & \displaystyle \quad \times \,(\hat{\unicode[STIX]{x1D707}_{t}}-(1-\min \{\unicode[STIX]{x1D70F}(P),\unicode[STIX]{x1D70F}(Q)\})\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-\max \{\unicode[STIX]{x1D70F}(P)+\unicode[STIX]{x1D70F}(Q)-1,0\}\unicode[STIX]{x1D6FF}_{0})(d\unicode[STIX]{x1D703}).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}\nonumber\\ \displaystyle & & \displaystyle \quad \times \,(\hat{\unicode[STIX]{x1D707}_{t}}-(1-\min \{\unicode[STIX]{x1D70F}(P),\unicode[STIX]{x1D70F}(Q)\})\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-\max \{\unicode[STIX]{x1D70F}(P)+\unicode[STIX]{x1D70F}(Q)-1,0\}\unicode[STIX]{x1D6FF}_{0})(d\unicode[STIX]{x1D703}).\nonumber\end{eqnarray}$$Proof. We can easily check the result from (3.4) by substituting ![]() $\unicode[STIX]{x1D70F}(P)=(1+\unicode[STIX]{x1D6FC})/2$,
$\unicode[STIX]{x1D70F}(P)=(1+\unicode[STIX]{x1D6FC})/2$, ![]() $\unicode[STIX]{x1D70F}(Q)=(1+\unicode[STIX]{x1D6FD})/2$ into the expression of
$\unicode[STIX]{x1D70F}(Q)=(1+\unicode[STIX]{x1D6FD})/2$ into the expression of ![]() $L(t,z)$. Then, we obtain
$L(t,z)$. Then, we obtain
 $$\begin{eqnarray}\displaystyle L(t,z) & = & \displaystyle \frac{1}{2}\int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}(2\hat{\unicode[STIX]{x1D707}_{t}}-(1-\min \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}\})\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-\max \{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD},0\}\unicode[STIX]{x1D6FF}_{0})(d\unicode[STIX]{x1D703})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}\int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}\left(2\hat{\unicode[STIX]{x1D707}_{t}}-\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2}\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}+|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2}\unicode[STIX]{x1D6FF}_{0}\right)(d\unicode[STIX]{x1D703})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}\left(H(t,z)-a\frac{1-z}{1+z}-b\frac{1+z}{1-z}\right).\square\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle L(t,z) & = & \displaystyle \frac{1}{2}\int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}(2\hat{\unicode[STIX]{x1D707}_{t}}-(1-\min \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}\})\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-\max \{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD},0\}\unicode[STIX]{x1D6FF}_{0})(d\unicode[STIX]{x1D703})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}\int _{-\unicode[STIX]{x1D70B}}^{\unicode[STIX]{x1D70B}}\frac{e^{i\unicode[STIX]{x1D703}}+z}{e^{i\unicode[STIX]{x1D703}}-z}\left(2\hat{\unicode[STIX]{x1D707}_{t}}-\frac{2-\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2}\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}\right.\nonumber\\ \displaystyle & & \displaystyle -\left.\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}+|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|}{2}\unicode[STIX]{x1D6FF}_{0}\right)(d\unicode[STIX]{x1D703})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}\left(H(t,z)-a\frac{1-z}{1+z}-b\frac{1+z}{1-z}\right).\square\nonumber\end{eqnarray}$$Remark 3.6. Note that, from the PDE (3.2) of [Reference Izumi and Ueda15], one easily sees that the function
solves the same PDE (2.9) as ![]() $H(t,z)$ and therefore, by uniqueness of solutions in the class of analytic function on the unit disc, we deduce the result of Corollary 3.5. This means that Theorem 3.3 can quite easily be deduced from Proposition 2.3 and [Reference Izumi and Ueda15, Section 3]. Nevertheless, the moments formula presented in Proposition 3.1 is of independent interest as it explains the appearance of the operator
$H(t,z)$ and therefore, by uniqueness of solutions in the class of analytic function on the unit disc, we deduce the result of Corollary 3.5. This means that Theorem 3.3 can quite easily be deduced from Proposition 2.3 and [Reference Izumi and Ueda15, Section 3]. Nevertheless, the moments formula presented in Proposition 3.1 is of independent interest as it explains the appearance of the operator ![]() $RU_{t}SU_{t}^{\ast }$ and so it gives a more natural presentation of the result of Theorem 3.3.
$RU_{t}SU_{t}^{\ast }$ and so it gives a more natural presentation of the result of Theorem 3.3.
From Corollary 3.5, the measure ![]() $\unicode[STIX]{x1D70E}_{t}:=\unicode[STIX]{x1D708}_{t}-a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-b\unicode[STIX]{x1D6FF}_{0}$ rewrites as
$\unicode[STIX]{x1D70E}_{t}:=\unicode[STIX]{x1D708}_{t}-a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-b\unicode[STIX]{x1D6FF}_{0}$ rewrites as
Thus, by virtue of [Reference Izumi and Ueda15, Proposition 3.1], we get the following descriptions for the measure ![]() $\unicode[STIX]{x1D708}_{t}$.
$\unicode[STIX]{x1D708}_{t}$.
Corollary 3.7. For every ![]() $t>0$, the positive measure
$t>0$, the positive measure ![]() $\unicode[STIX]{x1D70E}_{t}$ has no atom at both 0 and
$\unicode[STIX]{x1D70E}_{t}$ has no atom at both 0 and ![]() $\unicode[STIX]{x1D70B}$. Moreover, at
$\unicode[STIX]{x1D70B}$. Moreover, at ![]() $t=0$, we have
$t=0$, we have ![]() $\unicode[STIX]{x1D70E}_{0}\{0\}\geqslant 0$ and
$\unicode[STIX]{x1D70E}_{0}\{0\}\geqslant 0$ and ![]() $\unicode[STIX]{x1D70E}_{0}\{\unicode[STIX]{x1D70B}\}\geqslant 0$ with equalities (i.e.,
$\unicode[STIX]{x1D70E}_{0}\{\unicode[STIX]{x1D70B}\}\geqslant 0$ with equalities (i.e., ![]() $\unicode[STIX]{x1D70E}_{0}$ has no atom at both 0 and
$\unicode[STIX]{x1D70E}_{0}$ has no atom at both 0 and ![]() $\unicode[STIX]{x1D70B}$), if and only if the projections
$\unicode[STIX]{x1D70B}$), if and only if the projections ![]() $P$ and
$P$ and ![]() $Q$ are in generic position.
$Q$ are in generic position.
4 Subordination for the liberation of symmetries
The aim of this section is to derive a subordination relation for the Herglotz transform ![]() $H(t,z)$ and give an explicit formula for its unique subordinate family. For every
$H(t,z)$ and give an explicit formula for its unique subordinate family. For every ![]() $t\geqslant 0$ and
$t\geqslant 0$ and ![]() $|z|<1$, define the functionFootnote 2
$|z|<1$, define the functionFootnote 2
This is an analytic function in ![]() $\mathbb{D}$ with positive real part. Indeed,
$\mathbb{D}$ with positive real part. Indeed,
cannot take negative value in ![]() $\mathbb{D}$ since the two measures
$\mathbb{D}$ since the two measures ![]() $\unicode[STIX]{x1D708}_{t}-a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-b\unicode[STIX]{x1D6FF}_{0}$ and
$\unicode[STIX]{x1D708}_{t}-a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}-b\unicode[STIX]{x1D6FF}_{0}$ and ![]() $\unicode[STIX]{x1D708}_{t}+a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}+b\unicode[STIX]{x1D6FF}_{0}$ are finite positive measure in
$\unicode[STIX]{x1D708}_{t}+a\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70B}}+b\unicode[STIX]{x1D6FF}_{0}$ are finite positive measure in ![]() $\mathbb{T}$ (see Corollary 3.7). Thus, according to the Herglotz theorem (see [Reference Cima, Matheson and Ross3, Theorem 1.8.9]),
$\mathbb{T}$ (see Corollary 3.7). Thus, according to the Herglotz theorem (see [Reference Cima, Matheson and Ross3, Theorem 1.8.9]), ![]() $K(t,z)$ has the following integral representation
$K(t,z)$ has the following integral representation
for some probability measure ![]() $\unicode[STIX]{x1D6FE}_{t}$ on
$\unicode[STIX]{x1D6FE}_{t}$ on ![]() $\mathbb{T}$. The following result is an immediate consequence of Corollary 3.5 and [Reference Izumi and Ueda15, Proposition 3.1].
$\mathbb{T}$. The following result is an immediate consequence of Corollary 3.5 and [Reference Izumi and Ueda15, Proposition 3.1].
Corollary 4.1. There exists a unique subordinate family of conformal self-maps ![]() $\unicode[STIX]{x1D702}_{t}$ on
$\unicode[STIX]{x1D702}_{t}$ on ![]() $\mathbb{D}$ such that the subordination relation
$\mathbb{D}$ such that the subordination relation
holds for any ![]() $z\in \mathbb{D}$.
$z\in \mathbb{D}$.
Set ![]() $\unicode[STIX]{x1D719}_{t}:\unicode[STIX]{x1D6FA}_{t}:=\unicode[STIX]{x1D702}_{t}(\mathbb{D}){\twoheadrightarrow}\mathbb{D}$ the inverse function of
$\unicode[STIX]{x1D719}_{t}:\unicode[STIX]{x1D6FA}_{t}:=\unicode[STIX]{x1D702}_{t}(\mathbb{D}){\twoheadrightarrow}\mathbb{D}$ the inverse function of ![]() $\unicode[STIX]{x1D702}_{t}$. Then,
$\unicode[STIX]{x1D702}_{t}$. Then, ![]() $\unicode[STIX]{x1D719}_{t}$ satisfies the radial Loewner ODE driven by the probability measure
$\unicode[STIX]{x1D719}_{t}$ satisfies the radial Loewner ODE driven by the probability measure ![]() $\unicode[STIX]{x1D708}_{t}$ (see [Reference Izumi and Ueda15, Equation (3.5)])
$\unicode[STIX]{x1D708}_{t}$ (see [Reference Izumi and Ueda15, Equation (3.5)])
The next proposition gives an explicit expression for the transformation ![]() $\unicode[STIX]{x1D719}_{t}$.
$\unicode[STIX]{x1D719}_{t}$.
Proposition 4.2. For any ![]() $t\geqslant 0$ and
$t\geqslant 0$ and ![]() $z\in \unicode[STIX]{x1D6FA}_{t}\cap \mathbb{R}$, we have
$z\in \unicode[STIX]{x1D6FA}_{t}\cap \mathbb{R}$, we have
with
where ![]() $c=c(z):=K(0,z)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}$ and
$c=c(z):=K(0,z)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}$ and
Proof. In order to make easier computations, we use the Möbius transform
to introduce the function ![]() $F(t,y):=H(t,z)$. Since
$F(t,y):=H(t,z)$. Since ![]() $dy/dz=2/(1-z)^{2}$, the PDE (2.9) becomes
$dy/dz=2/(1-z)^{2}$, the PDE (2.9) becomes
Then, the characteristic curve ![]() $t\mapsto (w_{t}(z),F(t,w_{t}(z)))$ associated with the PDE (4.4) satisfies the system of ODEs:
$t\mapsto (w_{t}(z),F(t,w_{t}(z)))$ associated with the PDE (4.4) satisfies the system of ODEs:
with
Combining the two last ODEs, we get
Hence, integrating with respect to ![]() $t$, we get
$t$, we get
 $$\begin{eqnarray}\displaystyle F(t,w_{t}(y))^{2} & = & \displaystyle F(0,y)^{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}y^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4y^{2}}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}\nonumber\\ \displaystyle & & \displaystyle +\,1-F(\infty ,y)^{2}-\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle F(t,w_{t}(y))^{2} & = & \displaystyle F(0,y)^{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}y^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4y^{2}}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}\nonumber\\ \displaystyle & & \displaystyle +\,1-F(\infty ,y)^{2}-\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}.\nonumber\end{eqnarray}$$Consequently, the RHS of (4.5) rewrites as
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{w_{t}(y)^{2}-1}{2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,\sqrt{1+F(0,y)^{2}-F(\infty ,y)^{2}-\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}}\nonumber\\ \displaystyle & & \displaystyle =\frac{w_{t}(y)^{2}-1}{2w_{t}(y)}\sqrt{b^{2}w_{t}(y)^{4}+[1+F(0,y)^{2}-F(\infty ,y)^{2}-a^{2}-b^{2}]w_{t}(y)^{2}+a^{2}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{w_{t}(y)^{2}-1}{2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,\sqrt{1+F(0,y)^{2}-F(\infty ,y)^{2}-\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}+\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}w_{t}(y)^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4w_{t}(y)^{2}}}\nonumber\\ \displaystyle & & \displaystyle =\frac{w_{t}(y)^{2}-1}{2w_{t}(y)}\sqrt{b^{2}w_{t}(y)^{4}+[1+F(0,y)^{2}-F(\infty ,y)^{2}-a^{2}-b^{2}]w_{t}(y)^{2}+a^{2}}.\nonumber\end{eqnarray}$$ Let ![]() $u_{t}(y):=1-w_{t}^{2}(y)$, and set
$u_{t}(y):=1-w_{t}^{2}(y)$, and set
 $$\begin{eqnarray}\displaystyle c & = & \displaystyle 1+F(0,y)^{2}-F(\infty ,y)^{2},\nonumber\\ \displaystyle c_{1} & = & \displaystyle c+b^{2}-a^{2}=c+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD},\nonumber\\ \displaystyle c_{2} & = & \displaystyle b^{2}=\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}}{4}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle c & = & \displaystyle 1+F(0,y)^{2}-F(\infty ,y)^{2},\nonumber\\ \displaystyle c_{1} & = & \displaystyle c+b^{2}-a^{2}=c+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD},\nonumber\\ \displaystyle c_{2} & = & \displaystyle b^{2}=\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}}{4}.\nonumber\end{eqnarray}$$Then, we transform the ODE (4.5) into
In order to solve this last ODE, we are lead to compute the indefinite integral
Since
 $$\begin{eqnarray}\displaystyle c & = & \displaystyle F(0,y)^{2}-\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}y^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4y^{2}}+\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}-\left(\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|y^{2}+|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2y}\right)^{2}+\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}+|\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2}|}{2}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}-\left(\frac{by^{2}+a}{y}\right)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}\nonumber\\ \displaystyle & = & \displaystyle K(0,z)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle c & = & \displaystyle F(0,y)^{2}-\frac{(\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD})^{2}y^{4}+(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4y^{2}}+\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}}{2}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}-\left(\frac{|\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}|y^{2}+|\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}|}{2y}\right)^{2}+\frac{\unicode[STIX]{x1D6FC}^{2}+\unicode[STIX]{x1D6FD}^{2}+|\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6FD}^{2}|}{2}\nonumber\\ \displaystyle & = & \displaystyle F(0,y)^{2}-\left(\frac{by^{2}+a}{y}\right)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}\nonumber\\ \displaystyle & = & \displaystyle K(0,z)^{2}+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\},\nonumber\end{eqnarray}$$we easily see that
Hence (see the proof in [Reference Demni and Hmidi8, Theorem 3]), we have
It follows that,
for some ![]() $d=d(y,\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD})$ and hence
$d=d(y,\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD})$ and hence
where ![]() $\unicode[STIX]{x1D716}$ is the sign of
$\unicode[STIX]{x1D716}$ is the sign of ![]() $u$. Raising this equality to the square and rearranging it, we get
$u$. Raising this equality to the square and rearranging it, we get
Equivalently,
with ![]() $\tilde{d}=\unicode[STIX]{x1D716}d$. Hence
$\tilde{d}=\unicode[STIX]{x1D716}d$. Hence
 $$\begin{eqnarray}\displaystyle w_{t}(y)^{2} & = & \displaystyle \frac{(c_{1}+\tilde{d}e^{t\sqrt{c}})^{2}-4cc_{2}-4c\tilde{d}e^{t\sqrt{c}}}{(c_{1}+\tilde{d}e^{t\sqrt{c}})^{2}-4cc_{2}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}-4c\tilde{d}e^{t\sqrt{c}}}{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(b^{2}-a^{2}-c+\tilde{d}e^{t\sqrt{c}})^{2}-4ca^{2}}{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle w_{t}(y)^{2} & = & \displaystyle \frac{(c_{1}+\tilde{d}e^{t\sqrt{c}})^{2}-4cc_{2}-4c\tilde{d}e^{t\sqrt{c}}}{(c_{1}+\tilde{d}e^{t\sqrt{c}})^{2}-4cc_{2}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}-4c\tilde{d}e^{t\sqrt{c}}}{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(b^{2}-a^{2}-c+\tilde{d}e^{t\sqrt{c}})^{2}-4ca^{2}}{(b^{2}-a^{2}+c+\tilde{d}e^{t\sqrt{c}})^{2}-4cb^{2}}.\nonumber\end{eqnarray}$$ Finally, in order to find the value of ![]() $\tilde{d}$, we check the equality (4.8) for
$\tilde{d}$, we check the equality (4.8) for ![]() $t=0$, we get
$t=0$, we get
where ![]() $u_{0}:=u_{0}(y)=1-w_{0}(y)^{2}=1-y^{2}$. From (4.5) and (4.7), we can see that
$u_{0}:=u_{0}(y)=1-w_{0}(y)^{2}=1-y^{2}$. From (4.5) and (4.7), we can see that
Thus,
 $$\begin{eqnarray}\displaystyle \tilde{d} & = & \displaystyle -c_{1}+\frac{2c-2\sqrt{c}\sqrt{c-c_{1}u_{0}+c_{2}u_{0}^{2}}}{u_{0}}\nonumber\\ \displaystyle & = & \displaystyle -c-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}+\frac{2c-2y\sqrt{c}F(0,y)}{1-y^{2}}.\square\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \tilde{d} & = & \displaystyle -c_{1}+\frac{2c-2\sqrt{c}\sqrt{c-c_{1}u_{0}+c_{2}u_{0}^{2}}}{u_{0}}\nonumber\\ \displaystyle & = & \displaystyle -c-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}+\frac{2c-2y\sqrt{c}F(0,y)}{1-y^{2}}.\square\nonumber\end{eqnarray}$$Remark 4.3. Let
This is an analytic one-to-one map from the open unit disc ![]() $\mathbb{D}$ onto the cut plane
$\mathbb{D}$ onto the cut plane ![]() $z\in \mathbb{C}\setminus [1,\infty [$ (see, e.g., [Reference Demni and Hamdi6]). Its inverse is given by:
$z\in \mathbb{C}\setminus [1,\infty [$ (see, e.g., [Reference Demni and Hamdi6]). Its inverse is given by:
where the principal branch of the square root is taken. Then, the flow ![]() $\unicode[STIX]{x1D719}_{t}$ rewrites as
$\unicode[STIX]{x1D719}_{t}$ rewrites as ![]() $\unicode[STIX]{x1D719}_{t}(z)=-h^{-1}(u_{t}(z))$, for real values of
$\unicode[STIX]{x1D719}_{t}(z)=-h^{-1}(u_{t}(z))$, for real values of ![]() $z\in \unicode[STIX]{x1D6FA}_{t}$, where
$z\in \unicode[STIX]{x1D6FA}_{t}$, where
Remark 4.4. From ![]() $u_{t}(z)=h(-\unicode[STIX]{x1D719}_{t}(z))$, one easily sees, by analytic continuation, that
$u_{t}(z)=h(-\unicode[STIX]{x1D719}_{t}(z))$, one easily sees, by analytic continuation, that ![]() $u_{t}$ is one-to-one from
$u_{t}$ is one-to-one from ![]() $\unicode[STIX]{x1D6FA}_{t}$ onto
$\unicode[STIX]{x1D6FA}_{t}$ onto ![]() $\mathbb{C}\setminus [1,\infty [$. In particular, the identity
$\mathbb{C}\setminus [1,\infty [$. In particular, the identity ![]() $u_{t}(\unicode[STIX]{x1D702}_{t}(z))=h(-z)$ holds for any
$u_{t}(\unicode[STIX]{x1D702}_{t}(z))=h(-z)$ holds for any ![]() $z\in \mathbb{D}$. Moreover, from Proposition 4.2, we have
$z\in \mathbb{D}$. Moreover, from Proposition 4.2, we have
 $$\begin{eqnarray}\displaystyle d(z)=-c(z)-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}+2c(z)\frac{H(0,z)^{2}+b^{2}-a^{2}\left({\displaystyle \frac{1-z}{1+z}}\right)^{2}}{c(z)+{\displaystyle \frac{1+z}{1-z}}\sqrt{c(z)}H(0,z)}. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle d(z)=-c(z)-\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}+2c(z)\frac{H(0,z)^{2}+b^{2}-a^{2}\left({\displaystyle \frac{1-z}{1+z}}\right)^{2}}{c(z)+{\displaystyle \frac{1+z}{1-z}}\sqrt{c(z)}H(0,z)}. & & \displaystyle \nonumber\end{eqnarray}$$ Since ![]() $c(z)$ is an analytic function in
$c(z)$ is an analytic function in ![]() $\mathbb{D}$ (with values in
$\mathbb{D}$ (with values in ![]() $\mathbb{C}\setminus ]\infty ,0]$) and since
$\mathbb{C}\setminus ]\infty ,0]$) and since
does not vanish in the closed unit disc, we deduce that ![]() $d(z)$ is analytic on
$d(z)$ is analytic on ![]() $\mathbb{D}$ and therefore the function
$\mathbb{D}$ and therefore the function ![]() $u_{t}(z)$ is meromorphic on
$u_{t}(z)$ is meromorphic on ![]() $\mathbb{D}$.
$\mathbb{D}$.
5 Consequences
In this section, we derive some consequences of our preceding results on the regularity of the boundary of ![]() $\unicode[STIX]{x1D6FA}_{t}=\unicode[STIX]{x1D702}_{t}(\mathbb{D})$ and the spectra of
$\unicode[STIX]{x1D6FA}_{t}=\unicode[STIX]{x1D702}_{t}(\mathbb{D})$ and the spectra of ![]() $RU_{t}SU_{t}^{\ast }$. Observe from the equality
$RU_{t}SU_{t}^{\ast }$. Observe from the equality ![]() $\unicode[STIX]{x1D702}_{t}(\overline{z})=\overline{\unicode[STIX]{x1D702}_{t}(z)}$ that
$\unicode[STIX]{x1D702}_{t}(\overline{z})=\overline{\unicode[STIX]{x1D702}_{t}(z)}$ that ![]() $\unicode[STIX]{x1D6FA}_{t}$ is symmetric with respect to the real axis. Denote by
$\unicode[STIX]{x1D6FA}_{t}$ is symmetric with respect to the real axis. Denote by ![]() $\mathbb{D}^{+}$ and
$\mathbb{D}^{+}$ and ![]() $\mathbb{D}^{-}$ the upper and lower parts of
$\mathbb{D}^{-}$ the upper and lower parts of ![]() $\mathbb{D}$ and let
$\mathbb{D}$ and let ![]() $\tilde{h}$ be the restriction of
$\tilde{h}$ be the restriction of ![]() $h$ to the region
$h$ to the region ![]() $\mathbb{D}^{+}\cup (-1,1)$. Then,
$\mathbb{D}^{+}\cup (-1,1)$. Then, ![]() $\tilde{h}$ has a one-to-one continuation to the boundary
$\tilde{h}$ has a one-to-one continuation to the boundary ![]() $\unicode[STIX]{x2202}\mathbb{T}^{+}\cup \{\pm 1\}$ onto
$\unicode[STIX]{x2202}\mathbb{T}^{+}\cup \{\pm 1\}$ onto ![]() $[1,\infty ]$. Henceforth, we shall keep the same notation
$[1,\infty ]$. Henceforth, we shall keep the same notation ![]() $\tilde{h}$ for the continuous extension of the restriction of
$\tilde{h}$ for the continuous extension of the restriction of ![]() $h$ to
$h$ to ![]() $\mathbb{D}^{+}\cup (-1,1)$. Set
$\mathbb{D}^{+}\cup (-1,1)$. Set
From Remark 4.4, this function is meromorphic on ![]() $\mathbb{D}$ and its restriction to
$\mathbb{D}$ and its restriction to ![]() $\unicode[STIX]{x1D6FA}_{t}$ coincides with the conformal map
$\unicode[STIX]{x1D6FA}_{t}$ coincides with the conformal map ![]() $\unicode[STIX]{x1D719}_{t}$. Let
$\unicode[STIX]{x1D719}_{t}$. Let
be the analyticity region of ![]() $\unicode[STIX]{x1D6F7}_{t}$. Then, the region
$\unicode[STIX]{x1D6F7}_{t}$. Then, the region ![]() $\unicode[STIX]{x1D6FA}_{t}$ coincides with the connected component of
$\unicode[STIX]{x1D6FA}_{t}$ coincides with the connected component of ![]() $\unicode[STIX]{x1D6F4}_{t}$ containing the origin
$\unicode[STIX]{x1D6F4}_{t}$ containing the origin ![]() $z=0$. The ODE (4.7) can easily be transformed into
$z=0$. The ODE (4.7) can easily be transformed into
Which is nothing less than the ODE (4.3) (the radial Loewner ODE driven by ![]() $\unicode[STIX]{x1D708}_{t}$). Moreover, it holds for any
$\unicode[STIX]{x1D708}_{t}$). Moreover, it holds for any ![]() $z\in \unicode[STIX]{x1D6F4}_{t}$. The next proposition proves that the inverse
$z\in \unicode[STIX]{x1D6F4}_{t}$. The next proposition proves that the inverse ![]() $\unicode[STIX]{x1D702}_{t}$ of the restriction of
$\unicode[STIX]{x1D702}_{t}$ of the restriction of ![]() $\unicode[STIX]{x1D6F7}_{t}$ to
$\unicode[STIX]{x1D6F7}_{t}$ to ![]() $\unicode[STIX]{x1D6FA}_{t}$ extends continuously to the boundary
$\unicode[STIX]{x1D6FA}_{t}$ extends continuously to the boundary ![]() $\unicode[STIX]{x2202}\mathbb{D}$.
$\unicode[STIX]{x2202}\mathbb{D}$.
(1)
 $\unicode[STIX]{x1D702}_{t}$ extends continuously to
$\unicode[STIX]{x1D702}_{t}$ extends continuously to  $\unicode[STIX]{x2202}\mathbb{D}$ and
$\unicode[STIX]{x2202}\mathbb{D}$ and  $\unicode[STIX]{x1D702}_{t}$ is one-to-one on
$\unicode[STIX]{x1D702}_{t}$ is one-to-one on  $\overline{\mathbb{D}}$.
$\overline{\mathbb{D}}$.(2)
 $\unicode[STIX]{x1D6FA}_{t}$ is a simply connected domain bounded by a simple closed curve.
$\unicode[STIX]{x1D6FA}_{t}$ is a simply connected domain bounded by a simple closed curve.
Proof. Since ![]() $\unicode[STIX]{x1D702}_{t}$ is a conformal map with image
$\unicode[STIX]{x1D702}_{t}$ is a conformal map with image ![]() $\unicode[STIX]{x1D6FA}_{t}$, and its inverse is the restriction of
$\unicode[STIX]{x1D6FA}_{t}$, and its inverse is the restriction of ![]() $\unicode[STIX]{x1D6F7}_{t}$ to
$\unicode[STIX]{x1D6F7}_{t}$ to ![]() $\unicode[STIX]{x1D6FA}_{t}$ then, it suffices to prove that the function
$\unicode[STIX]{x1D6FA}_{t}$ then, it suffices to prove that the function ![]() $\unicode[STIX]{x1D6F7}_{t}$ satisfies the properties in [Reference Belinschi and Bercovici1, Theorem 4.4]. It is not difficult to see that
$\unicode[STIX]{x1D6F7}_{t}$ satisfies the properties in [Reference Belinschi and Bercovici1, Theorem 4.4]. It is not difficult to see that ![]() $\unicode[STIX]{x1D6F7}_{t}(0)=\unicode[STIX]{x1D719}_{t}(0)=0$. Moreover, for any
$\unicode[STIX]{x1D6F7}_{t}(0)=\unicode[STIX]{x1D719}_{t}(0)=0$. Moreover, for any ![]() $z\in \unicode[STIX]{x1D6F4}_{t}$, we have
$z\in \unicode[STIX]{x1D6F4}_{t}$, we have
where the equality follows from the integration of the ODE (5.1) and the inequality is due to the fact that ![]() $\Re H>0$. Otherwise (i.e., if
$\Re H>0$. Otherwise (i.e., if ![]() $z$ is in the complementary set of
$z$ is in the complementary set of ![]() $\unicode[STIX]{x1D6F4}_{t}$ in
$\unicode[STIX]{x1D6F4}_{t}$ in ![]() $\mathbb{D}$), we have
$\mathbb{D}$), we have ![]() $u_{t}(z)\in [1,\infty [$. It follows that,
$u_{t}(z)\in [1,\infty [$. It follows that, ![]() $\tilde{h}^{-1}(u_{t}(z))\in \mathbb{T}$ and then
$\tilde{h}^{-1}(u_{t}(z))\in \mathbb{T}$ and then ![]() $|\unicode[STIX]{x1D6F7}_{t}(z)|=1>|z|$. Consequently,
$|\unicode[STIX]{x1D6F7}_{t}(z)|=1>|z|$. Consequently, ![]() $|\unicode[STIX]{x1D6F7}_{t}(z)|\geqslant |z|$ for any
$|\unicode[STIX]{x1D6F7}_{t}(z)|\geqslant |z|$ for any ![]() $z\in \mathbb{D}$, and therefore assertions (1) and (2) follow immediately from [Reference Belinschi and Bercovici1, Theorem 4.4 and Proposition 4.5].◻
$z\in \mathbb{D}$, and therefore assertions (1) and (2) follow immediately from [Reference Belinschi and Bercovici1, Theorem 4.4 and Proposition 4.5].◻
The following lemma shows that the boundary of ![]() $\unicode[STIX]{x1D6FA}_{t}$ is at a positive distance from
$\unicode[STIX]{x1D6FA}_{t}$ is at a positive distance from ![]() $\pm 1$.
$\pm 1$.
Lemma 5.2. The region ![]() $\overline{\unicode[STIX]{x1D6FA}_{t}}$ does not contain 1 (resp.
$\overline{\unicode[STIX]{x1D6FA}_{t}}$ does not contain 1 (resp. ![]() $-1$) whenever
$-1$) whenever ![]() $b>0$ or
$b>0$ or ![]() $b=0$ and
$b=0$ and ![]() $\unicode[STIX]{x1D708}_{0}\{0\}>0$ (resp.
$\unicode[STIX]{x1D708}_{0}\{0\}>0$ (resp. ![]() $a>0$ or
$a>0$ or ![]() $a=0$ and
$a=0$ and ![]() $\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}>0$).
$\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}>0$).
Proof. Since ![]() $\unicode[STIX]{x1D708}_{0}\{0\}\geqslant b$ (see Corollary 3.7), by the assumption
$\unicode[STIX]{x1D708}_{0}\{0\}\geqslant b$ (see Corollary 3.7), by the assumption ![]() $b>0$ or
$b>0$ or ![]() $b=0$ and
$b=0$ and ![]() $\unicode[STIX]{x1D708}_{0}\{0\}>0$ we deduce that
$\unicode[STIX]{x1D708}_{0}\{0\}>0$ we deduce that
 $$\begin{eqnarray}\displaystyle \lim _{y\rightarrow +\infty }c(y) & = & \displaystyle \lim _{y\rightarrow +\infty }\left[F(0,y)-by-\frac{a}{y}\right]\left[F(0,y)+by+\frac{a}{y}\right]+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}\nonumber\\ \displaystyle & = & \displaystyle +\infty .\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \lim _{y\rightarrow +\infty }c(y) & = & \displaystyle \lim _{y\rightarrow +\infty }\left[F(0,y)-by-\frac{a}{y}\right]\left[F(0,y)+by+\frac{a}{y}\right]+\max \{\unicode[STIX]{x1D6FC}^{2},\unicode[STIX]{x1D6FD}^{2}\}\nonumber\\ \displaystyle & = & \displaystyle +\infty .\nonumber\end{eqnarray}$$Moreover, from the equality (see Proposition 4.2)
we see that,
As a result,
 $$\begin{eqnarray}\displaystyle w_{t}(y)=\sqrt{\frac{\left(b^{2}-a^{2}-c(y)+d(y)e^{t\sqrt{c(y)}}\right)^{2}-4a^{2}c(y)}{\left(b^{2}-a^{2}+c(y)+d(y)e^{t\sqrt{c(y)}}\right)^{2}-4b^{2}c(y)}} & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle w_{t}(y)=\sqrt{\frac{\left(b^{2}-a^{2}-c(y)+d(y)e^{t\sqrt{c(y)}}\right)^{2}-4a^{2}c(y)}{\left(b^{2}-a^{2}+c(y)+d(y)e^{t\sqrt{c(y)}}\right)^{2}-4b^{2}c(y)}} & & \displaystyle \nonumber\end{eqnarray}$$ converges to 1 when ![]() $y$ goes to
$y$ goes to ![]() $+\infty$. Equivalently, in the
$+\infty$. Equivalently, in the ![]() $z$-variable we have,
$z$-variable we have, ![]() $\lim _{z\rightarrow 1^{-}}\unicode[STIX]{x1D719}_{t}(z)=0$ (see Proposition 4.2). Proceeding in the same way, we prove that
$\lim _{z\rightarrow 1^{-}}\unicode[STIX]{x1D719}_{t}(z)=0$ (see Proposition 4.2). Proceeding in the same way, we prove that ![]() $\lim _{z\rightarrow -1^{+}}\unicode[STIX]{x1D719}_{t}(z)=0$. Note that, in this case,
$\lim _{z\rightarrow -1^{+}}\unicode[STIX]{x1D719}_{t}(z)=0$. Note that, in this case, ![]() $\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}\geqslant a$ and the assumption
$\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}\geqslant a$ and the assumption ![]() $a>0$ or
$a>0$ or ![]() $a=0$ and
$a=0$ and ![]() $\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}>0$ implies that
$\unicode[STIX]{x1D708}_{0}\{\unicode[STIX]{x1D70B}\}>0$ implies that ![]() $\lim _{y\rightarrow 0}c(y)=+\infty$ and
$\lim _{y\rightarrow 0}c(y)=+\infty$ and ![]() $\lim _{y\rightarrow 0}d(y)/c(y)=1$. Since
$\lim _{y\rightarrow 0}d(y)/c(y)=1$. Since ![]() $\unicode[STIX]{x1D719}_{t}(0)=0$ and
$\unicode[STIX]{x1D719}_{t}(0)=0$ and ![]() $\unicode[STIX]{x1D6FA}_{t}$ is a simply connected domain bounded by a simple closed curve, we see that
$\unicode[STIX]{x1D6FA}_{t}$ is a simply connected domain bounded by a simple closed curve, we see that ![]() $\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA}_{t}$ intersect
$\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA}_{t}$ intersect ![]() $x$-axis at two points
$x$-axis at two points ![]() $x(t)_{\pm }$ from either side of the origin, with
$x(t)_{\pm }$ from either side of the origin, with ![]() $\unicode[STIX]{x1D719}_{t}(x(t)_{\pm })=\pm 1$. From
$\unicode[STIX]{x1D719}_{t}(x(t)_{\pm })=\pm 1$. From ![]() $\lim _{z\rightarrow \pm 1^{\mp }}\unicode[STIX]{x1D719}_{t}(z)=0$, we deduce that
$\lim _{z\rightarrow \pm 1^{\mp }}\unicode[STIX]{x1D719}_{t}(z)=0$, we deduce that ![]() $[x(t)_{-},x(t)_{+}]\subset (-1,1)$.◻
$[x(t)_{-},x(t)_{+}]\subset (-1,1)$.◻
Corollary 5.3. For any ![]() $t>0$,
$t>0$,
is a function of Hardy class ![]() $H^{\infty }(\mathbb{D})$.
$H^{\infty }(\mathbb{D})$.
Proof. By the first item of Proposition 5.1, we can easily confirm that ![]() $\unicode[STIX]{x1D702}_{t}$ is of hardy class
$\unicode[STIX]{x1D702}_{t}$ is of hardy class ![]() $H^{\infty }(\mathbb{D})$ and hence the function
$H^{\infty }(\mathbb{D})$ and hence the function
is of hardy class ![]() $H^{\infty }(\mathbb{D})$ by the previous Lemma, thanks to the fact that
$H^{\infty }(\mathbb{D})$ by the previous Lemma, thanks to the fact that ![]() $\unicode[STIX]{x1D702}_{t}$ cannot take the values
$\unicode[STIX]{x1D702}_{t}$ cannot take the values ![]() $\pm 1$ in
$\pm 1$ in ![]() $\mathbb{D}$.◻
$\mathbb{D}$.◻
We close this paragraph with the following result on the spectra of ![]() $RU_{t}SU_{t}^{\ast }$.
$RU_{t}SU_{t}^{\ast }$.
Proposition 5.4. For every ![]() $t>0$, 0 and
$t>0$, 0 and ![]() $\unicode[STIX]{x1D70B}$ does not belong to the continuous singular spectrum of
$\unicode[STIX]{x1D70B}$ does not belong to the continuous singular spectrum of ![]() $\unicode[STIX]{x1D70E}_{t}$.
$\unicode[STIX]{x1D70E}_{t}$.
Proof. By the second item in Proposition 5.1 and the subordination relation (4.2), the function ![]() $K(t,.)$ has an analytic continuation in some neighborhoods of
$K(t,.)$ has an analytic continuation in some neighborhoods of ![]() $\pm 1$ and
$\pm 1$ and
where ![]() $x(t)_{\pm }$ are the real boundaries of
$x(t)_{\pm }$ are the real boundaries of ![]() $\unicode[STIX]{x1D6FA}_{t}$ (see the proof of Lemma 5.2). Let
$\unicode[STIX]{x1D6FA}_{t}$ (see the proof of Lemma 5.2). Let ![]() $L(t,z)$ be as in Corollary 3.5, and rewrite (4.1) as
$L(t,z)$ be as in Corollary 3.5, and rewrite (4.1) as
Then, we have
Since
blows up as ![]() $z\rightarrow \pm 1^{\mp }$, we deduce that
$z\rightarrow \pm 1^{\mp }$, we deduce that ![]() $\lim _{z\rightarrow \pm 1^{\mp }}L(t,z)=0$. Consequently, the Poisson transform of
$\lim _{z\rightarrow \pm 1^{\mp }}L(t,z)=0$. Consequently, the Poisson transform of ![]() $\unicode[STIX]{x1D70E}_{t}$, which is nothing but the real part of
$\unicode[STIX]{x1D70E}_{t}$, which is nothing but the real part of ![]() $L(t,z)$, vanishes as
$L(t,z)$, vanishes as ![]() $z\rightarrow \pm 1^{\mp }$ and hence the desired assertion follows from Proposition 1.3.11 and equation (1.8.8) in [Reference Cima, Matheson and Ross3].◻
$z\rightarrow \pm 1^{\mp }$ and hence the desired assertion follows from Proposition 1.3.11 and equation (1.8.8) in [Reference Cima, Matheson and Ross3].◻
6 Free mutual information and orbital free entropy
Here is our main application to the proof of the conjecture ![]() $i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$. For a pair of projections
$i^{\ast }=-\unicode[STIX]{x1D712}_{\text{orb}}$. For a pair of projections ![]() $(P,Q)$, we use the same definitions of the free mutual information
$(P,Q)$, we use the same definitions of the free mutual information ![]() $i^{\ast }(\mathbb{C}P+\mathbb{C}(I-P);\mathbb{C}Q+\mathbb{C}(I-Q))$ (hereafter
$i^{\ast }(\mathbb{C}P+\mathbb{C}(I-P);\mathbb{C}Q+\mathbb{C}(I-Q))$ (hereafter ![]() $i^{\ast }(P:Q)$) and the orbital free entropy
$i^{\ast }(P:Q)$) and the orbital free entropy ![]() $\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ as expounded in the last section of the paper [Reference Izumi and Ueda15]. We refer the reader to [Reference Hiai and Petz13, Reference Hiai and Ueda14, Reference Voiculescu19] for more information. Using subordination technology, a partial result for the identity
$\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ as expounded in the last section of the paper [Reference Izumi and Ueda15]. We refer the reader to [Reference Hiai and Petz13, Reference Hiai and Ueda14, Reference Voiculescu19] for more information. Using subordination technology, a partial result for the identity ![]() $i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ is obtained in [Reference Izumi and Ueda15, Lemma 4.4] (note that the function
$i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ is obtained in [Reference Izumi and Ueda15, Lemma 4.4] (note that the function ![]() $H$ there is exactly our
$H$ there is exactly our ![]() $\frac{1}{4}K^{2}$). The result is as follows.
$\frac{1}{4}K^{2}$). The result is as follows.
Lemma 6.1. [Reference Izumi and Ueda15]
If ![]() $K(t,.)$ define a function of Hardy class
$K(t,.)$ define a function of Hardy class ![]() $H^{3}(\mathbb{D})$ for any
$H^{3}(\mathbb{D})$ for any ![]() $t>0$, then
$t>0$, then ![]() $i^{\ast }\left(\mathbb{C}P+\mathbb{C}(I-P);\mathbb{C}Q+\mathbb{C}(I-Q)\right)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
$i^{\ast }\left(\mathbb{C}P+\mathbb{C}(I-P);\mathbb{C}Q+\mathbb{C}(I-Q)\right)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
From
the assumption in Lemma 6.1 implies that ![]() $\unicode[STIX]{x1D70E}_{t}$ has an
$\unicode[STIX]{x1D70E}_{t}$ has an ![]() $L^{3}$-density. The converse remains true; that is, if
$L^{3}$-density. The converse remains true; that is, if ![]() $\unicode[STIX]{x1D70E}_{t}$ has an
$\unicode[STIX]{x1D70E}_{t}$ has an ![]() $L^{3}$-density for any
$L^{3}$-density for any ![]() $t>0$, then
$t>0$, then ![]() $K(t,.)$ becomes a function of Hardy class
$K(t,.)$ becomes a function of Hardy class ![]() $H^{3}(\mathbb{D})$. In fact, according to [Reference Conway5, Theorem 1.7, p. 208],
$H^{3}(\mathbb{D})$. In fact, according to [Reference Conway5, Theorem 1.7, p. 208], ![]() $L(t,.)$ is a function of Hardy class
$L(t,.)$ is a function of Hardy class ![]() $H^{3}(\mathbb{D})$. On the other hand, from Corollary 3.7 and Proposition 5.4, we see that
$H^{3}(\mathbb{D})$. On the other hand, from Corollary 3.7 and Proposition 5.4, we see that ![]() $L(t,.)$ has an analytic continuation across both points
$L(t,.)$ has an analytic continuation across both points ![]() $\pm 1$. Moreover, the limit
$\pm 1$. Moreover, the limit ![]() $\lim _{z\rightarrow \pm 1}L(t,z)=0$ implies that the constant term in the power series expansion around
$\lim _{z\rightarrow \pm 1}L(t,z)=0$ implies that the constant term in the power series expansion around ![]() $z=\pm 1$ is zero. So that
$z=\pm 1$ is zero. So that ![]() $L(t,z)(a((1-z)/(1+z))+b((1+z)/(1-z)))$ is bounded in some neighborhoods at both
$L(t,z)(a((1-z)/(1+z))+b((1+z)/(1-z)))$ is bounded in some neighborhoods at both ![]() $\pm 1$. Hence
$\pm 1$. Hence
becomes a function of Hardy class ![]() $H^{3/2}(\mathbb{D})$. From these discussions, we deduce that
$H^{3/2}(\mathbb{D})$. From these discussions, we deduce that
Lemma 6.2. If ![]() $\unicode[STIX]{x1D70E}_{t}$ has an
$\unicode[STIX]{x1D70E}_{t}$ has an ![]() $L^{3}$-density for every
$L^{3}$-density for every ![]() $t>0$, then
$t>0$, then ![]() $i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
$i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
Here we reprove the same result by an equivalent but more handy assumption.
Proposition 6.3. Assume that for every ![]() $t>0$,
$t>0$, ![]() $H(0,\unicode[STIX]{x1D702}_{t}(.))$ is a function of Hardy class
$H(0,\unicode[STIX]{x1D702}_{t}(.))$ is a function of Hardy class ![]() $H^{3}(\mathbb{D})$. Then the equality
$H^{3}(\mathbb{D})$. Then the equality ![]() $i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ holds.
$i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$ holds.
Proof. We will prove that the assumptions ![]() $H(0,\unicode[STIX]{x1D702}_{t}(.))\in H^{3}(\mathbb{D})$ and
$H(0,\unicode[STIX]{x1D702}_{t}(.))\in H^{3}(\mathbb{D})$ and ![]() $K(t,.)\in H^{3}(\mathbb{D})$ are equivalent and so we can use the result of Lemma 6.1. To this end, we use the subordination relation (4.2) to rewrite (4.1) as
$K(t,.)\in H^{3}(\mathbb{D})$ are equivalent and so we can use the result of Lemma 6.1. To this end, we use the subordination relation (4.2) to rewrite (4.1) as
But, the function (see Corollary 5.3)
is of hardy class ![]() $H^{\infty }(\mathbb{D})$. Hence we are done.◻
$H^{\infty }(\mathbb{D})$. Hence we are done.◻
Here is a sample application of Proposition 6.3 improving the result in [Reference Izumi and Ueda15, Corollary 4.5].
Lemma 6.4. Assume that ![]() $\unicode[STIX]{x1D70E}_{0}$ has an
$\unicode[STIX]{x1D70E}_{0}$ has an ![]() $L^{3}$-density with respect to
$L^{3}$-density with respect to ![]() $d\unicode[STIX]{x1D703}$. Then
$d\unicode[STIX]{x1D703}$. Then ![]() $i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
$i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
Proof. Under the assumption here and according to [Reference Conway5, Theorem 1.7, p. 208], ![]() $L(0,z)$ is a function of Hardy class
$L(0,z)$ is a function of Hardy class ![]() $H^{3}(\mathbb{D})$ and hence so does
$H^{3}(\mathbb{D})$ and hence so does ![]() $L(0,\unicode[STIX]{x1D702}_{t}(z))$ too by Littlewood’s subordination theorem (see [Reference Duren9, Theorem 1.7]). Hence, by Corollary 5.3, the function
$L(0,\unicode[STIX]{x1D702}_{t}(z))$ too by Littlewood’s subordination theorem (see [Reference Duren9, Theorem 1.7]). Hence, by Corollary 5.3, the function
is of hardy class ![]() $H^{3}(\mathbb{D})$ and then we are done thanks to Proposition 6.3.◻
$H^{3}(\mathbb{D})$ and then we are done thanks to Proposition 6.3.◻
We can now prove the main result of this section.
Theorem 6.5. For any two projections ![]() $P,Q$, if
$P,Q$, if
has an ![]() $L^{3}$-density with respect to
$L^{3}$-density with respect to ![]() $x(1-x)\,dx$ on
$x(1-x)\,dx$ on ![]() $[0,1]$ for
$[0,1]$ for ![]() $t=0$ or every
$t=0$ or every ![]() $t>0$, then
$t>0$, then ![]() $i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
$i^{\ast }(P:Q)=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.
Proof. From the relationship ![]() $\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$ and the assumption here (together with Remark 3.4), we deduce that
$\unicode[STIX]{x1D707}_{t}\leftrightsquigarrow \unicode[STIX]{x1D708}_{t}$ and the assumption here (together with Remark 3.4), we deduce that
has an ![]() $L^{3}$-density with respect to
$L^{3}$-density with respect to ![]() $d\unicode[STIX]{x1D703}$ on
$d\unicode[STIX]{x1D703}$ on ![]() $\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ for
$\mathbb{T}=(-\unicode[STIX]{x1D70B},\unicode[STIX]{x1D70B}]$ for ![]() $t=0$ or every
$t=0$ or every ![]() $t>0$ and hence by (3.5), the measure
$t>0$ and hence by (3.5), the measure ![]() $\unicode[STIX]{x1D70E}_{t}$ does so also. The desired identity immediately follows from Lemmas 6.2 and 6.4.◻
$\unicode[STIX]{x1D70E}_{t}$ does so also. The desired identity immediately follows from Lemmas 6.2 and 6.4.◻