Material and Methods

Smallflower umbrella sedge seeds were collected from 15 rice fields throughout California’s Northern Sacramento Valley (39º27′N; 121º48′W). Ten plants were randomly selected per rice field, and seeds were cleaned and dry stored at 7 C until used in germination tests. Whole-plant assays conducted using bensulfuron-methyl demonstrated that the seed set comprised mostly ALS-inhibitor resistant seeds (data not shown). Before germination tests, seeds were placed in containers filled with de-ionized water and stored under dark conditions at 10 C for 2 months to break dormancy by simulating overwintering conditions in California rice fields (Baskin and Baskin Reference Baskin and Baskin2001; Boddy et al. Reference Boddy, Bradford and Fischer2012). The cold-stratification procedure was repeated a month later for a second run of germination tests, allowing for the analysis of germination times as displayed by the nondormant fraction of the seed population.

Germination tests were carried out on a one-dimensional thermogradient table set at constant temperatures of 11.7, 13.2, 21.0, 24.5, 29.7, 33.5, 36.0, and 41.7 C, using a Conviron® CMP3244 unit (Controlled Environments Inc., Temecula, CA) as a heater and a VWR® Scientific 1171MD refrigerated chiller (VWR Scientific Products, Tempe, AZ). Approximately 50 seeds were placed in 3.5-cm–diameter petri dishes with two Whatman No. 1 filter paper discs. Approximately 2.0 mL of de-ionized water were added to each petri dish for filter paper saturation. The dish was then held on its side to drain excess water and avoid formation of a film of water around the seeds, thereby providing an aerobic environment for seed germination. Dishes were fitted with covers and sealed with Parafilm (Bemis Company, Inc, Neenah, WI) to prevent evaporation. Under fluorescent lights, seeds were exposed to a 14-h photoperiod at 18 µmol m⁻² s⁻¹ photosynthetic photon-flux density, an appropriate level for seed germination studies (Baskin and Baskin Reference Baskin and Baskin2001). Three replicate dishes containing 50 seeds each were placed within each isothermal lane on the thermogradient table. Because of their minute size, seeds were viewed under a microscope at ×10 magnification. Coleoptile protrusion of 0.5 mm was used as the germination criterion. Germinated seeds were counted and removed every 24 h over 30 d; water in dishes was replenished whenever needed, using isothermal water kept within each temperature lane for this purpose. It was assumed that 30 d was sufficient time for nondormant seeds to germinate.

The probit regression analysis shown in equation 3 was used to estimate thermal-time model parameters from the pool of observed germination data collected at the suboptimal temperature range of 21 to 33.5 C, given that such suboptimal incubation temperatures allowed for final germination greater than 50%, as required (Bradford Reference Bradford1990). Three sets of model parameters were derived by replication using the Solver tool in Microsoft Excel^® (Microsoft Corp., Redmond, WA) to minimize the root-mean-square error between observed and simulated germination data (Huarte and Benech-Arnold Reference Huarte and Benech-Arnold2010). Model parameters were subjected to ANOVA after Box-Cox transformation to meet assumptions (JMP 8.0 software; SAS Institute, Inc., Cary, NC), and comparisons were made across both germination trials. Using these parameters, the original germination time courses were reproduced as cumulative normal curves of the following function:

(4) $$G = {\rm{ }}\left[ {{\rm{log}}\,{t_{\rm{g}}}-{\rm{ }}\left( {{\rm{log}}\,{\theta _{\rm{T}}}\left( {{\rm{5}}0} \right){\rm{ }}-{\rm{ log }}\left( {T-{T_{\rm{b}}}} \right)} \right)} \right]{\rm{ }}{\sigma_\vartheta }_{\rm{T}}$$

where G is cumulative germination percentage (Bradford Reference Bradford1990). Time (in days) to median germination (t₅₀) was estimated by nonlinear regression analysis using the NLIN procedure in SAS (Steinmaus et al. Reference Steinmaus, Prather and Holt2000). GR ₅₀, median germination rates calculated as 1/t₅₀ and expressed in days, were estimated for each incubation temperature and germination test. GR ₅₀ values were plotted against test temperatures to estimate T_o (Boddy et al. Reference Boddy, Bradford and Fischer2012). Data are reported as mean ± SE.

Results and Discussion

Smallflower umbrella sedge seed viability was high throughout both runs of germination tests (Table 1). Final germination percentages for test temperatures between 21.0 C and 36.0 C were similar across experiments and averaged 82.0% ± 1.3% and 85.4% ± 1.7% for the first and second runs, respectively, suggesting that significant primary dormancy was either not present or had been substantially removed from the seed set during the stratification period. These results agree with findings by Chauhan and Johnson (Reference Chauhan and Johnson2009), Derakhshan and Gherekhloo (Reference Derakhshan and Gherekhloo2013), and Kim and Mercado (Reference Kim and Mercado1987), despite the use of nonstratified seeds in germination tests carried out by those authors.

Table 1. Final smallflower umbrella sedge seed germination.

^a Abbreviations: θ _T(50), SD in thermal time within the seed population; σ_{θ T(50)}, thermal time constant to median germination; Cd, degree-days; G, germination percentage;T_b, base temperature.

^b P values were obtained following ANOVA on each parameter at an α level of 0.05; n = 30. None was statistically significant.

^c Average percentage of total germinated seeds placed at temperatures between 21 C and 33.5 C.

^d Parameters are derived from equation 3.

^e θ _T(50) is presented as 10^θ T(50).

Seed germination did not take place at 11.7 C regardless of trial run (Figure 1). GR ₅₀ increased linearly until 33.5 C (Figure 2), suggesting data collected at temperatures of 36.0 ºC or higher were in the supraoptimal range and are inadequate for developing models to predict germination based on thermal-time accumulation (Steinmaus et al. Reference Steinmaus, Prather and Holt2000). These results agree with previous findings that smallflower umbrella sedge germination is favored by temperatures higher than 25 C (Chauhan and Johnson Reference Chauhan and Johnson2009; Derakhshan and Gherekhloo Reference Derakhshan and Gherekhloo2013; Ismail et al. Reference Ismail, Mansor and Rahman2007). There was thermo-inhibition of germination at 41.7 C (Figure 1). A ceiling temperature could not be determined, because germination occurred even at the highest incubation temperature.

Figure 1. Cumulative percentage of smallflower umbrella sedge germination plotted over days after seeding. Data points are averages based on three replicates of approximately 50 seeds each. Assessments were performed for 30 d. (A) First run of germination experiments. (B) Second run of germination, performed 1 month after run 1.

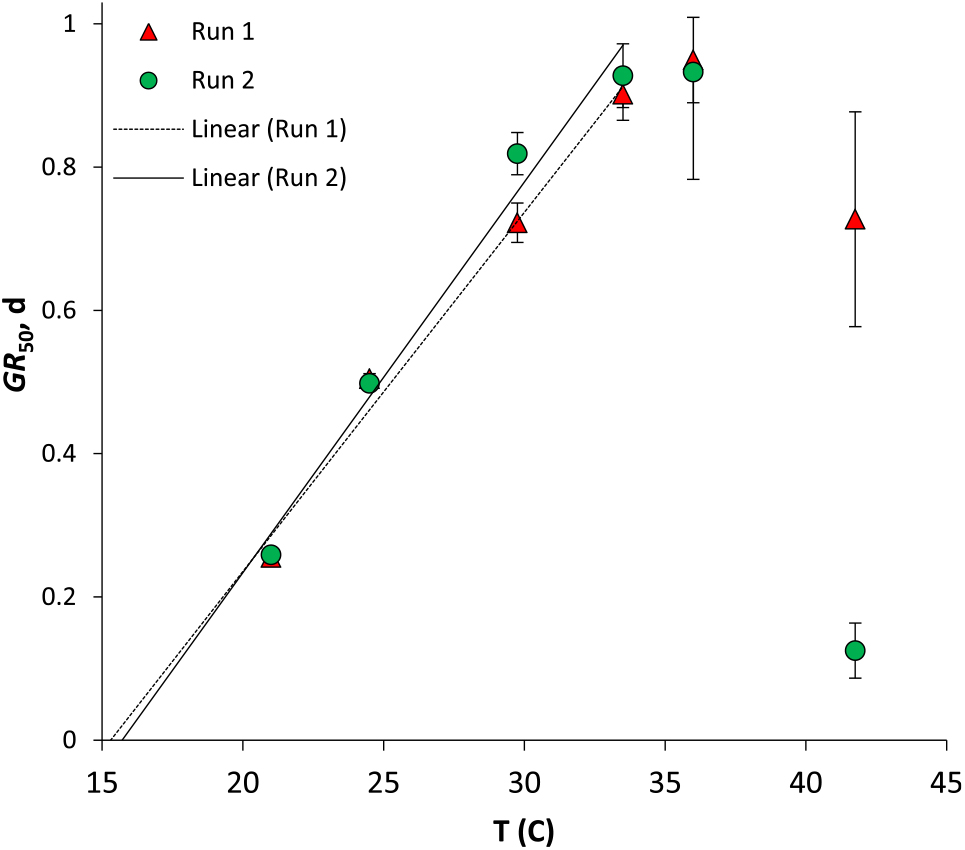
Figure 2. Median germination rates (days) for smallflower umbrella sedge across temperatures of 21 to 41.75 C. Bars represent SE based on three replicates of approximately 50 seeds. R ² > 0.95 for both lines. GR ₅₀, median germination rate; T, temperature.

The thermal-time model parameters Tb, θ _T(50), and σ_{θ T(50)} did not differ across trial runs (Table 1); T_b averaged 16.67 C. This value is slightly higher than that reported by Derakhshan and Gherekhloo (Reference Derakhshan and Gherekhloo2013), which could be related to the model presented in this work being developed using a wider range of test temperatures (Bradford Reference Bradford2002). Variations within a species due to genetic diversity of ecotypes from separate geographic regions can also be expected (Baskin and Baskin Reference Baskin and Baskin2001). Smallflower umbrella sedge θ _T(50) averaged 16.55 ± 0.7 Cd, which is nearly half the θ _T(50) estimated for another troublesome rice weed, late watergrass [Echinochloa oryzicola (Vasinger) Vasinger] (Boddy et al. Reference Boddy, Bradford and Fischer2012). Thermal-time model parameters were used in conjunction with equation 4 to reproduce the original germination time courses plotted against thermal units (Figure 3). Prediction lines fit observed germination and fitting errors were minimized; root-mean-square error values generated during T_b determination were 0.031 and 0.038, an indication of the model’s goodness of fit (Mayer and Butler Reference Mayer and Butler1993; Spokas and Forcella Reference Spokas and Forcella2006). Most smallflower umbrella sedge seeds germinate by 25 Cd (Figure 3). The SD in σ_{θ T(50)} averaged 0.1 Cd, indicating synchronous seed germination, as indicted by the steep slopes in Figure 3. In comparison, σ_{θ T(50)} calculated for late watergrass averaged 1.25 Cd.

Figure 3. Thermal-time model germination curves for smallflower umbrella sedge across four constant temperature regimes at 0 MPa, expressed in Cd. Cumulative observed (symbols) and predicted (dotted line) germination are plotted over thermal units calculated according to parameters in Table 1 and equation 4. Cd, degree-days; GDD, growing degree-days; predic, predicted.

Synchronous smallflower umbrella sedge seed germination constitutes a very desirable trait from a weed-control point of view because it is correlated with more uniform seedling emergence (Forcella et al. Reference Forcella, Arnold, Sanchez and Ghersa2000), which, in turn, could benefit its control using POST herbicides. Moreover, results also indicate that primary seed dormancy is not present in this species, which is corroborated by findings reported in the literature (Chauhan and Johnson Reference Chauhan and Johnson2009; Derakhshan and Gherekhloo Reference Derakhshan and Gherekhloo2013; Kim and Mercado Reference Kim and Mercado1987). This is also desirable from a modeling standpoint, because progressive dormancy alleviation could lead to multiple germination and seedling emergence fluxes (Boddy et al. Reference Boddy, Bradford and Fischer2012).

Thermal-time model parameters presented in this study are expected to aid in conceptualizing smallflower umbrella sedge management tactics in Californian rice fields. Depending on rice seeding date, smallflower umbrella sedge could initiate germination later than rice and some of its key weedy competitors, due to its higher T_b values (Table 2). In Colusa County, a major rice-growing area in California, if rice is seeded in mid-April when soil temperatures average 18 C (UC IPM 2013), models indicate smallflower umbrella sedge germination is completed within 7 d, longer than the 4.5 d needed for late watergrass germination in such conditions. However, if rice is sown 20 d later at optimum seeding date, warmer temperatures would mean germination of both weed species would require only 4 d to be completed.

Table 2. Base temperature for germination of smallflower umbrella sedge and other weedy species in rice, as well as indica and japonica type rice, according to studies in the literature.

^a Abbreviation: T_b, base temperature.

^b Estimated from preliminary germination results; SE values not available.

A small fraction of the seed set was able to germinate at 13.2 C in both experiments (Figure 1), which is below the calculated T_b values. This outcome suggests the presence of different T_b values among certain fractions of the seed set, which mostly comprised ALS-inhibitor–resistant individuals. Mutations at the proline 197 residue within the ALS enzyme endowing resistance to ALS inhibitors have been associated with altered germination at low temperatures, due to an altered enzyme feedback sensitivity, causing the accumulation of branched-chain amino acids in seeds (Dyer et al. Reference Dyer, Chee and Fay1993; Eberlein et al., Reference Eberlein, Guttieri, Berger, Fellman, Mallory-Smith, Thill, Baerg and Belknap1999; Park et al. Reference Park, Mallory-Smith, Ball and Mueller2004). Those individuals germinating at 13.2 C could represent the ALS-inhibitor-resistant fraction displaying a mutated proline 197 residue. Ongoing research efforts are aimed at validating this hypothesis, as well as determining smallflower umbrella sedge germination and emergence time courses in the field to assess potential differences among ALS-inhibitor–resistant and –susceptible populations.